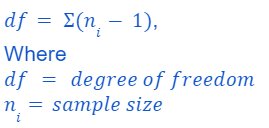t-test : Definition and Example

In this article, we will discuss t-test, types of t-test (one-sample, independent sample, and paired sample) with the help of examples.

Introduction

t-test is a statistical significant test for hypothesis testing

There are 3 steps in Hypothesis Testing:

1. State Null and Alternate Hypothesis
2. Perform Statistical Test
3. Accept and reject the Null Hypothesis

In this article, we will discuss the t-test.

Table of Content

• t-test
  • One-sample t-test
  • Independent (Two-sample) t-test
  • Paired t-test

What is t-test ?

Statistical method for the comparison of the mean of the two groups of the normally distributed sample(s).

It is used when:

• Population parameter (mean and standard deviation) is not known
• Sample size (number of observations) < 30

To know more about normal distribution, read the article Probability Distribution

To know more about mean and variance, To know more about mean and variance, read the article on measures of central tendency and measures of dispersion.

Type of t-test

The T-test is mainly classified into 3 parts:

• One sample 
• Independent sample
• Paired sample

One Sample

In one sample t-test, we compare the sample mean with the population mean.

Mathematical Formula:

• Region of rejection lies either on extreme left or extreme right of the distribution.
• In z-test, we use population standard deviation instead of sample standard deviation.

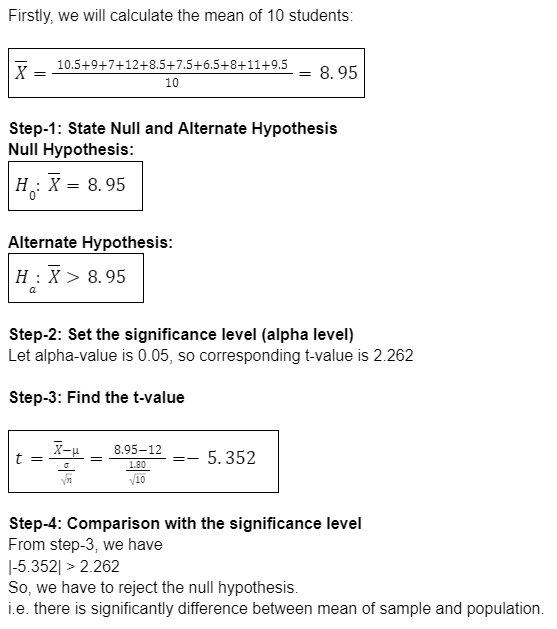
Let’s understand this by an example:

Problem Statement:

Marks of student are 10.5, 9, 7, 12, 8.5, 7.5, 6.5, 8, 11 and 9.5.

Mean population score is 12 and standard deviation is 1.80.

Is the mean value for student significantly differ from the mean population value.

Solution:

Firstly, we will calculate the mean of 10 students:

Independent (two-sample t-test):

In this test, we compare the means of two different samples.

Mathematical Formula:

Degree of Freedom:

Degree of freedom is defined as the the number of independent variables.

It is given by:

Note: There are two regions of rejection, one in either directions towards tail of each distribution.

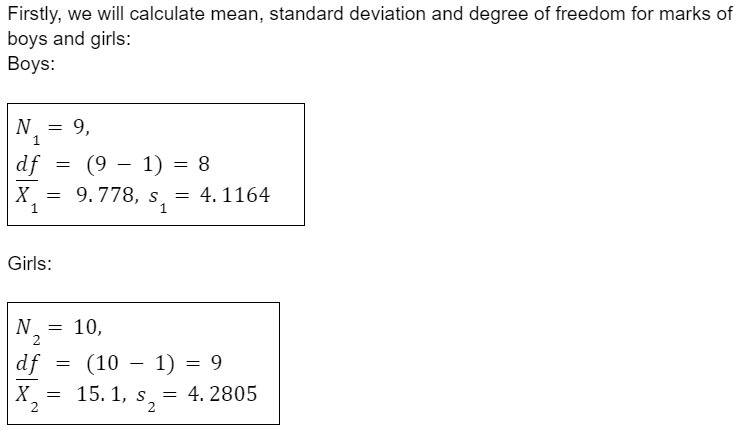
Let’s understand two-sample t-test by an example:

Problem Statement:

The marks of boys and girls are given:

Boys: 12, 14, 10, 8, 16, 5, 3, 9, and 11

Girls: 21, 18, 14, 20, 11, 19, 8, 12, 13, and 15

Is there any significant differnece between marks of males and females i.e. population means are different.

Solution:

Firstly, we will calculate mean, standard deviation and degree of freedom for marks of boys and girls:

Paired t-test:

In this test, we compare the means of two related or same group at two different time.

Mathematical Formula:

Note: Degree of freedom is n-1.

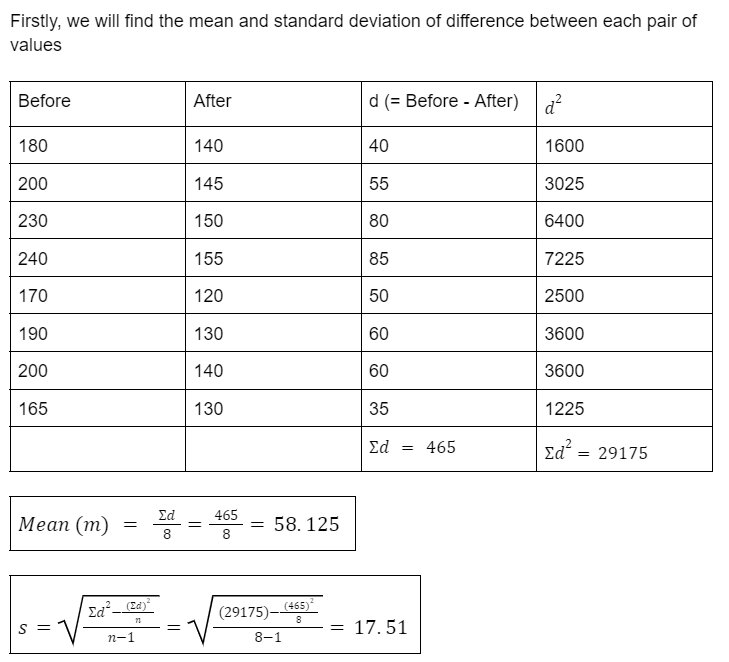
Let’s understand two-sample t-test by an example:

Problem Statement:

Blood pressure of 8 patients are before and after are recorded:

Before: 180, 200, 230, 240, 170, 190, 200, and 165

After: 140, 145, 150, 155, 120, 130, 140, and 130

Is there any significant difference between BP reading before and after.

Solution:

Conclusion:

t-test is a statistically significant test for the hypothesis testing (null and alternative hypotheses) when the sample size is small and the population parameter (mean and variance) is unknown.

Top Trending Articles:
Data Analyst Interview Questions | Data Science Interview Questions | Machine Learning Applications | Big Data vs Machine Learning | Data Scientist vs Data Analyst | How to Become a Data Analyst | Data Science vs. Big Data vs. Data Analytics | What is Data Science | What is a Data Scientist | What is Data Analyst