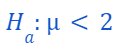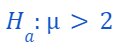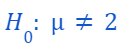z-test : Definition and Example

z-test is a statistical method for the comparison of mean in a sample from the normally distributed population or between two independent samples. In this article we will briefly discuss z-test, types of z-tests with different examples.

z-test is a statistically significant test for Hypothesis Testing. There are 3 steps in Hypothesis Testing:

• State Null and Alternate Hypothesis
• Perform Statistical Test
• Accept and reject the Null Hypothesis

In this article, we will discuss the z-test, the mathematical formula, and how to calculate it with the help of an example.

Must Check: Statistics Interview Questions

Table of Content

• Z-test
  • One-sample z-test
  • Two-sample z-test

What is z-test?

Statistical method for the comparison of mean in a sample from the normally distributed population or between two independent samples

Or 

Statistical test to validate the hypothesis (accept or reject) when the data is normally distributed.

z-test is used when:

• Population variance is unknown
• Sample size is greater than 30

Also Read: Difference between Null Hypothesis and Alternative Hypothesis

Also Read: Difference between One-tailed and Two-Tailed Test

Types of z-test:

Z-test is mainly classified into 2 types:

• One Sample
• Two Sample

One-Sample

• The one-sample test is used when we have to compare a sample mean with the population mean.
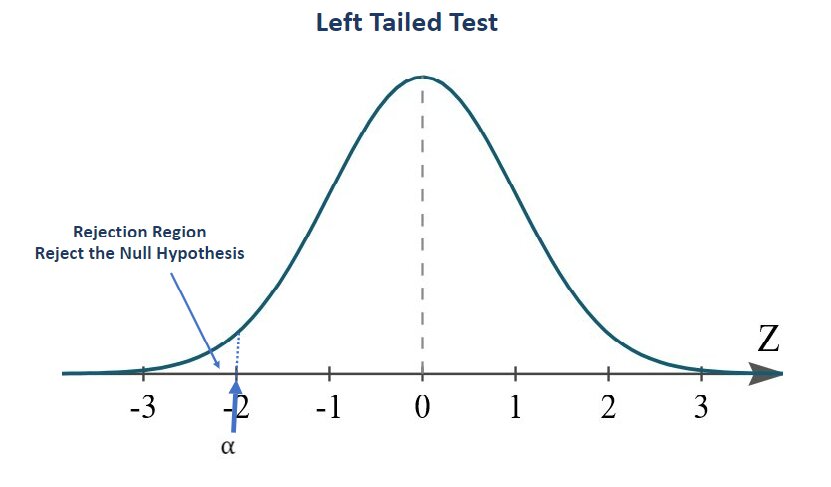
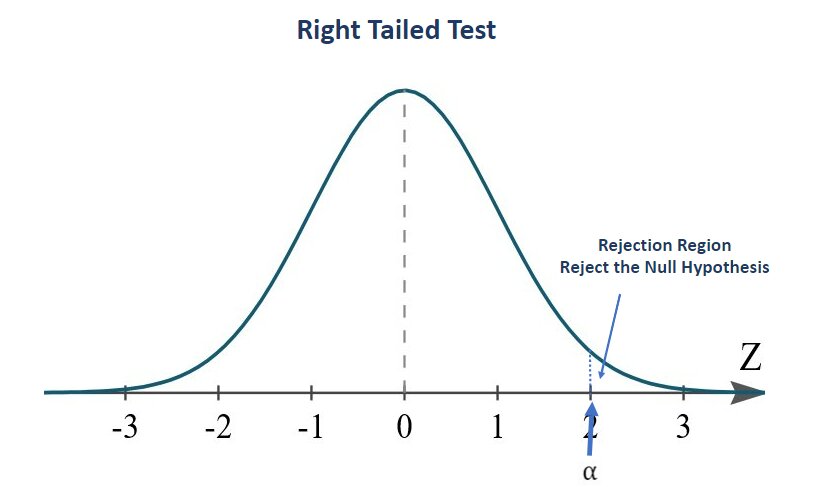
• The region of rejection is located either extreme left or extreme right of the distribution

i.e. if any null hypothesis: Sample mean is 2

Then, its Alternate hypothesis: Sample mean is either greater or less than 2

in this case, the rejection region will be on the left side of the distribution

 

Note: For the left tailed test, the claimed mean sample value for the null hypothesis will be less than or equal to the mean population value.

or

In this case, the rejection region will be on the right side of the distribution.

 

Note: For the right-tailed test, the claimed mean sample value for the null hypothesis will be greater than or equal to the mean population value.

Mathematical Formula:

Also Read: t-test

Also Read: chi-square test

Let’s understand the one-sample z-test by an example:

z-test Example

A gym trainer claimed that all the new boys in the gym are above average weight.

A random sample of thirty boys weight have a mean score of 112.5 kg and the population mean weight is 100 kg and the standard deviation is 15.

Is there a sufficient evidence to support the claim of gym trainer.

Also Read: p-value

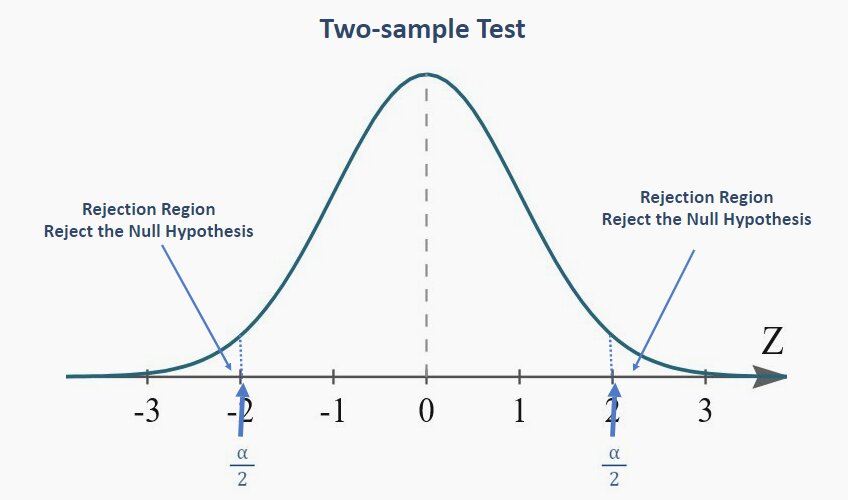
Two-Sample:

• A two-sample test is used when we have to compare the mean of two samples.
• The region of rejection is located on both the extreme (left and right) of the distribution

i.e. if any null hypothesis: Sample mean is 2

Then, its Alternate hypothesis: Sample mean is not equal to 2

 

Note: For two sample test, the claimed value for the null hypothesis will be equal to mean population value.

Mathematical Formula:

Let’s understand the two-sample z-test by an example:

Problem Statement:

Random samples of 75 males and 50 female’s donors yields mean concentration of 28 and 33 ppm respectively. The amount of trace elements in blood varies with the standard deviation 14.1 and 9.5 ppm respectively for males and females. What is the likelihood that the population means of concentration of elements are the same for men and women.

Conclusion:

Z-test is a statistically significant test for the hypothesis testing (null and alternative hypotheses) when the sample size is large, and the population parameter (mean and variance) is known. Hope you will like the article.

Keep Learning!!

Keep Sharing!!