Salviya AntonySenior Executive - Content
Rate of Reaction: The rate of reaction refers to the speed at which reactants are converted into products in a chemical reaction. It is a fundamental concept in the field of chemistry that plays a crucial role in understanding and controlling chemical processes. Understanding the factors that influence reaction rates and the mechanisms underlying them is essential for various scientific, industrial, and environmental applications.
This article will explore the concept of rate of reaction, its importance, and the factors that influence it. In NCERT Class 12 Chemistry, rate of reaction is an important topic. Students learn this topic in chapter Chemical Kinetics.
Factors Influencing the Rate of Reaction
Nature of Reactants:
The nature of reactants significantly affects the rate of reaction. In general, reactions between substances with similar properties tend to be slower than those between substances with different properties. For example, a reaction between two gases may be faster than a reaction between a solid and a liquid because gas molecules have more freedom to collide and react.
Concentration of Reactants:
The concentration of reactants plays a critical role in determining the rate of reaction. According to the collision theory, a higher concentration of reactants results in more frequent collisions between particles, increasing the chances of successful collisions. As a result, the reaction rate typically increases with increasing reactant concentration.
Temperature:
Temperature is a key factor that influences the rate of reaction. According to the Arrhenius equation, as the temperature increases, the rate of reaction also increases. This is because higher temperatures provide reactant molecules with more energy, allowing them to overcome the activation energy barrier and facilitating more successful collisions.
Surface Area:
In reactions involving solid reactants, the surface area of the solid can significantly impact the reaction rate. A larger surface area allows for more contact between the reactant particles, increasing the number of collisions and, subsequently, the rate of reaction. This is particularly relevant in processes like catalysis, where finely divided solid catalysts are used to increase reaction rates.
Catalysts:
Catalysts are substances that can increase the rate of a chemical reaction without being consumed in the process. They work by providing an alternative reaction pathway with a lower activation energy, making it easier for reactants to reach the transition state. Catalysts play a crucial role in many industrial processes, reducing energy consumption and increasing the efficiency of reactions.
Importance of Understanding Rate of Reaction
Control of Industrial Processes:
Understanding the rate of reaction is essential for industries that rely on chemical processes. By controlling the rate of reaction, industries can optimize production, minimize waste, and reduce energy consumption. This knowledge is vital in sectors like pharmaceuticals, petrochemicals, and food production.
Environmental Impact:
Many chemical reactions occur in natural environments and ecosystems. Understanding the rate of these reactions is crucial for assessing the environmental impact of various pollutants, such as the breakdown of hazardous chemicals and the oxidation of pollutants in the atmosphere.
Scientific Advancements:
A deep understanding of reaction rates is critical for advancing scientific knowledge. Researchers use this knowledge to explore new reactions, design novel catalysts, and develop innovative materials. This, in turn, contributes to the advancement of scientific fields like chemistry and materials science.
Rate of Reaction Formula
Consider the chemical reaction.
a A + b B → p P + q Q
Here A and B shows the reactants and P, Q denote products. The small letters a, b, p, q denote Stoichiometric coefficients.
The rate of reaction r occurring in a closed system without the formation of reaction intermediates under isochoric conditions is given by
r = - (1/a) (d[A]/dt = - (1/b) (d[B]/dt = - (1/p) (d[P]/dt = - (1/q) (d[Q]/dt
Negative sign denotes the decreasing concentration of the reactant.
The rate of reaction is a fundamental concept in chemistry that influences a wide range of scientific, industrial, and environmental processes. It is affected by various factors, including the nature of reactants, concentration, temperature, surface area, and the presence of catalysts. A comprehensive understanding of reaction rates is essential for controlling chemical processes, minimizing environmental impact, and driving scientific advancements. As our understanding of reaction kinetics continues to evolve, it holds the promise of unlocking new possibilities and innovations in various fields of science and technology.
FAQS on Rate of Reaction
Q: For the reaction given below with k = 2.0 × 10–6 mol–2 L2 s–1. Calculate the initial rate of the reaction when [A] = 0.1 mol L^–1, [B] = 0.2 mol L^–1. Calculate the rate of reaction after [A] is reduced to 0.06 mol L^–1. 2A + B → A2B the rate = k[A][B]2
A:
A 4.2 a) Rate = k[A][B]2, Rate = 0 × 10-6[0.1][0.2]2
Rate = 8 × 10-9 mol L-1sec-1
b) 2A +B = A2B
=0.06+ 0.18 = 0.02
Situation when A is remained 0.06 mol L-1
Now, According to rate law, Rate = k[A][B]2
Rate = 2 × 10-6[0.06][0.18]2
i.e. Rate = 3.888 × 10-9 mol L-1sec-1
Initial rate of reaction is 8 × 10-9 mol L-1sec-1. and rate when concentration of A is 0.06 mol L-1 is 3.888 × 10-9 mol L-1sec-1.
Q: The decomposition of NH3 on platinum surface is zero order reaction. What are the rates of production of N2 and H2 if k = 2.5 × 10^–4 mol^–1 L s^–1?
A:
2NH3(g) → N2(g) + 3H2(g)
Rate of zero order reaction is equal to rate constant. i.e. Rate = 2.5 × 10-4mol L-1sec-1.
According to rate law,
-d[NH3] / 2dt = d[N2] / dt
2.5 × 10-4mol L-1sec-1 = d[N2] / dt
i.e. the rate of production of N2 is 2.5 × 10-4mol L-1 sec-1.
According to rate law,
-d[NH3] / 2dt = d[H2] / 3dt
d[H2] / dt = -3 X d[NH3] / 2dt
i.e. rate of formation of H2 is 3 times rate of reaction = 3 × 2.5 × 10-4mol L-1sec-1
= 7.5 × 10-4mol L-1sec-1
Rate of formation of N2 and H2 is 2.5 × 10-4 mol L-1sec-1 and 7.5 × 10-4 mol L-1sec-1 respectively
Q: What is the effect of temperature on the rate constant of a reaction? How can this effect of temperature on rate constant be represented quantitatively?
A: Increase in temperature increases the rate constant of a reaction. as we know increase in temperature increases the rate of reaction to satisfy the equation Rate = k [concentration]n where n can be any real number. k have to increase as concentration is almost not changing over small temperature change.
Increasing temperature by 100C almost double the rate constant. This can be represented quantitively by the help of arrehinus equation-
K = Ae-Ea/RT, where k is rate constant, Ea is activation energy, R is universal gas constant, T is absolute temperature.
Q: In a pseudo first order hydrolysis of ester in water, the following results were obtained, Calculate the average rate of reaction between the time intervals 30 to 60 seconds.
A:
| t/s |
0 |
30 |
60 |
90 |
| [Ester]/mol L-1 |
0.55 |
0.31 |
0.17 |
0.085 |
A 4.8 (i) Average rate of reaction over interval is [change in concentration]/[time taken] e.
[0.31 - 0.17] / [60-30] = 0.00467 mol L-1 sec-1
- (ii) the pseudo first-order rate constant can be calculated by K = (2.303/t) log(Ci/Ct)
- where K is Rate constant,
- t is time taken,
- Ci is initial concentration
- Ct is Concentration at time t.
- K = (2.303/30) log (0.55/0.31)
⇒K = 1.9 × 10-2 sec-1
- (i)Average rate between 30 to 60 sec is 0.00467 mol L-1sec-1
- (ii) Pseudo first order rate constant is 1.× 10-2sec-1
Q: A reaction is first order in A and second order in B. (i) Write the differential rate equation. (ii) How is the rate affected on increasing the concentration of B three times? (iii) How is the rate affected when the concentrations of both A and B are doubled?
A: Order is power raised to reactant in rate law, hence,
Rate = k[A][B]2
- (ii) When the concentration of B is increased three times then the rate is affected by the square of The rate is increased by 9 Times.
- (iii) When concentration of reactant both A and B is doubled then the rate will have affected as square of reactant B and Two times of Reactant Overall increase in rate is 8 times
- (a) When the concentration of B is increased by three times, the rate is increased by nine
- (b) When of both reactants doubled then Rate increased 8 times.
Q: The following data were obtained during the first order thermal decomposition of SO2Cl2 at a constant volume. Calculate the rate of the reaction when total pressure is 0.65 atm.
A:
SO2Cl2(g)→ SO2(g) + Cl2(g)
| Experiment |
Time/s–1 |
Total pressure/atm |
| 1 |
0 |
0.5 |
| 2 |
100 |
0.6 |
A 4.21 When t = 0, the total partial pressure is P0 = 0.5 atm
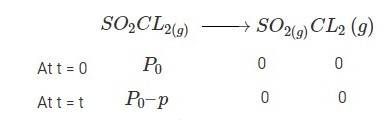
When time t = t, the total partial pressure is Pt = P0 + p
P0-p = Pt-2p, but by the above equation, we know p = Pt-P0
Hence, P0-p = Pt-2(Pt-P0)
Thus, P0-p = 2P0 – Pt
We know that time
t= 2.303/K log R0 / R
Where, k- rate constant
[R]° -Initial concentration of reactant [R]-Concentration of reactant at time ‘t’
Here concentration can be replaced by the corresponding partial pressures.
Hence, the equation becomes,
t= 2.303/K log P0 / P0 - P
t= 2.303/K log P0 / 2P0 - Pt
→ equation 1
At time t = 100 s, Pt = 0.6 atm and P0 = 0.5 atm,
Substituting in equation 1,
100 = 2.303/k log 0.5 / (2X0.5) - 0.6
Thus, k = 2.231 × 10-3 s-1
The rate of reaction R = k × PS02Cl2
When total pressure Pt = 0.65 atm and P0 = 0.5 atm, then
[PS02Cl2 = 2P0-Pt
Thus, substituting the values, PS02Cl2 = 2(0.5)-0.6 = 0.35 atm
R = k × PS02Cl2 = 2.231 × 10-3 s–1 × 0.35
Rate of the reaction R = 7.8 × 10-4atm s–1
News & Updates
Atoms and Molecules Exam
Student Forum
Anything you would want to ask experts?Popular Courses After 12th
Exams: BHU UET | KUK Entrance Exam | JMI Entrance Exam
Bachelor of Design in Animation (BDes)
Exams: UCEED | NIFT Entrance Exam | NID Entrance Exam
BA LLB (Bachelor of Arts + Bachelor of Laws)
Exams: CLAT | AILET | LSAT India
Bachelor of Journalism & Mass Communication (BJMC)
Exams: LUACMAT | SRMHCAT | GD Goenka Test