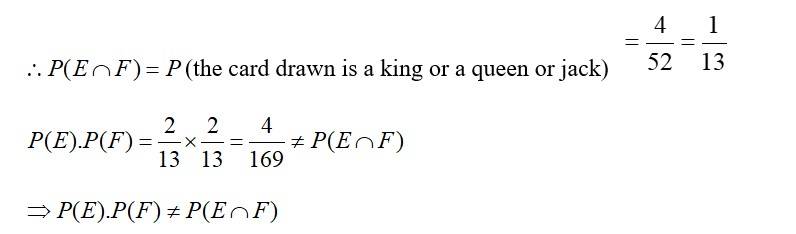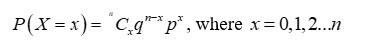NCERT Solutions Class 12 Maths Chapter 13 Probability: Download Exercise Solutions PDF

Class 12 Maths NCERT solutions Probability: Students can check the NCERT Solutions Maths Chapter 13 Probability Class 12 given on this page. We have provided detailed solutions of NCERT Maths Chapter 13 Probability Class 12 prepared by experts. Students can use this as a perfect learning resource to learn the problems of probability. Revising the solutions of NCERT Maths Class 12 Chapter 13 Probability will boost the confidence of the students and thus achieve better results. Students can use Class 12 Maths NCERT solutions Probability Class 12 as the best tool for cracking entrance exams.

We also provide the NCERT Solutions Maths Class 12 Chapter 13 Probability in PDF format for free. Students can download and save them for future reference. Probability is the measurement of the likelihood of an event to occur.

Chapter 13 Probability has a weightage of 8 marks for the Class 12 CBSE board exam. Students can easily score full marks from this chapter. This chapter begins with the topic conditional probability. It is the probability of an event happening, based on the existence of a previous event or outcome. Download NCERT Maths Class 12 Solutions Chapter 13 Probability PDF on this page. NCERT solutions are the best study material for the board exams as well as competitive exams.

Table of Contents

Probability Class 12 Solutions Exercise 13.1 & Exercise 13.2
Probability Class 12 Solutions Exercise 13.3 & Exercise 13.4
Probability Class 12 Solutions Exercise 13.5 & Miscellaneous Exercise

Probability Class 12 Solutions Exercise 13.1 & Exercise 13.2

Ex. 13.1

Q1. Given that E and F are events such that P(E) = 0.6, P(F) = 0.3 and P(E ∩ F) = 0.2, find P (E|F) and P(F|E).

A.1. Given,

$P (E) = 0.6$

$P (F) = 0.3$

$P (E \cap F) = 0.2$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{0.2}{0.3} = \frac{2}{3}$

$P (F / E) = \frac{P (F \cap E)}{P (E)} = \frac{0.2}{0.6} = \frac{2}{6} = \frac{1}{3}$

Q2. Compute P(A|B), if P(B) = 0.5 and P (A ∩ B) = 0.32

A.2. Given,

$P (B) = 0.5$

$P (A \cap B) = 0.32$

$P (A / B) = \frac{P (A \cap B)}{P (B)} = \frac{0.32}{0.5} = \frac{32}{50} = \frac{16}{45}$

Q3. If P(A) = 0.8, P(B) = 0.5 and P(B|A) = 0.4, find

(i) P(A ∩ B)

(ii) P(A|B)

(iii) P(A ∪ B)

A.3. Given,

$P (A) = 0.8$

$P (B) = 0.5$

$P (B / A) = 0.4$

$(i) P (A \cap B)$

$P (B / A) = \frac{P (B \cap A)}{P (A)}$ $[∵ P (A \cap B) = P (B \cap A)]$

$\Rightarrow 0.4 = \frac{P (A \cap B)}{0.8}$

$\Rightarrow P (A \cap B) = 0.4 \times 0.8$

$= 0.32$

$(ii) P (A / B)$

$P (A / B) = \frac{P (A \cap B)}{P (B)}$

$P (A / B) = \frac{0.32}{0.5}$

$= 0.64$

$(iii) P (A \cup B)$

$P (A \cup B) = P (A) + P (B) - P (A \cap B)$

$= 0.8 + 0.5 - 0.32$

$= 0.98$

Q4. Evaluate P (A ∪ B), if 2P (A) = P (B) =5/13 and P(A|B) =2/5

A.4. Given,

$2 P (A) = P (B) = \frac{5}{13}$

$P (A / B) = \frac{2}{5}$

$2 P (A) = \frac{5}{13} \Rightarrow P (A) = \frac{5}{13 \times 2} = \frac{5}{26}$

$\Rightarrow P (A / B) = \frac{P (A \cap B)}{P (B)}$

$\Rightarrow \frac{2}{5} = \frac{P (A \cap B)}{\frac{5}{13}}$

$\Rightarrow \frac{2}{5} \times \frac{5}{13} = P (A \cap B) \Rightarrow P (A \cap B) = \frac{2}{13}$

$∴ P (A \cup B) = P (A) + P (B) - P (A \cap B)$

$= \frac{5}{26} + \frac{5}{13} - \frac{2}{13}$

$= \frac{5 + 10 - 4}{26} = \frac{11}{26}$

Q5. If P(A)=6/11, P(B) =5/11 and P(A ∪ B) =7/11, find

(i) P(A ∩ B)

(ii) P(A|B)

(iii) P(B|A)

A.5. Given,

$P (A) = \frac{6}{11}$

$P (B) = \frac{5}{11}$

$P (A \cup B) = \frac{7}{11}$

$(i) P (A \cap B)$

$P (A \cup B) = P (A) + P (B) - P (A \cap B)$

$\Rightarrow \frac{7}{11} = \frac{6}{11} + \frac{5}{11} - P (A \cap B)$

$\Rightarrow \frac{7}{11} - \frac{11}{11} = - P (A \cap B)$

$\Rightarrow - \frac{4}{11} = - P (A \cap B)$

$\Rightarrow P (A \cap B) = \frac{4}{11}$

$(ii) P (A / B)$

$P (A / B) = \frac{P (A \cap B)}{P (B)}$

$= \frac{\frac{4}{11}}{\frac{5}{11}} = \frac{4}{5}$

$(iii) P (B / A)$

$P (B / A) = \frac{P (B \cap A)}{P (A)}$

$= \frac{\frac{4}{11}}{\frac{6}{11}} = \frac{4}{6} = \frac{2}{3}$

Q6. Determine: A coin is tossed three times.

(i) E : heads on third toss, F : heads on first two tosses.

(ii) E : at least two heads, F : at most two heads.

(iii) E : at most two tails, F : at least one tail.

A.6. The sample space has 8 outcome,

$S = {H H H, H H T, H T H, H T T, T H H, T H T, T T H, T T T}$

$(i) E = {H H H, H T H, T H H, T T H}$

$F = {H H H, H H T}$

and $E \cap F = {H H H}$

$P (F) = \frac{2}{8}$ $; P (E \cap F) = \frac{1}{8}$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{\frac{1}{8}}{\frac{2}{8}} = \frac{1}{2}$

$(ii) E = {H H H, H H T, H T H, T H H}$

$F = {H H T, H T H, H T T, T H H, T H T, T T H, T T T}$

$E \cap F = {H H H, H T H, T H H}$

$P (F) = \frac{7}{8}$ $; P (E \cap F) = \frac{3}{8}$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{\frac{3}{8}}{\frac{7}{8}} = \frac{3}{7}$

$(iii) E : {H H H, H H T, H T T, H T H, T H H, T H T, T T H}$

$F : {H H T, H T H, H T T, T H H, T H T, T T T, T T H}$

$E \cap F : {H H T, H T H, H T T, T H H, T H T, T T H}$

$P (F) = \frac{7}{8}$ $; P (E \cap F) = \frac{6}{8}$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{\frac{5}{8}}{\frac{6}{8}} = \frac{6}{7}$

Q7.Determine :Two coins are tossed once.

(i) E : tail appears on one coin, F : one coin shows head.

(ii) E : no tail appears, F : no head appears.

A.7. The sample space of given experiment is

$S = {H H, H T, T H, T T}$

$(i) E : {H T, T H}$

$\Rightarrow F : {H T, T H}$

$\Rightarrow E \cap F = {H T, T H}$

$P (F) = \frac{2}{4} = \frac{1}{2}$ $; P (E \cap F) = \frac{2}{4} = \frac{1}{2}$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{\frac{1}{2}}{\frac{1}{2}} = 1$

$(ii) E : {H H}$

$F : {T T}$

$E \cap F : {}$

So, $, P (F) = \frac{1}{4}$ $, P (E \cap F) = \frac{0}{4} = 0$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{0}{\frac{1}{4}} = 0$

Q8.Determine: E A dice is thrown three times.

E : 4 appears on the third toss, F : 6 and 5 appears respectively on first two tosses.

A.8. If a die is thrown three times, then the number of elements in the sample space will be $6 \times 6 \times 6 = 216$

Here, $E =$ ${\begin{cases} (1, 1, 4), (1, 2, 4), (1, 3, 4), (1, 4, 4), (1, 5, 4), (1, 6, 4) \\ (2, 1, 4), (2, 2, 4), (2, 3, 4), (2, 4, 4), (2, 5, 4), (2, 6, 4) \\ (3, 1, 4), (3, 2, 4), (3, 3, 4), (3, 4, 4), (3, 5, 4), (3, 6, 4) \\ (4, 1, 4), (4, 2, 4), (4, 3, 4), (4, 4, 4), (4, 5, 4), (4, 6, 4) \\ (5, 1, 4), (5, 2, 4), (5, 3, 4), (5, 4, 4), (5, 5, 4), (5, 6, 4) \\ (6, 1, 4), (6, 2, 4), (6, 3, 4), (6, 4, 4), (6, 5, 4), (6, 6, 4) \end{cases}}$

$F = {(6, 5, 1), (6, 5, 2), (6, 5, 3), (6, 5, 4), (6, 5, 5), (6, 5, 6)}$

$\Rightarrow E \cap F = {(6, 5, 4)}$

$i . e ., P (F) = \frac{6}{216}$ $, P (E \cap F) = \frac{1}{216}$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{\frac{1}{216}}{\frac{6}{216}} = \frac{1}{6}$

Q9. Determine: Mother, father and son line up at random for a family picture.

E : Son on one end, F : Father in middle.

A.9. The sample space of given condition is

$S = {(M F S, M S F, F M S, F S M, S M F, S F M)}$

$E : {M F S, F M S, S M F, S F M}$

$F : {M F S, S F M}$

$P (F) = \frac{2}{6} = \frac{1}{3}$ , $P (E \cap F) = \frac{2}{6} = \frac{1}{3}$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{\frac{1}{3}}{\frac{1}{3}} = 1$

Q.10.A black and a red die are rolled.

(a) Find the conditional probability of obtaining a sum greater than 9, given that the black die resulted in a 5.

(b) Find the conditional probability of obtaining the sum 8, given that the red die resulted in a number less than 4.

A.10. When two die are rolled, the sample space has $6 \times 6 = 36$

Let $A$ be obtaining a sum greater than

$9 = {(4, 6), (5, 5), (5, 6), (6, 4), (6, 5), (6, 6)}$

And $B$ be black die result in $5$ ,

$B = {(5, 1), (5, 2), (5, 3), (5, 4), (5, 5), (5, 6)}$

$A \cap B = {(5, 5), (5, 6)}$

$a. P (A / E) = \frac{P (A \cap B)}{P (B)} = \frac{\frac{2}{36}}{\frac{6}{36}} = \frac{1}{3}$

b. Let,

$E :$ sum of observation is $8$

$= {(2, 6), (3, 5), (4, 4), (5, 3), (6, 2)}$

$F :$ Red die resulted in number less than $4$

$= {\begin{cases} (1, 1), (1, 2), (1, 3), (2, 1), (2, 2), (2, 3) \\ (3, 1), (3, 2), (3, 3), (4, 1), (4, 2), (4, 3) \\ (5, 1), (5, 2), (5, 3), (6, 1), (6, 2), (6, 3) \end{cases}}$

$E \cap F = {(5, 3), (6, 2)}$

$P (F) = \frac{18}{36}$ and $P (E \cap F) = \frac{2}{36}$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{\frac{2}{36}}{\frac{18}{36}} = \frac{2}{18} = \frac{1}{9}$

Q11. A fair die is rolled. Consider events E = {1, 3, 5}, F = {2, 3} and G = {2, 3, 4, 5}. Find:

(i) P (E|F) and P (F|E)

(ii) P (E|G) and P (G|E)

(ii) P ((E ∪ F)|G) and P ((E ∩ G)|G)

A.11. The sample space of given condition is

A $S = {1, 2, 3, 4, 5, 6}$

$E = {1, 3, 5}$ $, F = {2, 3}$ $, G = {2, 3, 4, 5}$

$P (E) = \frac{3}{6} = \frac{1}{2}$ $, P (F) = \frac{2}{6} = \frac{1}{3}$ $P (G) = \frac{4}{6} = \frac{2}{3}$

$(i) P (E / F)$ and $P (F / E)$

$P (E \cap F) = \frac{1}{6}$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{\frac{1}{6}}{\frac{1}{3}} = \frac{1}{2}$

and $P (F / E) = \frac{P (F \cap E)}{P (E)} = \frac{\frac{1}{6}}{\frac{1}{2}} = \frac{1}{3}$

$(ii) P (E / G)$ and $P (G / E)$

$P (E \cap G) = \frac{2}{6} = \frac{1}{3}$

$P (E / G) = \frac{P (E \cap G)}{P (G)} = \frac{\frac{1}{3}}{\frac{2}{3}} = \frac{1}{2}$

$P (G / E) = \frac{P (G \cap E)}{P (E)} = \frac{\frac{1}{3}}{\frac{1}{2}} = \frac{2}{3}$

$(iii) P ((E \cup F) / G)$ and $P ((E \cap F) / G)$

$(E \cup F) = {1, 2, 3, 5}$

$(E \cup F) \cap G = {1, 2, 3, 5} \cap {2, 3, 4, 5}$

$= {2, 3, 5}$

and $E \cap F = {3}$

$(E \cap F) \cap G = {3}$

$P (E \cup G) = \frac{4}{6} = \frac{2}{3}$

Now,

$P ((E \cup F) \cap G) = \frac{3}{6} = \frac{1}{2}$

So,

$P ((E \cup F) / G) = \frac{\frac{1}{2}}{\frac{2}{3}} = \frac{3}{4}$

$P ((E \cap F) / G) = \frac{\frac{1}{6}}{\frac{2}{3}} = \frac{3}{12} = \frac{1}{4}$

Q12. Assume that each child born is equally likely to be a boy or a girl. If a family has two children, what is the conditional probability that both are girls given that (i) the youngest is a girl (ii) at least one is a girl?

A.12. Let $B$ and $G$ denote boy and girl respectively.

Sample space, $S = {G G, B B, G B, B G}$

Let, $E :$ Both are girls

$E = {G, G}$

i. Let $, F :$ youngest is a girl

$F = {G G, B, G}$

$E \cap F = {G, G}$ $, P (E \cap F) = \frac{1}{4}$

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{\frac{1}{4}}{\frac{2}{4}} = \frac{1}{2}$

ii. Let $A :$ at least one is girl

$A = {G G, G B, B G}$

$P (A) = \frac{3}{4}$

$E \cap A = {G G}$

$P (E \cap A) = \frac{1}{4}$

$P (E / A) = \frac{\frac{1}{4}}{\frac{3}{4}} = \frac{1}{3}$

Q13. An instructor has a test bank consisting of 300 easy True/False questions, 200 difficult True/False questions, 500 easy multiple-choice questions and 400 difficult multiple choice questions. If a question is selected at random from the test bank, what is the probability that it will be an easy question given that it is a multiple-choice question?

A.13. Here, there are two types of questions, True/False or Multiple-Choice Questions. They are divided into easy and difficult.

True/False: 300 easy, 200 difficult

$M C Q$ : 500 easy, 400 difficult

Let, $E =$ easy question

$M =$ multiple choice question

Total number of questions= $300 + 200 + 500 + 400 = 1400$

Total number of multiple-choice questions= $500 + 400 = 900$

Therefore, probability of selecting an easy $M C Q$ is

$P (E \cap M) = \frac{500}{1400} = \frac{5}{14}$

The probability of setting a $M C Q$ is

$P (M) = \frac{900}{1400} = \frac{9}{14}$

$∴$ $P (E / M) = \frac{P (E \cap M)}{P (M)} = \frac{\frac{5}{14}}{\frac{9}{14}} = \frac{5}{9}$

$∴$ Required probability is $\frac{5}{9}$

Q14.Given that the two numbers appearing on throwing two dice are different. Find the probability of the event ‘the sum of numbers on the dice is 4’.

A.14. When dice is thrown, number of observations in the sample space is $6 \times 6 = 36$

Let, $A :$ event that the sum of the number on the dice is $4$

$B :$ event that the two number appearing on throwing two dice is different

$A = {(1, 3), (2, 2), (3, 1)}$

$B = {\begin{cases} (1, 2), (1, 3), (1, 4), (1, 5), (1, 6) \\ (2, 1), (2, 3), (2, 4), (2, 5), (2, 6) \\ (3, 1), (3, 2), (3, 4), (3, 5), (3, 6) \\ (4, 1), (4, 2), (4, 3), (4, 5), (4, 6) \\ (5, 1), (5, 2), (5, 3), (5, 4), (5, 6) \\ (6, 1), (6, 2), (6, 3), (6, 4), (6, 5) \end{cases}}$

$(A \cap B) = {(1, 3), (3, 1)}$

$P (B) = \frac{30}{36}$ $, P (A \cap B) = \frac{2}{36}$

$P (A / B) = \frac{P (A \cap B)}{P (B)} = \frac{\frac{2}{36}}{\frac{30}{36}} = \frac{2}{30} = \frac{1}{15}$

$∴$ Required probability is $\frac{1}{15}$

Q.15.Consider the experiment of throwing a die, if a multiple of 3 comes up throw the die again and if any other number comes toss a coin. Find the conditional probability of the event “the coin shows a tail”, given that “at least one die shows a 3”.

A15. The sample space of experiment is

$S = {\begin{cases} (1, H), (1, T), (2, H), (2, T), (3, 1), \\ (3, 2), (3, 3), (3, 4), (3, 5), (3, 6), \\ (4, H), (4, T), (5, H), (5, T), \\ (6, 1), (6, 2), (6, 3), (6, 4), (6, 3), \\ (6, 5), (6, 6) \end{cases}}$

Let, $E$ be the event that ‘coin shows a tail’ and $F$ be the event that ‘atleast one die shows a $3$ ’

$\Rightarrow E = {1 T, 2 T, 4 T, 5 T}$

$F = {(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6)}$

$\Rightarrow E \cap F = \emptyset \Rightarrow P (E \cap F) = 0$

Now,

$P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{0}{P (F)} = 0$

$\Rightarrow P (E / F) = 0$

Q16. If P (A) = 1/2 P (B) = 0, then P(A|B) is:

(A) 0

(B) 1/2

(C) not defined

(D) 1

A.16. $P (A) = \frac{1}{2}$ $, P (B) = 0$

$P (A / B) = \frac{P (A \cap B)}{P (B)}$

$= \frac{P (A \cap B)}{0}$ = which is not defined

The correct answer is $(C)$ not defined.

Q17.If A and B are events such that P (A|B) = P(B|A), then

(A) A ⊂ B but A ≠ B

(B) A = B

(C) A ∩ B = Φ

(D) P(A) = P(B)

A.17. $P (A / B) = P (B / A)$

$P (A / B) = \frac{P (A \cap B)}{P (B)}$ ___(i)

$P (B / A) = \frac{P (B \cap A)}{P (A)}$ ___(ii)

Using (i) and (ii),

$P (A / B) = P (B / A)$

$\Rightarrow \frac{P (A \cap B)}{P (B)} = \frac{P (B \cap A)}{P (A)}$ $[∵ B \cap A = A \cap B]$

$\Rightarrow P (A) = P (B)$

$∴$ The correct answer is $(D) . P (A) = P (B)$

Ex.13.2

Q1. If P (A) = 3/5 and P (B) = 1/5 find P (A ∩ B) if A and B are independent events.

A.1. $P (A) = \frac{3}{5}$

$P (B) = \frac{1}{5}$

As $A$ and $B$ are independent event,

$P (A \cap B) = P (A) . P (A)$

$= \frac{3}{5} . \frac{1}{5}$

$= \frac{3}{25}$

Q2.Two cards are drawn at random and without replacement from a pack of 52 playing cards. Find the probability that both the cards are black.

A.2. There are $26$ black cards in a deck of $52$ cards.

Let, $P (A) =$ the probability of getting a black card at first draw

$P (B) =$ Probability of getting a black card at second draw

$P (A) = \frac{26}{52} = \frac{1}{2}$

Since, it is not replaced, $P (B) = \frac{25}{51}$

Probability of getting both card are black

$= P (A) . P (B)$

$= \frac{1}{2} \times \frac{25}{51}$

$= \frac{25}{102}$

Q3. A box of oranges is inspected by examining three randomly selected oranges drawn without replacement. If all the three oranges are good, the box is approved for sale otherwise it is rejected. Find the probability that a box containing 15 oranges out of which 12 are good and 3 are bad ones will be approved for sale.

A.3. Let $A$ , $B$ and $C$ be the respective events that the first, second and third drawn orange is good.

$P (A) =$ Probability that first drawn orange is good= $\frac{12}{15}$

The orange is not replaced;

$P (B) =$ Probability of getting second orange is good= $\frac{11}{14}$

Similarity, probability of getting third orange is good, $P (C) = \frac{10}{13}$

$∴$ Probability of getting all orange good= $\frac{12}{15} \times \frac{11}{14} \times \frac{10}{13}$ $= \frac{44}{91}$

Therefore, probability that will approve for sale $= \frac{44}{91}$

Q4. A fair coin and an unbiased die are tossed. Let A be the event ‘head appears on the coin’ and B be the event ‘3 on the die’. Check whether A and B are independent events or not.

A.4. The sample space of given condition are

$S = {\begin{cases} (H, 1), (H, 2), (H, 3), (H, 4), (H, 5), (H, 6) \\ (T, 1), (T, 2), (T, 3), (T, 4), (T, 5), (T, 6) \end{cases}}$

Let $A$ = head appear on the coin

$B$ = $3$ on the die

$A = {(H, 1), (H, 2), (H, 3), (H, 4), (H, 5), (H, 6)}$

$P (A) = \frac{6}{12} = \frac{1}{2}$

$B = {(H, 3), (T, 3)}$

$P (B) = \frac{2}{12} = \frac{1}{6}$

$A \cap B = {(H, 3)}$

$i . e ., P (A \cap B) = \frac{1}{12}$

$P (A) . P (B) = \frac{1}{2} \times \frac{1}{6} = \frac{1}{12} = P (A \cap B)$

Therefore, $A$ and $B$ are independent.

Q5.A die marked 1, 2, 3 in red and 4, 5, 6 in green is tossed. Let A be the event ‘number is even’ and B be the event ‘number is red’. Are A and B independent?

A.5. When die is thrown, the sample space

$S = {1, 2, 3, 4, 5, 6}$

Let, $A$ =the number is even $= {2, 4, 6}$

$B$ =the number is red $= {1, 2, 3}$

$\begin{array}{l} P (A) = \frac{3}{6} = \frac{1}{2} \\ P (B) = \frac{3}{6} = \frac{1}{2} \\ A \cap B = {2} \\ P (A \cap B) = \frac{1}{6} \\ ∴ P (A) . P (B) = \frac{1}{2} \times \frac{1}{2} = \frac{1}{4} \neq P (A \cap B) \end{array}$

Hence, $A$ and $B$ are not independent.

Q.6.Let E and F be events with $P (E) - \frac{3}{5}, P (F) = \frac{3}{10} a n d P (E \cap F) = \frac{1}{2}$ Are E and F independent?

A.6. $P (E) = \frac{3}{5}$

$P (F) = \frac{3}{10}$

$P (E \cap F) = \frac{1}{5}$

$P (E) . P (F) = \frac{3}{5} \times \frac{3}{10}$

$= \frac{9}{50} \neq P (E \cap F)$

Here, $P (E) . P (F) \neq P (E \cap F)$ . $A$ and $B$ are not independent.

Q7. Given that the events A and B are such that P (A) = 1/2 P (A ∩ B) = 3/5 and P (B) = p Find p if they are (i) mutually exclusive, (ii) independent.

A.7. Given,

$P (A) = \frac{1}{2}$

$P (A \cup B) = \frac{3}{5}$

$P (B) = p$

(i) We know that,

when $A$ and $B$ are mutually exclusive

$(A \cap B) = \emptyset$ $, P (A \cap B) = 0$

$P (A \cup B) = P (A) + P (B) - P (A \cap B)$

$\Rightarrow \frac{3}{5} = \frac{1}{2} + p$

$\Rightarrow p = \frac{3}{5} - \frac{1}{2} = \frac{6 - 5}{10} = \frac{1}{10}$

(ii) when $A$ and $B$ are independent

$\begin{array}{l} P (A \cap B) = P (A) . P (B) \\ P (A \cap B) = \frac{1}{2} \times p \end{array}$

Now,

$P (A \cup B) = P (A) + P (B) - P (A \cap B)$

$\Rightarrow \frac{3}{5} = \frac{1}{2} + p - \frac{1}{2} p$

$\Rightarrow \frac{3}{5} - \frac{1}{2} = \frac{2 p - p}{2}$

$\Rightarrow \frac{6 - 5}{10} = \frac{p}{2}$

$\Rightarrow p = \frac{1 \times 2}{10} = \frac{2}{10} = \frac{1}{5}$

Q8.Let A and B independent events, P (A) = 0.3 and P (B) = 0.4. Find:

(A) P (A ∩ B)

(B) P (A U B)

(C) P (A|B)

(D) P (B|A)

A.8. Given,

$P (A) = 0.3$

$P (B) = 0.4$

$A$ and $B$ are independent

$(i) P (A \cap B) = P (A) . P (B)$

$\begin{array}{l} = 0.3 \times 0.4 \\ = 0.12 \end{array}$

$(ii) P (A \cup B) = P (A) + P (B) - P (A \cap B)$

$\begin{array}{l} P (A \cup B) = 0.3 + 0.4 - 0.12 \\ = 0.58 \end{array}$

$(iii) P (A / B) = \frac{P (A \cap B)}{P (B)} = \frac{0.12}{0.4} = 0.3$

$(iv) P (B / A) = \frac{P (B \cap A)}{P (A)} = \frac{0.12}{0.3} = 0.4$

Q9.If A and B are two events such that P (A) = 1/4 P (B) = 1/2 and P (A ∩ B) = 1/8 find P (not A and not B).

A.9. Given,

$P (A) = \frac{1}{4}$

$P (B) = \frac{1}{2}$

$P (A \cap B) = \frac{1}{8}$ Here, $P$ (not $A$ not $B$ ) $= P (A^{'} \cap B^{'}) = P (A \cup B)^{'}$

$\Rightarrow 1 - P (A \cup B)$

$\Rightarrow 1 - [P (A) + P (B) - P (A \cap B)]$

$\Rightarrow 1 - [\frac{1}{4} + \frac{1}{2} - \frac{1}{8}]$

$\Rightarrow 1 - [\frac{2 + 4 - 1}{8}]$

$\Rightarrow 1 - \frac{5}{8} = \frac{8 - 5}{8}$

$\Rightarrow \frac{3}{8}$

Q10. Events A and B are such that P (A) = 1/2 P (B) = 7/12 and P (not A or not B) = 1/4 State whether A and B are independent.

A.10. Given,

$P (A) = \frac{1}{2}$

$P (B) = \frac{7}{12}$

$P$ (not $A$ or not $B$ )= $\frac{1}{4}$

$\Rightarrow P (A^{'} \cup B^{'}) = P (A \cap B)^{'}$

$\Rightarrow P (A \cap B)^{'} = \frac{1}{4}$

$\Rightarrow 1 - P (A \cap B) = \frac{1}{4}$

$\Rightarrow P (A \cap B) = 1 - \frac{1}{4}$

$\Rightarrow \frac{4 - 1}{4}$

$\Rightarrow \frac{3}{4}$

Now,

$P (A) . P (B) = \frac{1}{2} \times \frac{7}{12}$

$= \frac{7}{24} \neq \frac{3}{4}$

$\Rightarrow P (A) . P (B) \neq P (A \cap B)$

Therefore, $A$ and $B$ are not independent.

Q11.Given two independent events A and B such that P (A) = 0.3, P (B) = 0.6. Find:

(A) P (A and B)

(B) P (A and not B)

(C) P (A or B)

(D) P (neither A nor B)

A.11. Given,

$P (A) = 0.3$

$P (B) = 0.6$

$i. A$ and $B$ are independent

$\Rightarrow P (A \cap B) = P (A) . P (B)$

$= 0.3 \times 0.6$

$= 0.18$

$ii. P$ ( $A$ and not $B$ )

$\Rightarrow P (A \cap B^{'}) = P (A) - P (A \cap B)$

$= 0.3 - 0.18$

$= 0.12$

$iii. P$ ( $A$ or $B$ )

$\Rightarrow P (A \cup B) = P (A) + P (B) - P (A \cap B)$

$= 0.3 + 0.6 - 0.18$

$= 0.72$

$iv. P$ (neither $A$ nor $B$ )

$\Rightarrow P (A^{'} \cap B^{'}) = P (A \cup B)^{'}$

$= 1 - P (A \cup B)$

$= 1 - 0.72$

$= 0.28$

Q12.A die is tossed thrice. Find the probability of getting an odd number at least once.

A.12. The sample space of given condition is

$S = {1, 2, 3, 4, 5, 6}$

Let, $P (A) =$ probability of getting an odd number in first throw

$\Rightarrow P (A) = \frac{3}{6} = \frac{1}{2}$

$P (B) =$ probability of getting an even number

$\Rightarrow P (B) = \frac{3}{6} = \frac{1}{2}$

Probability of getting an even number in three times $= \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} = \frac{1}{8}$

So, Probability of getting an odd number at least once

$= 1 -$ probability of getting an odd number in no throw

$= 1 - \frac{1}{8}$

$= \frac{8 - 1}{8}$

$= \frac{7}{8}$

$∴$ Probability of getting an odd number at least once $= \frac{7}{8}$

Q13. Two balls are drawn at random with replacement from a box containing 10 black and 8 red balls. Find the probability that:

(i) both balls are red.

(ii) first ball is black and second is red.

(iii) one of them is black and other is red.

A.13. Total number of balls $= 18$

Number of red balls $= 8$

Number of black balls $= 10$

i. Probability of getting red ball at first draw $= \frac{8}{18} = \frac{4}{9}$

The red ball is replaced after the first draw

$∴$ Probability of getting red ball in second draw $= \frac{8}{18} = \frac{4}{9}$

$∴$ Probability of getting both the balls red $= \frac{4}{9} \times \frac{4}{9} = \frac{16}{81}$

ii. Probability of getting first black ball $= \frac{10}{18} = \frac{5}{9}$

The ball is replaced after first draw

So, the probability of getting second ball as red $= \frac{8}{18} = \frac{4}{9}$

Probability of getting first ball black and second ball as red $= \frac{5}{9} \times \frac{4}{9} = \frac{20}{81}$

iii. Probability of getting first ball as red $= \frac{8}{18} = \frac{4}{9}$

The ball is replaced after the first draw

Probability of getting second ball as black $= \frac{10}{18} = \frac{5}{9}$

Therefore, probability of getting first ball as black and second ball as red $= \frac{4}{9} \times \frac{5}{9} = \frac{20}{81}$

Therefore, probability that one of them is black and other is red= probability of getting first black ball and second as red + probability of getting first ball red second ball black $= \frac{20}{81} + \frac{20}{81} = \frac{40}{81}$

Q14. Probability of solving specific problem independently by A and B are 1/2 and 1/3 respectively. If both try to solve the problem independently, find the probability that:

(i) the problem is solved.

(ii) exactly one of them solves the problem.

A.14. Let, $P (A) =$ probability of solving problem by $A = \frac{1}{2}$

$P (B) =$ probability of solving problem by $B = \frac{1}{3}$

Since, the problem is solved independently by $A$ and $B$ , then,

$\Rightarrow P (A \cap B) = P (A) . P (B)$

$\Rightarrow P (A \cap B) = \frac{1}{2} \times \frac{1}{3}$

$= \frac{1}{6}$

$P (A^{'}) = 1 - P (A) = 1 - \frac{1}{2} = \frac{1}{2}$

$P (B^{'}) = 1 - P (B) = 1 - \frac{1}{3} = \frac{2}{3}$

i. Probability of the problem is solved

$\Rightarrow P (A \cup B) = P (A) + P (B) - P (A \cap B)$

$\Rightarrow P (A \cup B) = \frac{1}{2} + \frac{1}{3} - \frac{1}{6}$

$= \frac{3 + 2 - 1}{6}$

$= \frac{4}{6} = \frac{2}{3}$

ii. Probability that exactly one of the solved problem is given by $P (A) P (B^{'}) + P (B) P (A^{'}) = \frac{1}{2} \times \frac{2}{3} + \frac{1}{2} \times \frac{1}{3}$

$= \frac{2}{6} + \frac{1}{6} = \frac{3}{6} = \frac{1}{2}$

Q15. One card is drawn at random from a well shuffled deck of 52 cards. In which of the following cases are the events E and F independent?

A.15. i. In a deck of $52$ cards,

$13$ are spades and $4$ are aces.

$∴ P (E) = P$ (the card drawn is spade) $= \frac{13}{52} = \frac{1}{4}$

$∴ P (F) = P$ (the card drawn is an ace) $= \frac{4}{52} = \frac{1}{13}$

$∴ P (E \cap F) = P$ (the card drawn is spade and an ace) $= \frac{1}{52}$

$P (E) . P (F) = \frac{1}{4} \times \frac{1}{13} = \frac{1}{52} = P (E \cap F)$

$\Rightarrow P (E) . P (F) = P (E \cap F)$

$E$ and $F$ are independent.

ii. In a deck of $52$ cards,

$26$ cards are black and $4$ are kings.

$∴ P (E) = P$ (the card drawn is black) $= \frac{26}{52} = \frac{1}{2}$

$∴ P (F) = P$ (the card drawn is a king) $= \frac{4}{52} = \frac{1}{13}$

$∴ P (E \cap F) = P$ (the card drawn is black king) $= \frac{2}{52} = \frac{1}{26}$

$P (E) . P (F) = \frac{1}{2} \times \frac{1}{13} = \frac{1}{26} = P (E \cap F)$

$\Rightarrow P (E) . P (F) = P (E \cap F)$

Therefore, the event $E$ and $F$ are independent.

iii. In a deck of $52$ cards,

$4$ are king cards

$4$ are queen cards

$4$ are jack cards

$∴ P (E) = P$ (the card drawn is king or queen) $= \frac{8}{52} = \frac{2}{13}$

$∴ P (F) = P$ (the card drawn is a jack or queen) $= \frac{8}{52} = \frac{2}{13}$

Therefore, the event $E$ and $F$ are not independent.

Q16. In a hostel 60% of the students read Hindi newspaper, 40% read English newspaper and 20% read both Hindi and English newspapers. A student is selected at random.

(a) Find the probability that she reads neither Hindi nor English newspapers.

(b) If she reads Hindi newspaper, find the probability that she reads English newspaper.

(c) If she reads English newspapers, find the probability she reads Hindi newspaper.

A.16. Let,

$P (H) =$ denote the student who read Hindi newspaper

$P (E) =$ denote the student who read English newspaper

Then, $P (H) = 60 % = \frac{6}{10} = \frac{3}{5}$

$P (E) = 40 % = \frac{4}{10} = \frac{2}{5}$

$i. P (H \cap E) = 20 % = \frac{2}{10} = \frac{1}{5}$

$\Rightarrow P (H \cup E)^{'} = 1 - [P (H) + P (E) - P (H \cap E)]$

$= 1 - [\frac{3}{5} + \frac{2}{5} - \frac{1}{5}]$

$= 1 - \frac{3 + 2 - 1}{5}$

$= 1 - \frac{4}{5} = \frac{1}{5}$

ii. Probability of randomly chosen student that reads English newspaper, if she reads Hindi newspaper, is given by

$P (E / H) = \frac{P (E \cap H)}{P (H)} = \frac{\frac{1}{5}}{\frac{3}{5}} = \frac{1}{3}$

iii. Probability that randomly chosen student reads Hindi newspaper, if she reads English newspaper, is given by

$P (H / E) = \frac{P (H \cap E)}{P (E)} = \frac{\frac{1}{5}}{\frac{2}{5}} = \frac{1}{2}$

Q17.The probability of obtaining an even prime number on each die when a pair of dice is rolled is:

(A) 0 (B) 1/3 (C) 1/12 (D) 1/36

A.17. The outcome of rolling $2$ dice is $36$ . The only prime number is $2$ .

Let, $E =$ event of getting an even prime number on each die

$E = {(2, 2)}$ therefore

$P (E) = \frac{1}{36}$

Therefore, the correct answer is $(D)$ $\frac{1}{36}$

Q18. Two events A and B are said to be independent, if:

(A) A and B are mutually exclusive.

(B) P (A’B’) = [1 – P (A)] [1 – P (B)]

(C) P (A) = P (B)

(D) P (A) + P (B) = 1

A.18. $(B) P (A^{'} B^{'}) = [1 - P (A)] [1 - P (B)]$

$P (A^{'} B^{'}) = [1 - P (A)] [1 - P (B)]$

$\Rightarrow P (A^{'} \cap B^{'}) = 1 - P (A) - P (B) + P (A) P (B)$

$\Rightarrow - [P (A) + P (B) - P (A \cap B)] = - P (A) - P (B) + P (A) (B)$

$\Rightarrow - P (A) - P (B) + P (A \cap B) = - P (A) - P (B) + P (A) P (B)$

$\Rightarrow P (A \cap B) = P (A) . P (B)$

Therefore, it shows $A$ and $B$ are independent.

Probability Class 12 Solutions Exercise 13.3 & Exercise 13.4

Ex.13.3

Q1.An urn contains 5 red and 5 black balls. A ball is drawn at random, its colour is noted and is returned to the urn. Moreover, 2 additional balls of the colour drawn are put in the urn and then a ball is drawn at random. What is the probability that the second ball is red?

A.1. Number of balls contain in win $= 5$ red

$= 5$ black

Let the red ball be drawn in the first attempt

$∴$ $P$ (drawing a red ball) $= \frac{5}{10} = \frac{1}{2}$

By question, if added two red balls to the win, then $7$ red balls and $5$ black balls contain.

$P$ (drawing a red ball) $= \frac{7}{12}$

Let a black ball be drawn at first attempt

$∴$ $P$ (drawing a black ball) $= \frac{5}{10} = \frac{1}{2}$

If two black balls are added to the win, then $7$ black balls and $5$ red balls contain.

$P$ (drawing a red ball) $= \frac{5}{12}$

Therefore, probability of drawing the second ball as of red colour is

$= (\frac{1}{2} \times \frac{7}{12}) + (\frac{1}{2} \times \frac{5}{12}) = \frac{1}{2} (\frac{7}{12} + \frac{5}{12}) = \frac{1}{2} \times \frac{12}{12} = \frac{1}{2} \times 1 = \frac{1}{2}$

Q2. A bag contains 4 red and 4 black balls, another bag contains 2 red and 6 black balls. One of the two bags is selected at random and a ball is drawn from the bag which is found to be red. Find the probability that the ball is drawn from the first bag.

A.2. Let $E_{1}$ be the event of selecting first bag

$E_{2}$ be the event of selecting second bag

$∴ P (E_{1}) = P (E_{2}) = \frac{1}{2}$

Let $A$ be the event of getting a red ball

$P (A / E_{1}) = P$ (drawing a red ball from first bag)

$= \frac{4}{8} = \frac{1}{2}$

$P (A / E_{2}) = P$ (drawing a red ball from second bag)

$= \frac{2}{8} = \frac{1}{4}$

Then, probability of drawing a ball from the first bag which is red, is given by Baye’s theorem,

$P (E_{1} / A) = \frac{P (E_{1}) . P (A / E_{1})}{P (E_{1}) . P (A / E_{1}) + P (E_{2}) . P (A / E_{2})}$

$= \frac{\frac{1}{2} \times \frac{1}{2}}{\frac{1}{2} \times \frac{1}{2} + \frac{1}{2} \times \frac{1}{4}}$

$= \frac{\frac{1}{4}}{\frac{3}{8}} = \frac{2}{3}$

Q3. Of the students in a college, it is known that 60% reside in hostel and 40% are day scholars (not residing in hostel). Previous year results report that 30% of all students who reside in hostel attain A grade and 20% of day scholars attain A grade and 20% of day scholars attain A grade in their annual examination. At the end of the year, one student is chosen at random from the college and he has an A grade, what is the probability that the student is a hosteller?

A.3. Let, $E_{1}$ :event that the student in hostel

$E_{2}$ : event that the student is day scholar

$A$ : be the event of the chosen student get grade $A$

$P (E_{1}) = 60 % = \frac{60}{100} = 0.6$

$P (E_{2}) = 40 % = \frac{40}{100} = 0.4$

$P (A / E_{1}) = P$ (student getting an $A$ grade is hostler) $= 30 %$ $= \frac{30}{100} = 0.3$

$P (A / E_{2}) = P$ ( student getting an $A$ grade is a day scholar) $= 20 % = \frac{20}{100} = 0.2$

Then, by Baye’s theorem,

$P (E_{1} / A) = \frac{P (E_{1}) . P (A / E_{1})}{P (E_{1}) . P (A / E_{1}) + P (E_{2}) . P (A / E_{2})}$

$= \frac{0.6 \times 0.3}{0.6 \times 0.3 + 0.4 \times 0.2} = \frac{0.18}{0.26} = \frac{18}{26} = \frac{9}{13}$

Q4. In answering a question on a multiple choice test a student either knows the answer or guesses. Let 3/4 be the probability that he knows the answer and 1/4 be the probability that he guesses. Assuming that a student who guesses the answer, will be correct with probability 1/4. What is the probability that a student knows the answer given that he answered it correctly?

A.4. Let, $E_{1}$ :event that knows the answer

$E_{2}$ :event that he guesses the answer

$P (E_{1}) = \frac{3}{4}$ and $P (E_{2}) = \frac{1}{4}$

Let $A$ be the event that the answer is correct

Also, $P (A / E_{1}) = P$ (correct answer given that he knows) $= 1$

$P (A / E_{2}) = P$ (correct answer given that he guess) $= \frac{1}{4}$

Now, probability that he knows the answer given that he answer it correctly is $P (E_{1} / A)$ ,

By Baye’s theorem,

$P (E_{1} / A) = \frac{P (E_{1}) . P (A / E_{1})}{P (E_{1}) . P (A / E_{1}) + P (E_{2}) . P (A / E_{2})}$

$= \frac{\frac{3}{4} \times 1}{\frac{3}{4} \times 1 + \frac{1}{4} \times \frac{1}{4}} = \frac{\frac{3}{4}}{\frac{3}{4} + \frac{1}{16}}$

$= \frac{\frac{3}{4}}{\frac{12 + 1}{16}} = \frac{\frac{3}{4}}{\frac{13}{16}}$

$= \frac{3}{4} \times \frac{16}{13} = \frac{12}{13}$

$P (E_{1} / A) = \frac{12}{13}$

Q5. A laboratory blood test is 99% effective in detecting a certain disease when it is, in fact, present. However, the test also yields a false positive result for 0.5% of the healthy person tested (i.e., if a healthy person is tested, then, with probability 0.005, the test will imply he has the disease). If 0.1 percent of the population actually has the disease, what is the probability that a person has the disease given that his test result is positive?

A.5. Let, $E_{1} :$ event person has disease

$E_{2} :$ event person has no disease

$A :$ be event that blood test is positive

As $E_{1}$ and $E_{2}$ are events which are complementary to each other,

Then, $P (E_{1}) + P (E_{2}) = 1$

$P (E_{2}) = 1 - P (E_{1})$

$P (E_{1}) = 0.1 % = \frac{0.1}{100} = 0.001$

$∴$ $P (E_{2}) = 1 - 0.001$

$= 0.999$

$P (A / E_{1}) = P$ (result is positive given that person has disease) $= 99 % = 0.99$

$P (A / E_{2}) = P$ (result is positive given that person has no disease) $= 0.5 % = 0.005$

Now, the probability that person has a disease, given that his test result is positive is $P (E_{1} / A)$

By using Baye’s theorem,

$P (E_{1} / A) = \frac{P (E_{1}) . P (A / E_{1})}{P (E_{1}) . P (A / E_{1}) + P (E_{2}) . P (A / E_{2})}$

$= \frac{0.001 \times 0.99}{0.001 \times 0.99 + 0.999 \times 0.005}$

$= \frac{0.00099}{0.00099 + 0.004995}$

$= \frac{0.00099}{0.005985} = \frac{990}{5985} = \frac{110}{665}$

$\Rightarrow P (E_{1} / A) = \frac{22}{133}$

Q6.There are three coins. One is a two headed coin, another is a biased coin that comes up heads 75% of the time and third is an unbiased coin. One of the three coins is chosen at random and tossed, it shows head, what is the probability that it was the two headed coin?

A.6. Let, $E_{1} :$ event of choosing $Q$ headed coin

$E_{2} :$ event of choosing biased coin

$E_{3} :$ event of choosing unbiased coin

$P (E_{1}) = P (E_{2}) = P (E_{3}) = \frac{1}{3}$

Let $A$ be the event that shows head,

$P (A / E_{1}) = P$ (coin shows heads, given that it is a headed coin) $= 1$

$P (A / E_{2}) = P$ (coin showing up head, given that it is biased coin) $= \frac{75}{100} = \frac{3}{4}$

$P (A / E_{3}) = P$ (coin showing head, given that it is unbiased coin) $= \frac{1}{2}$

The probability that the coin is two headed, given that it shows heads, is given by $P (E_{1} / A)$

Using Baye’s theorem,

$P (E_{1} / A) = \frac{P (E_{1}) . P (A / E_{1})}{P (E_{1}) . P (A / E_{1}) + P (E_{2}) . P (A / E_{2}) + P (E_{3}) . P (A / E_{3})}$

$= \frac{\frac{1}{3} \times 1}{\frac{1}{3} \times 1 + \frac{1}{3} \times \frac{3}{4} + \frac{1}{3} \times \frac{1}{2}}$

$= \frac{\frac{1}{3}}{\frac{1}{3} + \frac{1}{4} + \frac{1}{6}}$

$= \frac{\frac{1}{3}}{\frac{4 + 3 + 2}{12}} = \frac{\frac{1}{3}}{\frac{9}{12}} = \frac{1}{3} \times \frac{12}{9} = \frac{4}{9}$

Q7. An insurance company insured 2000 scooter driver, 4000 car drivers and 6000 truck drivers. The probability of accidents are 0.01, 0.03 and 0.15 respectively. One of the insured persons meets with an accident. What is the probability that he is a scooter driver?

A.7. Let, $E_{1} :$ event that the driver is scooter driver

$E_{2} :$ event that the driver is car driver

$E_{3} :$ event that the driver is truck driver

$A :$ event the person meets with accident

Total number of drivers $= 2000 + 4000 + 6000 = 12000$

$P (E_{1}) = P$ (driver is a scooter driver) $= \frac{2000}{12000} = \frac{1}{6}$

$P (E_{2}) = P$ (driver is a car driver) $= \frac{4000}{12000} = \frac{1}{3}$

$P (E_{3}) = P$ (driver is a truck driver) $= \frac{6000}{12000} = \frac{1}{2}$

$P (A / E_{1}) = P$ (scooter driver met with an accident) $= 0.01 = \frac{1}{100}$

$P (A / E_{2}) = P$ (car driver met with an accident) $= 0.03 = \frac{3}{100}$

$P (A / E_{3}) = P$ (truck driver met with an accident) $= 0.15 = \frac{15}{100}$

The probability that the driver is scooter driver, given that he met with an accident is given by $P (E_{1} / A)$

By Baye’s theorem,

$P (E_{1} / A) = \frac{P (E_{1}) . P (A / E_{1})}{P (E_{1}) . P (A / E_{1}) + P (E_{2}) . P (A / E_{2}) + P (E_{3}) . P (A / E_{3})}$

$= \frac{\frac{1}{6} \times \frac{1}{100}}{\frac{1}{6} \times \frac{1}{100} + \frac{1}{3} \times \frac{3}{100} + \frac{1}{2} \times \frac{15}{100}}$

$= \frac{\frac{1}{600}}{\frac{1}{100} (\frac{1}{6} + 1 + \frac{15}{2})}$

$= \frac{\frac{1}{600}}{\frac{1}{100} (\frac{1 + 6 + 45}{6})}$

$= \frac{\frac{1}{600}}{\frac{1}{100} \times \frac{52}{6}}$

$= \frac{1}{600} \times \frac{600}{52} = \frac{1}{52}$

Q8. A factory has two machines A and B. Past record shows that machine A produced 60% of the items of output and machine B produced 40% of the items. Further, 2% of the items produced by machine A and 1% produced by machine B were defective. All the items are put into one stockpile and then one item is chosen at random from this and is found to be defective. What is the probability that it was produced by machine B?

A.8. Let, $E_{1} :$ event items produced by $A$

$E_{2} :$ event items produced by $B$

$X :$ event that produced item was found to be defective

$P (E_{1}) = 60 % = \frac{3}{5}$

$P (E_{2}) = 40 % = \frac{2}{5}$

$P (X / E_{1}) = P$ (items produced by machine $A$ which is defective) $= 2 % = \frac{2}{100}$

$P (X / E_{2}) = P$ ( items produced by machine $B$ which is defective) $= 1 % = \frac{1}{100}$

Therefore, by Baye’s theorem,

$P (E_{2} / X) =$ probability that the randomly selected item was from machine $B$ ,which is defective,

$P (E_{2} / X) = \frac{P (E_{2}) . P (X / E_{2})}{P (E_{1}) . P (X / E_{1}) + P (E_{2}) . P (X / E_{2})}$

$= \frac{\frac{2}{5} \times \frac{1}{100}}{\frac{3}{5} \times \frac{2}{100} + \frac{2}{5} \times \frac{1}{100}}$

$= \frac{\frac{2}{500}}{\frac{6}{500} + \frac{2}{500}}$

$= \frac{2}{500} \times \frac{500}{8} = \frac{1}{4}$

Q9.Two groups are competing for the position on the Board of directors of a corporation. The probabilities that the first and the second groups will win are 0.6 and 0.4 respectively. Further, if the first group wins, the probability of introducing a new product is 0.7 and the corresponding probability if 0.3, if the second group wins. Find the probability that the new product introduced was by the second group.

A.9. Let, $E_{1} :$ event that first group will win

$E_{2} :$ event that second group will win

$A :$ event that new product will produce

$P (E_{1}) = 0.6 = \frac{6}{10} = \frac{3}{5}$

$P (E_{2}) = 0.4 = \frac{4}{10} = \frac{2}{5}$

$P (A / E_{1}) = P$ (introducing new product by group $A$ ) $= 0.7 = \frac{7}{10}$

$P (A / E_{2}) = P$ ( introducing new product by group $B$ ) $= 0.3 = \frac{3}{10}$

Therefore, by Baye’s theorem,

$P (E_{2} / A) =$ probability that new product introduced was produced by second group

$P (E_{2} / A) = \frac{P (E_{2}) . P (A / E_{2})}{P (E_{1}) . P (A / E_{1}) + P (E_{2}) . P (A / E_{2})}$

$= \frac{\frac{2}{5} \times \frac{3}{10}}{\frac{3}{7} \times \frac{7}{10} + \frac{2}{5} \times \frac{3}{10}}$

$= \frac{\frac{6}{50}}{\frac{21}{50} + \frac{6}{50}}$

$= \frac{6}{50} \times \frac{50}{27} = \frac{2}{9}$

Q10.Suppose a girl throws a die. If she gets a 5 or 6, she tosses a coin three times and notes the number of heads. If she gets 1, 2, 3 or 4 she tosses a coin once and noted whether a head or tail is obtained. If she obtained exactly one head, what is the probability that she threw 1, 2, 3 and 4 with the die?

A.10. Let, $E_{1} :$ event that outcome on the die is $5$ or $6$

$E_{2} :$ event that outcome on the die is $1, 2, 3$ or $4$

$A :$ event of getting exactly one head

$P (E_{1}) = \frac{2}{6} = \frac{1}{3}$

$P (E_{2}) = \frac{4}{6} = \frac{2}{3}$

$P (A / E_{1}) =$ probability of getting exactly one head by tossing the coin three times if she gets $5$ or $6$ $= \frac{3}{8}$

$P (A / E_{2}) =$ probabilit7y of getting exactly one head by tossing the coin three times if she gets $1, 2, 3$ or $4$ $= \frac{1}{2}$

Therefore, by Baye’s theorem,

$P (E_{2} / A) =$ probability that the girl threw $1, 2, 3$ or $4$ with die, if she obtained exactly one head,

$P (E_{2} / A) = \frac{P (E_{2}) . P (A / E_{2})}{P (E_{1}) . P (A / E_{1}) + P (E_{2}) . P (A / E_{2})}$

$= \frac{\frac{2}{3} \times \frac{1}{2}}{\frac{1}{3} \times \frac{3}{8} + \frac{2}{3} \times \frac{1}{2}}$

$= \frac{\frac{2}{6}}{\frac{3}{24} + \frac{1}{3}} = \frac{\frac{1}{3}}{\frac{3 + 8}{24}}$

$= \frac{1}{3} \times \frac{24}{10} = \frac{8}{11}$

Q11.A manufacturer has three machine operators A, B and C. The first operator A produces 1% defective items, whereas the other two operators B and C produce 5% and 7% defective items respectively. A is on the job for 50% of the time, B on the job for 30% of the time and C on the job for 20% of the time. A defective item is produced, what is the probability that it was produced by A?

A.11. Let, $E_{1} :$ event of time consume by $A$

$E_{2} :$ event of time consume by $B$

$E_{3} :$ event of time consume by $C$

$P (E_{1}) = 50 % = \frac{50}{100} = \frac{1}{2}$

$P (E_{2}) = 30 % = \frac{30}{100} = \frac{3}{10}$

$P (E_{3}) = 20 % = \frac{20}{100} = \frac{1}{5}$

Let, $A :$ event of producing the defective item. Therefore,

$P (A / E_{1}) = 1 % = \frac{1}{100}$

$P (A / E_{2}) = 5 % = \frac{5}{100}$

$P (A / E_{3}) = 7 % = \frac{7}{100}$

Therefore, by Baye’s theorem,

$P (E_{1} / A) =$ probability that the defective item was produced by $A$ ,

$P (E_{1} / A) = \frac{P (E_{1}) . P (A / E_{1})}{P (E_{1}) . P (A / E_{1}) + P (E_{2}) . P (A / E_{2}) + P (E_{3}) . P (A / E_{3})}$

$= \frac{\frac{1}{2} \times \frac{1}{100}}{\frac{1}{2} \times \frac{1}{100} + \frac{3}{10} \times \frac{5}{100} + \frac{1}{5} \times \frac{7}{100}}$

$= \frac{\frac{1}{2} \times \frac{1}{100}}{\frac{1}{100} (\frac{1}{2} + \frac{15}{10} + \frac{7}{5})} = \frac{\frac{1}{2} \times \frac{1}{100}}{\frac{1}{100} (\frac{5 + 15 + 14}{10})} = \frac{\frac{1}{2}}{\frac{34}{10}}$

$= \frac{1}{2} \times \frac{10}{34} = \frac{5}{34}$

Q12.A card from a pack of 52 cards is lost. From the remaining cards of the pack, two cards are drawn and are found to be both diamonds. Find the probability of the lost card being a diamond.

A.12. Let, $E_{1} :$ event of choosing a diamond card

$E_{2} :$ event of choosing a card which is not diamond

$A :$ denote the lost card, out of $52$ cards

We know,

$13$ cards are diamond

$39$ cards are not diamond

$P (E_{1}) = \frac{13}{52} = \frac{1}{4}$

$P (E_{2}) = \frac{39}{52} = \frac{3}{4}$

When one diamond card is lost, there are $12$ diamond cards out of $51$ cards, two cards can be drawn out of $12$ diamond cards in $12$ ${C_{2}}$ ways. Similarly, $2$ diamond cards can be drawn out of $51$ cards in $51$ ${C_{2}}$ ways.

The probability of getting two cards, when one diamond card is lost, is given by $P (A / E_{1}),$

$P (A / E_{1}) = \frac{{}_{12}{C_{2}}}{{}_{51}{C_{2}}} = \frac{12!}{2! \times 10!} \times \frac{2! \times 49!}{51!}$

$= \frac{11 \times 12}{50 \times 51} = \frac{22}{425}$

By using Baye’s theorem,

$P (E_{1} / A) =$ probability that the lost card is diamond, given that the card is lost

$P (E_{1} / A) = \frac{P (E_{1}) . P (A / E_{1})}{P (E_{1}) . P (A / E_{1}) + P (E_{2}) . P (A / E_{2})}$

$= \frac{\frac{1}{4} \times \frac{22}{425}}{\frac{1}{4} \times \frac{22}{425} + \frac{3}{4} \times \frac{26}{425}}$

$= \frac{\frac{1}{4} \times \frac{22}{425}}{\frac{1}{425} (\frac{22}{4} + \frac{26 \times 3}{4})}$

$= \frac{11}{2} \times \frac{4}{100} = \frac{11}{50}$

Q13. Probability that A speaks truth is 4/5 A coin is tossed. A report that a head appears. The probability that actually there was head is:

(A) 4/5

(B) 1/2

(C) 1/5

(D) 2/5

A.13. Let, $E_{1}$ and $E_{2}$ be the event such that

$E_{1} :$ $A$ speak truth

$E_{2} :$ $A$ speak false

$X :$ that head appears

$P (E_{1}) = \frac{4}{5}$ and $P (E_{2}) = 1 - P (E_{1})$ = $= 1 - \frac{4}{5} = \frac{1}{5}$

If a coin tossed, then it may result either head $(H)$ or tail $(T)$ . The probability of getting a head is $\frac{1}{2}$ whether $A$ speak truth or not.

$P (X / E_{1}) = P (X / E_{2}) = \frac{1}{2}$

The probability that there are actually a head is given by $P (E_{1} / X)$

$P (E_{1} / X) = \frac{P (E_{1}) . P (X / E_{1})}{P (E_{1}) . P (X / E_{1}) + P (E_{2}) . P (X / E_{2})}$

$= \frac{\frac{4}{5} \times \frac{1}{2}}{\frac{4}{5} \times \frac{1}{2} + \frac{4}{5} \times \frac{1}{2}}$

$= \frac{\frac{4}{5} \times \frac{1}{2}}{\frac{1}{2} (\frac{4}{5} + \frac{1}{5})} = \frac{4}{5}$

Option $(A)$ is correct $\frac{4}{5}$

Q14.If A and B are two events such that A ⊂ B and P (B) ≠ 0, then which of the following is correct:

$\begin{array}{l} A . P (A | B) = \frac{P (B)}{P (A)} \\ B . P (A | B) < P (A) \\ C . P (A | B) \leq P (A) \\ D . N o n e o f t h e s e \end{array}$

A.14. If $A \subset B,$ then $A \cap B = A$

$P (A \cap B) = P (A)$ also $P (A) < P (B)$

Consider,

$P (A / B) = \frac{P (A \cap B)}{P (B)} = \frac{P (A)}{P (B)} \neq \frac{P (B)}{P (A)}$

$\Rightarrow P (A / B) = \frac{P (A \cap B)}{P (B)} = \frac{P (A)}{P (B)}$ $_____(1)$

We know, $P (B) \leq 1$ $\Rightarrow \frac{1}{P (B)} \geq 1$

From eq. $(1)$ , we have

$\frac{P (A)}{P (B)} \geq P (A)$

$\Rightarrow P (A / B) \geq P (A)$ $_____(2)$

$\Rightarrow P (A / B)$ is not less than $P (A)$

Hence, from eq. $(2)$ it can be concluded that the relation given in alternative $(C)$ is correct.

Ex 13.4

Q1. State which of the following are not the probability distributions of a random variable. Give reasons for your answer.

(i)

X	0	1	2
P (X)	0.4	0.4	0.2

(ii)

X	0	1	2	3	4
P (X)	0.1	0.5	0.2	– 0.1	0.3

(iii)

Y	– 1	0	1
P (Y)	0.6	0.1	0.2

(iii)

Y	– 1	0	1
P (Y)	0.6	0.1	0.2

(iv)

Z	3	2	1	0	– 1
P (Z)	0.3	0.2	0.4	0.1	0.05

A.1. It is known that the sum of all the probabilities in a probability distribution is one.

(i) Sum of the probabilities = 0.4 + 0.4 + 0.2 = 1

Therefore, the given table is a probability distribution of random variables.

(ii) It can be seen that for X = 3, P (X) = −0.1

It is known that probability of any observation is not negative. Therefore, the given table is not a probability distribution of random variables.

(iii) Sum of the probabilities = 0.6 + 0.1 + 0.2 = 0.9 ≠ 1

Therefore, the given table is not a probability distribution of random variables.

(iv) Sum of the probabilities = 0.3 + 0.2 + 0.4 + 0.1 + 0.05 = 1.05 ≠ 1

Therefore, the given table is not a probability distribution of random variables.

Q2. An urn contains 5 red and 2 black balls. Two balls are randomly selected. Let X represents the number of black balls. What are the possible values of X? Is X a random variable?

A.2. There two balls may be selected as BR, RB, BR, BB, where R represents red ball and B represents black ball.

Variable X has the value 0, 1, 2, i.e., there may be no black ball, may be one black ball or both the balls are black.

Q3. Let X represents the difference between the number of heads and the number of tails obtained when a coin is tossed 6 times. What are possible values of X?

A.3 A coin is tossed six times and X represents the difference between the number of heads and the number of tails.

∴ X (6 H, 0T) = |6 - 0| = 6

X (5 H, 1 T) = |5 - 1| = 4

X (4 H, 2 T) = |4 - 2| = 2

X (3 H, 3 T) = 3 - 3| = 0

X (2 H, 4 T) = |2 - 4| = 2

X (1 H, 5 T) = |1 - 5| = 4

X (0H, 6 T) = | 0 - 6| = 6

Thus, the possible values of X are 6, 4, 2, and 0.

Q4. Find the probability distribution of:

(i) Number of heads in two tosses of a coin.

(ii) Number of tails in the simultaneous tosses of three coins.

(iii) Number of heads in four tosses of a coin.

A.4. (i) When one coin is tossed twice, the sample space is

{HH, HT, TH, TT}

Let X represent the number of heads.

∴ X (HH) = 2, X (HT) = 1, X (TH) = 1, X (TT) = 0

Therefore, X can take the value of 0, 1, or 2.

It is known that,

P(HH) = P(HT) = P(TH) = P(TT) = 1/4

P (X = 0) = P (TT) = 1/4

P (X = 1) = P (HT) + P (TH) = 14 + 1/4 = 1/2

P (X = 2) = P (HH) = 1/4

Thus, the required probability distribution is as follows.

X	0	1	2
P (X)	1/4	1/2	1/4

(ii) When three coins are tossed simultaneously, the sample space is {HHH, HHT, HTH, HTT, THH, THT, TTH, TTT}

Let X represent the number of tails.

It can be seen that X can take the value of 0, 1, 2, or 3.

P (X = 0) = P (HHH) = 1/8

P (X = 1) = P (HHT) + P (HTH) + P (THH) = 1/8 + 1/8 + 1/8 = 3/8

P (X = 2) = P (HTT) + P (THT) + P (TTH) = 1/8 + 1/8 + 1/8 = 3/8

P (X = 3) = P (TTT) = 1/8

Thus, the probability distribution is as follows.

X	0	1	2	3
P (X)	1/8	3/8	3/8	1/8

(iii) When a coin is tossed four times, the sample space is

S = {HHHH,HHHT,HHTH,HHTT,HTHT,HTHH,HTTH,HTTT,THHH,THHT,THTH,THTT,TTHH,TTHT,TTTH,TTTT}

Let X be the random variable, which represents the number of heads.

It can be seen that X can take the value of 0, 1, 2, 3, or 4.

P (X = 0) = P (TTTT) = 1/16

P (X = 1) = P (TTTH) + P (TTHT) + P (THTT) + P (HTTT)

= 1/16 + 1/16 + 1/16 + 1/16 = 4/16 = 1/4

P (X = 2) = P (HHTT) + P (THHT) + P (TTHH) + P (HTTH) + P (HTHT)

+ P (THTH)

= 1/16 + 1/16 + 1/16 + 1/16 + 1/16 + 1/16 = 6/16 = 3/8

P (X = 3) = P (HHHT) + P (HHTH) + P (HTHH) P (THHH)

= 1/16 + 1/16 + 1/16 + 1/16 = 4/16 = 1/4

P (X = 4) = P (HHHH) = 1/16

Thus, the probability distribution is as follows.

X	0	1	2	3	4
P (X)	1/16	1/4	3/8	1/4	1/16

Q5. Find the probability distribution of the number of success in two tosses of a die where a success is defined as:

(i) number greater than 4.

(ii) six appears on at least one die.

A.5. When a die is tossed two times, we obtain (6 × 6) = 36 number of observations.

Let X be the random variable, which represents the number of successes.

(i) Here, success refers to the number greater than 4.

P (X = 0) = P (number less than or equal to 4 on both the tosses) = 4/6 X 4/6 = 4/9

P (X = 1) = P (number less than or equal to 4 on first toss and greater than 4 on second toss) + P (number greater than 4 on first toss and less than or equal to 4 on second toss)

= 4/6 X 2/6 + 4/6 X 2/6 = 4/9

P (X = 2) = P (number greater than 4 on both the tosses)

= 2/6 X 2/6 = 1/9

Thus, the probability distribution is as follows.

X	0	1	2
P (X)	4/9	4/9	1/9

(ii) Here, success means six appears on at least one die.P (Y = 0 ) = P (six appears on none of the dice) = 5/6 x 5/6 = 25/36

P (Y = 1) = P (six appears on at least one of the dice) = 1/6 x 5/6 + 5/6 x 1/6 + 1/6 x 1/6 = 11/36

Thus, the required probability distribution is as follows.

Y	0	1
P (Y)	25/36	11/26

Q6. From a lot of 30 bulbs which include 6 defectives, a sample of 4 bulbs is drawn at random with replacement. Find the probability distribution of the number of defective bulbs.

A.6. It is given that out of 30 bulbs, 6 are defective.

⇒ Number of non-defective bulbs = 30 − 6 = 24

4 bulbs are drawn from the lot with replacement.

Let X be the random variable that denotes the number of defective bulbs in the selected bulbs.

$∴ P (X = 0) = P$ (4 non-defective and 0 defective) $=^{4} C_{0} . \frac{4}{5} . \frac{4}{5} . \frac{4}{5} . \frac{4}{5} = \frac{256}{625}$

$P (X = 1) = P$ (3 non-defective and 1 defective) $=^{4} C_{1} . (\frac{1}{5}) . {(\frac{4}{5})}^{3} = \frac{256}{625}$

$P (X = 2) = P$ (2 non-defective and 2 defective) $=^{4} C_{2} . {(\frac{1}{5})}^{2} . {(\frac{4}{5})}^{2} = \frac{96}{625}$

$P (X = 3) = P$ (1 non-defective and 3 defective) $=^{4} C_{3} . {(\frac{1}{5})}^{3} . (\frac{4}{5}) = \frac{16}{625}$

$P (X = 4) = P$ (0 non-defective and 4 defective) $=^{4} C_{4} . {(\frac{1}{5})}^{4} . {(\frac{4}{5})}^{0} = \frac{1}{625}$

Therefore, the required probability distribution is as follows.

X	0	1	2	3	4
P (X)	256/625	256/625	96/625	16/625	1/625

Q7. A coin is biased so that the head is 3 times as likely to occur as tail. If the coin is tossed twice, find the probability distribution of number of tails.

A.7. Let the probability of getting a tail in the biased coin be x.

∴ P (T) = x

⇒ P (H) = 3x

For a biased coin, P (T) + P (H) = 1

$\begin{array}{l} \Rightarrow x + 3 x = 1 \\ \Rightarrow 4 x = 1 \\ \Rightarrow x = \frac{1}{4} \\ ∴ P (T) = \frac{1}{4}, a n d, P (H) = \frac{3}{4} \end{array}$

When the coin is tossed twice, the sample space is {HH, TT, HT, TH}.

Let X be the random variable representing the number of tails.

∴ P (X = 0) = P (no tail) = P (H) × P (H) = 3/4 x 3/4 = 9/16

P (X = 1) = P (one tail) = P (HT) + P (TH)

= 3/4 . 1/4 + 1/4 . 3/4

= 3/16 + 3/16= 3/8

P (X = 2) = P (two tails) = P (TT) = 1/4 x 1/4 = 1/16

Therefore, the required probability distribution is as follows.

X	0	1	2
P (X)	9/16	3/8	1/16

Q.8. A random variable X has the following probability distribution:

X	0	1	2	3	4	5	6	7
P (X)	0	k	2k	2k	3k	k²	2k²	7k²+ k

Determine:

(i) k

(ii) P (X < 3)

(iii) P (X > 6)

(iv) P (0 < X < 3)

A.8. (i) Since, the sum of all the probabilities of a distribution is 1.

$\begin{array}{l} \Rightarrow 0 + k + 2 k + 2 k + 3 k + k^{2} + 2 k^{2} + (7 k^{2} + k) = 1 \\ \Rightarrow 10 k^{2} + 9 k = 0 \\ \Rightarrow (10 k - 1) (k + 1) = 0 \\ \Rightarrow k = - 1, \frac{1}{4} \end{array}$

K=-1 is not possible as the probability of an event is never negative.

$∴ k = \frac{1}{10}$

(ii) $P (X < 3) = P (X = 0) + P (X = 1) + P (X = 2)$

$\begin{array}{l} = 0 + k + 2 k \\ = 3 k \\ = 3 \times \frac{1}{10} \\ = \frac{3}{10} \end{array}$

(iii) $P (X > 6) = P (X = 7)$

$\begin{array}{l} = 7 k^{2} + k \\ = 7 \times {(\frac{1}{10})}^{2} + \frac{1}{10} \\ = \frac{7}{100} + \frac{1}{10} \\ = \frac{17}{100} \end{array}$

(iv) $P (0 < X < 3) = P (X = 1) + P (X = 2)$

$\begin{array}{l} = k + 2 k \\ = 3 k \\ = 3 \times \frac{1}{10} \\ = \frac{3}{10} \end{array}$

Q9. The random variable X has a probability distribution P(X) of the following form, where k is some number:

$P (X) = {\begin{cases} k, i f, x = 0 \\ 2 k, i f, x = 1 \\ 3 k, i f, x = 2 \\ 0, o t h e r w w i s e \end{cases}$

(a) Determine the value of k.

(b) Find P(X < 2), P(X ≥ 2), P(X ≥ 2).

A.9. (a) It is known that the sum of probabilities of a probability distribution of random variables is one.

∴ k + 2k + 3k + 0 = 1

⇒ 6k = 1

⇒ k =1/6

(b) P(X < 2) = P(X = 0) + P(X = 1)

= k + 2k

= 3k

=3/6 = 1/2

$\begin{array}{l} P (X \leq 2) = P (X = 0) + P (X = 1) + P (X = 2) \\ = k + 2 k + 3 k \\ = 6 k \\ = \frac{6}{6} \\ = 1 \end{array}$

$\begin{array}{l} P (X \geq 2) = P (X = 2) + P (X > 2) \\ = 3 k + 0 \\ = 3 k \\ = \frac{3}{6} \\ = \frac{1}{2} \end{array}$

Q10. Find the mean number of heads in three tosses of fair coin.

A.10. Let X denote the success of getting heads.

Therefore, the sample space is

S = {HHH, HHT, HTH, HTT, THH, THT, TTH, TTT}

It can be seen that X can take the value of 0, 1, 2, or 3

$\begin{array}{l} ∴ P (X = 0) = P (T T T) \\ = P (T) . P (T) . P (T) \\ = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \\ = \frac{1}{8} \end{array}$

$\begin{array}{l} ∴ P (X = 1) = P (H H T) + P (H T H) + P (T H H) \\ = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \\ = \frac{3}{8} \end{array}$

$\begin{array}{l} ∴ P (X = 2) = P (H H T) + P (H T H) + P (T H H) \\ = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \\ = \frac{3}{8} \end{array}$

$\begin{array}{l} ∴ P (X = 3) = P (H H H) \\ = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \\ = \frac{1}{8} \end{array}$

Therefore, the required probability distribution is as follows.

X	0	1	2	3
P(X)	1/8	3/8	3/8	1/8

Mean of $X E (X), μ = \sum_{}^{} X_{i} P (X_{i})$

$\begin{array}{l} = 0 \times \frac{1}{8} + 1 \times \frac{3}{8} + 2 \times \frac{3}{8} + 3 \times \frac{1}{8} \\ = \frac{3}{8} + \frac{3}{4} + \frac{3}{8} \\ = \frac{3}{2} \\ = 1.5 \end{array}$

Q11. Two dice are thrown simultaneously. If X denotes the number of sixes, find expectation of X.

A.11. Two dice thrown simultaneously is the same the die thrown 2 times.

Here, X represents the number of sixes obtained when two dice are thrown simultaneously. Therefore, X can take the value of 0, 1, or 2.

∴ P (X = 0) = P (not getting six on any of the dice) = 25/36

P (X = 1) = P (six on first die and no six on second die) + P (no six on first die and six on second die)

=2(1/6 x 5/6) = 10/36

P (X = 2) = P (six on both the dice) =1/36

Therefore, the required probability distribution is as follows.

X	0	1	2
P(X)	25/36	10/36	1/36

Then, expectation of X = E(X) =∑ X iP(Xi)

= 0 x 25/36 + 1 x 10/36 + 2 x 1/36

= 1/3

Q12. Two numbers are selected at random (without replacement), from the first six positive integers. Let X denotes the larger of two numbers obtained. Find E (X).

A.12. The two positive integers can be selected from the first six positive integers without replacement in 6 × 5 = 30 ways

X represents the larger of the two numbers obtained. Therefore, X can take the value of 2, 3, 4, 5, or 6.

For X = 2, the possible observations are (1, 2) and (2, 1).

P(X = 2) $= \frac{2}{30} = \frac{1}{15}$

For X = 3, the possible observations are (1, 3), (2, 3), (3, 1), and (3, 2).

P(X = 3) $= \frac{4}{30} = \frac{2}{15}$

For X = 4, the possible observations are (1, 4), (2, 4), (3, 4), (4, 3), (4, 2), and (4, 1).

P(X = 4) $= \frac{6}{30} = \frac{1}{5}$

For X = 5, the possible observations are (1, 5), (2, 5), (3, 5), (4, 5), (5, 4), (5, 3), (5, 2), and (5, 1).

P(X = 5) $= \frac{8}{30} = \frac{4}{15}$

For X = 6, the possible observations are (1, 6), (2, 6), (3, 6), (4, 6), (5, 6), (6,5) , (6, 4), (6, 3), (6, 2), and (6, 1).

P(X = 6) $= \frac{10}{30} = \frac{1}{3}$

Therefore, the required probability distribution is as follows.

X	2	3	4	5	6
P(X)	1/15	2/15	1/5	4/15	1/3

$\begin{array}{l} T h e n, E (X) = \sum_{}^{} X_{i} P (X_{i}) \\ = 2 . \frac{1}{5} + 3 . \frac{2}{15} + 4 . \frac{1}{5} + 5 . \frac{4}{15} + 6 . \frac{1}{3} \\ = \frac{2}{15} + \frac{2}{5} + \frac{4}{5} + \frac{4}{3} + 3 \\ = \frac{70}{15} \\ = \frac{14}{3} \end{array}$

Q13. Let X denotes the sum of the numbers obtained when two fair dice are rolled. Find the variance and standard deviation of X.

A.13. When two fair dice are rolled, 6 × 6 = 36 observations are obtained.

P(X = 2) = P(1, 1) = 1/36

P(X = 3) = P (1, 2) + P(2, 1) = 2/36 = 1/18

P(X = 4) = P(1, 3) + P(2, 2) + P(3, 1) = 3/36 = 1/12

P(X = 5) = P(1, 4) + P(2, 3) + P(3, 2) + P(4, 1) = 4/36 = 1/9

P(X = 6) = P(1, 5) + P (2, 4) + P(3, 3) + P(4, 2) + P(5, 1) = 5/36

P(X = 7) = P(1, 6) + P(2, 5) + P(3, 4) + P(4, 3) + P(5, 2) + P(6, 1) = 6/36 = 1/6

P(X = 8) = P(2, 6) + P(3, 5) + P(4, 4) + P(5, 3) + P(6, 2) = 5/36

P(X = 9) = P(3, 6) + P(4, 5) + P(5, 4) + P(6, 3) = 4/36 = 1/9

P(X = 10) = P(4, 6) + P(5, 5) + P(6, 4) = 3/36 = 1/12

P(X = 11) = P(5, 6) + P(6, 5) = 2/36 = 1/18

P(X = 12) = P(6, 6) = 1 x 36

Therefore, the required probability distribution is as follows.

X	2	3	4	5	6	7	8	9	10	11	12
P(X)	1/36	1/18	1/12	1/9	5/36	1/6	5/36	1/9	1/12	1/18	1/36

$\begin{array}{l} T h e n, E (X) = \sum_{}^{} X_{i} . P (X_{i}) \\ = 2 \times \frac{1}{36} + 3 \times \frac{1}{18} + 4 \times \frac{1}{12} + 5 \times \frac{1}{9} + 6 \times \frac{5}{36} + 7 \times \frac{1}{6} + 8 \times \frac{5}{36} + 9 \times \frac{1}{9} + 10 \times \frac{1}{12} + 11 \times \frac{1}{18} + 12 \times \frac{1}{36} \\ = \frac{1}{18} + \frac{1}{6} + \frac{1}{3} + \frac{5}{9} + \frac{5}{6} + \frac{7}{6} + \frac{10}{9} + 1 + \frac{5}{6} + \frac{11}{18} + \frac{1}{3} \\ = 7 \end{array}$

$\begin{array}{l} E (X^{2}) - \sum_{}^{} {X^{2}}_{i} . P (X_{i}) \\ = 4 \times \frac{1}{36} + 9 \times \frac{1}{18} + 16 \times \frac{1}{12} + 25 \times \frac{1}{9} + 36 \times \frac{5}{36} + 49 \times \frac{1}{6} + 64 \times \frac{5}{36} + 81 \times \frac{1}{9} + 100 \times \frac{1}{12} + 121 \times \frac{1}{18} + 144 \times \frac{1}{36} \\ = \frac{1}{9} + \frac{1}{2} + \frac{4}{3} + \frac{25}{9} + 5 + \frac{49}{6} + \frac{80}{9} + 9 + \frac{25}{3} + \frac{121}{18} + 4 \\ = \frac{987}{18} = \frac{329}{6} = 54.833 \end{array}$

$\begin{array}{l} T h e n, V a r (X) = E (X^{2}) - [E {(X)}^{2}] \\ = 54.833 - {(7)}^{2} \\ = 54.833 - 49 \\ = 5.833 \end{array}$

$∴$ Standard derivation $\begin{array}{l} =\sqrtvar(X) \\ =\sqrt5.833 \\ = 2.415 \end{array}$

Q14. A class has 15 students whose ages are 14, 17, 15, 14, 21, 17, 19, 20, 16, 18, 20, 17, 16, 19 and 20 years. One student is selected in such a manner that each has the same chance of being chosen and the age X of the selected student is recorded. What is the probability distribution of the random variable X? Find mean, variance and standard deviation of X.

A.14. There are 15 students in the class. Each student has the same chance to be chosen. Therefore, the probability of each student to be selected is 1/15.

The given information can be compiled in the frequency table as follows.

14	15	16	17	18	19	20	21
2	1	2	3	1	2	3	1

P(X = 14) =2/15, P(X = 15) =1/15, P(X = 16) =2/15, P(X = 16) =3/15,

P(X = 18) =115, P(X = 19) =2/15, P(X = 20) =3/15, P(X = 21) =1/15

Therefore, the probability distribution of random variable X is as follows.

X	14	15	16	17	18	19	20	21
f	2/15	1/15	2/15	3/15	1/15	2/15	3/15	1/15

Then, mean of X = E(X)

$\begin{array}{l} = \sum_{}^{} X_{i} P (X_{i}) \\ = 14 \times \frac{2}{15} + 15 \times \frac{1}{15} + 16 \times \frac{2}{15} + 17 \times \frac{3}{15} + 18 \times \frac{1}{15} + 19 \times \frac{2}{15} + 20 \times \frac{3}{15} + 21 \times \frac{1}{15} \\ = \frac{1}{15} (28 + 15 + 32 + 51 + 18 + 38 + 60 + 21) \\ = \frac{263}{15} \\ = 17.53 \end{array}$

$\begin{array}{l} E (X^{2}) = \sum_{}^{} {X^{2}}_{i} P (X_{i}) \\ = {(14)}^{2} . \frac{2}{15} + {(15)}^{2} . \frac{1}{15} + {(16)}^{2} . \frac{2}{15} + {(17)}^{2} . \frac{3}{15} + {(18)}^{2} . \frac{1}{15} + {(19)}^{2} . \frac{2}{15} + {(20)}^{2} . \frac{3}{15} + {(21)}^{2} . \frac{1}{15} \\ = \frac{1}{15} . (392 + 225 + 512 + 867 + 324 + 722 + 1200 + 441) \\ = \frac{4683}{15} \\ = 312.2 \end{array}$

$\begin{array}{l} ∴ V a r i a n c e (X) = E (X^{2}) - {[E (X)]}^{2} \\ = 312.2 - {(\frac{263}{15})}^{2} \\ = 312.2 - 307.4177 \\ = 4.7823 \\ \approx 4.78 \end{array}$

Standard derivation $\begin{array}{l} =\sqrtvariance (X) \\ =\sqrt4.78 \\ = 2.186 \approx 2.19 \end{array}$

Q15. In a meeting 70% of the members favour a certain proposal, 30% being opposed. A member is selected at random and we let X = 0 if the opposed and X = 1 if he is in favour. Find E (X) and Var (X).

A.15. It is given that P(X = 0) = 30% = 30/100 = 0.3

P(X = 1) = 70% = 70/100 = 0.7

Therefore, the probability distribution is as follows.

X	0	1
P(X)	0.3	0.7

$\begin{array}{l} T h e n, E (X) = \sum_{}^{} X_{i} P (X_{i}) \\ = 0 \times 0.3 + 1 \times 0.7 \\ = 0.7 \end{array}$

$\begin{array}{l} E (X^{2}) = \sum_{}^{} {X^{2}}_{i} P (X_{i}) \\ = 0^{2} \times 0.3 + {(1)}^{2} \times 0.7 \\ = 0.7 \end{array}$

It is know that, $V a r (X) = E (X^{2}) - {[E (X)]}^{2}$

= 0.7 − (0.7)²

= 0.7 − 0.49

= 0.21

Choose the correct answer in each of the following:

Q.16. The mean of the numbers obtained on throwing a die having written 1 on three faces, 2 on two faces and 5 on one face is:

(A) 1

(B) 2

(C) 5

(D) $\frac{8}{3}$

A.16. Let X be the random variable representing a number on the die.

The total number of observations is six.

$\begin{array}{l} ∴ P (X = 1) = \frac{3}{6} = \frac{1}{2} \\ P (X = 2) = \frac{2}{6} = \frac{1}{3} \\ P (X = 5) = \frac{1}{6} \end{array}$

Therefore, the probability distribution is as follows.

X	1	2	5
P(X)	1/2	1/3	1/6

$M e a n = E (X) = \sum_{}^{} P_{i} X_{i}$

$\begin{array}{l} = \frac{1}{2} \times 1 + \frac{1}{3} \times 2 + \frac{1}{6} \times 5 \\ = \frac{1}{2} + \frac{2}{3} + \frac{5}{6} \\ = \frac{3 + 4 + 5}{6} \\ = \frac{12}{6} \\ = 2 \end{array}$

Therefore, option (B) is correct.

Q17. Suppose that two cards are drawn at random from a deck of cards. Let X be the number of aces obtained. What is the value of E(X)?

(A) $\frac{37}{221}$

(B) $\frac{5}{13}$

(C) $\frac{1}{13}$

(D) $\frac{2}{13}$

A.17. Let X denote the number of aces obtained. Therefore, X can take any of the values of 0, 1, or 2.

In a deck of 52 cards, 4 cards are aces. Therefore, there are 48 non-ace cards.

X	0	1	2
P(X)	1128/1326	192/1326	6/1326

$T h e n, E (X) = \sum_{}^{} P_{i} X_{i}$

$\begin{array}{l} = 0 \times \frac{1128}{1326} + 1 \times \frac{192}{1326} + 2 \times \frac{6}{1326} \\ = \frac{204}{1326} \\ = \frac{2}{13} \end{array}$

Therefore, option (D) is correct.

Probability Class 12 Solutions Exercise 13.5 & Miscellaneous Exercise

EX 13.5

Q1. A die is thrown 6 times. If ‘getting an odd number’ is a success, what is the probability of:

(i) 5 successes?

(ii) at least 5 successes?

(iii) at most 5 successes?

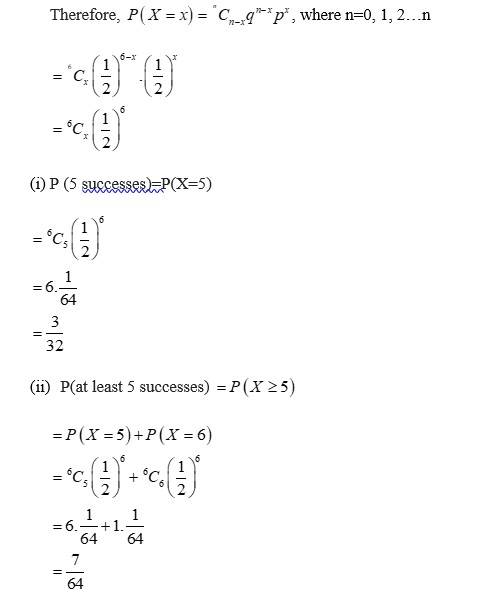
A.1. The repeated tosses of a die are Bernoulli trials. Let X denote the number of successes of getting odd numbers in an experiment of 6 trials.

Probability of getting an odd number in a single throw of a die is, p - 3/6 = 1/2

q = 1 - p = 1/2

X has a binomial distribution.

P(at most 5 successes) $= P (X \leq 5)$

$\begin{array}{l} = 1 - P (X > 5) \\ = 1 - P (X = 6) \\ = 1 - {6C}_{6} {(\frac{1}{2})}^{6} \\ = 1 - \frac{1}{64} \\ = \frac{63}{64} \end{array}$

Q2. A pair of dice is thrown 4 times. If getting a doublet is considered a success, find the probability of two successes.

A.2. The repeated tosses of a pair of dice are Bernoulli trials. Let X denote the number of times of getting doublets in an experiment of throwing two dice simultaneously four times.

Probability of getting doublets in a single throw of the pair of dice is

$\begin{array}{l} p = \frac{6}{36} = \frac{1}{6} \\ ∴ q = 1 - p = 1 - \frac{1}{6} = \frac{5}{6} \end{array}$

Clearly, X has the binomial distribution with n=4, $p = \frac{1}{6}$ , and $q = \frac{5}{6}$

$∴ P (X = x) =^{n} C_{x} q^{n - x} p^{x}$ , where $x = 0, 1, 2, 3 . . . n$

$\begin{array}{l} =4 {C_{x}} {(\frac{5}{6})}^{4 - x} . {(\frac{1}{6})}^{x} \\ =4 {C_{x}} . {\frac{5}{6^{4}}}^{4 - x} \end{array}$

$∴ P$ (2 successes) $= P (X = 2)$

$\begin{array}{l} = {4C}_{2} . {\frac{5}{6^{4}}}^{4 - 2} \\ = 6 . \frac{25}{1296} \\ = \frac{25}{216} \end{array}$

Q3. There are 5% defective items in a large bulk of items. What is the probability that a sample of 10 items will include not more than one defective item?

A.3. Let X denote the number of defective items in a sample of 10 items drawn successively. Since the drawing is done with replacement, the trials are Bernoulli trials.

$\begin{array}{l} \Rightarrow p = \frac{5}{100} = \frac{1}{20} \\ ∴ q = 1 - \frac{1}{20} = \frac{19}{20} \end{array}$

X has a binomial distribution with n=10 and $p = \frac{1}{20}$

$= {10C}_{x} {(\frac{19}{20})}^{10 - x} . {(\frac{1}{20})}^{x}$

P (not more than 1 defective item) $= P (X \leq 1)$

$\begin{array}{l} = P (X = 0) + P (X = 1) \\ = {10C}_{0} {(\frac{19}{20})}^{10} + {(\frac{1}{20})}^{0} +10 {C_{1}} {(\frac{19}{20})}^{9} + {(\frac{1}{20})}^{1} \\ = {(\frac{19}{20})}^{10} + 10 {(\frac{19}{20})}^{9} . (\frac{1}{20}) \\ = {(\frac{19}{20})}^{9} . [\frac{19}{20} + \frac{10}{20}] \\ = {(\frac{19}{20})}^{9} . (\frac{29}{20}) \\ = (\frac{29}{20}) . {(\frac{19}{20})}^{9} \end{array}$

Q 4. Five cards are drawn successively with replacement from a well-shuffled deck of 52 cards. What is the probability that:

(i) all the five cards are spade?

(ii) only 3 cards are spades?

(iii) none is spade?

A.4. Let X represent the number of spade cards among the five cards drawn. Since the drawing of card is with replacement, the trials are Bernoulli trials.

In a well shuffled deck of 52 cards, there are 13 spade cards.

$\begin{array}{l} \Rightarrow p = \frac{13}{52} = \frac{1}{4} \\ ∴ q = 1 - \frac{1}{4} = \frac{3}{4} \end{array}$

X has a binomial distribution with n=5 and $p = \frac{1}{4}$

$= {5C}_{x} {(\frac{3}{4})}^{5 - x} {(\frac{1}{4})}^{x}$

P (all five cards are spades) $= P (X = 5)$

$\begin{array}{l} = {5C}_{5} {(\frac{3}{4})}^{0} . {(\frac{1}{4})}^{5} \\ = 1 . \frac{1}{1024} \\ = \frac{1}{1024} \end{array}$

(ii) P (only 3 cards are spades) $= P (X = 3)$

$\begin{array}{l} =5 {C_{3}} {(\frac{3}{4})}^{2} . {(\frac{1}{4})}^{3} \\ = 10 . \frac{9}{16} . \frac{1}{64} \\ = \frac{45}{512} \end{array}$

(ii) P (none is a spades) $= P (X = 0)$

$\begin{array}{l} = {5C}_{0} {(\frac{3}{4})}^{5} . {(\frac{1}{4})}^{0} \\ = 1 . \frac{243}{1024} \\ = \frac{243}{1024} \end{array}$

Q5. The probability that a bulb produced by a factory will fuse after 150 days of use is 0.05. Find the probability that out of 5 such bulbs.

A.5. Let X represent the number of bulbs that will fuse after 150 days of use in an experiment of 5 trials. The trials are Bernoulli trials.

It is given that, p = 0.05

$∴ q = 1 - p = 1 - 0.05 = 0.95$

X has a binomial distribution with n=5 and $p = 0.05$

$= {5C}_{x} {(0.95)}^{5 - x} . {(0.05)}^{x}$

$P (n o n e) = P (X = 0)$

$\begin{array}{l} = {5C}_{0} {(0.95)}^{5} . {(0.05)}^{0} \\ = 1 \times {(0.95)}^{5} \\ = {(0.95)}^{5} \end{array}$

(ii) P (not more than one) $= P (X \leq 1)$

$\begin{array}{l} = P (X = 0) + P (X = 1) \\ =5 {C_{0}} {(0.95)}^{5} \times {(0.05)}^{0} + {5C}_{1} {(0.95)}^{4} \times {(0.05)}^{1} \\ = 1 \times {(0.95)}^{5} + 5 \times {(0.95)}^{4} \times {(0.05)}^{1} \\ = {(0.95)}^{5} + (0.05) {(0.95)}^{4} \\ = {(0.95)}^{4} \times 1.2 \end{array}$

(iii) P (more than 1) $= P (X > 1)$

$= 1 - P (X \leq 1)$

$= 1 - P$ (not more than 1)

$= 1 - {(0.95)}^{4} \times 1.2$

(iv) P (at least one) $= P (X \geq 1)$

$\begin{array}{l} = 1 - p (X < 1) \\ = 1 - p (X = 0) \\ = 1 - {5C}_{0} {(0.95)}^{5} \times {(0.05)}^{0} \\ = 1 - 1 \times {(0.95)}^{5} \\ = 1 - {(0.95)}^{5} \end{array}$

Q6. A bag consists of 10 balls each marked with one of the digits 0 to 9. If four balls are drawn successively with replacement from the bag, what is the probability that none is marked with the digit 0?

A.6. Let X denote the number of balls marked with the digit 0 among the 4 balls drawn.

Since the balls are drawn with replacement, the trials are Bernoulli trials.

X has a binomial distribution with n = 4 and p = 1/10

$\begin{array}{l} ∴ q = 1 - p = 1 - \frac{1}{10} = \frac{9}{10} \\ ∴ P (X = x) = {nC}_{x} q^{n - x} . p^{x}, x = 1, 2, . . . n \\ = {4C}_{x} {(\frac{9}{10})}^{4 - x} . {(\frac{1}{10})}^{x} \end{array}$

P (none marked with 0)=P(X=0)

$\begin{array}{l} = {4C}_{0} {(\frac{9}{10})}^{4} . {(\frac{1}{10})}^{0} \\ = 1 . {(\frac{9}{10})}^{4} \\ = {(\frac{9}{10})}^{4} \end{array}$

Q7. In an examination, 20 questions of true-false are asked. Suppose a student tosses a fair coin to determine his answer to each question. If the coin falls heads, he answers ‘true’, if it falls tails, he answers ‘false’. Find the probability that he answers at least 12 questions correctly.

A.7. Let X represent the number of correctly answered questions out of 20 questions.

The repeated tosses of a coin are Bernoulli trails. Since “head” on a coin represents the true answer and “tail” represents the false answer, the correctly answered questions are Bernoulli trials.

$\begin{array}{l} ∴ P = \frac{1}{2} \\ ∴ q = 1 - p = 1 - \frac{1}{2} = \frac{1}{2} \end{array}$

X has a binomial distribution with n=20 and $P = \frac{1}{2}$

$∴ P = (X = x) = {nC}_{x} q^{n - x} . p^{x},$ where $x = 0, 1, 2, . . . n$

$\begin{array}{l} = {20C}_{x} {(\frac{1}{2})}^{20 - x} . {(\frac{1}{2})}^{x} \\ = {20C}_{x} {(\frac{1}{2})}^{20} \end{array}$

P (at least 12 questions answered correctly) $= P (X \geq 12)$

$\begin{array}{l} = P (X = 12) + P (X = 13) + . . . + P (X = 20) \\ = {20C}_{12} {(\frac{1}{2})}^{20} + {20C}_{13} {(\frac{1}{2})}^{20} + . . . + {20C}_{20} {(\frac{1}{2})}^{20} \\ = {(\frac{1}{2})}^{20} . [20 {C_{12}} + {20C}_{13} + . . . + {20C}_{20}] \end{array}$

Q8. Suppose X has a binomial distribution B(6,1/2) Show that X = 3 is the most likely outcome.

(Hint: P(X = 3) is the maximum among all P (xi), xi = 0, 1, 2, 3, 4, 5, 6)

A.8. X is the random variable whose binomial distribution is B(6,1/2).

Therefore, n = 6 and p = ½

$\begin{array}{l} ∴ q = 1 - p = 1 - \frac{1}{2} = \frac{1}{2} \\ T h e n, P (X = x) = {nC}_{x} q^{n - x} p^{x} \\ = {6C}_{x} {(\frac{1}{2})}^{6 - x} . {(\frac{1}{2})}^{x} \\ = {6C}_{x} {(\frac{1}{2})}^{6} \end{array}$

It can be seen that P (X=x) will be maximum, if ${6C}_{x}$ will be maximum.

$\begin{array}{l} T h e n, {6C}_{0} = {6C}_{6} = \frac{6!}{0! 6!} = 1 \\ {6C}_{1} = {6C}_{5} = \frac{6!}{1! 5!} = 6 \\ {6C}_{2} = {6C}_{4} = \frac{6!}{2! 4!} = 15 \\ {6C}_{3} = \frac{6!}{3! 3!} = 20 \end{array}$

The value of ${6C}_{3}$ is maximum. Therefore, for x=3, P(X=x) is maximum.

Therefore, P(X=3) is maximum.

Q9. On a multiple choice examination with three possible answers for each of the five questions, what is the probability that a candidate would get four or more correct answers just by guessing?

A.9. The repeated guessing of correct answers from multiple choice questions are Bernoulli trials. Let X represent the number of correct answers by guessing in the set of 5 multiple choice questions.

Probability of getting a correct answer is, p = 1/3

$∴ q = 1 - p = 1 - \frac{1}{3} = \frac{2}{3}$

Clearly, X has a binomial distribution with n=5 and $P = \frac{1}{3}$

$\begin{array}{l} ∴ P = (X = x) = {nC}_{x} q^{n - x} p^{x} \\ = {5C}_{x} {(\frac{2}{3})}^{5 - x} . {(\frac{1}{3})}^{x} \end{array}$

P (guessing more than 4 correct answers) $= P (X \geq 4)$

$\begin{array}{l} = P (X = 4) + P (X = 5) \\ = {5C}_{4} (\frac{2}{3}) . {(\frac{1}{3})}^{4} + {5C}_{5} {(\frac{1}{3})}^{5} \\ = 5 . \frac{2}{3} . \frac{1}{81} + 1 . \frac{1}{243} \\ = \frac{10}{243} + \frac{1}{243} \\ = \frac{11}{243} \end{array}$

Q10. A person buys a lottery ticket in 50 lotteries, in each of which his chance of winning a prize is $\frac{1}{100}$ What is the probability that he will win a prize:

(a) at least once

(b) exactly once

(c) at least twice?

A.10. Let X represent the number of winning prizes in 50 lotteries. The trials are Bernoulli trials.

Clearly, X has a binomial distribution with n = 50 and p = 1/100

$\begin{array}{l} ∴ q = 1 - p = 1 - \frac{1}{100} = \frac{99}{100} \\ ∴ P (X = x) = {nC}_{x} q^{n - x} p^{x} = {50C}_{x} {(\frac{99}{100})}^{50 - x} . {(\frac{1}{100})}^{x} \end{array}$

P (winning at least once) $= P (X \geq 1)$

$\begin{array}{l} = 1 - P (X < 1) \\ = 1 - P (X = 1) \\ = 1 - {50C}_{0} {(\frac{99}{100})}^{50} \\ = 1 - 1 . {(\frac{99}{100})}^{50} \\ = 1 - {(\frac{99}{100})}^{50} \end{array}$

P (winning exactly once) $= P (X = 1)$

$\begin{array}{l} = {50C}_{1} {(\frac{99}{100})}^{49} . {(\frac{1}{100})}^{1} \\ = 50 (\frac{1}{100}) {(\frac{99}{100})}^{49} \\ = \frac{1}{2} {(\frac{99}{100})}^{49} \end{array}$

P (at least twice) $= P (X \geq 2)$

$\begin{array}{l} = 1 - P (X < 2) \\ = 1 - P (X \leq 1) \\ = 1 - [P (X = 0) + P (X = 1)] \\ = [1 - P (X = 0)] - P (X = 1) \\ = 1 - {(\frac{99}{100})}^{50} - \frac{1}{2} . {(\frac{99}{100})}^{49} \\ = 1 - {(\frac{99}{100})}^{49} . [\frac{99}{100} + \frac{1}{2}] \\ = 1 - {(\frac{99}{100})}^{49} . (\frac{149}{100}) \\ = 1 - (\frac{149}{100}) {(\frac{99}{100})}^{49} \end{array}$

Q11. Find the probability of getting 5 exactly twice in 7 throws of a die.

A.11. The repeated tossing of a die are Bernoulli trials. Let X represent the number of times of getting 5 in 7 throws of the die.

Probability of getting 5 in a single throw of the die, p = 1/6

$∴ q = 1 - p = 1 - \frac{1}{6} = \frac{5}{6}$

Clearly, X has the probability distribution with n=7 and $P = \frac{1}{6}$

$∴ P (X = x) = {nC}_{x} q^{n - x} p^{x} = {7C}_{x} {(\frac{5}{6})}^{7 - x} . {(\frac{1}{6})}^{x}$

P (getting 5 exactly twice) $= P (X = 2)$

$\begin{array}{l} = {7C}_{2} {(\frac{5}{6})}^{5} . {(\frac{1}{6})}^{2} \\ = 21 . {(\frac{5}{6})}^{5} . \frac{1}{36} \\ = (\frac{7}{12}) + {(\frac{5}{6})}^{5} \end{array}$

Q12. Find the probability of throwing at most 2 sixes in 6 throws of a single die.

A.12. The repeated tossing of the die are Bernoulli trials. Let X represent the number of times of getting sixes in 6 throws of the die.

Probability of getting six in a single throw of die, p = 1/6

Let A represents the favourable event i.e., 6

$∴ q = 1 - p = 1 - \frac{1}{6} = \frac{5}{6}$

Clearly, X has the probability distribution with n=6

$∴ P (X = x) = {nC}_{x} q^{n - x} p^{x} = {6C}_{x} {(\frac{5}{6})}^{6 - x} . {(\frac{1}{6})}^{x}$

P (at most 2 sixes) $= P (X \leq 2)$

$\begin{array}{l} = P (X = 0) + P (X = 1) + P (X = 2) \\ = {6C}_{0} {(\frac{5}{6})}^{6} + {6C}_{1} {(\frac{5}{6})}^{5} . (\frac{1}{6}) + {6C}_{2} {(\frac{5}{6})}^{4} . {(\frac{1}{6})}^{2} \\ = 1 . {(\frac{5}{6})}^{6} + 6 . \frac{1}{6} . {(\frac{5}{6})}^{5} + 15 . \frac{1}{36} . {(\frac{5}{6})}^{4} \\ = {(\frac{5}{6})}^{6} + {(\frac{5}{6})}^{5} + \frac{5}{12} . {(\frac{5}{6})}^{4} \\ = {(\frac{5}{6})}^{4} . [\frac{25}{36} + \frac{5}{6} + \frac{5}{12}] \\ = {(\frac{5}{6})}^{4} . [\frac{25 + 30 + 15}{36}] \\ = \frac{70}{36} . {(\frac{5}{6})}^{4} \\ = \frac{35}{18} . {(\frac{5}{6})}^{4} \end{array}$

Q13. It is known that 10% of certain articles manufactured are defective. What is the probability that in a random sample of 12 such articles 9 are defective?

A.13. The repeated selections of articles in a random sample space are Bernoulli trails. Let X denote the number of times of selecting defective articles in a random sample space of 12 articles.

Clearly, X has a binomial distribution with n = 12 and p = 10% = 10/100 = 1/10

$\begin{array}{l} ∴ q = 1 - p = 1 - \frac{1}{10} = \frac{9}{10} \\ ∴ P (X = x) = {nC}_{x} q^{n - x} p^{x} = {12C}_{x} {(\frac{9}{10})}^{12 - x} . {(\frac{1}{10})}^{x} \end{array}$

P (selecting 9 defective articles) $= {12C}_{9} {(\frac{9}{10})}^{3} . {(\frac{1}{10})}^{9}$

$\begin{array}{l} = 220 . \frac{9^{3}}{1 0^{3}} . \frac{1}{1 0^{9}} \\ = \frac{22 \times 9^{3}}{1 0^{11}} \end{array}$

In each of the following, choose the correct answer:

Q14. Binomial distribution is given this name because:

(A) This distribution was evolved by James binomial.

(B) Each trial has only two outcomes. Namely success and failure.

(C) Its probability function is obtained by general of binomial expansion.

(D) It is obtained by combining two distributions.

A.14. option (C) is correct.

Q15. In a box containing 100 bulbs, 10 are defective. The probability that out of a sample of 5 bulbs, none is defective is:

${5C}_{4} {(\frac{4}{5})}^{4} \frac{1}{5}$

${(\frac{4}{5})}^{4} \frac{1}{5}$

${5C}_{1} \frac{1}{5} {(\frac{4}{5})}^{4}$

None of these

A.15. The repeated selection of students who are swimmers are Bernoulli trials. Let X denote the number of students, out of 5 students, who are swimmers.

Probability of students who are not swimmers, q = 1/5

$∴ p = 1 - q = 1 - \frac{1}{5} = \frac{4}{5}$

Clearly, X has a binomial distribution with n=5 and $p = \frac{4}{5}$

$P (X = x) = {nC}_{x} q^{n - x} p^{x} =5 {C_{x}} {(\frac{1}{5})}^{5 - x} . {(\frac{4}{5})}^{x}$

P (four students are swimmers) $= P (X = 4) = {5C}_{4} (\frac{1}{5}) . {(\frac{4}{5})}^{4}$

Therefore, option (A) is correct.

Miscellaneous Exercise

Q1. A and B are two events such that P (A) ≠ 0. Find P (B/A) if:

(i)A is a subset of B

(ii) A ∩ B = Φ

A.1. $P (A) \neq 0$

$A$ is a subset of $B$

$\Rightarrow A \cap B = A$

$∴ P (A \cap B) = P (B \cap A) = P (A)$

$= P (B / A) = \frac{P (B \cap A)}{P (A)} = 1$

$A \cap B \neq 0$

$(A \cap B) = 0$

$\Rightarrow P (B / A) = \frac{P (B \cap A)}{P (A)} = 0$

Q2.A couple has two children.

(i)Find the probability that both children are males I it is known that at least one of the children is male.

(ii)Find the probability that both children are females if it is known that the elder child is a female.

A.2. i. Here, Sample space of given condition,

$S = {(B, B), (B, G), (G, B), (G, G)}$

Let, $E :$ denotes the event both children are male

$F :$ denotes the event at least one of the children is a male

$∴ E \cap F = {(B, B)}$

$\Rightarrow P (E \cap F) = \frac{1}{4}$

Here, $P (E) = \frac{1}{4}$ and $P (F) = \frac{3}{4}$

$\Rightarrow P (E / F) = \frac{P (E \cap F)}{P (F)} = \frac{\frac{1}{4}}{\frac{3}{4}} = \frac{1}{3}$

ii. Let, $A :$ event that both children are female

$B :$ event that elder child is female

$A = {G, G}$ $P (A) = \frac{1}{4}$

$B = {(G, G), (G, B)}$ $P (B) = \frac{2}{4} = \frac{1}{2}$

$A \cap B = {(G, G)}$ $P (A \cap B) = \frac{1}{4}$

$P (A / B) = \frac{P (A \cap B)}{P (B)}$

$= \frac{\frac{1}{4}}{\frac{1}{2}} = \frac{1}{4} \times 2 = \frac{1}{2}$

Q3.Suppose that 5% of men and 0.25% of women have grey hair. A grey haired person is selected at random. What is the probability of this person being male? Assume that there are equal number of males and females.

A.3. Given, Men having grey hair $= 5 %$

Women having grey hair $= 0.25 %$

Total people with grey hair $= (5 + 0.25) %$ $= 5.25 %$

Probability that the selected person’s hair is of male $= \frac{5}{5.25} = \frac{1}{1.05} = \frac{100}{105} = \frac{20}{21}$

Q4. Suppose that 90% of people are right-handed. What is the probability that at most of 6 of a random sample of 10 people are right-handed?

A.4. It is given that $90 %$ of people are right handed.

$p = P$ (right handed) $= 90 % = \frac{90}{100} = \frac{9}{10}$ and $q = P$ (left handed) $= 1 - \frac{9}{10} = \frac{1}{10}$

Using Binomial distribution,

Probability more than $6$ people are right handed is given by

$= \sum_{r = 7}^{10} {10c}_{r} p^{r} q^{n - r}$

$= \sum_{r = 7}^{10} {10c}_{r} {(\frac{9}{10})}^{r} {(\frac{1}{10})}^{10 - r}$

Hence, probability of having at most $6$ right handed people:

$= 1 - P$ (more than $6$ people are right handed)

$= 1 - \sum_{r = 7}^{10} {10c}_{r} {(\frac{9}{10})}^{r} {(\frac{1}{10})}^{10 - r}$

Q5. An urn contains 25 balls of which 10 balls bear a mark ‘X’ and the remaining 15 bear a mark ‘Y’. A ball is drawn at random from the urn, its mark noted down and it is replaced. If 6 balls are drawn in this way, find the probability that:

(i) all will bear ‘X’ mark.

(ii)not more than 2 will bear ‘Y’ mark.

(iii)at least one ball will bear ‘Y’ mark.

(iv)the number of balls with ‘X’ mark and ‘Y’ mark will be equal.

A.5. Total number of balls in the urn = 25

Balls bearing mark ‘X’ = 10

Balls bearing mark ‘Y’ = 15

p = P (ball bearing mark ‘X’) =10/25 = 2/5

q = P (ball bearing mark ‘Y’) =15/25 = 3/5

Six balls are drawn with replacement. Therefore, the number of trials are Bernoulli trials.

Let Z be the random variable that represents the number of balls with ‘Y’ mark on them in the trials.

Clearly, Z has a binomial distribution with n = 6 and p =2/5.

$∴ P = (Z = z) = {nC}_{z} p^{n - z} q^{z}$

P (all will bear ‘X’ mark) $= P (Z = 0) = {6C}_{0} {(\frac{2}{5})}^{6} = {(\frac{2}{5})}^{6}$

P (not more than 2 bear ‘Y’ mark) $= P (Z \leq 2)$

$\begin{array}{l} = P (Z = 0) + P (Z = 1) + P (Z = 2) \\ = {6C}_{0} {(p)}^{6} {(q)}^{0} +6 {C_{1}} {(p)}^{5} {(q)}^{1} + {6C}_{2} {(p)}^{4} {(q)}^{2} \\ = {(\frac{2}{5})}^{6} + 6 {(\frac{2}{5})}^{5} (\frac{3}{5}) + 15 {(\frac{2}{5})}^{4} {(\frac{3}{5})}^{2} \\ = {(\frac{2}{5})}^{4} [{(\frac{2}{5})}^{2} + 6 (\frac{2}{5}) (\frac{3}{5}) + 15 {(\frac{3}{5})}^{2}] \\ = {(\frac{2}{5})}^{4} [\frac{4}{25} + \frac{36}{25} + \frac{135}{25}] \\ = {(\frac{2}{5})}^{4} [\frac{175}{25}] \\ = 7 {(\frac{2}{5})}^{4} \end{array}$

P (at least one ball bears ‘Y’ mark) $= P (Z \geq 1) = 1 - P (Z = 0)$

$= 1 - {(\frac{2}{5})}^{6}$

P (equal number of balls with ‘X’ mark and ‘Y’ mark) $= P (Z = 3)$

$\begin{array}{l} = {6C}_{3} {(\frac{2}{5})}^{3} {(\frac{3}{5})}^{3} \\ = \frac{20 \times 8 \times 27}{15625} \\ = \frac{864}{3125} \end{array}$

Q6. In a hurdle race, a player has to cross 10 hurdles. The probability that he will clear each hurdle is 5/6 What is the probability that he will knock down fewer than 2 hurdles?

A.6. Let p and q respectively be the probabilities that the player will clear and knock down the hurdle.

$\begin{array}{l} ∴ P = \frac{5}{6} \\ \Rightarrow q = 1 - p = 1 - \frac{5}{6} = \frac{1}{6} \end{array}$

Let X be the random variable that represents the number of times the player will knock down the hurdle.

Therefore, by binomial distribution, we obtain

$P (X = x) = {nC}_{x} p^{n - x} q^{x}$

P (player knocking down less than 2 hurdles) $= P (X < 2)$

$\begin{array}{l} = P (X = 0) + P (X = 1) \\ = {10C}_{0} {(q)}^{0} {(p)}^{10} + {10C}_{1} (q) {(p)}^{9} \\ = {(\frac{5}{6})}^{10} + 10 . \frac{1}{6} . {(\frac{5}{6})}^{9} \\ = {(\frac{5}{6})}^{9} [\frac{5}{6} + \frac{10}{6}] \\ = \frac{5}{2} {(\frac{5}{6})}^{9} \\ = \frac{{(5)}^{10}}{2 \times {(6)}^{9}} \end{array}$

Q7. A die is thrown again and again until three sixes are obtained. Find the probability of obtaining the third six in the sixth throw of the die.

A.7. The probability of getting a six in a throw of die is 1/6 and not getting a six is 5/6.

Let p = 1/6 and q = 5/6

The probability that the 2 sixes come in the first five throws of the die is

${5C}_{2} {(\frac{1}{6})}^{2} {(\frac{5}{6})}^{3} = \frac{10 \times {(5)}^{3}}{{(6)}^{5}}$

∴ Probability that third six comes in the sixth throw =

$\begin{array}{l} = \frac{10 \times {(5)}^{3}}{{(6)}^{5}} \times \frac{1}{6} \\ = \frac{10 \times 125}{{(6)}^{6}} \\ = \frac{10 \times 125}{46656} \\ = \frac{625}{23328} \end{array}$

Q8. If a leap year is selected at random, what is the change that it will contain 52 Tuesday?

A.8. A leap year has 366 days which means 52 complete weeks and 2 days. If any one of these two days in a Tuesday, then the year will have 53 Tuesdays.

Number of total days in a week = 7

Number of favourable days = 2

Therefore, P (the year will have 53 Tuesday) = 2/7

Q9. An experiment succeeds twice as often as it fails. Find the probability that in the next six trails, there will be at least 4 successes.

A.9. The probability of success is twice the probability of failure.

Let the probability of failure be x.

∴ Probability of success = 2x

$\begin{array}{l} x + 2 x = 1 \\ \Rightarrow 3 x = 1 \\ \Rightarrow x = \frac{1}{3} \\ ∴ 2 x = \frac{2}{3} \end{array}$

Let $p = \frac{1}{3}$ and $q = \frac{2}{3}$

Let X be the random variable that represents the number of successes in six trials.

By binomial distribution, we obtain

$P (X = x) = {nC}_{x} p^{n - x} q^{x}$

Probability of at least 4 successes $= P (X \geq 4)$

$\begin{array}{l} = P (X = 4) + P (X = 5) + P (X = 6) \\ = {6C}_{4} {(\frac{2}{3})}^{4} {(\frac{1}{3})}^{2} + {6C}_{5} {(\frac{2}{3})}^{5} (\frac{1}{3}) + {6C}_{6} {(\frac{2}{3})}^{6} \\ = \frac{15 {(2)}^{4}}{3^{6}} + \frac{6 {(2)}^{5}}{3^{6}} + \frac{{(2)}^{6}}{3^{6}} \\ = \frac{{(2)}^{4}}{{(3)}^{6}} [15 + 12 + 4] \\ = \frac{31 \times 2^{4}}{{(3)}^{6}} \\ = \frac{31}{9} {(\frac{2}{3})}^{4} \end{array}$

Q10. How many times must a man toss a fair coin so that the probability of having at least one head is more than 90%?

A.10. Let the man toss the coin n times. The n tosses are n Bernoulli trials.

Probability (p) of getting a head at the toss of a coin is 1/2.

∴ p = 1/2 ⇒ q = 1/2

$∴ P (X = x) = {nC}_{x} p^{n - x} q^{x} = {nC}_{x} {(\frac{1}{2})}^{n - x} {(\frac{1}{2})}^{x} = {nC}_{x} {(\frac{1}{2})}^{n}$

It is given that,

P (getting at least one head) > 90/100

P (x ≥ 1) > 0.9

⇒ 1 − P (x = 0) > 0.9

$\begin{array}{l} 1 - {nC}_{0} . \frac{1}{2^{n}} > 0.9 \\ {nC}_{0} . \frac{1}{2^{n}} < 0.1 \\ \frac{1}{2^{n}} < 0.1 \\ 2^{n} > \frac{1}{0.1} \\ 2^{n} > 10 . . . . . . (1) \end{array}$

The minimum value of n that satisfies the given inequality is 4.

Thus, the man should toss the coin 4 or more than 4 times.

Q11. In a game a man wins a rupee for a six and looses a rupee for any other number when a fair die is thrown. The man decided to throw a die thrice but to quit as and when he gets a six. Find the expected value of the amounts he wins/looses.

A.11. When a die is thrown, then probability of getting a six = 16

then, probability of not getting a six = 1 - 16 = 56

If the man gets a six in the first throw, then

probability of getting a six = 16

If he does not get a six in first throw, but gets a six in second throw, then

probability of getting a six in the second throw = 56×16 = 536

If he does not get a six in the first two throws, but gets in the third throw, then

probability of getting a six in the third throw = 56×56×16 = 25216

probability that he does not get a six in any of the three throws = 56×56×56 = 125216

In the first throw he gets a six, then he will receive Re 1.

If he gets a six in the second throw, then he will receive Re (1 - 1) = 0

If he gets a six in the third throw, then he will receive Rs(-1 - 1 + 1) = Rs (-1),

that means he will lose Re 1 in this case.

Expected value = 16×1 + 56×16 × 0 + 56×56×16×-1 = 11216

So, he will loose Rs 11216.

Q12. Suppose we have four boxes A, B, C and D containing coloured marbles as given below:

Box

Marble colour

Red

White

Black

A

B

C

D

1

6

8

0

6

2

1

6

3

2

1

4

One of the boxes has been selected at random and a single marble is drawn from it. If the marble is red, what is the probability that it was drawn from box A?, box B? box C?

A.12. Let R be the event of drawing the red marble.

Let EA, EB, and EC respectively denote the events of selecting the box A, B, and C.

Total number of marbles = 40

Number of red marbles = 15

P(R) = 15/40 = 3/8

Probability of drawing the red marble from box A is given by P (EA|R).

$∴ P (E_{A} | R) = \frac{P (E_{A} \cap R)}{P (R)} = \frac{\frac{1}{40}}{\frac{3}{8}} = \frac{1}{15}$

Probability that the red marble is from box B is P (EB|R).

$\Rightarrow P (E_{B} | R) = \frac{P (E_{B} \cap R)}{P (R)} = \frac{\frac{6}{40}}{\frac{3}{8}} = \frac{2}{5}$

Probability that the red marble is from box C is P (EC|R).

$\Rightarrow P (E_{C} | R) = \frac{P (E_{C} \cap R)}{P (R)} = \frac{\frac{8}{40}}{\frac{3}{8}} = \frac{8}{15}$

Q13. Assume that the chances of a patient having a heart attack is 40%. It is also assumed that a meditation and yoga course reduces the risk of heart attack by 30% and prescription of certain drug reduces its chances by 25%. At a time a patient can choose any one of the two options with equal probabilities. It is given that after going through one of the two options the patient selected at random suffers a heart attack. Find the probability that the patient followed a course of meditation and yoga.

A.13. A patient has options to have the treatment of yoga and meditation and that of prescription of drugs.

Let these events be denoted by E1 and E2 i.e.,

E1 = Treatment of yoga and meditation

E2 = Treatment of prescription of certain drugs

P (E1) = P (E2) = 1/2

Let A denotes that a person has heart attack, then P (A) = 40% = 0.40

Yoga and meditation reduces heart attack by 30.

Inspite of getting yoga and meditation treatment heart risk is 70% of 0.40

P (A|E1) = 0.40 x 0.70 = 0.28

Also, Drug prescription reduces the heart attack rick by 25%

Even after adopting the drug prescription hear rick is 75% of 0.40

P (A|E2) = 0.40 x 0.75 = 0.30

$\begin{array}{l} P (E_{1} | A) = \frac{P (E_{1}) P (A | E_{1})}{P (E_{1}) P (A | E_{1}) + P (E_{2}) P (A | E_{2})} \\ = \frac{\frac{1}{2} \times 0.28}{\frac{1}{2} \times 0.28 + \frac{1}{2} \times 0.30} \\ = \frac{14}{29} \end{array}$

Q14.If each element of a second order determinant is either zero or one, what is the probability that the value of the determinant is positive? (Assume that the individual entries of the determinant are chosen independently, each value being assumed with probability 1/2).

A.14. There are four entries in a determinant of 2 x 2 order. Each entry may be filled up in two ways with 0 or 1.

Number of determinants that can be formed = (2)⁴ = 16

The value of determinants is positive in the following cases:

$| \begin{matrix} 1 & 0 \\ 0 & 1 \end{matrix} | | \begin{matrix} 1 & 1 \\ 0 & 1 \end{matrix} | | \begin{matrix} 1 & 0 \\ 1 & 1 \end{matrix} |$

Therefore, the probability that the determinant is positive = 3/16

Q15. An electronic assembly consists of two sub-systems say A and B. From previous testing procedures, the following probabilities are assumed to be known:

P (A fails) = 0.2

P (B fails alone) = 0.15

P (A and B fail) = 0.15

Evaluate the following probabilities.

A.15. Let the event in which A fails and B fails be denoted by E_A and E_B.

P (EA) = 0.2

P (E_A∩ E_B) = 0.15

P (B fails alone) = P (E_B) − P (E_A ∩ E_B)

⇒ 0.15 = P (E_B) − 0.15

⇒ P (E_B) = 0.3

$P (E_{A} | E_{B}) = \frac{P (E_{A} \cap E_{B})}{P (E_{B})} = \frac{0.15}{0.3} = 0.5$

(ii) P (A fails alone) = P (E_A) − P (E_A∩ E_B)

= 0.2 − 0.15

= 0.05

Q16. Bag I contains 3 Red and 4 Black balls and B II contains 4 Red and % Black balls. One ball is transferred from Bag I to bag II and then a ball is drawn from bag II. The ball so drawn is found to be Red in colour. Find the probability that the transferred ball is Black.

A.16. Let E₁ = Ball transferred from Bag I to Bag II is red

E₂ = Ball transferred from Bag I to Bag Ii is black

A = Ball drawn from Bag II is red in colour

P (E₁) =3/7 and P (E₂) = 4/7

Let A be the event that the ball drawn is red.

When a red ball is transferred from bag I to II,

P (A | E₁) = 5/10 = 1/2

When a black ball is transferred from bag I to II,

P (A | E₂) = 4/10 = 2/5

$\begin{array}{l} ∴ P (E_{2} | A) = \frac{P (E_{2}) P (A | E_{2})}{P (E_{1}) P (A | E_{1}) + P (E_{2}) P (A | E_{2})} \\ = \frac{\frac{4}{7} \times \frac{2}{5}}{\frac{3}{7} \times \frac{1}{2} + \frac{4}{7} \times \frac{2}{5}} \\ = \frac{16}{31} \end{array}$

Choose the correct answer in each of the following:

Q17. If A and B are two events such that P (A) ≠ 0 and P(B|A) = 1, then:

(A) A ⊂ B

(B) B ⊂ A

(C) B = Φ

(D) A = Φ

A.17. $P (A) \neq 0$ and $P (B | A) = 1$

$\begin{array}{l} P (B \cap A) = \frac{P (B \cap A)}{P (A)} \\ 1 = \frac{P (B \cap A)}{P (A)} \\ P (A) = P (B \cap A) \\ \Rightarrow A \subset B \end{array}$

Therefore, option (A) is correct.

Q18. If P (A|B) > P (A), then which of the following is correct:

(A) P (B|A) < P (B)

(B) P (A ∩ B) < P (A).P (B)

(C) P (B|A) > P (B)

(D) P (B|A) = P (B)

A.18.

$\begin{array}{l} P (A | B) > P (A) \\ \Rightarrow \frac{P (A \cap B)}{P (B)} > P (A) \\ \Rightarrow P (A \cap B) > P (A) . P (B) \\ \Rightarrow \frac{P (A \cap B)}{P (A)} > P (B) \\ \Rightarrow P (B | A) > P (B) \end{array}$

Therefore, option (C) is correct.

Q19.If A and B are any two events such that P (A) + P (B) – P (A and B) = P (A), then

(A) P (B|A) = 1

(B) P (A|B) = 1

(C) P (B|A) = 0

(D)P (A|B) = 0

A.19.

$\begin{array}{l} P (A) + P (B) - P (A a n d B) = P (A) \\ \Rightarrow P (A) + P (B) - P (A \cap B) = P (A) \\ \Rightarrow P (B) - P (A \cap B) = 0 \\ \Rightarrow P (A \cap B) = P (B) \\ ∴ P (A | B) = \frac{P (A \cap B)}{P (B)} = \frac{P (B)}{P (B)} = 1 \end{array}$

Therefore, option (B) is correct.

NCERT Solutions Class 12 Maths Chapter 13 Probability: Download Exercise Solutions PDF

Probability Class 12 Solutions Exercise 13.1 & Exercise 13.2

Probability Class 12 Solutions Exercise 13.3 & Exercise 13.4

Probability Class 12 Solutions Exercise 13.5 & Miscellaneous Exercise

News & Updates

JEE Main Admit Card 2025 OUT Live Updates: January 28, 29, 30 Hall Ticket Release date, link jeemain.nta.nic.in

JEE Main 2025 January 22 Live Updates: Shift 1 Starts, Question Paper, Answer Key, Paper Analysis

WBJEE 2025 Registration Live Updates: Link (TODAY), Check Last Date to Apply, Process, Eligibility

AIBE 19 Result 2024 (Soon) Live Updates: BAR Exam Result & Final Answer Key at allindiabarexamination.com

RRB ALP 2024 CBT 1 Result Soon @rrbapply.gov.in LIVE Updates

JEE Main 2025 January 22 Question Paper Answer Key Solution

JEE Main Marks vs Percentile vs Rank 2025: Calculate Percentile and Rank Using Marks

List of NIT Colleges in India 2025 - NIRF Ranking, Courses, Seats, Cutoff, Fees and Placement

NEET Chapter Wise Weightage 2025 PDF by NTA: Physics, Chemistry & Biology

CBSE 10th Maths Question Paper 2024, 2023, 2022, 2020, 2019 PDF Download

List of Top IITs in India 2025: NIRF Ranking, Courses, Seats, Fees & Placement

Student Forum