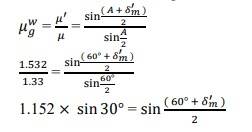What is Prism?
Prism is a transparent optical component with levelled surfaces that refract the light.
The horizontal faces join two polygonal bases. These horizontal faces are rectangular, but it can be a parallelogram in some cases depending upon the surface.
The formula of a Prism is as follows:
- The surface area of prism: (2 × Base Area) + Lateral Surface Area
- The volume of prism: base area x height
Types of prisms
There are five types of prisms which are as follows:
1. Dispersive prisms: Dispersive prisms break the light into different spectral colours. The example of dispersive prisms is amici prism grism and a triangular prism.
2. Reflective prisms: Reflective prisms rotate, invert, and displace the light beam from the source. Some of the examples of reflective prisms are pentaprism, dove prism, retroreflector, etc.
3. Polarising prisms: By the variation of polarisation, this type of prism is used to break or split the light beam like a Nicol prism.
4. Beam-splitting prisms: this type of prism is used to break the beams into more than two beams. Some of the examples include a beam splitter cube and the dichroic prism.
5. Deflecting prisms: At a fixed angle, a beam of light is deflected. This is done with the help of deflecting prisms. The example of deflecting prisms includes wedge prisms.
Derivation of a Prism formula
The prism formula was derived with the principle of Snell’s law. It can be written as:
μ= sini / sinr
This equation is called Snell's Law
Δ = i1 − r1 + i2 − r2… (1)
δ = i1 + i2 − (r1 + r2) ∠ALO + ∠AMO = 2rt ∠s
Here, ∠ALO = ∠AMO = 90°
∠LAM + ∠LOM = 2rt ∠s (sum of four ∠s of a quadrilateral = 4 rt ∠s) …... (2)
∠r1 + ∠r2 + ∠LOM = 2rt∠s …… (3)
By comparing equation 1 and 2, we get:
∠LAM = ∠r1 + ∠r2
The next step is to substitute the A in equation 1, by doing so we get:
A = ∠r1 + ∠r2
Δ = i1 + i2 − A
i1 + i2 = A + δ
∠i1 = ∠i2, ∠r1 = ∠r2 = ∠r
∠ALM = ∠LMA = 90∘ −∠r
Now, AL = LM and LM ∥ BC
∠A = ∠r1 + ∠r2
A = 2r (because ∠r1=∠r2=∠r)
R = A2, i1 + i2 = A + δ i1 + i1 = A + δ m2i1 = A + δ mi1 = A + δ m/2
∴ μ = sin A + δ m2 /sinA2 (formula of prism)
Derivation of a prism formula in class 11
The chapter optics holds a weightage of 7 marks in total. It consists of one long question (5 marks) and one short question (2 marks).
Illustrated Examples
Example 1) – Write the derived equation of the formula of Prism.
Answer – μ = sin A + δ m2 /sinA2 (formula of prism)
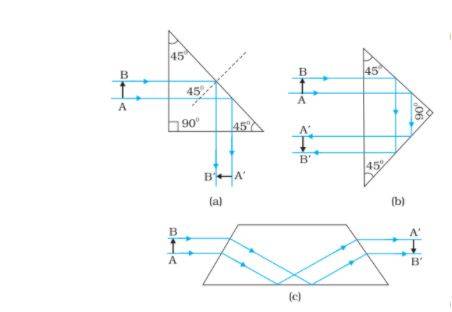
Example 2) – Illustrate bending of light by Prisms.
Answer –
Source-ncert
Example 3) – Illustrate refraction through a prism.
Answer –
FAQs on Derivation of Prism Formula
Q: A prism is made of glass of unknown refractive index. A parallel beam of light is incident on a face of the prism. The angle of minimum deviation is measured to be 40°. What is the refractive index of the material of the prism? The refracting angle of the prism is 60°. If the prism is placed in water (refractive index 1.33), predict the new angle of minimum deviation of a parallel beam of light
A:The angle of minimum deviation, ?? = 40°
Angle of prism, A = 60°
Let the refractive index of water, = 1.33 , and the refractive index of prism material = ?′
The angle of deviation is related to refractive index ?′ is given as
?′ =
sin( A + ??)
2
sin A/2
= Sin( 60 + 40)
2
sin 60/2
= sin 50° / sin 30°
= 1.532
So the refractive index of prism material is 1.532
Since the prism is placed in water, let ?′ m be the new angle of minimum deviation. The refractive index of glass with respect to water is given by the relation:
( 60° + ?′ m) / 2 = sin−1 0.576 = 35.2°
?′ m = 2 × 35.2° - 60° = 10.33°
Hence the new minimum angle of deviation is 10.33°
Q: At what angle should a ray of light be incident on the face of a prism of refracting angle 60° so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524.
A: The incident, refracted and emergent rays associated with a glass prism ABC is shown in the adjoined figure.
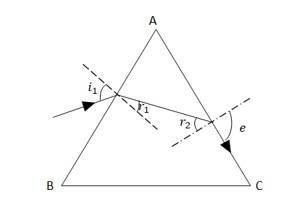
Angle of the prism, ∠? = 60°
Refractive index of the prism, ? = 1.524
Let ∠??1 be the incident angle, ∠?1 be the refracted angle, ∠?2 be the incidence angle on face
AC and ∠? be the emergent angle from the prism = 90 °
According to Snell’s law, for face AC, we can have:
sin ? / sin ?2= ?
sin ?2 = sin ? / ? = sin 90° / 1.524= 0.656
∠?2 = 41°
From the Δ ABC, ∠? = ∠?1 + ∠?2 . Hence ∠?1 = 60° − 41° = 19°
According to Snell’s law, for face AB, we have
? = ???? ??1 / sin ?1 or sin ?? 1 = ? × sin ? = 1.524 × sin 19° = 0.496
∠??1 = 29.75 °
Hence the angle of incidence is 29.75 °
Q: How will you calculate the surface area of a prism?
A: The surface area can be calculated by using this formula - (2 × Base Area) + Lateral Surface Area
Q: How will you calculate the volume of a prism?
A: Volume can be calculated by using this formula - Base area x height
Q: What do you understand by Snell’s law?
A: Prism formula was derived with the principle of Snell’s law. It can be written as: μ= sini / sinr - Snell’s Law
Q: What are Polarizing prisms?
A: By the variation of polarisation, this prism is used to break or split the light beam like Nicol prism.
Q: What are reflecting prisms?
A: Reflective prisms rotate, invert, and displace the light beam from the source.
News & Updates
Physics Optics Exam
Student Forum
Popular Courses After 12th
Exams: BHU UET | KUK Entrance Exam | JMI Entrance Exam
Bachelor of Design in Animation (BDes)
Exams: UCEED | NIFT Entrance Exam | NID Entrance Exam
BA LLB (Bachelor of Arts + Bachelor of Laws)
Exams: CLAT | AILET | LSAT India
Bachelor of Journalism & Mass Communication (BJMC)
Exams: LUACMAT | SRMHCAT | GD Goenka Test