- What is a Vector Addition?
- Weightage of Vector Addition
- Illustrative Example of Vector Addition
- FAQs on Vector Addition
What is a Vector Addition?
Any entity that has magnitude and position is a vector. Examples of vector quantities are displacement, velocity, acceleration, force, strain, etc. Mathematical operations can be done between two or more vectors.
Vector Addition
The method of adding two or more vectors is called vector addition. Depending on the position of the vector, the addition of the vector is divided into two groups.
They're –
Law of the parallelogram of vector addition
Triangular law of addition of vector
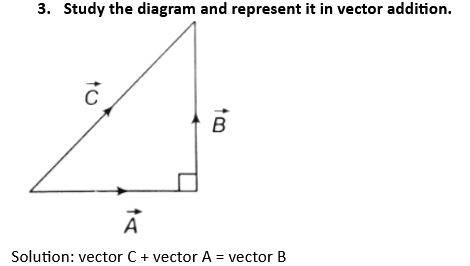
Consider vector A and vector B
The form of vector inclusion is selected based on the configuration of the vector head and tail.
If two vectors are arranged head to tail, the triangular rule of vector addition shall be followed.
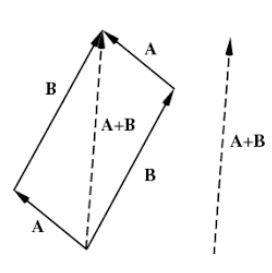
Law of the Parallelogram of Vector Addition
If two vectors are arranged head to head or tail to tail so parallelogram rule of vector addition shall be applied.
Statement: If we consider two vectors as the adjacent sides of a parallelogram, the result is a diagonal vector cutting through two vectors' contact point.
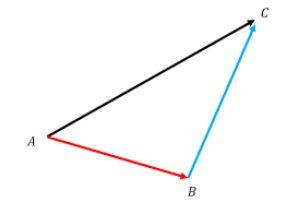
Triangular Law of Addition of Vectors
If two vectors are arranged head to tail, the triangular rule of vector addition shall be applied.
Statement: When two sides of a triangle represent two vectors in direction and magnitude in the same order, then the third side of that triangle represents the resultant vector in direction and magnitude.
Why is Vector Addition Important?
In mathematics, the quantity of vectors like force combines and creates an effect on which they are applied. As the effect of all these forces is taken into account when deciding the purpose of the mechanism's motion, certain processes, such as addition, subtraction, and multiplication, are essential to determine the outcome of these forces.
Weightage of Vector Addition
This concept is taught in chapter Vector Algebra. You will learn about the basics of vectors, their addition, and subtraction. The weightage of this chapter is 14 marks.
Illustrative Example of Vector Addition
1. Two forces of 3 N and 4 N operate when the angle between them is 60 degrees. Find the resultant force that arises from this.
Solution.
Resultant force, R = √(42 + 32 + 2 x 3 x 4 Cos 60°)
= √(9 + 16 + 12)
= √(37 = 6.08 N
The direction of R =
tan q = (3 Sin 60°)/(4 + 3 Cos 60°) = 0.472
q = tan-1 0.472
= 25.3°
2. Find the x and y components with a displacement of 25 m at an angle of 210 deg.
The horizontal axis angle is 210 deg-180 deg = 30 deg.
Component x = OB =-25 Cos 30°= -21.7
Component Y = AB =-25 Sin 30° = -12.5 m
Remember that each component points along the negative coordinate path, so we have to take it as negative.
FAQs on Vector Addition
Q: When are we going to use the parallelogram rule of vector addition?
Q: State parallelogram law of addition of vectors.
Q: State triangular law of the addition of vectors.
Q: What is the resulting vector in the parallelogram law of vector addition?
Maths Vector Algebra Exam
Student Forum
Popular Courses After 12th
Exams accepted
CA FoundationExams accepted
ICSI ExamExams accepted
BHU UET | GLAET | GD Goenka TestBachelor of Business Administration & Bachelor of Law
Exams accepted
CLAT | LSAT India | AIBEExams accepted
IPMAT | NMIMS - NPAT | SET
Exams accepted
BHU UET | KUK Entrance Exam | JMI Entrance ExamBachelor of Design in Animation (BDes)
Exams accepted
UCEED | NIFT Entrance Exam | NID Entrance ExamBA LLB (Bachelor of Arts + Bachelor of Laws)
Exams accepted
CLAT | AILET | LSAT IndiaBachelor of Journalism & Mass Communication (BJMC)
Exams accepted
LUACMAT | SRMHCAT | GD Goenka Test