Mach Number: Overview, Questions, Preparation

Q: Q: What are the uses of Mach numbers?

A: For compressibility assumptions. It acts as a tool to assess the noise generated by aircraft and the equipment. Mach number applies to the objects travelling with high speed and high-speed fluid flow inside tunnels or nozzles.

This chapter has multiple topics listed below:

Laws of Physics Law of Conservation of Mass Biot Savart Law Ideal Gas Law Radioactive Decay Law Continuity Equation Charge Definition Mach Number Chandrasekhar Limit Poissons Ratio Stefan Boltzmann Law Stokes Law Derivation Vectors Orbit and Orbital Sound Definition Quantum Number Definition Thermonuclear Reaction Time Period of Simple Pendulum ...

Rajdeep Das

Updated on Aug 19, 2021 01:00 IST

Table of Contents

Introduction
Mach Number
Classification of Mach Regimes
Mach Number for Class 11
Illustrative Examples
FAQs

Introduction

Compressible flows are the variable density flows while the incompressible flows deal with constant density. When the variation in the flow of density is negligible, the flow is incompressible. The study of the motion of compressible flows is discussed under Gas Dynamics. So, Mach number is a measure of compressibility characteristics of a fluid flow.

Mach Number

In fluid dynamics, the Mach number (M or Ma) is a dimensionless quantity representing the ratio of velocity past a boundary to the local sound. The name comes after the Austrian physicist and philosopher Ernst Mach.

M = μ/c

M = Local Mach number

μ = Local flow velocity

C = speed of sound in the medium

It shows the compressibility effect for a fluid, i.e., M < 0.3 implies that fluid is incompressible.

Classification of Mach Regimes

Different Mach Regimes	Behaviour
Subsonic Flow	When object speed is less than the velocity of sound(M<1) Subsonic flows have smooth streamlines. Free stream Mach number M≤ 0.8
Transonic Flow	It is a mixed flow lying between both subsonic regions M < 1 and supersonic M > 1. Shock waves form on the upper and lower surface. As speed increases and when Mach number =1, both surfaces meet each other.
Supersonic Flow	A flow is supersonic if the Mach number is >1 at every point. Here, oblique and expansion shock waves occur.
Hypersonic Flow	Here the Free stream Mach number is increased to higher supersonic speed(M>>1). As the number increases, the shock wave moves closer to the body surface.

Mach Number for Class 11

Mach number is the property of the fluid, which is a dimensionless quantity to study the flow of motion. It is classified into different regimes based on Mach number value. The topic’s weightage is around 5-10% of long answer type of question with the problem included.

Illustrative Examples

An Aeroplane travelling at a speed of 1800 km/hr in the air of pressure of 1 bar at 10-degree celsius. Find the Mach number. ( k=1.4 and R=287 J/Kg K).

Temperature t = 10˚ C

T = 10 + 273 = 283 k

Speed V = 1800 km/hr = 500m/s

Velocity, C=KRT =√1.4×287×283

C = 337.20 m/s.

Mach number = V/C =500/337.20 = 1.48

The Mach number for a body moving through the fluid is 0.5. What is the velocity of the fluid, if the speed of the sound is 345 m/s?

Mach number = V/C =0.5*345

velocity =172.5 m/s

Determine the velocity of a bullet if the Mach angle is 30˚. Given the temperature of the air is 22˚ C. ( k=1.4 and R=287 J/Kg K).

C=KRT =√1.4×287×295 = 344.52 m/s

Mach angle =sinα =1/m

sin 30˚ = ½ =0.5

M =2

V = m*c= 2*344.5 =689.04m/s

FAQs

Q: What is compressibility?

A: Compressibility is the fractional change in the density of the fluid element per unit change in pressure. The gas compression involves an increase in temperature depending on the amount of heat added or taken away from gas.

Q: How are shock waves created?

A: When the object travels faster than the speed of sound, the wavefronts form a cone-shaped region at the vertex of high-pressure is a shock wave.

Mach reflection of shock waves is employed for removing micron-size dust particles from the surface of silicon wafers.

Q: What are the other dimensionless quantities related to fluid mechanics?

A: Reynolds number, Brownwell Katz number, capillary number, excess temperature coefficient, Laplace number, drag coefficient are some of the other dimensionless quantities.

Q: What are the uses of Mach numbers?

For compressibility assumptions.
It acts as a tool to assess the noise generated by aircraft and the equipment.
Mach number applies to the objects travelling with high speed and high-speed fluid flow inside tunnels or nozzles.

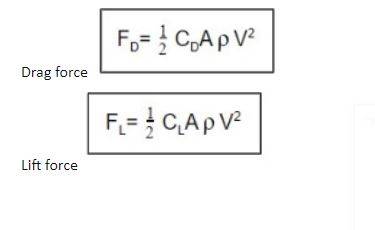
Q: What are the aerodynamic forces?

A: The aerodynamic force depends on fluid density. There are two types,