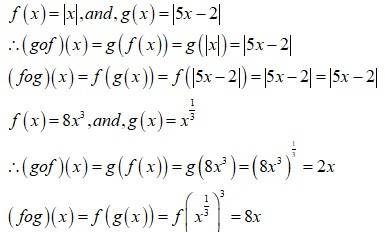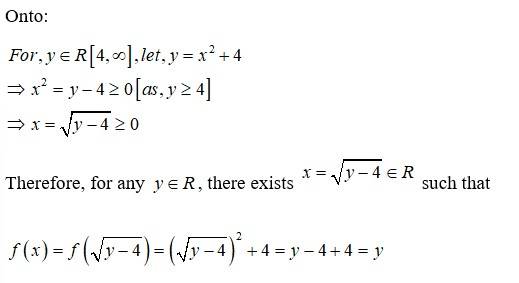Class 12 Chapter 1 Relation and Function NCERT Solutions: Shiksha provides a complete solution of NCERT Class 12 Maths Chapter 1 Relation and Function on this page. NCERT Maths Chapter 1 Relation and Function is one of the most important and complex topics in Class 12 Mathematics. Shiksha brings detailed Relation and Function NCERT Solutions in lucid language with strong conceptual fundamentals for students.
Students can also download the NCERT class 12 Maths chapter 1 solutions pdf below on this page. Relation and function Class 12 Solutions PDF will be very beneficial for board students as well as competitive exams such as JEE Main, BITSAT, etc... aspirants. Students must check NCERT Class 12 Maths Chapter 1 solutions to get a strong conceptual understanding and get better scores in board exams. Students must check the Class 11 Chapter 2 Relation and Function solutions to get better conceptual clarity of the concepts.
The Relation and Function chapter includes many important topics such as Types of relations, ordered pairs, Types of functions, range, domain and others. A relation R from a set A to a set B is a subset of the Cartesian Product A × B obtained by describing a relationship between the first element x and the second element y of the ordered pairs in A × B. A function can be defined as a relation f from set A to set B where every element of set A has only one image in set B. Students can check exercise-wise Class 12 Maths NCERT solutions of all chapters on Shiksha. Check below for more information;
| Related Important Math Chapter Solutions | ||
|---|---|---|
| Class 11 Chapter 1 Set Solutions | Class 11 Chapter 2 Relation and Function Solutions | Class 12 Chapter 2 Inverse Trigonometric Function Solution |
Commonly asked questions
Q: Is Class 12 Math Relation and Function chapter important for Board Exams?
Yes, Class 12 Math Relation and Function chapter is important for rither CBSE Board Exams or State Boards. The Relation and Function chapter have weightage of 6-8 marks in the CBSE board exam. Moreover Relation and function chapter forms the foundation for many other advanced topicssuch as types of relations, functions, domain, range, composition of functions, and invertibility. Relation and function is also important to understand complex JEE Mains topics such as calculus and inverse trigonometry. For more information check below;
Q: What are the key concepts discussed in the Relation and Function Class 12 Math?
Candidates must be aware of the important concepts discussed in the chapter and their application in other mathematics chapters. Students can check several fundamental and advanced topics discussed in the Relation and Function chapter.
- Basic Concepts: Definations, Sets cross product, Relation, Function, Domain, Range
- Types of Relations: Reflexive, Symmetric, Transitive, and Equivalence relations
- Types of functions: one-one, many-one, onto and into functions
- Other Important Functions: Modulus function, identity function, inverse function, and invertibility
- Operations on Functions: Commutativity, Associativity, Existence of identity, and inverse of Function
Students must check complete NCERT solution of Relation and Function by clicking on the below given link;
Q: What is weightage of Relation and Function in JEE Main?
Class 12 Math Relation and Function chapter have moderate weightage in the JEE Mains exam. Relation and function combined with sets comprises of 4-8 marks weight in the JEE Mains Exam. However, Students should know there is no fixed weightge of relation and fucntions chapter. Questions may be asked directly from relation and function or its applications can be used in forming the question. This makes Rleation and function a very important chapter to understand the basics of ITF and calculus also. JEE Aspirants can check the Class 12 Math Relation and Function Solution to undersatnd the basics of the chapter before starting their preparation. Check the lionk below;
Q: Will Class 12 Math Relation and Function NCERT Solutions be enough to prepare for the CBSE Boards exams?
Yes, Class 12 Math Relations and Functions includes all the key topics as per requirements for CBSE Board Exams. Relations and Functions has several exercises with plenty of practice problems to help students understand the concepts better. We have designed solutions in a simple and clear manner to build confidence and prepare students effectively for their CBSE exams
However, Students shouls also focus on understanding types of relations and functions, their graphs, and real-life applications with the help of other questions outside NCERT if possible. Practicing NCERT problems and previous years' questions asked in CBSE boards will make concepts easier and that will improve accuracy in exams. Check the NCERT Solutions below;
- Class 12 Math Chapter 1 Relation and Function: Key Topics, Weightage & Important Formulae
- Class 12 Math Chapter 1 Relation and Function Solution PDF
- Class 12 Math Chapter 1 Relation and Function Exercise -wise solutions
- Class 12 Math Chapter 1 Relation and Function Exercise 1.1 Solutions
- Class 12 Math Chapter 1 Relation and Function Exercise 1.2 Solutions
- Class 12 Math Chapter 1 Relation and Function Old NCERT Solutions
- Class 12 Math important reference books and preparation Tips
Class 12 Math Chapter 1 Relation and Function: Key Topics, Weightage & Important Formulae
The Relation and Function chapter carries up to 8 marks weightage in the Board exams. This chapter is also important to understand complex JEE Mains topics such as calculus and inverse trigonometry. Several key topics are discussed in the exercises;
- Types of relations: Reflexive, Symmetric, Transitive, and Equivalence relations
- Types of functions: one-one, many-one, onto and into functions
- Other Important Functions: Modulus function, identity function, inverse function, and invertibility
- Binary Operations: Commutativity, Associativity, Existence of identity, and inverse of Function
Read more: Most scoring topics in Mathematics for JEE Main
Check out Dropped topics of Relation and Function- 1.4 Composition of Functions and Invertible Function, Page 13-14 Full Pages, Page 15 Examples 24 and 25, Page 16-25 Full Pages, Page 26 Question 12 and 13, Page 27-28 Examples 45 and 49, Page 29-31 Ques. 1–3, 6–7, 9, 11–14, 18–19, Page 31-32 Summary points 11-13 and 15-19.
Relation and Function Important Formulae for CBSE and Competitive Exams
Relations
-
Domain of a Relation: The set of all first elements (x) in the ordered pairs of a relation :
-
Range of a Relation: The set of all second elements (y) in the ordered pairs of a relation :
-
The inverse of a Relation: If is a relation from set to set , then the inverse is a relation from set to set :
-
Types of Relations
- Reflexive Relation: is reflexive if
- Symmetric Relation: is symmetric if
- Transitive Relation: is transitive if
- Equivalence Relation: is an equivalence relation if it is reflexive, symmetric, and transitive.
- Reflexive Relation: is reflexive if
Functions
-
Function Definition: A function maps every element of to exactly one element of :
-
Domain and Range of a Function
- Domain: Set of all permissible inputs
of
- Range: Set of all possible outputs ().
- Domain: Set of all permissible inputs
of
-
Types of Functions
- One-One (Injective):
- Onto (Surjective): Every
has a pre-image
- Bijective: is both one-one and onto.
- One-One (Injective):
-
Composition of Functions: If and
-
Inverse of a Function: A function is invertible if it is bijective:
-
Binary Operation: A binary operation on
-
Modulo Function
-
Greatest Integer Function
Class 12 Math Chapter 1 Relation and Function Solution PDF
Students can find the solutions of all the exercises of relation and function in one place. This Relation and Function NCERT solution PDF will be really beneficial for all categories of students whether boards or competitive exams. access the PDF below;
Class 12 Math Chapter 1 Relation and Function Solution: Free PDF Download
Class 12 Math Chapter 1 Relation and Function Exercise -wise solutions
The class 12 Relation and Function chapter deals with many important topics, such as the type of relation, symmetric and asymmetric relations, functions, bijective functions, and surjective functions. Students will get problems related to different concepts and properties of relations and functions in the exercises. Candidates can check the exercise-wise chapter 1 math solutions below;
Class 12 Math Chapter 1 Relation and Function Exercise 1.1 Solutions
Chapter 1 Relation and Function Exercise 1.1 focuses on problems based on identifying reflexive, symmetric, and transitive relations & checking whether the relation is an equivalence relation or not. Class 12 Math Relation and Function Exercise 1.1 consists of 16 Questions (14 Short Answers, 2 MCQ). Students can check the complete the exercise 1.1 solutions below;
Relation and Function exercise 1.1 solutions
Q1. Determine whether each of the following relations are reflexive, symmetric and transitive:
(i)Relation R in the set A = {1, 2, 3…13, 14} defined as
R = {(x, y): 3x − y = 0}
(ii) Relation R in the set N of natural numbers defined as
R = {(x, y): y = x + 5 and x < 4}
(iii) Relation R in the set A = {1, 2, 3, 4, 5, 6} as
R = {(x, y): y is divisible by x}
(iv) Relation R in the set Z of all integers defined as
R = {(x, y): x − y is as integer}
(v) Relation R in the set A of human beings in a town at a particular time given by
(a) R = {(x, y): x and y work at the same place}
(b) R = {(x, y): x and y live in the same locality}
(c) R = {(x, y): x is exactly 7 cm taller than y}
(d) R = {(x, y): x is wife of y}
(e) R = {(x, y): x is father of y}
A.1. (i) We have, a relation in set A=
For or i.e.,
does not exist in R
R is not reflexive.
For
Then
So
R is not symmetric
For and . We have
and
Then
i.e.,
R is not Transitive
(ii) We have,
R= is a relation in N
=
=
Clearly, R is not reflexive as and
Also, R is not symmetric as but
And for . Hence, R is not Transitive.
(iii) R= is divisible by x is a relation in set
A=
So, R=
Hence, R is reflexive because i.e.,
R is not symmetric as but
And for and
and where
Then,
Hence,
R is transitive
(iv) R= is an integer is a relation in set Z
For
is an integer
So, i.e., R is reflexive
For and
is an integer
is an integer
is an integer
So, i.e., R is symmetric
For and We have,
is an integer
is an integer
So, is also an integer
is an integer
So, i.e., R is transitive.
(v) (a) R= and work at same place in set A of human being.
For we get
work at same place
So, R is reflexive.
For and We get,
work at same place
work at same place
. So, R is symmetric.
For and and . We have,
work at same place
work at same place
work at same place
i.e., . So, R is Transitive.
(b) R= live in same locality
For , we get,
live in same locality
So, R is reflexive.
For and we get,
live in same locality
live in same locality
i.e., . So, R is symmetric.
For and & . Then
live in same locality
live in same locality
live in same locality
So, i.e., R is transitive.
(c) R= is exactly 7 cm taller than y
For ,
Height height of
So, . i.e., R is not reflexive.
For, and we have,
Height
But
So, i.e., R is not symmetric.
For and and we have,
Height
And
So,
i.e.,
So, R is not transitive.
(d) R= is wife of y
For
X is not wife of x.
So, i.e., R is not reflexive.
For and ,
X is wife of y but y is not wife of x
So, . i.e., R is not symmetric.
For and and
X is wife of y
Y is wife of z
But y can never be husband & wife simultaneously
So, R is not transitive.
(e) R= is father of y
For ,
X is not a father of x
So, i.e., R is not reflexive.
For and
X is father of y
Y is father of z
But x is not father of z
So, i.e., R is not transitive.
Q2. Show that the relation R in the set R of real numbers, defined as
R = {(a, b): a ≤ b2} is neither reflexive nor symmetric nor transitive.
A.2. We have,
R= is a relation in R.
For then is is not true for all real number less than 1.
Hence, R is not reflexive.
Let and a=1 and b=2
Then, = = so,
But
i.e., = is not true
so,
hence, R is not symmetric.
For,
We have, => is true
So,
And => So,
But => is not true.
So,
Hence, R is not transitive.
Q3. Check whether the relation R defined in the set {1, 2, 3, 4, 5, 6} as
R = {(a, b): b = a + 1} is reflexive, symmetric or transitive.
A.3. We have,
R= is a relation in set
So, R=
As, , R is not reflexive
As, but , R is not symmetric
And as & but
Hence, R is not transitive.
Q4. Show that the relation R in R defined as R = {(a, b): a ≤ b}, is reflexive and transitive but not symmetric.
A.4. We have, R= is a relation in R.
For, ,
but is not possible i.e.,
Hence, R is not symmetric.
For and
and
So,
i.e.,
R is transitive.
Q5. Check whether the relation R in R defined as R = {(a, b): a ≤ b3} is reflexive, symmetric or transitive.
A.5. We have,
R= is a relation in R.
For, and we can write
=> => which is not true.
So, R is not reflexive.
For we have,
=> => is true.
So,
But => is not true
So, and
Hence, R is not symmetric.
For, and
=> is true=>
=> is true=>
But => is not true=>
Hence, for
So, R is not transitive.
Q6. Show that the relation R in the set {1, 2, 3} given by R = {(1, 2), (2, 1)} is symmetric but neither reflexive nor transitive.
A.6. We have,
R= is a relation in set
Then, as and
So, R is not reflective
As and
So, R is symmetric
And as but
So, R is not transitive.
Q7. Show that the relation R in the set A of all the books in a library of a college, given by R = {(x, y): x and y have same number of pages} is an equivalence relation.
A.7. We have,
R= have same number of pages is a relation in set of A of all books in
For
As x=y=same no. of pages
Then,
Hence, R is reflexive.
For and
Also, ,
Hence, R is symmetric.
For and and
x=y and y=z
x=z
i.e.,
hence, R is also transitive
R is an equivalence relation.
Q8. Show that the relation R in the set A = {1, 2, 3, 4, 5} given by R = {(a,b) : |a-b| is even} is an equivalence relation. Show that all the elements of {1, 3, 5} are related to each other and all the elements of {2, 4} are related to each other. But no element of {1, 3, 5} is related to any element of {2, 4}.
A.8. We have,
R= is even is a relation in set A=
For all , is even.
So, . Hence R is reflexive
For and
is even
is even
is even
is even
i.e.,
Hence, R is symmetric.
For and and
We have is even
and is even
then, is even as even + even=even
is even
is even
So, R is transitive.
R is an equivalence relation
All elements of [1,3,5] are odd positive numbers and its subset are odd and their difference given an even number. Hence, they are related to each other.
Similarly, all elements of [2,4] are even positive numbers and its subset are even and their difference gives an even number. Hence, they are related to each other.
However, elements of [1,3,5] are related to elements of [2,4] since the difference of the two subsets is never even.
Q9. Show that each of the relation R in the set A = {x ∈ Z: 0 ≤ x ≤ 12} ,given by:
(i) R = { (a,b) : |a - b| is a multiple of 4}
(ii) R = { (a,b) : a= b} is an equivalence relation. Find the set of all elements related to 1 in each case.
A.9. We have,
A=
The relation in set A is defined by
R= { is a multiple of 4}
For all ,
is a multiple of 4
So, i.e., R is reflexive
For we have,
is multiple of 4
is multiple of 4
is multiple of 4
So,
i.e., R is symmetric
for
& is a multiple of 4
So is also a multiple of 4
is a multiple of 4
is a multiple of 4
So,
i.e., R is transitive
Hence, R is an equivalence relation.
Finding all set of elements related to 1
For
Then, i.e., is a multiple of 4
So, a can be 0 ≤ a ≤ 12
Only,
is a multiple of 4
Hence, sets of elements related to A=
The relation in set A is defined as R=
For all ,
a=a is true
so,
i.e., R is reflexive
for we have,
a=b
b=a i.e.,
so, R is symmetric
for , and . We have,
a=b and b=c
a=c i.e.,
R is transitive
Hence, R is an equivalence relation
Find all sets of elements related to 1
For , we need
i.e., a=1
so, set of elements related to 1 in A is {1}.
Q10. Given an example of a relation. Which is
(i) Symmetric but neither reflexive nor transitive.
(ii) Transitive but neither reflexive nor symmetric.
(iii) Reflexive and symmetric but not transitive.
(iv) Reflexive and transitive but not symmetric.
(v) Symmetric and transitive but not reflexive.
A.10. Let A=
(i) R= is a relation in set A
So, and Symmetric
not reflexive
but not transitive
(ii) R= is a relation in set A
So, not reflexive
but not symmetric
and also transitive
(iii) R=
So, Reflexive
Symmetric
and
But not transitive
(iv) R= is s relation in set A
So, reflexive
so, transitive
but not symmetric
(v) R=
So, not reflexive
and symmetric
And
and also transitive
Q11. Show that the relation R in the set A of points in a plane given by R = {(P, Q): distance of the point P from the origin is same as the distance of the point Q from the origin}, is an equivalence relation. Further, show that the set of all point related to a point P ≠ (0, 0) is the circle passing through P with origin as centre.
A.11. The given relation in set A of points in a plane is
R= distance of point P from origin=distance of point Q from
If O is the point of origin
R=
Then, for we have PO=PO
So,
i.e., P is reflexive
for, and we have
PO=QO
QO=PO i.e.,
i.e., R is symmetric
for and
PO=QO and QO=SO
PO=SO
i.e.,
so, R is transitive
Hence, R is an equivalence relation
For a point the set of all points related to P i.e., distance from origin to the points are equal is a circle with center at origin (o,o) by the definition of circle
Q12. Show that the relation R defined in the set A of all triangles as R = {(T1, T2): T1 is similar to T2}, is equivalence relation. Consider three right angle triangles T1 with sides 3, 4, 5, T2 with sides 5, 12, 13 and T3 with sides 6, 8, 10. Which triangles among T1, T2 and T3 are related?
A.12. The given relation to set A of all triangles is defined as
R= is similar to
For ,
is always similar to
So, . Hence R is reflexive.
For and we have
i.e.,
so, R is symmetric.
for, and and
and
i.e.,
so, R is transitive
R is an equivalence relation.
Given, sides of are 3,4,5
Sides of are 5,12,13
Sides of are 6,8,10
As we conclude that is not similar to
As we conclude that is not similar to
But as we conclude that
Hence, is related to
Q13. Show that the relation R defined in the set A of all polygons as R = {(P1, P2): P1 and P2 have same number of sides}, is an equivalence relation. What is the set of all elements in A related to the right-angle triangle T with sides 3, 4 and 5?
A.13. The given relation in set A of all polygons is defined as
R= and have same number of sides
Let ,
As number of sides = number of sides
So, R is reflexive.
Let and
Then, number of sides of = number of sides of
Number of sides of = number of sides of
i.e.,
so, R is symmetric.
Let and and
Then, number of sides = number of sides
Number of sides = number of sides
So, number of sides = number of sides
I.e.,
So, R is transitive.
Hence, R is an equivalence relation.
The set of all triangles will be the elements in A related to the right-angle triangle T with sides 3,4 and 5 as they all have three number of sides.
Q14. Let L be the set of all lines in XY plane and R be the relation in L defined as R = {(L1, L2): L1 is parallel to L2}. Show that R is an equivalence relation. Find the set of all lines related to the line y = 2x + 4.
A.14. The given relation in the set all lines in plane is defined as
is parallel to
Let then as is parallel to ,
So, is reflexive
Let and
Then, is parallel to
is parallel to
So,
i.e., is symmetric
Let and and
Then, and
So,
i.e.,
So, is transitive
Hence, is an equivalence relation
The set of lines related to is given by the equation where is some constant.
Q15. Let R be the relation in the set {1, 2, 3, 4} given by R = {(1, 2), (2, 2), (1, 1), (4, 4), (1, 3), (3, 3), (3, 2)}. Choose the correct answer.
(A) R is reflexive and symmetric but not transitive.
(B) R is reflexive and transitive but not symmetric.
(C) R is symmetric and transitive but not reflexive.
(D) R is an equivalence relation.
A.15. The set in
The relation in this set is given by
is reflexive as
As, but
is not symmetric
For and
And for and
∴ is transitive
Hence, option (B) is correct
Q16. Let R be the relation in the set N given by R = {(a, b): a = b − 2, b > 6}. Choose the correct answer.
(A) (2, 4) ∈ R (B) (3, 8) ∈R (C) (6, 8) ∈R (D) (8, 7) ∈ R
A.16. The given relation in set N defined by
For (2,4), 4>6 is not true
For (3,8), 8>6 but 3= 8-2 ⇒3=6 is not true
For (6,8), 8>6 and 6= 8-2 ⇒6=6 is true
And for (8,7), 7>6 but 8= 7-2 ⇒8=5 is not true
Hence, option (C) is correct
Class 12 Math Chapter 1 Relation and Function Exercise 1.2 Solutions
Chapter 1 Relation and Function Exercise 1.2 mainly focuses on functions and their types, such as many-one, onto, and into, and one-one functions. It also deals with problems of finding the range, domain, co-domain, and other elements of functions. Class 12 Math Relation and Function Exercise 1.2 consists of 12 Questions (10 Short Answers, 2 MCQ). students can check the complete solutions for exercise 1.2 below;
Relation and Function Exercise 1.2 Solutions
Q1. Show that the function f: R* → R* defined by f(x) = 1/x is one-one and onto, where: R* is the set of all non-zero real numbers. Is the result true, if the domain: R* is replaced by N with the co-domain being same as: R*?
A.1. The n is , which is a and is set of all non-zero real numbers
For,
So, is one-one
For, such that
So,
So, every element in the co-domain has a pre-image in
So, is onto
If such that
For,
So, is one-one
For, and we have
Eg., so
So, is not onto
Q2. Check the injectivity and surjectivity of the following functions:
(i) f: N → N given by f(x) = x2
(ii) f: Z → Z given by f(x) = x2
(iii) f: R → R given by f(x) = x2
(iv) f: N → N given by f(x) = x3
(v) f: Z → Z given by f(x) = x3
A.2. (i) given by
For, ,
So, is one-one/ injective
For , i.e.,
Range of
i.e., co-domain of
So, is not onto/ subjective
(ii) given by
For, ,
i.e., and
So, is not one-one/ injective
For ,
Range of
co-domain
So, is not onto/ subjective
(iii) given by
For, ,
So, is not injective
For
Range of gives a set of all positive real numbers
Hence, range of co-domain of
So, is not subjective
(iv) given by
For, ,
So, is injective
For
Range of
co-domain of
So, is not subjective
(v) given by
For, ,
So, is injective
For
Range of
co-domain of R
So, is not subjective
Q3.Prove that the Greatest Integer Function f: R → R given by f(x) = [x], is neither one-one nor onto, where [x] denotes the greatest integer less than or equal to x.
A.3. The is given by
Let and Then,
So, but
i.e., but
So, is not one-one
The range of is a set of all integers, which is not a co-domain of
is not onto
Q4. Show that the Modulus Function f : R → R, given by f(x) = |x| is neither one-one nor onto, where is |x| if x is positive or 0 and |-x| is -x if x is negative.
A.4. The is given by
For and
So, but
i.e., is not one-one
For
i.e.,
So, range of is always a positive real number and is not equal to the co-domain
i.e., is not onto
Q5. Show that the Signum Function f: R → R , given by
is neither one-one nor onto.
A.5. The is given by
For
but
So, is not one-one
And the range of hence it is not equal to the co-domain
So, is not onto
Q6. Let A = {1, 2, 3}, B = {4, 5, 6, 7} and let f = {(1, 4), (2, 5), (3, 6)} be a function from A to B. Show that f is one-one.
A.6. Given, and
i.e., the image elements of under the given are unique
So, is one-one
Q7. In each of the following cases, state whether the function is one-one, onto or bijective. Justify your answer.
(i) f: R → R defined by f(x) = 3 − 4x
(ii) f: R → R defined by f(x) = 1 + x2
A.7. (i) defined as
For such that
So, is one-one
For , there exist
Hence, is onto
is bijective
(ii)Given, defined as
For such that
or
is not one-one
The range of is always a positive real number which is not equal to co-domain
So, is not onto
Q8. Let A and B be sets. Show that f: A × B → B × A such that (a, b) = (b, a) is bijective function.
A.8. Given, defined as
Let such that
So, and
is one-one
For
There exist such that
is onto
Hence, is bijective
Q9. Let N → N be defined by f(n) for all
State whether the function f is bijective. Justify your answer.
A.9. Given, defined
Let and
but
So, is not one-one
For odd and , say where
There exist such that
And for even , say where
There exist such that
So, is onto
But, is not bijective
Q10. Let A = R – {3} and B = R – {1}. Consider the function f : A → B defined by f(x) = (x-2/x-3) Is f one-one and onto? Justify your answer.
A.10. Given, defined by
Let such that
So, is one-one
For there exist such that
where
Thus,
is onto
Q11. Let f: R → R be defined as f(x) = x4. Choose the correct answer.
(A) f is one-one onto
(B) f is many-one onto
(C) f is one-one but not onto
(D) f is neither one-one nor onto
A.11. Given, defined by
For such that
or
So, is not one-one
The range of is a set of all positive real numbers which is not equal to co-domain
So, in not onto
Option (D) is correct
Q12.Let f: R → R be defined as f(x) = 3x. Choose the correct answer.
(A) f is one-one onto
(B) f is many-one onto
(C) f is one-one but not onto
(D) f is neither one-one nor onto
A.12. Given, defined as
For such that
So, is one-one
And for , there exist such that
is onto
Hence, option (A) is correct.
Class 12 Math Chapter 1 Relation and Function Old NCERT Solutions
Students can also check the old NCERT solutions of relation and function chapter below for exercises 1.3 and 1.4 solutions. These topics are dropped from the latest CBSE syllabus, Exercise 1.3 & 1.4 discusses composition of functions and the inverse of functions. These topics are still relevant for competitive exams like JEE main, CUSAT CAT, MHT CET, and others.
Relation and Function Exercise 1.3 Solutions
Q1. Let f: {1, 3, 4} → {1, 2, 5} and g: {1, 2, 5} → {1, 3} be given by f = {(1, 2), (3, 5), (4, 1)} and g = {(1, 3), (2, 3), (5, 1)}. Write down gof.
A.1. The functions f: {1, 3, 4} → {1, 2, 5} and g: {1, 2, 5} → {1, 3} are defined as
f = {(1, 2), (3, 5), (4, 1)} and g = {(1, 3), (2, 3), (5, 1)}.
gof(1) = g(f(1)) = g(2) = 3 [f(1) = 2 and g(2) = 3]
gof(3) = g(f(3)) = g(5) = 1[f(3) = 5 and g(5) = 1]
gof(4) = g(f(4)) = g(1) = 3[f(4) = 1 and g(1) = 3]
gof = {(1, 3), (3, 1), (4, 3)}
Q2. Let f, g and h be functions from R to R. Show that:
(f + g) oh = foh + goh
(f.g) oh = (foh). (goh)
A.2. To prove:
To prove
Q3. Find gof and fog , if:
(i) f(x) = |x| and g(x) = |5x - 2|
(ii) f(x) = 8x3 and g(x) = x1/3
A.3.
Q4. If show that( fof) (x) = x for all x ≠ 2/3 What is the inverse of f
A.4. It is given that
Therefore for all
Hence, the given function f is invertible and the inverse of f is itself.
Q5. State with reason whether following functions have inverse
(i) f: {1, 2, 3, 4} → {10} with f = {(1, 10), (2, 10), (3, 10), (4, 10)}
(ii) g: {5, 6, 7, 8} → {1, 2, 3, 4} with g = {(5, 4), (6, 3), (7, 4), (8, 2)}
(iii) h: {2, 3, 4, 5} → {7, 9, 11, 13} with h = {(2, 7), (3, 9), (4, 11), (5, 13)}
A.5. (i) f: {1, 2, 3, 4} → {10} defined as:
f = {(1, 10), (2, 10), (3, 10), (4, 10)}
From the given definition of f, we can see that f is a many one function as: f(1) = f(2) = f(3) = f(4) = 10
∴f is not one-one.
Hence, function f does not have an inverse.
(ii) g: {5, 6, 7, 8} → {1, 2, 3, 4} defined as:
g = {(5, 4), (6, 3), (7, 4), (8, 2)}
From the given definition of g, it is seen that g is a many one function as: g(5) = g(7) = 4.
∴g is not one-one,
Hence, function g does not have an inverse.
(iii) h: {2, 3, 4, 5} → {7, 9, 11, 13} defined as:
h = {(2, 7), (3, 9), (4, 11), (5, 13)}
It is seen that all distinct elements of the set {2, 3, 4, 5} have distinct images under h.
∴Function h is one-one.
Also, h is onto since for every element y of the set {7, 9, 11, 13}, there exists an element x in the set {2, 3, 4, 5} such that h(x) = y.
Thus, h is a one-one and onto function. Hence, h has an inverse.
Q6. Show that f: [-1, 1] → R given by f(x) = x/x+2 is one-one. Find the inverse of the function f: [-1, 1] → Range f
A.6. is given as
f is a one-one function.
It is clear that Range f is onto.
Range f is one-one onto and therefore, the inverse of the function:
Range f exists.
Let g: Range be the inverse of f.
Let y be an arbitrary element of range f.
Since Range f is onto, we have:
Q7. Consider f : R→ R given by f(x) = 4x + 3 Show that f is invertible. Find the inverse of f.
A.7. is given by,
f is a one-one function.
Onto:
Therefore, for any , there exists such that
f is onto.
Thus, f is one-one and onto and therefore, exists.
Let us define by
Hence, f is invertible and the inverse of f is given by
Q8. Consider f: R+ → [4, ∞) given by f(x) = x2 + 4 Show that f is invertible with the inverse f−1 of f given by f−1(y) = √y - 4 where R+ is the set of all non-negative real numbers.
A.8. is given as .
One-one:
is a one-one function.
Q10. Let f: X → Y be an invertible function. Show that f has unique inverse.
(Hint: suppose g1 and g2 are two inverses of f. Then for all y ∈ Y, fog1(y) = IY(y) = fog2(y). Use one-one ness of f).
A.10. Let be an invertible function.
Also, suppose f has two inverses (say g1 and g2 ).
Then, for all y ∈ Y, we have:
[f is invertible => f is one-one]
[g is one-one]
Hence, f has a unique inverse.
Q11. Consider f: {1, 2, 3} → {a, b, c} given by f(1) = a, f(2) = b and f(3) = c. Find f−1 and show that (f−1)−1 = f.
A.11.
If we define then we have:
Thus, the inverse of f exists and
is given by,
Let us now find the inverse of i.e., find the inverse of g.
If we define
, then we have
Thus, the inverse of g exists and
It can be noted that h=f.
Hence,
Q12. Let f: X → Y be an invertible function. Show that the inverse of f−1 is f, i.e., (f−1)−1 = f.
A.12. Let f: X → Y be an invertible function.
Then, there exists a function g: Y → X such that gof = IX and fog = IY.
Here, f−1 = g.
Now, gof = IX and fog = IY
⇒ f−1 of = IX and fof−1 = IY
Hence, f−1 : Y → X is invertible and f is the inverse of f−1
i.e., (f−1)−1 = f.
Q13. If be given by , then f of(x) is
(A) 1/x3
(B) x3
(C) x
(D) (3 − x3)
A.13. is given as
The correct answer is C.
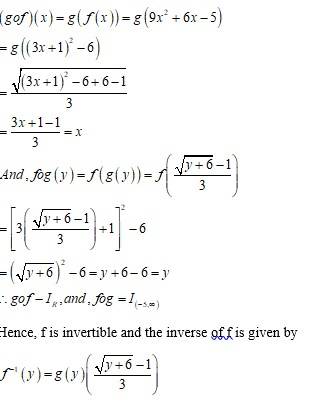
Q14. Let be a function defined as . The inverse of f is map g Range
A.14. It is given that is defined as
Let y be an arbitrary element of Range f.
Then, there exists such that
let us define g: Range
Thus, g is the inverse of f i.e.,
Hence, the inverse of f is the map g: Range which is given by
The correct answer is B.
EXERCISE 1.4
Q1. Determine whether or not each of the definition of given below gives a binary operation. In the event that * is not a binary operation, give justification for this.
(i) On Z+ , define * by a * b = a − b
(ii) On Z+, define * by a * b = ab
(iii) On R, define * by a * b = ab2
(iv) On Z+, define * by a * b = |a − b|
(v) On Z+, define * by a * b = a
A.1. (i) On Z+, * is defined by a * b = a − b.
It is not a binary operation as the image of (1, 2) under * is 1 * 2 = 1 − 2= −1 ∉ Z+.
(ii) On Z+, * is defined by a * b = ab.
It is seen that for each a, b ∈ Z+, there is a unique element ab in Z+.
This means that * carries each pair (a, b) to a unique element a * b = ab in Z+.
Therefore, * is a binary operation.
(iii) On R, * is defined by a * b = ab2.
It is seen that for each a, b ∈ R, there is a unique element ab2 in R.
This means that * carries each pair (a, b) to a unique element a * b = ab2 in R.
Therefore, * is a binary operation.
(iv) On Z+, * is defined by a * b = |a − b|.
It is seen that for each a, b ∈ Z+, there is a unique element |a − b| in Z+.
This means that * carries each pair (a, b) to a unique element a * b = |a − b| in Z+.
Therefore, * is a binary operation.
(v) On Z+, * is defined by a * b = a.
* carries each pair (a, b) to a unique element a * b = a in Z+.
Therefore, * is a binary operation.
Q2. For each binary operation * defined below, determine whether * is commutative or associative:
(i) On Z, define a * b = a − b
(ii) On Q, define a * b = ab + 1
(iii) On Q, define a * b =ab/2
(iv) On Z+, define a * b = 2ab
(v) On Z+, define a * b = ab
(vi) On R − {−1}, define a.b = a/b+1
A.2. (i) On Z, * is defined by a * b = a − b.
It can be observed that 1 * 2 = 1 − 2 = 1 and 2 * 1 = 2 − 1 = 1.
∴1 * 2 ≠ 2 * 1; where 1, 2 ∈ Z
Hence, the operation * is not commutative.
Also we have:
(1 * 2) * 3 = (1 − 2) * 3 = −1 * 3 = −1 − 3 = −4
1 * (2 * 3) = 1 * (2 − 3) = 1 * −1 = 1 − (−1) = 2
∴(1 * 2) * 3 ≠ 1 * (2 * 3) ; where 1, 2, 3 ∈ Z
Hence, the operation * is not associative.
(ii) On Q, * is defined by a * b = ab + 1.
It is known that:
ab = ba & mn For E; a, b ∈ Q
⇒ ab + 1 = ba + 1 & mn For E; a, b ∈ Q
⇒ a * b = a * b &mn For E; a, b ∈ Q
Therefore, the operation * is commutative.
It can be observed that:
(1 * 2) * 3 = (1 × 2 + 1) * 3 = 3 * 3 = 3 × 3 + 1 = 10
1 * (2 * 3) = 1 * (2 × 3 + 1) = 1 * 7 = 1 × 7 + 1 = 8
∴(1 * 2) * 3 ≠ 1 * (2 * 3) ; where 1, 2, 3 ∈ Q
Therefore, the operation * is not associative.
(iii) On Q, * is defined by a * b = ab/2
It is known that:
ab = ba &mnForE; a, b ∈ Q
⇒ab/2 = ba/2 &mnForE; a, b ∈ Q
⇒ a * b = b * a &mnForE; a, b ∈ Q
Therefore, the operation * is commutative.
For all a, b, c ∈ Q, we have:
Therefore, the operation * is associative.
(iv) On Z+, * is defined by a * b = 2ab.
It is known that:
ab = ba &mnForE; a, b ∈ Z+
⇒ 2ab = 2ba &mnForE; a, b ∈ Z+
⇒ a * b = b * a &mnForE; a, b ∈ Z+
Therefore, the operation * is commutative.
It can be observed that:
∴(1 * 2) * 3 ≠ 1 * (2 * 3) ; where 1, 2, 3 ∈ Z+
Therefore, the operation * is not associative.
(v) On Z+, * is defined by a * b = ab.
It can be observed that:
1 x2 = 12=1 and 2x 1 =21 = 2
∴ 1 * 2 ≠ 2 * 1 ; where 1, 2 ∈ Z+
Therefore, the operation * is not commutative.
It can also be observed that:
∴(2 * 3) * 4 ≠ 2 * (3 * 4) ; where 2, 3, 4 ∈ Z+
Therefore, the operation * is not associative.
(vi) On R, * − {−1} is defined by a.b = a/b+1
It can be observed that 1 x 2 = 1/2+1 = 1/2 and 2 x 1 = 2/1+1 = 2/2
∴1 * 2 ≠ 2 * 1 ; where 1, 2 ∈ R − {−1}
Therefore, the operation * is not commutative.
It can also be observed that:
∴ (1 * 2) * 3 ≠ 1 * (2 * 3) ; where 1, 2, 3 ∈ R − {−1}
Therefore, the operation * is not associative.
Q3. Consider the binary operation ∨ on the set {1, 2, 3, 4, 5} defined by a ∨b = min {a, b}. Write the operation table of the operation∨.
A.3. The binary operation ∨ on the set {1, 2, 3, 4, 5} is defined as a ∨ b = min {a, b} &mn For E; a, b ∈ {1, 2, 3, 4, 5}.
Thus, the operation table for the given operation ∨ can be given as:
| ∨ |
1 |
2 |
3 |
4 |
5 |
| 1 |
1 |
1 |
1 |
1 |
1 |
| 2 |
1 |
2 |
2 |
2 |
2 |
| 3 |
1 |
2 |
3 |
3 |
3 |
| 4 |
1 |
2 |
3 |
4 |
4 |
| 5 |
1 |
2 |
3 |
4 |
5 |
Q4. Consider a binary operation * on the set {1, 2, 3, 4, 5} given by the following multiplication table.
(i) Compute (2 * 3) * 4 and 2 * (3 * 4)
(ii) Is * commutative?
(iii) Compute (2 * 3) * (4 * 5).
(Hint: use the following table)
| * |
1 |
2 |
3 |
4 |
5 |
| 1 |
1 |
1 |
1 |
1 |
1 |
| 2 |
1 |
2 |
1 |
2 |
1 |
| 3 |
1 |
1 |
3 |
1 |
1 |
| 4 |
1 |
2 |
1 |
4 |
1 |
| 5 |
1 |
1 |
1 |
1 |
5 |
A.4. (i) (2 * 3) * 4 = 1 * 4 = 1
2 * (3 * 4) = 2 * 1 = 1
(ii) For every a, b ∈{1, 2, 3, 4, 5}, we have a * b = b * a. Therefore, the operation * is commutative.
(iii) (2 * 3) = 1 and (4 * 5) = 1
∴ (2 * 3) * (4 * 5) = 1 * 1 = 1
Q5. Let*′ be the binary operation on the set {1, 2, 3, 4, 5} defined by a *′ b = H.C.F. of a and b. Is the operation *′ same as the operation * defined in Exercise 4 above? Justify your answer.
A.5. The binary operation *′ on the set {1, 2, 3 4, 5} is defined as a *′ b = H.C.F of a and b.
The operation table for the operation *′ can be given as:
| *′ |
1 |
2 |
3 |
4 |
5 |
| 1 |
1 |
1 |
1 |
1 |
1 |
| 2 |
1 |
2 |
1 |
2 |
1 |
| 3 |
1 |
1 |
3 |
1 |
1 |
| 4 |
1 |
2 |
1 |
4 |
1 |
| 5 |
1 |
1 |
1 |
1 |
5 |
We observe that the operation tables for the operations * and *′ are the same.
Thus, the operation *′ is same as the operation*.
Q6. Let * be the binary operation on N given by a * b = L.C.M. of a and b. Find
(i) 5 * 7, 20 * 16 (ii) Is * commutative?
(iii) Is * associative? (iv) Find the identity of * in N
(v) Which elements of N are invertible for the operation *?
A.6. The binary operation * on N is defined as a * b = L.C.M. of a and b.
(i) 5 * 7 = L.C.M. of 5 and 7 = 35
20 * 16 = L.C.M of 20 and 16 = 80
(ii) It is known that:
L.C.M of a and b = L.C.M of b and a & mnForE; a, b ∈ N.
∴a * b = b * a
Thus, the operation * is commutative.
(iii) For a, b, c ∈ N, we have:
(a * b) * c = (L.C.M of a and b) * c = LCM of a, b, and c
a * (b * c) = a * (LCM of b and c) = L.C.M of a, b, and c
∴ (a * b) * c = a * (b * c)
Thus, the operation * is associative.
(iv) It is known that:
L.C.M. of a and 1 = a = L.C.M. 1 and a &mnForE; a ∈ N
⇒ a * 1 = a = 1 * a &mnForE; a ∈ N
Thus, 1 is the identity of * in N.
(v) An element a in N is invertible with respect to the operation * if there exists an element b in N, such that a * b = e = b * a.
Here, e = 1
This means that:
L.C.M of a and b = 1 = L.C.M of b and a
This case is possible only when a and b are equal to 1.
Thus, 1 is the only invertible element of N with respect to the operation *.
Q7. Is * defined on the set {1, 2, 3, 4, 5} by a * b = L.C.M. of a and b a binary operation? Justify your answer.
A.7. The operation * on the set A = {1, 2, 3, 4, 5} is defined as
a * b = L.C.M. of a and b.
Then, the operation table for the given operation * can be given as:
| * |
1 |
2 |
3 |
4 |
5 |
| 1 |
1 |
2 |
3 |
4 |
5 |
| 2 |
2 |
2 |
6 |
4 |
10 |
| 3 |
3 |
6 |
3 |
12 |
15 |
| 4 |
4 |
4 |
12 |
4 |
20 |
| 5 |
5 |
10 |
15 |
20 |
5 |
It can be observed from the obtained table that:
3 * 2 = 2 * 3 = 6 ∉ A, 5 * 2 = 2 * 5 = 10 ∉ A, 3 * 4 = 4 * 3 = 12 ∉ A
3 * 5 = 5 * 3 = 15 ∉ A, 4 * 5 = 5 * 4 = 20 ∉ A
Hence, the given operation * is not a binary operation.
Q8. Let * be the binary operation on N defined by a * b = H.C.F. of a and b. Is * commutative? Is * associative? Does there exist identity for this binary operation on N?
A.8. The binary operation * on N is defined as:
a * b = H.C.F. of a and b
It is known that:
H.C.F. of a and b = H.C.F. of b and a & mn For E; a, b ∈ N.
∴a * b = b * a
Thus, the operation * is commutative.
For a, b, c ∈ N, we have:
(a * b)* c = (H.C.F. of a and b) * c = H.C.F. of a, b, and c
a *(b * c)= a *(H.C.F. of b and c) = H.C.F. of a, b, and c
∴(a * b) * c = a * (b * c)
Thus, the operation * is associative.
Now, an element e ∈ N will be the identity for the operation * if a * e = a = e* a ∈ a ∈ N.
But this relation is not true for any a ∈ N.
Thus, the operation * does not have any identity in N.
Q9. Let * be a binary operation on the set Q of rational numbers as follows:
(i) a * b = a − b
(ii) a * b = a2 + b2
(iii) a * b = a + ab
(iv) a * b = (a − b)2
(v) a * b = ab/4 (vi) a * b = ab2
Find which of the binary operations are commutative and which are associative.
A.9. (i) On Q the operation* is defined as a*b=a-b.
It can be observed that:
Thus, the operation* is not commutative.
It can also be observed that:
Thus, the operation* is not associative.
(ii) On Q the operation* is defined as a*b=a2+b.
For , we have:
Thus, the operation* is commutative.
It can be observed that:
Thus, the operation* is not commutative.
(iii) On Q the operation* is defined as a*b=a+ab.
It can be observed that:
Thus, the operation* is not commutative.
It can also be observed that:
Thus, the operation* is not associative.
(iv) On Q the operation* is defined as a*b=(a-b)2
For , we have:
Thus, the operation* is commutative.
It can be observed that:
Thus, the operation* is not associative.
(v) On Q the operation* is defined as a.b=ab/4
For , we have:
Thus, the operation* is commutative.
For , we have:
Thus, the operation* is associative.
(vi) On Q the operation* is defined as a*b=ab2
It can be observed that:
Thus, the operation* is not commutative.
It can be observed that:
Thus, the operation* is not associative.
Hence, the operations defined (ii), (iv), (v) are commutative and the operation defined in (v) is associative.
Q10. Find which of the operations given above has identity.
A.10. Let the identity be I.
An element will be the identity element for the operation* if
We are given
Similarly, it can be checked for , we get e=4. Thus, e=4 is the identity
Q11. Let A = N × N and * be the binary operation on A defined by
(a, b) * (c, d) = (a + c, b + d)
Show that * is commutative and associative. Find the identity element for * on A, if any.
A.11. A = N × N
* is a binary operation on A and is defined by:
(a, b) * (c, d) = (a + c, b + d)
Let (a, b), (c, d) ∈ A
Then, a, b, c, d ∈ N
We have:
(a, b) * (c, d) = (a + c, b + d)
(c, d) * (a, b) = (c + a, d + b) = (a + c, b + d)
[Addition is commutative in the set of natural numbers]
∴(a, b) * (c, d) = (c, d) * (a, b)
Therefore, the operation * is commutative.
Now, let (a, b), (c, d), (e, f) ∈A
Then, a, b, c, d, e, f ∈ N
We have:
Therefore, the operation* is associative.
An element will be an identity element for the operation* if
which is not true for any element in A.
Therefore, the operation* does not have any identity element.
Which is not true for any element in A.
Therefore, the operation * does not have any identity element.
Q12. State whether the following statements are true or false. Justify.
(i) For an arbitrary binary operation * on a set N, a * a = a ∀ a * N.
(ii) If * is a commutative binary operation on N, then a * (b * c) = (c * b) * a
A.12. (i) Define an operation * on N as:
a * b = a + b a, b ∈ N
Then, in particular, for b = a = 3, we have:
3 * 3 = 3 + 3 = 6 ≠ 3
Therefore, statement (i) is false.
(ii) R.H.S. = (c * b) * a
= (b * c) * a [* is commutative]
= a * (b * c) [Again, as * is commutative]
= L.H.S.
∴ a * (b * c) = (c * b) * a
Therefore, statement (ii) is true.
Q13. Consider a binary operation * on N defined as a * b = a3 + b3. Choose the correct answer.
(A) Is * both associative and commutative?
(B) Is * commutative but not associative?
(C) Is * associative but not commutative?
(D) Is * neither commutative nor associative?
A13. On N, the operation * is defined as a * b = a3 + b3.
For, a, b, ∈ N, we have:
a * b = a3 + b3 = b3 + a3 = b * a [Addition is commutative in N]
Therefore, the operation * is commutative.
It can be observed that:
(1*2)*3 = (13+23)*3 = 9 * 3 = 93 + 33 = 729 + 27 = 756
Also, 1*(2*3) = 1*(23 +33) = 1*(8 +27) = 1 × 35
= 13 +353 = 1 +(35)3 = 1 + 42875 = 42876.
∴(1 * 2) * 3 ≠ 1 * (2 * 3) ; where 1, 2, 3 ∈ N
Therefore, the operation * is not associative.
Hence, the operation * is commutative, but not associative. Thus, the correct answer is B.
MISCELLANEOUS
Q1. Let f: R → R be defined as f(x) = 10x + 7. Find the function g: R → R such that g o f = f o g = 1R.
A.1. It is given that is defined as
One-one:
is a one-one function.
Onto:
Therefore, for any ,there exists
Such that
is onto.
Therefore, f is one-one and onto.
Thus, f is an invertible function.
Let us define as
Now, we have
Hence, the required function is defined as
Q2. Let f: W → W be defined as f(n) = n − 1, if is odd and f(n) = n + 1, if n is even. Show that f is invertible. Find the inverse of f. Here, W is the set of all whole numbers.
A.2. It is given that:
is defined as
One-one:
Let,
It can be observed that if n is odd and m is even, then we will have n-1=m+1.
However, the possibility of n being even and m being odd can also be ignored under a similar argument.
Both n and m must be either odd or even.
Now, if both n and m are odd, then we have:
Again, if both n and m are even, then we have:
is one-one.
It is clear that any odd number 2r+1 in co-domain N is the image of 2r in domain N and any even 2r in co-domain N is the image of 2r+1 in domain N.
is onto.
Hence, f is an invertible function.
Let us define as:
Now, when n is odd:
And, when n is even:
Similarly, when m is odd:
When m is even:
Thus, f is invertible and the inverse of f is given by , which is the same as f.
Hence, the inverse of f is f itself.
Q3. If f: R → R is defined by f(x) = x2 − 3x + 2, find f(f(x)).
A.3. It is given that is defined as
Q4. Show that function f: R → {x ∈ R: −1 < x < 1} defined by f(x) = x/1+|x| x ∈R is one-one and onto function.
A.4. It is given that is defined as
Suppose , where
Since x is positive and y is negative:
But, 2xy is negative.
Then, .
Thus, the case of x being positive and y being negative can be ruled out.
Under a similar argument, x being negative and y being positive can also be ruled out
x and y have to be either positive or negative.
When x and y are both positive, we have:
When x and y are both negative, we have:
is one-one.
Now, let such that .
If x is negative, then there exists such that
If x is positive, then there exists such that
is onto.
Hence, f is one-one and onto.
Q5. Show that the function f: R → R given by f(x) = x3 is injective.
A.5. f: R → R is given as f(x) = x3.
Suppose f(x) = f(y), where x, y ∈ R.
⇒ x3 = y3 … (1)
Now, we need to show that x = y.
Suppose x ≠ y, their cubes will also not be equal.
⇒ x3 ≠ y3
However, this will be a contradiction to (1).
∴ x = y
Hence, f is injective.
Q6. Give examples of two functions f: N → Z and g: Z → Z such that g o f is injective but g is not injective.
(Hint: Consider f(x) = x and g(x) = |x| )
A.6. Define as and as
We first show that g is not injective.
It can be observed that:
is not injective.
Now, is defined as
Let such that
Since , both are positive.
Hence, gof is injective
Q7. Given examples of two functions f: N → N and g: N → N such that gof is onto but f is not onto.
(Hint: Consider f(x) = x + 1 and
A.7. Define by
And, by,
We first show that g is not onto.
For this, consider element 1 in co-domain N. it is clear that this element is not an image of any of the elements in domain.
is not onto.
Now, is defined by,
Then, it is clear that for , there exists such that
Hence, gof is onto.
Q8. Given a non empty set X, consider P(X) which is the set of all subsets of X.
Define the relation R in P(X) as follows: For subsets A, B in P(X), ARB if and only if A ⊂ B. Is R an equivalence relation on P(X)? Justify you answer:
A.8. Since every set is a subset of itself, ARA for all A ∈ P(X).
∴R is reflexive.
Let ARB ⇒ A ⊂ B.
This cannot be implied to B ⊂ A.
For instance, if A = {1, 2} and B = {1, 2, 3}, then it cannot be implied that B is related to A.
∴ R is not symmetric.
Further, if ARB and BRC, then A ⊂ B and B ⊂ C.
⇒ A ⊂ C
⇒ ARC
∴ R is transitive.
Hence, R is not an equivalence relation since it is not symmetric.
Q9. Given a non-empty set X, consider the binary operation *: P(X) × P(X) → P(X) given by A * B = A ∩ B &mn For E; A, B in P(X) is the power set of X. Show that X is the identity element for this operation and X is the only invertible element in P(X) with respect to the operation*.
A.9. Let S be a non-empty set and P(S) be its power set. Let any two subsets A and B of S.
It is given that: is defined as
We know that
Thus, X is the identity element for the given binary operation*.
Now, an element is invertible if there exists such that
(As X is the identity element)
i.e.
This case is possible only when
Thus, X is the only invertible element in P(X) with respect to the given operation*.
Hence, the given result is proved.
Q10. Find the number of all onto functions from the set {1, 2, 3, … , n) to itself.
A.10. Onto functions from the set {1, 2, 3, … ,n} to itself is simply a permutation on n symbols 1, 2, …, n.
Thus, the total number of onto maps from {1, 2, … , n} to itself is the same as the total number of permutations on n symbols 1, 2, …, n, which is n!.
Q11. Let S = {a, b, c} and T = {1, 2, 3}. Find F−1 of the following functions F from S to T, if it exists.
(i) F = {(a, 3), (b, 2), (c, 1)} (ii) F = {(a, 2), (b, 1), (c, 1)}
A.11. S = {a, b, c}, T = {1, 2, 3}
(i) F: S → T is defined as:
F = {(a, 3), (b, 2), (c, 1)}
⇒ F (a) = 3, F (b) = 2, F(c) = 1
Therefore, F−1 : T → S is given by
F−1 = {(3, a), (2, b), (1, c)}.
(ii) F: S → T is defined as:
F = {(a, 2), (b, 1), (c, 1)}
Since F (b) = F (c) = 1, F is not one-one.
Hence, F is not invertible i.e., F−1 does not exist.
Q12. Consider the binary operations*: R ×R → and o: R × R → R defined as a * b = |a - b| and ao b = a, &mn For E; a, b ∈ R. Show that * is commutative but not associative, o is associative but not commutative. Further, show that &mn For E;a, b, c ∈ R, a*(b o c) = (a * b) o (a * c). [If it is so, we say that the operation * distributes over the operation o]. Does o distribute over *? Justify your answer.
A.12. It is given that*: and is defined as
For , we have:
The operation* is commutative.
It can be observed that,
The operation* is not associative.
Now, consider the operation o:
It can be observed that
The operation o is not commutative.
Let, . Then we have:
The operation o is associative.
Now, . Then we have:
The operation o does not distribute over*.
Q13. Given a non-empty set X, let *: P(X) × P(X) → P(X) be defined as A * B = (A − B) ∪ (B − A), &mn For E; A, B ∈ P(X). Show that the empty set Φ is the identity for the operation * and all the elements A of P(X) are invertible with A−1 = A. (Hint: (A − Φ) ∪ (Φ − A) = A and (A − A) ∪ (A − A) = A * A = Φ).
A.13. : It is given that ∗: P(X) × P(X) → P(X) be defined as
A * B = (A – B) ∪ (B – A), A, B ∈ P(X).
Now, let A ϵ P(X). Then, we get,
A * ф = (A – ф) ∪ (ф –A) = A∪ф = A
ф * A = (ф - A) ∪ (A - ф ) = ф∪A = A
A * ф = A = ф * A, A ϵ P(X)
Therefore, ф is the identity element for the given operation *.
Now, an element A ϵ P(X) will be invertible if there exists B ϵ P(X) such that
A * B = ф = B * A. (as ф is an identity element.)
Now, we can see that A * A = (A –A) ∪ (A – A) = ф∪ф = ф AϵP(X).
Therefore, all the element A of P(X) are invertible with A-1 = A.
Q14. Define binary operation * on the set {0, 1, 2, 3, 4, 5} as
Show that zero is the identity for this operation and each element a ≠ 0 of the set is invertible with 6 - abeing the inverse of a
A.14. Let X={0, 1, 2, 3, 4, 5}.
The operation* on X is defined as:
An element is the identity element for the operation*, if
For we observed that
Thus, 0 is the identity element for the given operation*.
An element is invertible if there exists such that
i.e.,
But, X={0, 1, 2, 3, 4, 5} and . Then, .
is the inverse of
Hence, the inverse of an element is 6-a i.e.,
| * |
0 |
1 |
2 |
3 |
4 |
5 |
| 0 |
0 |
1 |
2 |
3 |
4 |
5 |
| 1 |
1 |
2 |
3 |
4 |
5 |
0 |
| 2 |
2 |
3 |
4 |
5 |
0 |
1 |
| 3 |
3 |
4 |
5 |
0 |
1 |
2 |
| 4 |
4 |
5 |
0 |
1 |
2 |
3 |
| 5 |
5 |
0 |
1 |
2 |
3 |
4 |
Q15. Let A = {–1, 0, 1, 2}, B = {–4, –2, 0, 2} and f; g: A→B be the functions defined by f(x) = x2 − x, x ∈ A and g(x) = 2x - 1/2 - 1. x∈ A. Are f and g equal? Justify your answer.
(Hint: One may note that two functions f: A → B and g: A → B such that f(a) = g(a) & mn For E; a ∈A, are called equal functions).
A.15. It is given that A = {–1, 0, 1, 2}, B = {–4, –2, 0, 2}
Also, it is given that are defined by and .
It is observed that:
Hence, the functions f and g are equal.
Q16. Let A = {1, 2, 3}. Then number of relations containing (1, 2) and (1, 3) which are reflexive and symmetric but not transitive is:
(A) 1
(B) 2
(C) 3
(D) 4
A.16. It is clear that 1 is reflexive and symmetric but not transitive.
Therefore, option (A) is correct.
Q17. Let A = {1, 2, 3}. Then number of equivalence relations containing (1, 2) is:
(A) 1
(B) 2
(C) 3
(D) 4
A.17. 2, Therefore, option (B) is correct.
Q18. Let R → R be the Signum Function defined as and R → R be the Greatest Function given by g(x) = [x] where [x] is greatest integer less than or equal to x
Then, does fog and gof coincide in (0, 1)?
A.18. It is given that,
is defined as
Also, is defined as , where [x] is the greatest integer less than or equal to x.
Now, let
Then, we have:
if and if
Thus, when , we have
Hence, fog and gof do not coincide in (0, 1).
Therefore, option (B) is correct.
Q19. Number of binary operations on the set {a, b} are:
(A) 10
(b) 16
(C) 20
(D) 8
A.19.
A binary operation * on {a, b} is a function from {a, b} × {a, b} → {a, b}
i.e., * is a function from {(a, a), (a, b), (b, a), (b, b)} → {a, b}.
Hence, the total number of binary operations on the set {a, b} is 24 i.e., 16.
The correct answer is B.
Class 12 Math important reference books and preparation Tips
Candidates can check the suggestions of the most recommended books for competitive exam maths preparation. Candidates must choose the right resources and correct preparation plan to excel in both board and competitive exams such as JEE, COMEDK, etc... Candidates can check the preparation tips and book recommendations below;
Important Reference Books for JEE Main Maths Preparation
- RD Sharma Mathematics for Class 12 ( ( Vol.I and II)
- Objective Mathematics by R.D. Sharma (For JEE Mains)
- Cengage Mathematics Book Series by G. Tewani (For JEE Mains and Advance)
- Problems in Calculus of One Variable by I.A. Maron
- Trigonometry and Co-ordinate Geometry by SL Loney
Several other books are available in the market for JEE Main preparation for Maths. Candidates should discuss the options with their teachers or mentors before buying the books.
Preparation Tips for Class 12 Math and JEE Main
- Know Your Exam Well: Students should first check the exam pattern, including the level of questions asked, the time allotted to solve the paper, the format of the question paper (MCQs or descriptive), the required time for prep, resources, and other important things related to the exam. Knowing the exam thoroughly helps students make better and more realistic preparation strategies.
- Select Preparation Resources: Students should choose the right resources with the help of mentors or teachers to start their preparation. The Resources must match the level of exam targeted by the student, different levels of questions might hamper the prep speed and disturb the regular prep plan.
- Make a holistic preparation plan: Students should make a flexible and complete preparation plan which should include enough time for practice and revision of the already learned concepts.
- Practice and Attempt Sample/ Mock Papers: Practice and mock tests are key to scoring higher on any board or entrance exam. Students should take as many mock tests as possible during the preparation days, Mock tests boost the confidence and critical thinking skills of the student. In between the preparation, students can attempt the section and chapter-wise mock tests.
Maths Ncert Solutions class 12th Exam