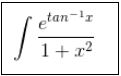- What is Integration by Substitution?
- Illustrated Examples on Integration by Substitution
- FAQs on Integration by Substitution
What is Integration by Substitution?
Integration is a method under calculus, opposite of differentiation, where we find the integrals of functions. Integration by substitution is one of the methods to solve integrals. This method is also called u-substitution.
The integration of a function f(x) is given by F(x), where F(x) is obtained by substitution. It is represented by:
∫f(x)dx = F(x) + C
The anti-derivatives of basic functions are known to us as integrals. But this integration technique is limited to basic functions and determines the integrals of various functions; so different integration methods are used. Among these methods of integration, the following is integration by substitution.
Here, any given integral is transformed into a simple form of integral by substituting others’ independent variable. The point to be kept in mind is that the substituted variable’s differentiation should also exist in the equation. Only then the equation is suitable to be solved using Integration by Substitution.
Example:
Integrate 2x/(1 + x2)
Let 2x = t, (1 + x2) = dt,
∫t.dt = t2/2 + C = (2x)2/2 + C = 4x2/2 + C = 2x2 + C
Details of the Topic
This topic is an essential part of the integration topic and is introduced in Class 12 to solve complex equations to obtain a simpler solution. Calculus problems carry 35 marks in board exams and give a deeper understanding of the integration and differentiation topic. Knowing the integrals are mandatory to solve the problems faster. Other methods of integration are essential, but this by far has maximum importance in exams. Students will also understand differentiation better in this topic, and they will learn to recognise when and where to apply this method on seeing the question.
Illustrated Examples on Integration by Substitution
1. Solve ∫sin(x3).3x2.dx with respect x
Solution. Substituting x3 = t --(2)
Differentiation of the above equation will give:
3x2.dx = dt -- (1)
Substituting the value of (2) in (1), we have
∫sin(x3).3x2.dx = ∫ sin t.dt
Thus the integration of the above equation will give
∫ sin t.dt = −cos t + c
Again putting back the value of t from equation (ii), we get
∫ sin(x3).3x2.dx = −cos(x3) + c
2.Integrate 2x.cos(x2−5) with respect to x.
Solution.
I = ∫2x cos (x2−5).dx
Let (x2–5) = t -- (1)
Hence, 2x.dx = dt
Substituting these values, we have
I = ∫cos(t). dt
= sin t + C --(2)
Substituting the value of (1) in (2), we have
I = sin (x2−5) + C
3. Find the integration of
Solution. Let t = tan-1x -- (1)
dt = (1/ 1+x2 ) . dx
I = ∫ et . dt
= et + C --(2)
Substituting the value of (1) in (2), we have I = etan-1x + C.
FAQs on Integration by Substitution
Q: What is the general form of integration by substitution?
Q: What should be kept in mind while choosing a term for substitution?
Q: Why is integration by substitution done?
A: There will be lesser terms to integrate under the integration by substitution method; hence making it easier to solve stepwise.
Q: Explain the terms in the following equation: ∫ f(x) dx = F(x) + C
Q: What are the different methods of integration?
Maths Integrals Exam
Student Forum
Popular Courses After 12th
Exams accepted
CA FoundationExams accepted
ICSI ExamExams accepted
BHU UET | GLAET | GD Goenka TestBachelor of Business Administration & Bachelor of Law
Exams accepted
CLAT | LSAT India | AIBEExams accepted
IPMAT | NMIMS - NPAT | SET
Exams accepted
BHU UET | KUK Entrance Exam | JMI Entrance ExamBachelor of Design in Animation (BDes)
Exams accepted
UCEED | NIFT Entrance Exam | NID Entrance ExamBA LLB (Bachelor of Arts + Bachelor of Laws)
Exams accepted
CLAT | AILET | LSAT IndiaBachelor of Journalism & Mass Communication (BJMC)
Exams accepted
LUACMAT | SRMHCAT | GD Goenka Test