Eccentricity: Overview, Questions, Preparation

Eccentricity is a concept in the conic section. It can be defined as how much deviation has occurred for any shape from the uniform circularity around a point, given the nature of the shape. There are a few different types of conic sections in geometry. These may be a parabola, hyperbola, ellipse, and circle. 

Eccentricity of Conic Sections

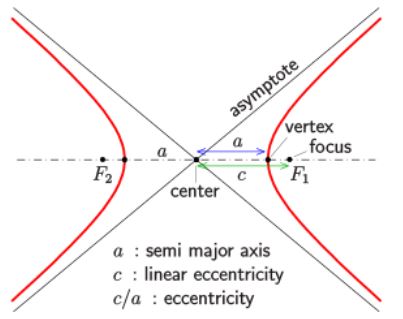
The eccentricity can be defined as the distance between any point to its fixed-point or focus- divided by the perpendicular distance from the focus to its closest directrix. This value is constant for any conic section. 

Eccentricity, which is denoted by ‘e’ = c/a

Where,
c = distance from the focus to the centre 
a = distance from the vertex to the centre

The following are a few of the essential shapes and their eccentricity equations:

Eccentricity of Parabola: 

The general equation to find out the eccentricity of a parabola is given by the equation x² = 4ay. And, the eccentricity of a parabola is equal to 1. 

Eccentricity of Ellipse: 

The general equation of an ellipse is given by the formula x²/a² +y²/b² =1. And, the eccentricity of an ellipse is less than 1. Thus, the eccentricity formula of an ellipse is given by the general equation √(1-b²/a²).

In the above equations, ‘a’ and ‘b’ are called the semi-major axis and semi-minor axis lengths, respectively.

Eccentricity of Hyperbola: 

The general equation of a hyperbola is given by the formula x²/a² - y²/b² = 1. And, the eccentricity of a hyperbola is greater than 1. Thus, the eccentricity formula for a hyperbola is given by the general equation √(1+ b²/a²).

In the above equations, ‘a’ and ‘b’ are called the semi-major and semi-minor axes’ lengths respectively.

This topic is taught in classes 11 and 12 according to the prescribed NCERT norms. It is also important for competitive exams. A student can expect 1 2-3 mark questions and one 6 mark questions from this topic.

1. Find the eccentricity of the ellipse for the given equation 16x² + 25y² = 400
Solution: It is given that:
16x² + 25y² = 400

The general equation for any ellipse:
x²/a²+y²/b²= 1

So to convert the given information to its general form we get: 16x²/400 + 25y²/400 =1

Solving this we get a=5 and b=4.

To find the eccentricity, we need to substitute the values of a, b, and simplify the form.

The general form: 
 √(1-b²/a²).

After solving the above form, we will get that the e=⅗ for this ellipse.

Q: What is eccentricity in mathematics?

Q: How to find the eccentricity of a parabola?

Q: What are the different types of conics?

Q: What is an ellipse?

Q: What is the eccentricity of a circle?