Cos Meaning: Overview, Questions, Preparation

The cos, in mathematics, refers to the cosine function, which is the very basic part of trigonometry. There are three main functions of trigonometry, which are: sine, cosine and tangent. Cos is one of the main functions of trigonometry. It is defined as the ratio between the lengths of sides and the hypotenuse. Cosine helps in finding the length and angles of various triangles.

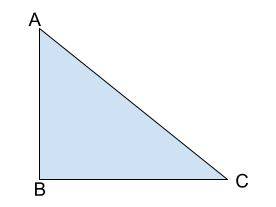
Suppose we have a triangle named ABC.

For angle C in the given triangle ABC, the formula of cos is:

cos C = base / hypotenuse = BC / AC

(Here, AC is the hypotenuse, AB is the perpendicular, and BC is the base.)

Similarly, for angle A in triangle ABC, cos will be written as:

cos A = base/hypotenuse = AB/AC

(Here, BC acts as the perpendicular, AB as the base, and AC as the hypotenuse)

Therefore, the general formula of cos is:

cosӨ = adjacent side to angle Ө/hypotenuse

We can derive many values of cos. But some of the basic values that are required to solve questions are 0°, 30°, 45°, 60°, 90°, etc. These help us find angles and solve the question further. You can easily learn these values as given below:

cos 0° = 1

cos 30° = 3/ 2 

cos 45° = 1/2

cos 60° = 1/2

cos 90° = 0

cos 120° = -1/2

cos 150° = -3/ 2

cos 180° = -1

cos 270° = 0

cos 360° = 1

Identities are essential when it comes to solving trigonometric equations. Some basic and important identities of cos are as follows:

cos2Ө + sin2Ө = 1

cos Ө  = 1/sec Ө

cos (−Ө) = cos (Ө)

arccos ( cos ( Ө ) ) = Ө + 2kӨ  (where k is an integer)

Cos (2Ө) =  cos2 (Ө) − sin2 (Ө)

cos (Ө) = sin (π/2 − Ө)

This chapter holds the basic knowledge of the concepts of trigonometry. It is important to learn this chapter with attention as the level of difficulty increases in classes 11 and 12. The chapter carries 12 marks in your class 10 board exams.

1. In triangle OPQ, right-angled at P, OP = 7 cm and OQ – PQ = 1 cm (see Fig.). Determine the values of sin Q and cos Q.

source:ncert

Solution: In triangle OPQ,

OQ2 =OP2 +PQ2

(1 + PQ)2 = OP2 +PQ2

1 +PQ2 +2PQ=OP2 +PQ2

1+2PQ =  7*7

2PQ = 48

PQ = 24

Therefore, OQ = 1 + PQ 

OQ = 1 +24= 25

Thus, sin Q = OP/OQ

Sin Q= 7/25 

And cos Q = PQ/OQ

Cos Q= 24/25

2. Express the ratios cos A, tan A and sec A in terms of sin A.

Solution: We know that cos2A+ sin2A= 1

cos2A= 1 -sin2A

cosA= 1 -sin2A

tan A = sin A/cos A

tan A = sin A/1 -sin2A

sec A = 1/cos A = 1 /1 -sin2A

3. If sin 3A = cos (A – 26°), where 3A is an acute angle, find the value of A.

Solution : Since sin 3A = cos (90° - 3A) 

Therefore, cos ( 90° - 3A) = cos (A - 26°)

90° - 3A = A - 26°

90° + 26° = A + 3A

4A = 116

A = 116/4

A = 29°

Q: What is the unit of cos?

A: Cos is a ratio and ratios do not have any unit.

Q: What is the reciprocal of cos?

A: The reciprocal relation of cos is written as cos Ө = 1/sec Ө

Q: State all the types of trigonometric functions.

A: sin = opposite/hypotenuse 

cos = adjacent/hypotenuse 

tan = opposite/adjacent 

cot = adjacent/opposite 

cosec = hypotenuse/opposite 

sec = hypotenuse/adjacent

Q: Write tan in terms of cos.

A: tan Ө = sin Ө/cos Ө

Q: What is the maximum possible value of cos?

A: 1