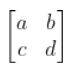- What is Matrices?
- Weightage of the topic
- Illustrative examples on Matrices and Determinants
- FAQs on Matrices and Determinants
What is Matrices?
An orderly arrangement of numbers or functions in a rectangular form is defined as a matrix. A matrix is denoted by capital letters. The numbers or functions are called elements and are distinguished by its position in rows and columns. The elements are represented by aij, where ‘i’ denotes the corresponding row, and ‘j’ denotes the corresponding column.
Let A be a matrix, A=
In the above example above, a11=1, a12= 2, a13=3,a21=4 and so on.
Order of the matrix is denoted as m n, where m is the number of rows and n is the number of columns a matrix has. The number of rows multiplied by the number of columns gives the number of elements, i.e., number of elements=m x n. In the above example above, an order of the matrix = 3x3 and nNumber of elements=3x3=9.
Properties ( when A and B are two matrices)
- AB ≠ BA
- (AB)C = A(BC)
- A.(B+C) = A.B + A.C
Determinants
For every square matrix, a number can be computed (real/complex), called the particular matrix’s determinant.
For instance, consider a matrix of order 2, A=
For a matrix of order 3, a determinant can be calculated by expanding a determinant along a row or column. Hence, there are six ways to compute a determinant of order 3, along the three rows or [A] along with the three columns. Let us understand it better using an example.
Properties
- The values of a determinant remain unchanged even after interchanging its rows and columns.
- The sign of a determinant changes if any two rows or columns are interchanged.
- The value of a determinant in which any two rows are identical is zero.
- If each element of a row or column is multiplied by a constant, then the value gets multiplied by the same constant.
- If some or all elements of a row or column are expressed as the sum of two or more items, then the determinant can be defined as the sum of two or more determinants.
- If equimultiples of corresponding elements are added to each element of a row or column, then the determinant’s value remains the same.
Weightage of the topic
The fundamentals of matrix and determinants are introduced to students in grade 12. The CBSE Board gives a weightage of 12 marks for the full unit of Algebra, under which these topics are discussed.
Explore exams which ask questions on Maths Matrices and Determinants
Select your preferred stream
Illustrative examples on Matrices and Determinants
1. In the matrix A=
Solution:
The order of the matrix
The elements a13, a21, a33, a24, a23
The number of rows is 3, and the number of columns is 4. Order= 34.
a13=19,
a21=35,
a33= - 5,
a24=12,
a23= 5/2.
2. Evaluate the determinant
Solution:
= 2x(-1) - (-5) x 4
= - 2 + 20= 18.
3. Evaluate the determinant
Solution:
= (cosθ x cosθ) - (sinθ x - sinθ)
= cos2θ + sin2θ
=1
FAQs on Matrices and Determinants
Q: What are Name the different types of matrices?.
Q: When are two matrices equal?
Q: Does every matrix have its corresponding determinant?
Q: What is a determinant of the matrix of order 1?
Q: What is the determinant’s value if all the row or column elements are zeroes?
- Column matrix
- Row matrix
- Square matrix
- Diagonal matrix
- Scalar matrix
- Identity matrix
- Zero matrix
Maths Matrices and Determinants Exam
Student Forum
Popular Courses After 12th
Exams accepted
CA FoundationExams accepted
ICSI ExamExams accepted
BHU UET | GLAET | GD Goenka TestBachelor of Business Administration & Bachelor of Law
Exams accepted
CLAT | LSAT India | AIBEExams accepted
IPMAT | NMIMS - NPAT | SET
Exams accepted
BHU UET | KUK Entrance Exam | JMI Entrance ExamBachelor of Design in Animation (BDes)
Exams accepted
UCEED | NIFT Entrance Exam | NID Entrance ExamBA LLB (Bachelor of Arts + Bachelor of Laws)
Exams accepted
CLAT | AILET | LSAT IndiaBachelor of Journalism & Mass Communication (BJMC)
Exams accepted
LUACMAT | SRMHCAT | GD Goenka Test