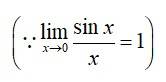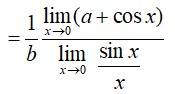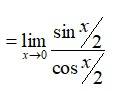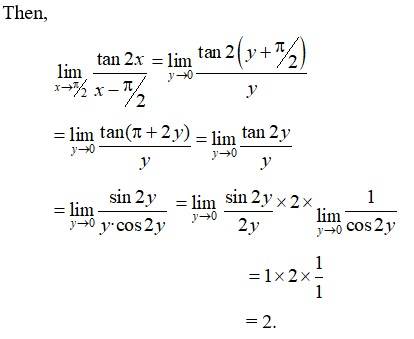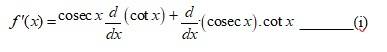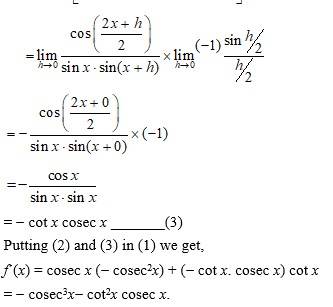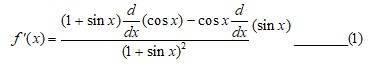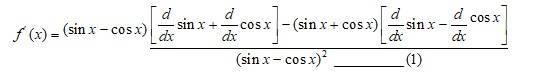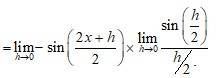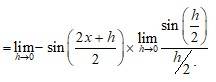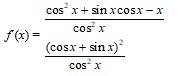NCERT Solutions Maths Class 11 Chapter 12 Limits and Derivatives: Topics, Questions, Preparation

Ex 13.1

Q1.  $\underset{x\to 3}{lim}$ x+ 3

A.1.  $\underset{x\to 3}{lim}$ x + 3 = 3 + 3 = 6.

 

Q2.  $\underset{x\to \pi }{\lim }\left(x-\frac{22}{7}\right)$

A.2.  $\underset{x\to \pi }{lim}\left(x-\frac{22}{7}\right)=\pi -\frac{22}{7}$

 

Q3.  $\underset{r\to 1}{\lim }\pi r^2$

A.3.  $\underset{r\to 1}{lim}\pi r^2=\pi ·{(1)}^2=\pi$

 

Q4.  $\underset{x\to 4}{\lim }\frac{4x+3}{x-2}$

A4.  $\underset{x\to 4}{lim}\frac{4x+3}{x-2}=\frac{4\times 4+3}{4-2}=\frac{16+3}{2}=\frac{19}{2}$

 

Q5.  $\underset{x\to -1}{\lim }\frac{x^{10}+x^5+1}{x-1}$

A.5.  $\underset{x\to -1}{lim}\frac{x^{10}+x^5+1}{x-1}=\frac{{(-1)}^{10}+{(-1)}^5+1}{(-1)-1}=\frac{1-1+1}{-2}=-\frac{1}{2}$

 

Q6.  $\underset{x\to 0}{\lim }\frac{{\left(x+1\right)}^5-1}{x}$

A.6.  $\underset{x\to 0}{lim}\frac{{(x+1)}^5-1}{x}=\underset{x\to 0}{lim}\frac{x^5+5x^4+10x^3+10x^2+5x+1-1}{x}$

$=\underset{x\to 0}{lim}{x}^4+5x^3+10x^2+10x+5.$

(0)4 + 5(0)3 + 10(0)2 + 10(0) + 5

= 5.

 

Q7.  $\underset{x\to 2}{\lim }\frac{3x^2-x-10}{x^2-4}$

A.7.

$=\underset{x\to 2}{lim}\frac{x(3x+5)-2(3x+5)}{(x-2)(x+2)}$

$=\underset{x\to 2}{lim}\frac{(x-2)(3x+5)}{(x-2)(x+2)}$

$=\underset{x\to 2}{lim}\frac{3x+5}{x+2}$

$=\frac{3\times 2+5}{2+2}$

$=\frac{6+5}{4}$

$=\frac{11}{4}.$

 

Q8.  $\underset{x\to 3}{\lim }\frac{x^4-81}{2x^2-5x-3}$

 

$=\underset{x\to 3}{lim}\frac{(x+3)(x^2+9)}{2x+1.}$

$=\frac{(3+3)\left(3^2+9\right)}{2\times 3+1}$

$=\frac{6\times 18}{6+1}$

$=\frac{108}{7}$

 

 

Q.10  $\underset{z\to 1}{\lim }\frac{z^{\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$3$}\right.}-1}{z^{\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$6$}\right.}-1}$

A.10. 

$=\underset{z\to 1}{lim}\left[\frac{z^{\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$3$}\right.}-1^{\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$3$}\right.}}{z-1}\right]÷\underset{z\to 1}{lim}\left[\frac{z^{\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$6$}\right.}-1^{\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$6$}\right.}}{z-1}\right]$

We know that,

$\underset{}{}$ $\underset{x\to a}{lim}\frac{x^n-a^n}{x-a}=n{a}^{n-1}$

So,

$=\frac{1}{3}\times 6=2$

 

Q11.  $\underset{x\to 1}{\lim }\frac{a{x}^2+bx+c}{c{x}^2+bx+a}=a+b+c \ne 0$

A.11.  $\underset{x\to 1}{lim}\frac{a{x}^2+bx+c}{c{x}^2+bx+a}=\frac{a+b+c}{c+b+a}=1$

 

Q12.  $\underset{x\to -2}{\lim }\frac{\frac{1}{x}+\frac{1}{2}}{x+2}$

A.12.

$=\frac{1}{2\times (-2)}=-\frac{1}{4}.$

 

Q13.  $\underset{x\to 0}{\lim }\frac{ax}{bx}$

A.13.  $\underset{x\to 0}{lim}\frac{sinax}{bx}=\frac{1}{b}\underset{x\to 0}{lim}\frac{sinax}{ax}\times a$

$=\frac{a}{b}\underset{x\to 0}{lim}\frac{sinax}{ax}$ 

$=\frac{a}{b}$

 

Q14.  $\underset{x\to 0}{lim}\frac{sinax}{sinbx},a,b\ne 0$

A.14.  $\underset{x\to 0}{lim}\frac{sinax}{sinbx}=\underset{x\to 0}{lim}\frac{\frac{sinax}{ax}\times ax}{\frac{sinbx}{bx}\times bx}$

$=\frac{a}{b}$

 

Q15.  $\underset{x\to \pi }{lim}\frac{sin(\pi -x)}{\pi (\pi -x)}$

A15.  $\underset{x\to \pi }{lim}\frac{sin(\pi -x)}{\pi (\pi -x)}=\frac{1}{\pi }\times \underset{x\to \pi }{lim}\frac{sin(\pi -x)}{\pi -x}$

$=\frac{1}{\pi }.$

 

Q16.  $\underset{x\to 0}{lim}\frac{cosx}{\pi -x}$

A.16.  $\underset{x\to 0}{lim}\frac{cosx}{\pi -x}=\frac{cos0}{\pi -0}=\frac{1}{\pi }$

 

Q17.

 

$=\underset{x\to 0}{lim}\frac{2si{n}^{2}x}{2si{n}^2\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}$

$=\frac{\underset{x\to 0}{lim}{\left(\frac{sinx}{x}\right)}^2\times x^2}{\underset{x\to 0}{lim}{\left(\frac{si{n}^{}\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}{\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}\right)}^2{\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}^2}$

$=\frac{{(1)}^2\times x^2\times 4}{{(1)}^2\times x^2}$

= 4

 

Q18.  $\underset{x\to 0}{lim}\frac{ax+xcosx}{bsinx}$

A18.  $\underset{x\to 0}{lim}\frac{ax+xcosx}{bsinx}=\underset{x\to 0}{lim}\frac{x(a+cosx)}{bsinx}$

$=\frac{1}{b}\times \frac{(a+cos0)}{1}$

$=\frac{a+1}{b}$

 

Q19.  $\underset{x\to 0}{lim}xsecx$

A.19.  $\underset{x\to 0}{lim}xsecx=\underset{x\to 0}{lim}\frac{x}{cosx}$

$=\frac{0}{cos0}$

$=\frac{0}{1}$

= 0.

 

Q20.  $\underset{x\to 0}{lim}\frac{sinax+bx}{ax+sinbx}a,b,a+b\ne 0$

A.20.  $\underset{x\to 0}{lim}\frac{sinax+bx}{ax+sinbx}=\frac{\underset{x\to 0}{lim}\frac{sinax}{ax}\times ax+bx}{\underset{x\to 0}{lim}ax+\frac{sinbx}{bx}\times bx}.$

$\frac{\underset{x\to 0}{lim}\frac{sinax}{ax}\times \underset{x\to 0}{lim}ax+\underset{x\to 0}{lim}bx}{\underset{x\to 0}{lim}ax+\underset{x\to 0}{lim}\frac{sinbx}{bx}\times \underset{x\to 0}{lim}bx}$

$=\frac{\underset{x\to 0}{lim}ax+\underset{x\to 0}{lim}bx}{\underset{x\to 0}{lim}ax+\underset{x\to 0}{lim}bx}$

$=\underset{x\to 0}{lim}\frac{ax+bx}{ax+bx}$

$=\underset{x\to 0}{lim}1$

= 1.

 

Q21. $\underset{x\to 0}{lim}(cosecx-cotx)$

A.21.  $\underset{x\to 0}{lim}(cosecx-cotx)=\underset{x\to 0}{lim}\left(\frac{1}{sinx}-\frac{cosx}{sinx}\right)$

$=\underset{x\to 0}{lim}\left(\frac{1-cosx}{sinx}\right)$

$=\underset{x\to 0}{lim}\frac{2si{n^2}^{\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}}{2si{n}^{\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}co{s}^{\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}}$  $\{\begin{array}{}\end{array}\because cos2x=1-2si{n}^{2}x\Rightarrow 1-cos2x=2si{n}^{2}x1-cosx=2si{n}^2\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\because sin2x=2sinxcosxsinx=2sin\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.cos\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\{$

$=\underset{x\to 0}{lim}\raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.$

$=tan\raisebox{1ex}{$0$}\!\left/ \!\raisebox{-1ex}{$2$}\right.=0.$

 

Q.22.  $\underset{x\to \frac{\pi }{2}}{lim}\frac{tan2x}{x-\frac{\pi }{2}}$

A.22.  $\underset{x\to \raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}{lim}\frac{tan2x}{x-\raisebox{1ex}{$\pi $}\!\left/ \!\raisebox{-1ex}{$2$}\right.}$

Put y = x  $\raisebox{1ex}{$\pi $}\!\left/ \!\raisebox{-1ex}{$2$}\right.$ . So that as y 0 cos  $\raisebox{1ex}{$\pi $}\!\left/ \!\raisebox{-1ex}{$2$}\right.$

 

Q23. Find  $\underset{x\to 0}{lim}f(x)$ and  $\underset{x\to 1}{lim}f(x)$ , where f (x)  $\{\begin{array}{ll}2x+3,\hfill & x\le 0\hfill \\ 3(x+1),\hfill & x>0\hfill \end{array}\}$

A.23. Given f (x)  $\{\begin{array}{ll}2x+3,\hfill & x\le 0\hfill \\ 3(x+1),\hfill & x>0\hfill \end{array}\}$

for  $\underset{x\to 0}{lim}f(x),$

left hand limit, L.H.S =  $\underset{x\to 0^{}}{lim}f(x)$ =  $\underset{x\to 0^{}}{lim}(2x+3)$

= 2 0 + 3 = 3.

Right hand limit, R.H.L =  $\underset{x\to 0^{+}}{lim}f(x)=\underset{x\to 0^{+}}{lim}3(x+1)$

= (0 + 1) = 3 1 = 3.

Thus,  $\underset{x\to 0^{-}}{lim}f(x)=\underset{x\to 0^{+}}{lim}f(x)=\underset{x\to 0^{-}}{lim}f(x)=3$

For  $\underset{x\to 1}{lim}f(x),$

L.H.L =  $\underset{x\to 1^{-}}{lim}f(x)=\underset{x\to 1^{-}}{lim}3(x+1)=3(1+1)=3\times 2=6$

R.H.L =  $\underset{x\to 1^{+}}{lim}f(x)=\underset{x\to 1^{+}}{lim}3(x+1)=3(1+1)=3\times 2=6.$

Thus,  $\underset{x\to 1^{-}}{lim}f(x)=\underset{x\to 1^{+}}{lim}f(x)=\underset{x\to 1}{lim}f(x)=6.$

 

 

Q26.Find  $\underset{x\to 0}{lim}f(x),$ wheref (x)  $\{\begin{array}{ll}\frac{x}{\left|x\right|},\hfill & x\ne 0\hfill \\ 0,\hfill & x=0\hfill \end{array}$

A.26.Given, f (x)  $\{\begin{array}{ll}\frac{x}{\left|x\right|},\hfill & x\ne 0\hfill \\ 0,\hfill & x=0\hfill \end{array}$

 

L.H.S =  $\underset{x\to 0^{-}}{lim}f(x)=\underset{x\to 0^{-}}{lim}\frac{x}{-x}=\underset{x\to 0^{-}}{lim}-1=-1$

R.H.L  $\underset{x\to 0^{+}}{lim}f(x)=\underset{x\to 0^{+}}{lim}\frac{x}{x}=\underset{x\to 0^{+}}{lim}1=1$

Thus,  $\underset{x\to 0^{-}}{lim}f(x)\ne \underset{x\to 0^{+}}{lim}f(x)$

i e,  $\underset{x\to 0}{lim}f(x)$ does not exist.

 

Q27.Find  $\underset{x\to 5}{lim}f(x),where$ f (x)  $=\left|x\right|-5$

A27.  $\underset{x\to 5}{lim}f(x)=\underset{x\to 5}{lim}\left|x\right|-5$

= | 5 | $-\mathrm{}$ 5

= 5 $-\mathrm{}$5

= 0

Q28. Supposef (x) =  $\{\begin{array}{l}a+bx,x<1\\ 4,x=1\\ b-ax,x>1\end{array}$ and if  $\underset{x\to 1}{lim}f(x)$ = f (1) what are possible values of a and b?

A.28. Given, f (x) =  $\begin{array}{l}\{\begin{array}{l}a+bx,x<1\\ 4,x=1\end{array}\\ b-ax,x>1\end{array}$

Since we need  $\underset{x\to 1}{lim}f(x)$ we need,

LHL =

$\underset{x\to 1^{-}}{lim}f(x)=\underset{x\to 1^{-}}{lim}(a+bx)$ = a + b × 1 = a + b

and RHL =

$\underset{x\to 1^{+}}{lim}f(x)=\underset{x\to 1^{+}}{lim}(b-ax)$ = b - a × 1 = b - a

Given,  $\underset{x\to 1}{lim}f(x)=f(1):$ we have the following equations

a + b = 4 ____ (1)

b - a = 4 ____ (2)

Adding (1) and (2) we get,

2b = 8

b = 4

Putting b = 4 in (1) we get

a + 4 = 4

a = 0

 

Thus,  $\underset{x\to a^{-}}{lim}f(x)\ne \underset{x\to a^{+}}{lim}f(x)$

So,  $\underset{x\to a}{lim}f(x)$ does not exist at a = 0.

 

Q31. If the function f(x) satisfies  $\underset{x\to 1}{lim}\frac{f(x)-2}{x^2-1}=\pi$ , evaluate  $\underset{x\to 1}{lim}f(x).$

A.31. Given,  $\underset{x\to 1}{lim}\frac{f(x)-2}{x^2-1}=\pi$

$\frac{\underset{x\to 1}{lim}f(x)-2}{\underset{x\to 1}{lim}{x}^2-1}=\pi$

$\Rightarrow \underset{x\to 1^{}}{lim}[f(x)-2]=\pi \underset{x\to 1}{lim}\left(x^2-1\right)$

$\Rightarrow \underset{x\to 1}{lim}f(x)-\underset{x\to 1}{lim}2=\pi \left(1^2-1\right)$

$\Rightarrow \underset{x\to 1}{lim}f(x)-2=0.$

$\Rightarrow \underset{x\to 1}{lim}f(x)=2$

 

 

Ex 13.2.

Q1.Find the derivative

A.1. Given, f(x)=x²- 2 ., $f^{\prime }(10)=?$

We have, 

$f^{\prime }(10)=\underset{h\to 0}{lim}\frac{f(10+h)-f(10)}{h}$

$=\underset{h\to 0}{lim}\frac{\left[{(10+h)}^2-2\right]-\left[10^2-2\right]}{h}$

=  $\underset{h\to 0}{lim}\frac{10^2+h^2+20h-2-10^2+2}{h}$

=  $\underset{h\to 0}{lim}\frac{h(h+20)}{h}$

$\Rightarrow \underset{h\to 0}{lim}h+20$

=20

 

Q2.Find the derivative of x at x = 1.

A.2. Given, f(x)=x, f  (1)=?

We have,

$f^{\prime }(1)=\underset{h\to 0}{lim}\frac{f(1+h)-f(1)}{h}$

$$  $=\underset{h\to 0}{lim}\frac{1+h-1}{h}$

$=\underset{h\to 0}{lim}\frac{h}{h}$

$=\underset{h\to 0}{lim}1$

=1

 

Q3.Find the derivative of 99x at x = l00

A.3. Given, f(x)= 99x, f  (100)= ?

So, f(100)=  ${}_{h\to 0}\frac{f(100+h)-f(100)}{h}$

$=\underset{h\to 0}{lim}\frac{99(100+h)-99(100)}{h}$

$=\underset{h\to 0}{lim}\frac{99\times 100+99\times h-99\times 100}{h}$

$=\underset{h\to 0}{lim}\frac{99h}{h}$

$=\underset{h\to 0}{lim}99$

=99

 

Q4.Find the derivative of the following functions from first principle.

(i)  $x^3-27$ (ii) (x -1)(x-2)

(iii)  $\frac{1}{x^2}$ (iv)  $\frac{x+1}{x-1}$

A.4.(i) Given,  $f(x)=x^3-27.$

So,  $f^{\prime }(x)=\frac{\underset{h\to 0}{lim}f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}\frac{\left[{(x+h)}^3-27\right]-\left[x^3-27\right]}{h}$

$=\underset{h\to 0}{lim}\frac{x^3+h^3+3xh(x+h)-27-x^3+27}{h}$

$=\underset{h\to 0}{lim}\frac{h\left(h^2+3x(x+h)\right)}{h}$

$=\underset{h\to 0}{lim}{h}^2+3x(x+h)$

=0+3 x(x+ 0)

=3x²

(ii) Given, f(x) =(x-1)(x-2)

=x²- 3x+2

So,  $f^{\prime }(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$\underset{h\to 0}{lim}\frac{\left[{(x+h)}^2-3(x+h)+2\right]-\left[x^2-3x+2\right]}{h}$

=  $\underset{h\to 0}{lim}\frac{x^2+h^2+2xh-3x-3h+2-x^2+3x-2}{h}$

=  $\underset{h\to 0}{lim}\frac{h(h+2x-3)}{h}$

$=\underset{h\to 0}{lim}h+2x-3$

= 2x – 3.

(iii) Given, f(x)=  $\frac{1}{x^2}$

So,  $f^{\prime }(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}\frac{\frac{1}{{(x+h)}^2}-\frac{1}{x^2}}{h}$

$=\frac{\underset{h\to 0}{lim}\frac{x^2-{(x+h)}^2}{{(x+h)}^2-x^2}}{h}$

$=\underset{h\to 0}{lim}\frac{x^2-x^2-h^2-2xh}{h{x}^2(x+h){}^2}$  $$

$=\underset{h\to 0}{lim}\frac{h(-h-2x)}{h{x}^2(x+h){}^2}$

$=\underset{h\to 0}{lim}\frac{-h-2x}{x^2(x+h){}^2}$

$=\frac{-0-2x}{x^2(x+0){}^2}$

$=\frac{-2x}{x^4}$

$=\frac{-2}{x^3}$

(iv) Given, f(x)=  $\frac{x+1}{x-1}$

$f(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{fx+h+1}{x+h-1}-\frac{x+1}{x-1}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{(x+h+1)(x-1)-(x+1)(x+h-1)}{(x+h-1)(x-1)}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{x^2-x+hx-h+x-1-x^2-hx+x-x-h+1}{(x-1)(x+h-1)}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{-2h}{(x-1)(x+h-1)}\right]$

$=\underset{h\to 0}{lim}\frac{-2}{(x-1)(x+h-1)}$

$=\frac{-2}{(x-1)(x-1)}=\frac{-2}{{(x-1)}^2}$

 

Q5.For the function

$f(x)=\frac{x^{100}}{100}+\frac{x^{99}}{99}+\cdots +\frac{x^2}{2}+x+1$

Prove that  $f^{\prime }(1)=100f^{\prime }(0)$

A.5. Given $f(x)=\frac{x^{100}}{100}+\frac{x^{99}}{99}+\cdots +\frac{x^2}{2}+x+1$

$\underset{h\to 0}{lim}\frac{{(x+h)}^{100}-x^{100}}{100\cdot h}+\underset{h\to 0}{lim}\frac{{(x+h)}^{99}-x^{99}}{99\cdot h}+\dots +\underset{x\to 0}{lim}\frac{{(x+h)}^2-x^2}{2h}$  $+\underset{x\to 0}{lim}x+\underset{x\to 0}{lim}1$

$=\frac{100\cdot x^{99}}{100}+\frac{99x^{98}}{99}+\cdots +\frac{2x}{2}+0+1$

$=x^{99}+x^{98}+\cdots +x+1$

At x=0,

f(X)  =1.

and at x=1,

$f^{\prime }(1)=1^{99}+1^{98}+\dots +1^2+1+1$

=100  $\times$ 1

=100  $\times f^{\prime }(0)$

Hence,  $f^{\prime }(1)=100f^{\prime }(0)$

 

Q6.Find the derivative of  $x^n+a{x}^{n-1}+a^2{x}^{n-2}+\cdots +a^{n-1}x+a^n$ for some fixed real number a.

A.6. Given, $f(x)=x^n+a{x}^{n-1}+a^2{x}^{n-2}+\cdots +a^{n-1}x+a^n.$

We know that,

$\frac{d}{dx}\left(x^x\right)=n{x}^{x-1}$

So,

$f^{\prime }(x)=\frac{d}{dx}{x}^x+\frac{d}{dx}a{x}^{x-1}+\frac{d}{dx}{a}^2{x}^{x-2}+\cdots +\frac{d}{dx}$  $\begin{array}{l}{a}^{x-1}x+\frac{d}{dx}{a}^x=n{x}^{n-1}+a(n-1)x^{n-2}+a^2(n-2)x^{n-3}+\cdots +a^{n-1}+0.\\ <br>\end{array}$

$\begin{array}{l}(\because \frac{dax}{dx}=a\frac{dx}{dx}\frac{\mathrm{and\; d}a}{dx}=\mathrm{0whereaisconstant}\end{array}$ $\begin{array}{l}\end{array}$ $\begin{array}{l}\mathrm{<br>}\end{array}$

 

Q7. For some constants aand b, find the derivative of

(i)(x- a) + (x - b)(ii)  ${\left(a{x}^2+b\right)}^2$ (iii)  $\frac{x-9}{x-b}$

A.7. (c) Given, f(x)=(x-a)(x-b)

where a and b are constants.

So,

$f^{\prime }(x)=(x-a)\frac{d}{dx}(x-b)+(x-b)\frac{d}{dx}(x-a)$

=(x-a)+(x-b)

= 2x– a- b.  $$

(ii) Given f(x)=  ${\left(a{x}^2+b\right)}^2.$ where ab are constant

So,  $f^{\prime }(x)=\frac{d}{dx}{\left(a{x}^2+b\right)}^2$

$=\frac{d}{dx}\left(a^2{x}^4+b^2+2a{x}^{2}b\right)$

$=\frac{d}{dx}{a}^2{x}^4+\frac{d}{dx}{b}^2+\frac{d}{dx}2a{x}^{2}b$

=  $4a^2{x}^3+0+4axb$

=  $4ax\left(a{x}^2+b\right).$

(iii) Given, f(x)=  $\frac{x-9}{x-b}$ where a and bare constants

So,  $f(x)=\frac{(x-b)\frac{d}{dx}(x-a)-(x-a)\frac{d}{dx}(x-b)}{{(x-b)}^2}$

$=\frac{(x-b)-(x-a)}{{(x-b)}^2}$

$=\frac{a-b}{{(x-b)}^2}$

 

Q8. Find the derivative of  $\frac{x^n-a^n}{x-a}$ for some constant a.

A.8. Given, f(x)=  $\frac{x^n-a^n}{x-a}.$

So,  $f^{\prime }(x)=\frac{(x-a)\frac{d}{dx}\left(x^n-a^3\right)-\frac{d}{dx}(x-a)\cdot \left(x^n-a^n\right)}{{(x-a)}^2}=\frac{(x-a)\cdot n{x}^{n-1}-\left(x^n-a^n\right)}{{(x-a)}^2}$

$=\frac{(x-a)\cdot n{x}^{n-1}-\left(x^n-a^n\right)}{{(x-a)}^2}$

$=\frac{n{x}^{n-1}\cdot x-na{x}^{n-1}-x^n+a^n}{{(x-a)}^2}$

$=\frac{n{x}^n-x^x-na{x}^{n-1}+a^n}{{(x-a)}^2}$

 

Q9. Find the derivative of

(i)  $2x-\frac{3}{4}$ (ii)  $\left(5x^3+3x-1\right)(x-1)$

(iii)  $x^{-3}(5+3x)$ (iv)  $x^5\left(3-6x^{-9}\right)$

(v)  $x^{-4}\left(3-4x^{-5}\right)$ (vi)  $\frac{2}{x+1}-\frac{x^2}{3x-1}$

A.9. (i)  $f(x)=2x-\frac{3}{4}$

$f^{\prime }(x)=\frac{d}{dx}\left(2x-\frac{3}{4}\right)$

$=2\frac{dx}{dx}-0$

=2.

(ii) Given, f(x)=  $\left(5x^3+3x-1\right)(x-1)$

So,  $f(x)=\left(5x^3+3x-1\right)\frac{d}{dx}(x-1)+(x-1)\frac{d}{dx}\left(5x^3+3x-1\right)$

$=\left(5x^3+3x-1\right).1+(x-1)\left(15x^2+3\right)$

=  $5x^3+3x-1+i5x^3+3x-15x^2-3$

$=20x^3-15x^2+6x-4.$

(iii) Given, f(x) =  $x^{-3}(5+3x)$

So,  $\begin{array}{l}{f}^{\prime }(x)=x^{-3}\frac{d}{dx}(5+3x)+(5+3x)\frac{d{x}^{-3}}{dx}\\ =x^{-3}\cdot 3+(5+3x)\cdot (-3)x^{-4}\end{array}$

$=\frac{3}{x^3}-\frac{15}{x^4}-\frac{9}{x^3}$

$=\frac{-15}{x^4}-\frac{6}{x^3}$

$=-\frac{3}{x^4}(5+2x).$

(iv) Given, f(x)=  $x^5\left(3-6x^{-9}\right).$

$f^{\prime }(x)=x^5\frac{d}{dx}\left(3-6x^{-9}\right)+\left(3-6x^{-9}\right)\frac{d}{dx}{x}^5.$

$=x^5\left(-6x-9x^{-10}\right)+\left(3-6x^{-9}\right)\cdot 5x^4$

=  $x^5\left(54x^{-10}\right)+15x^4-30x^{-5}$

$=54x^{-5}-30x^{-5}+15x^4$

$=\frac{24}{x^5}+15x^4$

(v) Given, f(x)=  $x^{-4}\left(3-4x^{-5}\right).$

So,  $f^{\prime }(x)=x^{-4}\cdot \frac{d}{dx}\left(3-4x^{-5}\right)+\left(3-4x^{-5}\right)\frac{d}{dx}{x}^{-4}$

$=x^{-4}\cdot \left(-4x-5\times x^{-6}\right)+\left(3-4x^{-5}\right)\cdot \left(-4x^{-5}\right)$

$=20x^{-10}-12x^{-5}+16x^{-10}.$

$=36x^{-10}-12x^{-5}.$

$=\frac{36}{x^{10}}-\frac{12}{x^5}.$

(vi) Given, f(x)=  $\frac{2}{x+1}-\frac{x^2}{3x-1}$

So,  $f^{\prime }(x)=\frac{d}{dx}\left(\frac{2}{x+1}\right)-\frac{d}{dx}\left(\frac{x^2}{3x-1}\right)$

$=\frac{(x+1)\frac{d}{dx}-2\frac{d}{dx}(x+1)}{{(x+1)}^2}-\frac{(3x-1)\frac{d{x}^2}{dx}-x^2\frac{d}{dx}(3x-1)}{{(3x-1)}^2}$

$=\frac{-2}{{(x+1)}^2}-\frac{2x(3x-1)-3x^2}{{(3x-1)}^2}$

$=-\frac{2}{{(x+1)}^2}-\frac{6x^2-2x-3x^2}{{(3x-1)}^2}$

$=-\frac{2}{{(x+1)}^2}-\frac{3x^2-2x}{{(3x-1)}^2}$

$=-\frac{2}{{(x+1)}^2}-\frac{x(3x-2)}{{(3x-1)}^2}$

 

Q10. Find the derivative of cos x from first principle.

A.10. Given, f(x) = cos x

$f(x+h)=cos(x+h)$

By first principle,

$f^{\prime }(x)=\underset{x\to h}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{x\to h}{lim}\frac{1}{h}[cos(x+h)-cosx]$

$=\underset{x\to h}{lim}\frac{1}{h}{\left[-2sin\left(\frac{x+h+x}{2}\right)sin\left(\frac{x+h-x}{2}\right)\right]}^{\prime }$

$=\underset{x\to h}{lim}\frac{1}{h}\left[-2sin\left(\frac{2x+h}{2}\right)sin\left(\frac{h}{2}\right)\right]$

$=\underset{x\to h}{lim}\frac{1}{h}\left[-2sin\left(\frac{2x+h}{2}\right)sin\left(\frac{h}{2}\right)\right]$

$=\underset{x\to h}{lim}-sin\left(\frac{2x+h}{2}\right)\times \underset{x\to h}{lim}\frac{sinh/2}{h/2}$

$=-sin\left(\frac{2x+0}{2}\right)\times 1=-sinx.$

 

Q11.Find the derivative of the following functions:

(i)sin x cos x  $$ (ii) $secx$ (iii)5 sec x + 4 cosx

$$(iv) $cosecx$ (v)3cot x + 5 cosec x

(vi)  $5sinx-6cosx+7$ (vii)  $2tanx-7secx$

A.11. (i) f(x)=sin x cos x

So,  $f^{\prime }(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}\frac{sin(x+h)cos(x+h)-sinxcosx}{h}$

$=\underset{h\to 0}{lim}\frac{1}{2h}\times [2sin(x+h)cos(x+h)-2sinxcosx]$

$=\underset{h\to 0}{lim}\frac{1}{2h}[sin2(x+h)-sin2x]$

$=\underset{h\to 0}{lim}\frac{1}{2h}\left[2cos\frac{2(x+h)+2x}{2}sin\frac{2(x+h)-2x}{2}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[cos\left(2x+h\right)sinh\right]$

$=\underset{h\to 0}{lim}cos(2x+h)\times \underset{h\to 0}{lim}\frac{sinh}{h}$

$=cos(2x+0)$

$=cos2x$

$\mathrm{(ii)\; f}(x)=secx$

So,  $f^{\prime }(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}[sec(x+h)-secx]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{1}{cos(x+h)}-\frac{1}{cosx}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{cosx-cos(x+h)}{cos(x+h)cosx}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{-2sin\left(\frac{x+x+h}{2}\right)sin\left(\frac{x-(x+h)}{2}\right)}{cos(x+h)cosx}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[-\frac{2sin\left(\frac{2x+h}{2}\right)sin(-h/2)}{cos(x+h)cosx}\right]$

$=\underset{h\to 0}{lim}\frac{(-1sin\left(\frac{2x+h}{2}\right)}{cos(x+h)cosx}\times \underset{h\to 0}{lim}(-1)\frac{sinh/2}{h/2}$

$=\frac{sinx}{cosx\cdot cosx}\times 1$

$=tanx\cdot secx.$

(iii) Given f(x)=5 sec x+4 cosx.

So,  $f^{\prime }(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$\begin{array}{l}\\ =\underset{h\to 0}{lim}\frac{5sec(x+h)+4cos(x+h)-[5secx+4cosx]}{h}.\end{array}$

$=\underset{h\to 0}{lim}\frac{5}{h}[sec(x+h)-secx]+\underset{h\to 0}{lim}\frac{4}{h}[cos(x+h)-cosx]$

$=\underset{h\to 0}{lim}\frac{5}{h}\left[\frac{1}{cos(x+h)}-\frac{1}{cosx}\right]+\underset{h\to 0}{lim}\frac{4}{h}\left[-2sin\left(\frac{x+h+x}{2}\right)sin\left(\frac{x+h-x}{2}\right)\right]$

$=\underset{h\to 0}{lim}\frac{5}{h}\left[\frac{cosx-cos(x+h)}{cos(x+h)(cosx)}\right]+\underset{h\to 0}{lim}\frac{4}{h}\left[-2sin\left(\frac{2x+h}{2}\right)sin\frac{h}{2}\right]$

$=\underset{h\to 0}{lim}\frac{5}{h}\left[-\frac{2sin\left(\frac{2x+h}{2}\right)sin(-h/2)}{cos(x+h)cosx}\right]-4\underset{h\to 0}{lim}sin\left(\frac{2x+h}{2}\right)\underset{h\to 0}{lim}\frac{sinh/2}{h/2}$

$=\frac{sin\left(\frac{2x+0}{2}\right)}{cos(x+0)cosx}\times 1-4sin\left(\frac{2x}{2}\right)$

$=5\frac{sinx}{cosx}\cdot \frac{1}{cosx}-4sinx$

$=5tanx\cdot secx-4sinx$

$\mathrm{(iv)\; Given\; f}(x)=cosecx$

$f^{\prime }(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}[cosec(x+h)-cosecx]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{1}{sin(x+h)}-\frac{1}{sinx}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{sinx-sin(x+h)}{sin(x+h)sinx}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{2cos\left(\frac{x+x+h}{2}\right)sin\left(\frac{x-(x+h)}{2}\right)]}{sin(x+h)sinx}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{2cos\left(\frac{x+x+h}{2}\right)sin\left(\frac{x-(x+h)}{2}\right)}{sin(x+h)sinx}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{2cos\left(\frac{2x+h}{2}\right)sin(-h/2)]}{sin(x+h)sinx}\right]$

$=\underset{h\to 0}{lim}\frac{cos\left(\frac{2x+h}{2}\right)}{sin(x+h)sinx}\times \frac{(-1)sin(2)}{h/2})$

$=\frac{cos\left(\frac{2x+0}{2}\right)}{sin(x+0)sinx}\times (-1)$

$=-\frac{cosx}{sinx}\times \frac{1}{sinx}$

$=-cotx\cdot cosecx$

(v) Given,f(x)=3 cot x+5cosecx.

So,  $f^{\prime }(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$  $=\frac{2}{cos(x+0)cosx}\times 1+7sin\left(\frac{2x+0}{2}\right)\times (-1)$

$=\underset{h\to 0}{lim}3cot(x+h)+5cosec(x+h)-[3cotx+5cosx)$

${}_{h\to 0}\frac{3}{h}[cot(x+h)-cotx]+\underset{h\to 0}{lim}$

$=\frac{5}{h}[cosec(x+h)-cosecx]$

$=\underset{h\to 0}{lim}\frac{3}{h}\left[\frac{cos(x+h)}{sin(x+h)}-\frac{cosx}{sinx}\right]+\underset{h\to 0}{lim}\frac{5}{h}\left[\frac{1}{sin(x+h)}-\frac{1}{sinx}\right]$

$=\underset{h\to 0}{lim}\frac{3}{h}\left[\frac{cos(x+h)sinx-cosxsin(x+h)}{sin(x+h)sinx}\right]+\underset{h\to 0}{lim}\frac{5}{h}\left[\frac{sinx-sin(x+h)}{sin(x+h)sinx}\right]$

$=\underset{h\to 0}{lim}\frac{3}{h}[\frac{sin(x-(x+h))}{sin(x+h)sinx}+\underset{h\to 0}{lim}\frac{5}{h}[\frac{2cos\left(\frac{x+x+h}{2}\right)sin\left(\frac{x-(x+h)}{2}\right)}{sin(x+h)sinx}$

$=\underset{h\to 0}{lim}\frac{3}{sin(x+h)sinx}\times \underset{h\to 0}{lim}(-1)\frac{sinh}{h}+\underset{h\to 0}{lim}\frac{5}{h}[\frac{2cos\left(\frac{2x+h}{2}\right)sin(-h/2)}{sin(x+h)sinx}$

$=\frac{3}{sinx\cdot sinx}\times (-1)+5\underset{h\to 0}{lim}\frac{cos\left(\frac{2x+h}{2}\right)}{sin(x+h)sinx}\times (-1)\underset{h\to 0}{lim}\frac{sinh/2}{h/2}.$

$=-3cose{c}^{2}x-5cotx\cdot cosecx.$

(vi) Given,  $f(x)=5sinx-6cosx+7$

$f^{\prime }(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}[5sin(x+h)-6cos(x+h)+7-5sinx+6cosx-7]$

$=\underset{h\to 0}{lim}\frac{1}{h}[sin(x+h)-sinx]-\underset{h\to 0}{lim}\frac{6}{h}[cos(x+h)-cosx]$

$=\underset{h\to 0}{lim}\frac{5}{h}\left[2cos\left(\frac{x+h+x}{2}\right)sin\left(\frac{x+h-x}{2}\right)\right]-\underset{h\to 0}{lim}\frac{6}{h}\left[-2sin\left(\frac{x+h+x}{2}\right)sin\left(\frac{x+h-x}{2}\right)\right]$

$=\underset{h\to 0}{lim}5\cdot cos\left(\frac{2x+h}{2}\right)\times \underset{h\to 0}{lim}\frac{sin(h/2)}{h/2}+\underset{h\to 0}{lim}sin\left(\frac{2x+h}{2}\right)\times \underset{h\to 0}{lim}\frac{sinh/2}{h/2}$

$=5\cdot cos\left(\frac{2x+0}{2}\right)\times 1+6\times sin\left(\frac{2x+0}{2}\right)\times 1$

$=5cosx+6sinx.$

(vii) Given

$f(x)=2tanx-7secx$

$f(x)=\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}\frac{2tan(x+h)-7sec(x+h)-(2tanx-7secx)}{h}$

$=\underset{h\to 0}{lim}\frac{2}{h}[tan(x+h)-tanx]-\underset{h\to 0}{lim}\frac{7}{h}[sec(x+h)-secx]$

$=\underset{h\to 0}{lim}\frac{2}{h}\left[\frac{sin(x+h)}{cos(x+h)}-\frac{sinx}{cosx}\right]-\underset{h\to 0}{lim}\frac{7}{h}\left[\frac{1}{cos(x+h)}-\frac{1}{x}\right].$

$=\underset{h\to 0}{lim}\frac{2}{h}\left[\frac{sin(x+h)cosx-sinxcos(x+h)}{cos(x+h)cosx}\right]-\underset{h\to 0}{lim}\frac{7}{h}\left[\frac{cosx-cos(x+h)}{cos(x+h)cosx}\right]$

$=\underset{h\to 0}{lim}\frac{2}{h}\left[\frac{sin((x+h)-x)}{cos(x+h)cosx}\right]-\underset{h\to 0}{lim}\frac{7}{h}[-2sin\left(\frac{x+h+x}{2}\right)sincos\left(\frac{x-(x+h))}{2}\right]$

$=\underset{h\to 0\cdot h}{lim}\frac{2}{cos(x+h)cos(x+h)cosx}]+\underset{h\to 0}{lim}\frac{7}{h}\left[\frac{2sin\left(\frac{2x+h}{2}\right)sin(-h/2)}{cos(x+h)cosx}\right]$

$=\underset{h\to 0}{lim}\frac{2}{cos(x+h)cosx}\times \underset{h\to 0}{lim}\frac{sinh}{h}+\underset{h\to 0}{lim}\frac{sin\left(\frac{2x+h}{2}\right)}{cos(x+h)cosx}.(-1)\underset{h\to 0}{lim}\frac{sinh/2}{h/2}$

$=\frac{2}{cos(x+0)cosx}\times 1+7sin\left(\frac{2x+0}{2}\right)\times (-1)$

$$  $=2s{c}^{2}x-7secxtanx$

 

Miscellaneous Exercise.

 

Q2.  $(x+a)$

A.2. Given, f(x) = x + a.

So, f(x) =  $\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}\frac{(x+h+a)-(x+a)}{h}$

$=\underset{h\to 0}{lim}\frac{h}{h}$

= 1

 

Q3.  $(px+q)\left(\frac{r}{x}+s\right)$

A.3. Given, f(x) = $(px+q)\left(\frac{r}{x}+s\right)=pr+pox.+\frac{qr}{x}+qs.$

$=\underset{h\to 0}{lim}So,f(x)=\frac{d}{dx}\left(pr+psx+\frac{qr}{x}+qs\right)$

$=\frac{d}{dx}(pr)+\frac{d}{dx}(psx)+\frac{d}{dx}\left(\frac{qr}{x}\right)+\frac{d}{dx}(qs)$

$=0+ps-\frac{qy}{x^2}+0$

$=ps-\frac{qr}{x^2}.$

 

Q4. (ax + b) (cx + d)²

A.4. Given, f (x) = (ax + b) (cx + d)²

So, f´(x) = (ax +b)  $\frac{d}{dx}{(cx+d)}^2+{(cx+d)}^2\frac{d}{dx}(ax+b)$

$=(ax+b)\frac{d}{dx}\left[(c+d)(cx+d)\right]+{(cx+d)}^{2}a$

$=(ax+b)\left[(c+d)\frac{d}{dx}(x+d)+(c+d)\frac{d}{dx}(c+d)\right]+a{(cx+d)}^2$

$=(ax+b)[c(cx+d)+c(cx+d)]+a{(cx+d)}^2$

$=(ax+b)·2·c(cx+d)+a{(cx+d)}^2$

 

Q5.  $\frac{ax+b}{cx+d}$

A.5. Given, f (x) = $\frac{ax+b}{cx+d}$

So, f (x) =  $\frac{(cx+d)\frac{d}{dx}(ax+b)-(ax+b)\frac{d}{dx}(cx+d)}{({(x+d)}^2}$

$=\frac{(cx+d)a-(ax+b)c}{{(cx+d)}^2}$

$=\frac{acx+ad-acx-cb}{{(cx+d)}^2}$

$=\frac{ad-bc}{{(cx+d)}^2}$

 

Q6.  $\frac{1+\frac{1}{x}}{1-\frac{1}{x}}$

A.6. Given, f (x) = $\frac{1+\frac{1}{x}}{1-\frac{1}{x}}=\frac{\frac{x+1}{x}}{\frac{x-1}{x}}=\frac{x+1}{x-1}$

So, f´(x) =  $\frac{(x-1)\frac{d}{dx}(x+1)-(x+1)\frac{d}{dx}(x-1)}{{(x-1)}^2}$

=  $\frac{(x-1)-(x+1)}{{(x-1)}^2}$

$=\frac{x-1-x-1}{{(x-1)}^2}=\frac{-2}{{(x-1)}^2}$

 

Q7.  $\frac{1}{a{x}^2+bx+c}$

A.7. Given, f (x) = $\frac{1}{a{x}^2+bx+c}$

So, f´(x) =  $\frac{\left(a{x}^2+bx+c\right)\frac{d}{dx}(1)-1·\frac{d}{dx}\left(a{x}^2+bx+c\right)}{{\left(a{x}^2+bx+c\right)}^2}$

$=\frac{\left(a{x}^2+bx+c\right)(0)-(2ax+b)}{{\left(a{x}^2+bx+c\right)}^2}$

$=-\frac{(2ax+b)}{{\left(a{x}^2+bx+c\right)}^2}$

 

Q8.  $\frac{(ax+b)}{p{x}^2+qx+r}$

A.8. Given, f (x) = $\frac{(ax+b)}{p{x}^2+qx+r}$

So, f(x) =  $\frac{\left(p{x}^2+qx+r\right)\frac{d}{dx}(ax+b)-(ax+b)\frac{d}{dx}\left(p{x}^2+qx+r\right)}{{\left(p{x}^2+qx+r\right)}^2}$

  $=\frac{\left(p{x}^2+qx+r\right)a-(ax+b)(2xp+q)}{{\left(p{x}^2+qx+r\right)}^2}$

$=\frac{ap{x}^2+aqx+ar-2ap{x}^2-aqx-2bpx-b}{{\left(p{x}^2+qx+9\right)}^2}q.$

$=\frac{-ap{x}^2-2bpx+ar-bq}{{\left(p{x}^2+qx+r\right)}^2}$

 

Q9.  $\frac{p{x}^2+qx+r}{ax+b}$

A.9. Given, f (x) =  $\frac{p{x}^2+qx+r}{ax+b}$

$f^{\prime }(x)=\frac{(ax+b)\frac{d}{dx}\left(p{x}^2+qx+r\right)-\left(p{x}^2+qx+r\right)\frac{d}{dx}(ax+b)}{{(ax+b)}^2}$

$=\frac{(ax+b)(2xp+q)-\left(p{x}^2+qx+r\right)(a)}{{(ax+b)}^2}$

$=\frac{2ap{x}^2+2bpx+aqx+bq-ap{x}^2-aqx-ar}{{(ax+b)}^2}$

$=\frac{ap{x}^2+2bpx+bq-ar}{{(ax+b)}^2}$

 

Q10.  $\frac{a}{x^4}-\frac{b}{x}+cosx$

A.10. Given, f (x) = $=\frac{a}{x^4}-\frac{b}{x}+cosx$

So, f´(x) =  $\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{a}{{(x+n)}^4}-\frac{b}{{(x+h)}^2}+cos(x+h)-\left(\frac{a}{x^4}-\frac{b}{x^2}+cosx\right)\right]$

$=\underset{h\to 0}{lim}\frac{a}{h}\left[\frac{1}{{(x+h)}^4}-\frac{1}{x^4}\right]-\underset{h\to 0}{lim}\frac{b}{h}\left[\frac{1}{{(x+h)}^2}-\frac{1}{x^2}\right]+\underset{h\to 0}{lim}\frac{1}{h}[cos(x+h)-cosx]$

$=\underset{h\to 0}{lim}\frac{a}{h}\left[\frac{x^4-{(x+h)}^4}{{(x+h)}^4·x^4}\right]-\underset{h\to 0}{lim}\frac{b}{h}\left[\frac{x^2-{(x+h)}^2}{{(x+h)}^2·x^2}\right]+$

$\underset{h\to 0}{lim}\frac{1}{h}\left[-2sin\left(\frac{x+h+x}{2}\right)sin\left(\frac{x+h-x}{2}\right)\right]$

$=\underset{h\to 0}{lim}\frac{a}{h}\left[\frac{x^4-x^4-4x^{3}h-6x^2{h}^2-4x{h}^3-h^3}{x^4·{(x+h)}^4}\right]-\underset{h\to 0}{lim}\frac{b}{h}\left[\frac{x^2-x^2-h^2-2xh}{x^2(x+h){}^2}\right]$

$+\underset{h\to 0}{lim}\frac{a}{h}\left[-2sin\left(\frac{2x+h}{2}\right)sin\frac{h}{2}\right]$

$=\underset{h\to 0}{lim}\frac{a}{h}\left[\frac{h\left(-4x^3-6x^{2}h-4x{h}^2-h^2\right)}{x^4(x+h){}^4}\right]-\underset{h\to 0}{lim}\frac{b}{h}\left[\frac{h(-h-2x)}{x^2(x+h){}^2}\right]$

$-\underset{h\to 0}{lim}sin\left(\frac{2x+h}{2}\right)\underset{h\to 0}{lim}\frac{sin\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}{\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}$

$=\underset{h\to 0}{lim}\frac{a\left(-4x^3-6x^{2}h-4x{h}^2-h^2\right)}{x^4(x+h){}^4}-\underset{h\to 0}{lim}\frac{b(-h-2x)}{x^2(x+h){}^2}$

$sin\left(\frac{2x+0}{2}\right)\times 1$

$=\frac{a\left(-4x^3\right)}{x^4·x^4}-\frac{b(-2x)}{x^2·x^2}-sinx.$

$=-\frac{4a}{x^5}+\frac{2b}{x^3}-sinx$

 

 

Q12. (ax + b)ⁿ

A.12. Given, f (x) = (ax + b)ⁿ

Chain rule,  $\frac{d}{dx}u{(x)}^n=nu{(x)}^{n-1}\frac{du}{dx}u(x)$ where

u(x) is a function of x.

So, f´(x) =  $\frac{d}{dx}{(ax+b)}^n$

$=n{(ax+b)}^{n-1}\frac{d}{dx}(ax+b)$

$=na{(ax+b)}^{n-1}$

 

Q13. (ax + b)ⁿ (cx + d)^m

A.13. Given, f´ (x) = (ax + b)ⁿ (cx + d)^m

So, f’(x) =  ${(ax+b)}^n\frac{d}{dx}{(cx+d)}^n+{(cx+d)}^m\frac{d}{dx}{(ax+b)}^n$

$={(ax+b)}^x·mc{(cx+d)}^{m-1}+{(cx+d)}^{m}na{(ax+b)}^{x-1}$

$={(ax+b)}^{n-1}(cx+d){}^{m-1}[(ax+b)·mc+(cx+d)na]$

$={(ax+b)}^{n-1}(cx+d){}^{m-1}[mc(ax+b)+na(cx+d)]$

 

Q14. sin(x + a)

A.14. Given, f (x) = sin(x + a)

So, f (x) =  $\underset{h\to 0}{lim}\frac{f(x+h)-f(x)}{h}$

$=\underset{h\to 0}{lim}sin\frac{(x+h+a)-sin(x+a)}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}·2cos\left(\frac{x+h+a+x+a}{2}\right)sin\left(\frac{x+h+a-(x+a)}{2}\right)$

$=\underset{h\to 0}{lim}cos\left(\frac{2x+2a+h}{2}\right)\underset{h\to 0}{lim}\frac{sin(\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.)}{\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}$

$=cos\frac{(2x+2a+0)}{2}\times 1$

= cos (x + a)

 

Q15. cosec x. cot x

A.15. Given f (x) = cosec x. cot x.

By Leibnitz product rule,

So, g´(x) =  $\underset{h\to 0}{lim}\frac{g(x+h)-g(x)}{h}$

$=\underset{h\to 0}{lim}\frac{cot(x+h)-cotx}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{cos(x+h)}{sin(x+h)}-\frac{cosx}{sinx}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{sinxcos(x+h)-cosxsin(x+h)}{sinxsin(x+h)}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{sin\left(x-(x+h)\right)}{sinxsin(x+h)}\right]$  $\left[\begin{array}{cc}\hfill \because csin(A-B)=\hfill & \hfill sinAcosB-cosAsinB\hfill \end{array}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{sin(-h)}{sinx.sin(x+h)}\right]$

$=\underset{h\to 0}{lim}\frac{1}{sinxsin(x+h)}\times (-1)\underset{h\to 0}{lim}\frac{sinh}{h}$

$=\frac{1}{sinxsin(x+0)}\times (-1)$

= -cosec²x.______(2)

And h´(x) =  $\underset{h\to 0}{lim}\frac{h(x+h)-h(x)}{h}$

$=\underset{h\to 0}{lim}\frac{cosec(x+h)-cosecx}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{1}{sin(x+h)}-\frac{1}{sinx}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[\frac{sinx-sin(x+h)}{sinx·sin(x+h)}\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}[\frac{2cos\left(\frac{x+x+h}{2}\right)sin\left(\frac{x-(x+h)}{2}\right)}{sinxsin(x+h).}]$

 

 

Q16.  $\frac{cosx}{1+sinx}$

A.16. Given, f (x) =  $\frac{cosx}{1+sinx}$

So, f´(x) =  $\frac{(1+sinx)\frac{d}{dx}(cosx)-cosx\frac{d}{dx}(1+sinx)}{{(1+sinx)}^2}$

Putting (2) and (3) in (1) we get,

$f^{\prime }(x)=\frac{(1+sinx)(-sinx)-cosx(cosx)}{{(1+sinx)}^2}$

$=\frac{sinx-si{n}^{2}x-co{s}^{2}x}{{(1+sinx)}^2}$

$=\frac{-sinx-\left(si{n}^{2}x+co{s}^{2}x\right)}{{(1+sinx)}^2}$

$=\frac{-(sinx+1)}{{(1+sinx)}^2}=-\frac{1}{1+sinx}.$

 

Q17.  $\frac{sinx+cosx}{sinx-cosx}$

A.17. Given, f (x) =  $\frac{sinx+cosx}{sinx-cosx}$

So, f´(x) =  $\frac{sinx-cosx\frac{d}{dx}\left(sinx+cosx\right)-\left(sinx+cos2\right)\frac{d}{dx}\left(sinx-cosx\right)}{{\left(sinx-cosx\right)}^2}$

Let g(x) = cos x and p(x) = sin x.

{from so g’(x) A ) (upto equation 3)

Let g(x) = cos2 and p(x) = sin x.

So, g´(x) =  $\underset{h\to 0}{lim}\frac{g(x+h)-g(x)}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}[cos(x+h)-cosx]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[-2·sin\left(\frac{x+h+x}{2}\right)sin\left(\frac{x+h-x}{2}\right)\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[-2sin\left(\frac{2x+h}{2}\right)sin\left(\frac{h}{2}\right)\right]$

$=-sin\left(\frac{2x+0}{2}\right)\times 1$

= -sin x ______ (2)

And p´(x) =  $\underset{h\to 0}{lim}\frac{p(x+h)-p(x)}{h}$

$=\underset{h\to 0}{lim}\frac{sin(x+h)-sinx}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}\cdot 2cos\left(\frac{2x+h}{2}\right)sin\left(\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\right)$

$=\underset{h\to 0}{lim}cos\left(\frac{2x+h}{2}\right)\times \underset{h\to 0}{lim}\frac{sin\left(\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\right)}{\left(\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\right)}$

= cos x _____ (3)

Putting (2) and (3) in (1) we get,

$f^{\prime }(x)=\frac{(sinx-cosx)[cosx-sinx]-(csinx+cosx)[cosx+sinx]}{{(sinx-cosx)}^2}$

$=\frac{-{(sinx-cosx)}^2-{(sinx+cosx)}^2}{{(sinx-cosx)}^2}$

$=\frac{-\left(si{n}^{2}x+co{s}^{2}x\right)+2sinxcosx-\left(si{n}^{2}x+co{s}^{2}x\right)-2sinxcosx}{{(sinx-cosx)}^2}$

$=\frac{-1-1}{{(sinx-cosx)}^2}=\frac{-2}{{(sinx-cosx)}^2}$

 

Q18.  $\frac{secx-1}{secx+1}$

A.18. Given, f (x) =  $\frac{secx-1}{secx+1}$

$=\frac{\frac{1}{cosx}-1}{\frac{1}{cosx}+1}$

$=\frac{1-cosx}{1+cosx}$

So, f´(x) =  $\frac{(1+cosx)\frac{d}{dx}(1-cosx)-(1-cosx)\frac{d}{dx}(1+cosx)}{{(1+cosx)}^2}$

$=\frac{(1+cosx)(-1)\frac{d}{dx}(-cosx)-(1-cosx)\frac{d}{dx}(cosx)}{{(1+cosx)}^2}$

Let g(x) = cos x.

So, g´(x)  $=\underset{h\to 0}{lim}\frac{g(x+h)-g(x)}{h}$

$=\underset{h\to 0}{lim}\frac{cos(x+h)-cosx}{h}$

$=\underset{h\to 0}{lim}-\frac{2}{h}sin\left(\frac{x+h+x}{2}\right)sin\left(\frac{x+h-x}{2}\right)$

$=\underset{h\to 0}{lim}\frac{-2}{h}sin\left(\frac{2x+h}{2}\right)sin\left(\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\right)$

$=\underset{h\to 0}{lim}-sin\left(\frac{2x+h}{2}\right)\times \underset{h\to 0}{lim}\frac{sin\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}{\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}$

$=-sin\left(\frac{2x+0}{2}\right)\times 1$

= -sin x.

So, f´(x)  $\frac{(1+cosx)(-1)(-sinx)-(1-cosx)(-sinx)}{{(1+cosx)}^2}$

$=\frac{sinx+cosxsinx+sinx-sinxcosx}{{(1+cosx)}^2}$

$=\frac{2sinx}{{(1+cosx)}^2}$

$=\frac{2sinx}{{\left(1+\frac{1}{secx}\right)}^2}=\frac{2sinx\times se{c}^{2}x}{{(secx+1)}^2}=\frac{2secx·sinx}{{(sinx+1)}^2·cosx}.$

$=\frac{2secx·tanx}{{(sinx+1)}^2}$

 

Q19. sinⁿx

A.19. Given, f(x) =sinⁿx

By chain rule,

f´(x) = n(sin x)^n-1 $\frac{d}{dh}$ sin x

Let (gx) = sinx

So, g´(x)  $\underset{h\to 0}{lim}\frac{g(x+h)-g(x)}{h}$

$=\underset{h\to 0}{lim}\frac{sin(x+h)-sinx}{h}$

$=\underset{h\to 0}{lim}\frac{2}{h}cos\left(\frac{2a+h}{2}\right)sin\left(\frac{h}{2}\right)$

$=\underset{h\to 0}{lim}cos\left(\frac{2x+h}{2}\right)\times \underset{h\to 0}{lim}\frac{sin\left(\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\right)}{\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}$

=  $cos\frac{(2x+0)}{2}\times 1$

= cos x.

So, f´(x) = n(sin x)^n-1 cos x.

 

Q20.  $\frac{a+bsinx}{c+dcosx}$

A.20. Given, f (x) = $\frac{a+bsinx}{c+dcosx}$

f´(x) =  $\frac{(c+dcosx)\frac{d}{dx}(bsinx)-(a+bsinx)\frac{d}{dx}(c+dcosx)}{{(c+dcosx)}^2}$

$f^{\prime }(x)=\frac{(c+dcosx)·b·\frac{d}{dx}(sinx)-(a+bsinx)·d·\frac{d}{dx}(cosx)}{{(c+dcosx)}^2}\_\_\_\_\_(1)$

{Copy (A)}

So, g´(x) =  $\underset{h\to 0}{lim}\frac{g(x+h)-g(x)}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}[cos(x+h)-cosx]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[-2·sin\left(\frac{x+h+x}{2}\right)sin\left(\frac{x+h-x}{2}\right)\right]$

$=\underset{h\to 0}{lim}\frac{1}{h}\left[-2sin\left(\frac{2x+h}{2}\right)sin\left(\frac{h}{2}\right)\right]$

$=-sin\left(\frac{2x+0}{2}\right)\times 1$

= sin x ______ (2)

And p´(x) =  $\underset{h\to 0}{lim}\frac{p(x+h)-p(x)}{h}$

$=\underset{h\to 0}{lim}\frac{sin(x+h)-sinx}{h}$

$=\underset{h\to 0}{lim}\frac{1}{h}\cdot 2cos\left(\frac{2x+h}{2}\right)sin\left(\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\right)$

$=\underset{h\to 0}{lim}cos\left(\frac{2x+h}{2}\right)\times \underset{h\to 0}{lim}\frac{sin\left(\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\right)}{\left(\raisebox{1ex}{$h$}\!\left/ \!\raisebox{-1ex}{$2$}\right.\right)}$

= cos x _____ (3)

So, put (2) and (3) in (1) we get,

$f^{\prime }(x)=\frac{(c+dcosx)(b·cosx)-(a+bsinx)(-d·sinx)}{{(c+dcosx)}^2}$

$=\frac{beccosx+bdco{s}^{2}x+adsinx+bdsi{n}^{2}x}{{(c+dcosx)}^2}$

$=\frac{bccosx+adsinx+bd\left(co{s}^{2}x+si{n}^{2}x\right)}{{(c+dcosx)}^2}$

$=\frac{bccosx+adsinx+bd}{{(c+dcosx)}^2}$

 

 

Q22. x⁴ (5 sin x 3 cos x)

A.22. Given, f (x) = x⁴. (5 sin x 3 cos x)

$f^{\prime }(x)=x^4\frac{d}{dx}(5sinx-3cosx)+(5sinx-3cosx)\frac{d{x}^4}{dx}.$

$=x^4\left[5\frac{d}{dx}sinx-3·\frac{dcosx}{dx}\right]+[5sinx-3cosx]·4x^3$

As  $\frac{d}{dx}sinx=cosx$

and  $\frac{d}{dx}cosx=-sinx$

Thus,

$f^{\prime }(x)=x^4[5cosx+3sinx]+[5sinx-3cosx]·4x^3$

$=x^3[5cosx+3xsinx+20sinx-12cosx]$

$=x^3[5xcosx+3xsinx+20sinx-12cosx].$

 

Q23. (x² + 1) cos x

A.23. Given, f (x) = (x² + 1) cos x

f´(x) = (x² + 1) $\frac{d}{dx}cosx+cosx\frac{d}{dx}\left(x^2+1\right)$

$=\left(x^2+1\right)(-sinx)+cosx(2x+0)\left[\because \frac{d}{dx}cosx=-sinx\right]$

= x² sin x sin x + 2x cos x.

 

Q24. (ax² + sin x) (p +q cos x)

A.24. Given, f (x) = (ax² + sin x) (p +q cos x).

So, f´(x) = (ax² + sin x) $\frac{d}{dx}$  $(p+qcosx)+(p+qcosx)\frac{d}{dx}(a{x}^2+sinx)$

$=\left(a{x}^2+sinx\right)\left(0+q\frac{d}{dx}cosx\right)+(p+qcosx)\left(a\frac{d}{dx}\left(x^2\right)+\frac{d}{dx}sinx\right)$

$=\left(a{x}^2+sinx\right)(q(-sinx))+(p+qcosx)(a·2x+cosx)$

= q sin x(ax² + sin x) + (p + q cos x) (2ax + cos x)

 

 

Q26.  $\frac{4x+5sinx}{3x+7cosx}$

A.26. Given, f (x) =  $\frac{4x+5sinx}{3x+7cosx}$

So,

$f^{\prime }(x)=\frac{(3x+7cosx)\frac{d}{dx}(4x+5sinx)-(4x+5sinx)\frac{d}{dx}(3x+7cosx)}{{(3x+7cosx)}^2}$

$=\frac{(3x+7cosx)(4x+5cosx)-(4x+5sinx)(3-7sinx)}{{(3x+7cosx)}^2}$

 

$=\frac{15xcosx+28cosx+28xsinx-15sinx+35\left(co{s}^{2}x+si{n}^{2}x\right)}{{(3x+7cosx)}^2}$

$=\frac{35+15xcosx+28cosx+28xsinx-15sinx}{{(3x+7cosx)}^2}$

 

Q27.  $\frac{x^{2}cos\left(\frac{\pi }{4}\right)}{sinx}$

A.27. Given, f (x) =  $\frac{x^{2}cos\left(\frac{\pi }{4}\right)}{sinx}$

$=\frac{x^2}{sinx}\times cos\frac{\pi }{4}$

So, f´(x) =

$=cos\frac{\pi }{4}\left[\frac{2·xsinx-x^{2}cosx}{si{n}^{2}x}\right]$

$=xcos\frac{\pi }{4}\left[\frac{2sinx-xcosx}{si{n}^{2}x}\right].$

 

Q28.  $\frac{x}{1+tanx}$

A.28. Given, f (x) =  $\frac{x}{1+tanx}$

$=\frac{x}{1+\frac{sinx}{cosx}}$

$=\frac{cosx·x}{cosx+sinx}$

$So,f^{\prime }(x)=\frac{(cosx+sinx)\frac{d}{dx}(x\cdot cosx)-\left(x\cdot cosx\right)\frac{d}{dx}\left(cosx+sinx\right)}{{(cosx+sinx)}^2}$

$=\frac{(cosx+sinx)(-xsinx+cosx)-\left(-x·sinxcosx+xco{s}^{2}x\right)}{{(cosx+sinx)}^2}$

$=\frac{-xcosxsinx+co{s}^{2}x-xsi{n}^{2}x+sinxcosx+xsinxcosx-xco{s}^{2}x}{{(cosx+sinx)}^2}$

$=\frac{co{s}^{2}x+sinxcosx-x\left(si{n}^{2}x+co{s}^{2}x\right)}{{(cosx+csinx)}^2}$

Dividing numerator and denominator by cos2x we get,

$=\frac{\frac{co{s}^{2}x}{co{s}^{2}x}+\frac{sinx}{cosx}·\frac{cosx}{cosx}-x\frac{1}{co{s}^{2}x}}{{\left(\frac{cosx}{cosx}+\frac{sinx}{cosx}\right)}^2}$

$=\frac{1+tanx-xse{c}^{2}x}{{(1+tanx)}^2}$

 

Q29. (x + sec x) (x tan x)

A.29. Given, f (x) = (x + sec x) (x tan x)

So, f´(x) = (x + see x)  $\frac{d}{dx}(x-tanx)+(x-tanx)\frac{d}{dx}(x+secx).$

$=(x+secx)\left(\frac{dx}{dx}-\frac{d}{dx}tanx\right)+(x-tanx)\left(\frac{dx}{dx}+\frac{d}{dx}secx\right)$

Let g(x) = tan x.

 

Q30.  $\frac{x}{si{n}^{n}x}$

A.30. Given, f (x) =  $\frac{x}{si{n}^{n}x}$

So, f´(x) =  $\frac{\left(si{n}^{x}x\frac{dx}{dx}\right)-\left(x\frac{d}{dx}si{n}^{x}x\right)}{{\left(si{n}^{x}x\right)}^2}$

=  $\frac{si{n}^{x}x-x·n{(sinx)}^{x-1}\frac{d}{dx}sinx}{{(sinx)}^{2x}}$

$=\frac{{(sinx)}^n-x·x{(sinx)}^{n-1}·cosx}{{(sinx)}^{2n}}$

$=\frac{{(sinx)}^{n-1}[sinx-nx·cosx]}{{(sinx)}^{2n}}$

$=\frac{sinx-n·x·cosx}{{(sinx)}^{2n-(n-1)}}$

$=\frac{sinx-nxcosx}{{(sinx)}^{n+1}}$