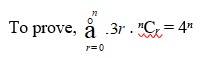NCERT Solutions for Class 11 Maths Chapter 8 Binomial Theorem: Overview, Questions, Preparation

Exercise 8.1

Expand each of the expressions in Exercises 1 to 5.

Q1. (1–2x)⁵

A.1. (1 – 2x)⁵

By using binomial theorem we have,

= ⁵C₀ (1)⁵+⁵C₁ (1)⁴ (-2x) + ⁵C₂ (1)³ (-2x)²+⁵C₃ (1)² (-2x)³+⁵C₄ (1)¹ (-2x)⁴+⁵C₅ (-2x)⁵

=  $\left(\frac{5!}{0!\left(5-0\right)!}<br>\mathrm{\prime \; 1}\right)$ + $\left[\frac{5!}{1!\left(5-1\right)!}\prime 1\prime (-2x)\right]$ +[x1× (4x²)] +  $\left[\frac{5!}{3!\left(5-3\right)!}\prime 1\prime (-8x^3)\right]$ +   + 

= 1 +  $\left[\frac{\mathrm{5\prime }<br>4!}{\mathrm{1\prime } <br>4!}\prime \left(-2x\right)\right]$ +  $\left[\frac{\mathrm{5\prime }<br>\mathrm{4\prime }<br>3!}{\mathrm{2\prime }<br>\mathrm{1\prime }<br>3!}\times 4x^2\right]$ +  $\left[\frac{\mathrm{5\prime }<br>\mathrm{4\prime }<br>3!}{3!\prime <br>2!}\times (-8x^3)\right]$ +  $\left[\frac{\mathrm{5\prime }<br>4!}{4!\prime <br>1}\times 16x^4\right]$ + [1 × (-32x⁵)]

= 1 + [5 × (–2x)] + [10 × 4x²] + [10 × (–8x³)] + [5 × 16x⁴] + [–32x⁵]

= 1 – 10x + 40x² – 80x³ + 80x⁴ – 32x⁵

 

Q2. ${\left(\frac{2}{x}-\frac{x}{2}\right)}^5$

A.2. ${\left(\frac{2}{x}-\frac{x}{2}\right)}^5$

Using (x – y)ⁿ

=  ⁵C₀ ${\left(\frac{2}{x}\right)}^5$ – ⁵C₁ ${\left(\frac{2}{x}\right)}^4$  $\left(\frac{x}{2}\right)$ + ⁵C₂ ${\left(\frac{2}{x}\right)}^3$  ${\left(\frac{x}{2}\right)}^2$ –⁵C₃ ${\left(\frac{2}{x}\right)}^2$  ${\left(\frac{x}{2}\right)}^3$ + ⁵C₄ $\left(\frac{2}{x}\right)$  ${\left(\frac{x}{2}\right)}^4$ – ⁵C₅ ${\left(\frac{x}{2}\right)}^5$

=  $\left[\frac{5!}{0!\left(5-0\right)!}<br>\frac{\mathrm{\prime 3}2}{x^5}\right]$ –  $\left[\frac{5!}{1!\left(5-1\right)!}\prime \frac{16}{x^4}\times \frac{x}{2}\right]$ +  $\left[\frac{5!}{2!\left(5-2\right)!}\prime \frac{8}{x^3}\prime \frac{x^2}{4}\right]$ –  $\left[\frac{5!}{3!\left(5-3\right)!}\prime \frac{4}{x^2}\prime \frac{x^3}{8}\right]$ +  $\left[\frac{5!}{4!\left(5-4\right)!}\prime \frac{x}{2}\prime \frac{x^4}{16}\right]$ –  $\left[\frac{5!}{5!\left(5-5\right)!}\prime \frac{x^5}{32}\right]$

=  $\left[1\prime \frac{32}{x^5}\right]$ –  $\left[\frac{5\prime 4!}{1\prime 4!}\prime \frac{8}{x^3}\right]$ +  $\left[\frac{5\prime \mathrm{4\prime }<br>3!}{2\prime <br>\mathrm{1\prime }<br>3!}\prime \frac{2}{x}\right]$ –  $\left[\frac{\mathrm{5\prime }<br>\mathrm{4\prime }<br>3!}{3!\prime <br>2!}\times \frac{x}{2}\right]$ +  $\left[\frac{\mathrm{5\prime } <br>4!}{4!\prime <br>1!}\prime \frac{x^3}{8}\right]$ –  $\left[\mathrm{1\prime }<br>\frac{x^5}{32}\right]$

=  $\frac{32}{x^5}$ –  $\frac{40}{x^3}$ +  $\frac{20}{x}$ – 5x +  $\frac{5}{8}{x}^3$ –  $\frac{x^5}{32}$

 

Q3. (2x – 3)⁶

A.3. (2x – 3)⁶

By using binomial theorem,

(2x – 3)⁶

= ⁶C₀ (2x)⁶  +  ⁶C₁ (2x)⁵(–3)  +  ⁶C₂ (2x)⁴(–3)²  +  ⁶C₃ (2x)³(–3)³  +  ⁶C₄ (2x)²(–3)⁴  +  ⁶C₅ (2x)(–3)⁵ +  ⁶C₆ (–3)⁶

 

= 64x⁶  –  576x⁵  +  2160x⁴  –  4320x³  +  4860x²  –  2916x  +  729

 

Q4. ${\left(\frac{x}{3}+\frac{1}{x}\right)}^5$

A.4. ${\left(\frac{x}{3}+\frac{1}{x}\right)}^5$

By using binomial theorem,

 

=  $\frac{x^5}{243}$ +  $\frac{5x^3}{81}$ +  $\frac{10x}{27}$ +  $\frac{10}{9x}$ +  $\frac{5}{3x^3}$ +  $\frac{1}{x^5}$

 

Q5. ${\left(x+\frac{1}{x}\right)}^6$

A.5. ${\left(x+\frac{1}{x}\right)}^6$

By binomial theorem,

 

=  $x^6$ + 6  $x^4$ + 15  $x^2$ + 20 +  $\frac{15}{x^2}$ +  $\frac{6}{x^4}$ +  $\frac{1}{x^6}$

Using binomial theorem, evaluate each of the following:

 

Q6. (96)³

A.6. (96)³ = (100 – 4)³

Using (x – y)ⁿ expansion

= ³C₀(100)³ – ³C₁(100)²(4) + ³C₂(100)(4)² – ³C₃(4)³

 

= 1000000 – 120000 + 4800 – 64

= 1004800 – 120064

= 884736

 

Q7. (102)⁵

A.7. (102)⁵ = (100 + 2)⁵

By using binomial theorem,

= ⁵C₀(100)⁵ + ⁵C₁(100)⁴(2) + ⁵C₂(100)³(2)² + ⁵C₃(100)²(2)³ + ⁵C₄(100)(2)⁴ + ⁵C₅(2)⁵

=  $\left[\frac{5!}{0!\left(5-0\right)!}\prime 10000000000\right]$ +  $\left[\frac{5!}{1!\left(5-1\right)!}\prime 00000000\prime 2\right]$ +  $\left[\frac{5!}{2!\left(5-2\right)!}\prime 1000000\prime 4\right]$ +  $\left[\frac{5!}{3!\left(5-3\right)!}\prime 10000\prime 8\right]$ +  $\left[\frac{5!}{4!\left(5-4\right)!}\prime 100\prime 16\right]$ +  $\left[\frac{5!}{5!\left(5-5\right)!}\prime 32\right]$

= [1 × 10000000000] +  $\left[\frac{\mathrm{5\prime }<br>4!}{\mathrm{1\prime }<br>4!}\prime 00000000\right]$ +  $\left[\frac{\mathrm{5\prime }<br>\mathrm{4\prime }<br>3!}{\mathrm{2\prime }<br>\mathrm{1\prime }<br>3!}\prime 4000000\right]$ +  $\left[\frac{\mathrm{5\prime }<br>\mathrm{4\prime }<br>3!}{3!\prime <br>2!}\prime 80000\right]$ +  $\left[\frac{\mathrm{5\prime }<br>4!}{4!\prime <br>1!}\prime 1600\right]$ + [1 × 32]

= 10000000000 + 1000000000 + 40000000 + 800000 + 8000 + 32

= 11040808032

 

Q8. (101)⁴

A.8. (101)⁴ = (100 + 1)⁴

By binomial theorem we get,

= ⁴C₀(100)⁴ + ⁴C₁(100)³(1) + ⁴C₂(100)²(1)² + ⁴C₃(100)(1)³ + ⁴C₄(1)⁴

=  $\left[\frac{4!}{0!\left(4-0\right)!}\prime 00000000\right]$ +  $\left[\frac{4!}{1!\left(4-1\right)!}\prime 000000\prime 1\right]$ +  $\left[\frac{4!}{2!\left(4-2\right)!}\prime 10000\prime 1\right]$ +  $\left[\frac{4!}{3!\left(4-3\right)!}\prime 100\prime 1\right]$ +  $\left[\frac{4!}{4!\left(4-4\right)!}\prime 1\right]$

= [1 × 100000000] +  $\left[\frac{\mathrm{4\prime }<br>3!}{\mathrm{1\prime }<br>3!}\prime 1000000\right]$ +  $\left[\frac{\mathrm{4\prime }<br>\mathrm{3\prime }<br>2!}{\mathrm{2\prime }<br>\mathrm{1\prime }<br>2!}\prime 10000\right]$ +  $\left[\frac{\mathrm{4\prime }<br>3!}{3!\prime <br>1!}\prime 100\right]$ +[1 × 1]

= 100000000 + 4000000 + 60000 + 400 + 1

= 104060401

 

Q9. (99)⁵

A.9. (99)⁵ = (100 – 1)⁵

By binomial theorem we get,

= ⁵C₀(100)⁵ –⁵C₁(100)⁴(1) + ⁵C₂(100)³(1)²–⁵C₃(100)²(1)³ + ⁵C₄(100)(1)⁴ –⁵C₅(1)⁵

=  $\left[\frac{5!}{0!\left(5-0\right)!}\prime 10000000000\right]$ –  $\left[\frac{5!}{1!\left(5-1\right)!}\prime 00000000\prime 1\right]$ +  $\left[\frac{5!}{2!\left(5-2\right)!}\prime 000000\prime 1\right]$ –  $\left[\frac{5!}{3!\left(5-3\right)!}\prime 10000\prime 1\right]$ +  $\left[\frac{5!}{4!\left(5-4\right)!}\prime 100\prime 1\right]$ –  $\left[\frac{5!}{5!\left(5-5\right)!}\prime 1\right]$

= [1 × 10000000000] –  $\frac{\mathrm{5\prime }<br>4!}{\mathrm{1\prime }<br>4!}\prime 00000000$ +  $\left[\frac{\mathrm{5\prime }<br>\mathrm{4\prime }<br>3!}{\mathrm{2\prime }<br>\mathrm{1\prime }<br>3!}\prime 100000\right]$ –  $\left[\frac{\mathrm{5\prime }<br>\mathrm{4\prime }<br>3!}{3!\prime <br>2!}\prime 10000\right]$ +  $\left[\frac{\mathrm{5\prime }<br>4!}{4!\prime <br>1!}\prime 100\right]$ – [1 × 1]

= 10000000000 – 500000000 + 10000000 – 100000 + 500 – 1

= 10010000500 – 500100001

= 9509900499

 

Q10. Using Binomial Theorem, indicate which number is larger (1.1)¹⁰⁰⁰⁰or 1000.

A.10. We know that,

(1.1)¹⁰⁰⁰⁰ = (1 + 0.1)¹⁰⁰⁰⁰

By using binomial theorem,

= ¹⁰⁰⁰⁰C₀(1)¹⁰⁰⁰⁰ + ¹⁰⁰⁰⁰C₁ (1)^{(10000 – 1)} (0.1) + other positive terms

=  $\left[\frac{10000!}{0!\left(10000-0\right)!}\prime 1\right]$ +  $\left[\frac{10000!}{1!\left(10000-1\right)!}\prime 1\prime 0.1\right]$ + other positive terms

= [1 × 1] +  $\left[\frac{1000\mathrm{0\prime } <br>9999!}{\mathrm{1\prime }<br>9999!}\prime 0.1\right]$ + other positive terms

= 1 + 1000 + other positive terms

= 1001 + other positive terms

Hence, (1.1)¹⁰⁰⁰⁰> 1000

 

Q11. Find (a + b)4– (a – b)4. Hence, evaluate ${(\surd 3+\surd 2)}^4-{(\surd 3-\surd 2)}^4.$

A.11. Using binomial theorem we have,

(a + b)⁴ – (a – b)⁴

= [⁴C₀a⁴ + ⁴C₁a³b + ⁴C₂a²b² + ⁴C₃ab³ + ⁴C₄b⁴] – [⁴C₀a⁴ – ⁴C₁a³b + ⁴C₂a²b² – ⁴C₃ab³ + ⁴C₄b⁴]

= ⁴C₀a⁴ + ⁴C₁a³b + ⁴C₂a²b² + ⁴C₃ab³ + ⁴C₄b⁴– ⁴C₀a⁴ + ⁴C₁a³b – ⁴C₂a²b² + ⁴C₃ab³ – ⁴C₄b⁴

= [2 ×⁴C₁a³b] + [2 ×⁴C₃ab³]

=  $\left[\mathrm{2\prime }<br>\frac{4!}{1!\left(4-1\right)!}{a}^{3}b\right]$ +  $\left[\mathrm{2\prime }<br>\frac{4!}{3!\left(4-3\right)!}a{b}^3\right]$

=  $\left[\mathrm{2\prime }<br>\frac{\mathrm{4\prime }<br>3!}{\mathrm{1\prime }<br>3!}{a}^{3}b\right]$ +  $\left[\mathrm{2\prime }<br>\frac{\mathrm{4\prime }<br>3!}{3!\prime <br>1!}a{b}^3\right]$

= 8a³b + 8ab³

Hence putting a = √3 $$ and b = √2 $$ we have,

${(\surd 3+\surd 2)}^4$ –  ${(\surd 3-\surd 2)}^4$

= 8  ${(\surd 3)}^3(\surd 2)$ + 8  $(\surd 3)$  ${(\surd 2)}^3$

= (8 × 3 √3 $$ ×√2  $$ )+ (8 × √3 $$ × 2 √2 $$ )

= 24 √6 $$ + 16 √6 $$

= 40 √6 $$

Q12. Find (x + 1)6+ (x – 1)6. Hence or otherwise evaluate ${(\surd 2+1)}^6+{(\surd 2-1)}^6.$

A.12. By binomial expansion we have,

${\left(x+1\right)}^6$ +  ${\left(x-1\right)}^6$

= [⁶C₀(x⁶) + ⁶C₁(x⁵)(1) + ⁶C₂(x⁴)(1) ² + ⁶C₃(x³)(1)³ + ⁶C₄(x²)(1)⁴ + ⁶C₅(x)(1)⁵ + ⁶C₆(1)⁶] + [⁶C₀(x⁶) – ⁶C₁(x⁵)(1) + ⁶C₂(x⁴)(1) ² – ⁶C₃(x³)(1)³ + ⁶C₄(x²)(1)⁴ – ⁶C₅(x)(1)⁵ + ⁶C₆(1)⁶]

= ⁶C₀(x⁶) + ⁶C₁(x⁵)(1) + ⁶C₂(x⁴)(1) ² + ⁶C₃(x³)(1)³ + ⁶C₄(x²)(1)⁴ + ⁶C₅(x)(1)⁵ + ⁶C₆(1)⁶ + ⁶C₀(x⁶) – ⁶C₁(x⁵)(1) + ⁶C₂(x⁴)(1) ² – ⁶C₃(x³)(1)³ + ⁶C₄(x²)(1)⁴ – ⁶C₅(x)(1)⁵ + ⁶C₆(1)⁶

= 2 × [⁶C₀(x⁶) + ⁶C₂(x⁴)(1) ² + ⁶C₄(x²)(1)⁴ + ⁶C₆(1)⁶]

= 2 ×  $[\left(\frac{6!}{0!\left(6-0\right)!}\prime x^6\right)+\left(\frac{6!}{2!\left(6-2\right)!}\frac{6!}{2!\left(6-2\right)!}+x^4\prime 1\right)$ +  $\left(\frac{6!}{4!\left(6-4\right)!}\prime x^2\prime 1\right)+\left(\frac{6!}{6!\left(6-6\right)!}\prime 1\right)]$

= 2 × [(1 ×  $x^6$ ) +  $\left(\frac{\mathrm{6\prime }<br>\mathrm{5\prime }<br>4!}{\mathrm{2\prime } <br>\mathrm{1\prime }<br>4!}\prime x^4\right)+\left(\frac{\mathrm{6\prime }<br>\mathrm{5\prime }<br>4!}{4!\prime <br>2!}\prime x^2\right)$ + (1 × 1)]

= 2[  $x^6$ + 15  $x^4$ + 15  $x^2$ + 1]

Hence putting x = √2 $$ we have,

( √2 $$ + 1)⁶ + ( √2 $$ – 1)⁶

= 2 × [  ${(\surd 2)}^6$ + 15  ${(\surd 2)}^4$ + 15  ${(\surd 2)}^2$ + 1]

= 2 × [2³ + (15 x 2²) + (15 x 2) + 1]

= 2 × [8 + 60 + 30 + 1]

= 2 × 99

= 198

 

Q13. Show that 9ⁿ⁺¹– 8n – 9 is divisible by 64, whenever n is a positive integer.

A.13. For a number x to be divisible by y, we can write x as a factor of y i.e., x = ky where k is some natural number. Thus in order for 9ⁿ⁺¹ – 8n – 9 to be divisible by 64 we need to show that 9ⁿ⁺¹ – 8n – 9 = 64k where k is some natural number.

We have, by binomial theorem

(1 + a)^m = ^mC₀ + ^mC₁(a) + ^mC₂(a)² + ^mC₃(a)³ + ………… + ^mC_m(a)^m

Putting, a = 8 and m = n + 1

(1 + 8)ⁿ⁺¹ = ⁿ⁺¹C₀ + ⁿ⁺¹C₁.8 + ⁿ⁺¹C₂.8² + ⁿ⁺¹C₃.8³ + …….. + ⁿ⁺¹C_n+1.(8)ⁿ⁺¹

=> 9ⁿ⁺¹=  1 + (n + 1)8 + 8²×[ⁿ⁺¹C₂ + ⁿ⁺¹C₃.8 + ……….. + ⁿ⁺¹C_n+1.(8)^n+1–2]  [since, ⁿ⁺¹C₀ = 1, ⁿ⁺¹C₁= n + 1]

=> 9ⁿ⁺¹ = 1 + 8n + 8 + 64 × [ⁿ⁺¹C₂ + ⁿ⁺¹C₃.8 + ……….. + ⁿ⁺¹C_n+1.(8)^n+1-2]

=> 9ⁿ⁺¹ – 8n – 9 = 64 × [ⁿ⁺¹C₂ + ⁿ⁺¹C₃.8 + ……….. + 8^n–1][since, ⁿ⁺¹C_n+1 = 1]

=> 9ⁿ⁺¹ – 8n – 9 = 64k,

where k = ⁿ⁺¹C₂ + ⁿ⁺¹C₃.8 + ……….. + 8^n–1 is a natural number.

This shows that 9ⁿ⁺¹ – 8n – 9 is divisible by 64

 

Q14. Prove that ${\displaystyle \underset{r=0}{\overset{n}{\sum }}{3}^r{}^n{C}_r=4^n.}$

A.14.

By binomial theorem,

(a + b)ⁿ = ⁿC₀(a)ⁿ(b)⁰ + ⁿC₁(a)^n–1(b)¹ + ………….. + ⁿC_r(a)^n–r(b)^r + …………… + ⁿC_n(a)^n–n(b)ⁿ

Where, b⁰ = 1 = a^n–n

So, (a + b)ⁿ = ⁿC_r(a)^n–r(b)^r

Putting a = 1 and b = 3 such that a + b = 4 , we can rewrite the above equation as

(1 + 3)ⁿ = ⁿC_r(1)^n–r.3^r

=>4ⁿ = $\underset{r=0}{{\displaystyle \sum }}.3r$ .ⁿC_r

Hence proved.

 

Exercise 8.2

Find the coefficient of

Q1. x⁵in (x + 3)⁸

A.1. Let x⁵ occurs in (r + 1)^th term of the expansion (x + 3)⁸.

Now, T_r+1 = ⁸C_r $.x^{8-r}.3^r$

Comparing indices of x in T_r+1 with x⁵ we get,

8 – r = 5

=>r = 8 – 5 = 3

So, Co-efficient of x⁵ is

⁸C₃× 3³

=  $\frac{8!}{3!\left(8-3\right)!}$ × 27

=  $\frac{\mathrm{8\prime }<br>\mathrm{7\prime }<br>\mathrm{6\prime }<br>5!}{\mathrm{3\prime }<br>\mathrm{2\prime }<br>\mathrm{1\prime }<br>5!}$ × 27

= 56 × 27

= 1512

 

Q2. a⁵b⁷in (a – 2b)¹².

A.2. Let a⁵b⁷ occurs in (r + 1)^th term of [removed]a – 2b)¹².

Now, T_r+1 = ¹²C_ra^12-r (–2b)^r

= (–1)^r12C_ra^12–r . 2^r. b^r

Comparing indices of a and b in T_r-1 with a⁵ and b⁷ we get,

r = 7

So, co-efficient of a⁵b⁷ is (–1)⁷¹²C₇ 2⁷

= –1 ×  $\frac{12!}{7!\prime <br>\left(12-7\right)!}$ × 2⁷

= –1 ×  $\frac{1\mathrm{2\prime }<br>1\mathrm{1\prime }<br>1\mathrm{0\prime }<br>\mathrm{9\prime }<br>\mathrm{8\prime }<br>7!}{7!\prime <br>5!}$ × 128

= –1 ×  $\frac{1\mathrm{2\prime }<br>1\mathrm{1\prime }<br>1\mathrm{0\prime }<br>\mathrm{9\prime }<br>8}{\mathrm{5\prime }<br>\mathrm{4\prime }<br>\mathrm{3\prime }<br>\mathrm{2\prime }<br>1}$ × 128

= –1 × 792 × 128

= – 101376

Write the general term in the expansion of

 

Q3. (x²–y)⁶

A.3. Let (r + 1)^th term be the general term of (x²–y)⁶.

So, T_r-1 = ⁶C_r (x²)^6-r (-y)^r

= (–1)^r .⁶C_r . $x^{12-r}$ .  $y^r$

 

Q4.(  $x^2- yx){}^{12}$ , x≠ 0.

A.4. Let (r + 1)th be the general term of ( $x^2- yx){}^{12}$

So, T_r-1 = ¹²C_r (x²)^12–r (–yx)^r

= (–1)^r12C_rx^24–2ry^rx^r

= (–1)^r12C_r $x^{24-2r+r}{y}^r$

= (-1)^r12C­_r $x^{24-r}{y}^r$

 

Q5. Find the 4thterm in the expansion of (x – 2y)¹².

A.5. General term of the expansion ${\left(x-2y\right)}^{12}$ is given by

T_r+1 = ¹²C_r ${\left(x\right)}^{12-r}{\left(-2y\right)}^r$

For 4th term, r + 1 = 4 i.e., r = 3

Therefore, T₄ = T₃₊₁ = ¹²C₃ ${\left(x\right)}^{12-3}{\left(-2y\right)}^3$

=  $\frac{12!}{3!\left(12-3\right)!}$  $x^9{\left(-1\right)}^3{2}^3{y}^3$

=  $-\frac{1\mathrm{2\prime }<br>1\mathrm{1\prime }<br>1\mathrm{0\prime }<br>9!}{\mathrm{3\prime }<br>\mathrm{2\prime }<br>\mathrm{1\prime }<br>9!}$  ${\left(x\right)}^9$ × (8  $y){}^3$

= – 220 x⁹× 8y³

= – 1760x⁹y³

 

Find the middle terms in the expansions of

 

Q7.  ${\left(3-\frac{x^3}{6}\right)}^7$

A.7. As n = 7 is odd, the middle term are the ${\left(\frac{n+1}{2}\right)}^{th}$ term and  ${\left(\frac{n+1}{2}+ 1\right)}^{th}$ term. i.e.  ${\left(\frac{7+1}{2}\right)}^{th}$ and  ${\left(\frac{7+1}{2}+1\right)}^{th}$ term which is 4^th term and 5^th term. i.e. T₄ and T₅.

Hence, T₄ = T₃₊₁ = ⁷C₃(3)^7-3 ${\left(-\frac{x^3}{6}\right)}^3$

=  $\frac{7!}{3!\left(7-3\right)!}$ × 3⁴ (–1)³ $\left(\frac{x^{\mathrm{3\times }3}}{2^3.3^3}\right)$

=  $-\frac{\mathrm{7\prime }<br>\mathrm{6\prime }<br>\mathrm{5\prime }<br>4!}{\mathrm{3\prime }<br>\mathrm{2\prime }<br>\mathrm{1\prime }<br>4!}$ ×  $\frac{3^4}{3^3}$ ×  $\frac{x^9}{2^3}$

= – 35 ×  $\frac{3x^9}{8}$

=  $-\frac{105x^9}{8}$

T₅ = T₄₊₁ = ⁷C₄× (3)^7-4 ${\left(\frac{-x^3}{6}\right)}^4$

=  $\frac{7!}{4!\left(7-4\right)!}$ ×  $\frac{3^3\prime x^{12}}{2^4\prime 3^4}$

=  $\frac{\mathrm{7\prime }<br>\mathrm{6\prime }<br>\mathrm{5\prime }<br>4!}{4! \prime 3!}$ ×  $\frac{x^{12}}{2^4\prime 3}$

=  $\frac{\mathrm{7\prime }<br>\mathrm{6\prime }<br>5}{\mathrm{3\prime }<br>\mathrm{2\prime }<br>1}$ ×  $\frac{x^{12}}{1\mathrm{6\prime } <br>3}$

=  $\frac{35x^{12}}{48}$

 

Q8.  ${\left(\frac{x}{3}+9y\right)}^{10}.$

A.8. As n = 10 is even the middle term is ${\left(\frac{n}{2}+1\right)}^{th}$ i.e.  ${\left(\frac{10}{2}+1\right)}^{th}$ term i.e. 6th term.

So, T₆ = T₅₊₁ = ¹⁰C₅ ${\left(\frac{x}{3}\right)}^{10-5}$ (9y)⁵

=  $\frac{10!}{5!\left(10-5\right)!}$ ×  $\frac{x^5}{3^5}$ × 3^5x2y⁵

=  $\frac{1\mathrm{0\prime }<br>\mathrm{9\prime }<br>\mathrm{8\prime }<br>\mathrm{7\prime }<br>\mathrm{6\prime }<br>5!}{\mathrm{5\prime }<br>\mathrm{4\prime }<br>\mathrm{3\prime }<br>\mathrm{2\prime }<br>\mathrm{1\prime }<br>5!}$ × 3⁵x⁵y⁵

= 2 × 9 × 2 × 7 × 243 x⁵y⁵

= 61236 x⁵y⁵

 

Q9. In the expansion of (1 + a)^m+n,prove that coefficients of anand anare equal.

A.9. The general term of the expansion (1 + a)^m+n is

T_r+1 = ^m+nC_ra^r   [since, 1^m+n-r = 1]

At r = m we have,

T_m+1 = ^m+nC_ma^m

=  $\frac{\left(m+n\right)!}{m!\left(m+n-m\right)!}$ (a)^m

=  $\frac{\left(m+n\right)!}{m! n!}$ a^m --------------- (1)

Similarly at r = n we have,

T_n+1 = ^m+nC_naⁿ

=  $\frac{\left(m+n\right)!}{n!\left(m+n-n\right)!}$ (a)ⁿ

=  $\frac{\left(m+n\right)!}{n! m!}$ aⁿ --------------- (2)

Hence from (1) & (2),

Co-efficient of am = Co-efficient of aⁿ = $\frac{\left(m+n\right)!}{m!n!}$

 

Q10. The coefficients of the (r – 1)^th, r^thand (r + 1)^thterms in the expansion of (x + 1)ⁿ are in the ratio 1 : 3 : 5. Find n and r.

A.10. The general term of the expansion (x +1)ⁿ is

T_r+1 = ⁿC_rx^n–r1^r

i.e. co-efficient of  ${\left(r+1\right)}^{th}$ term = ⁿC_r

So, co-efficient of  ${\left(r-1\right)}^{th}$ term =ⁿC_{(r–1) – 1} = ⁿC_{r – 2}

Similarly, co-efficient of rth term = ⁿC_{r – 1}

Given that, ⁿC_{r – 2} :ⁿC_{r – 1} : ⁿC_r = 1 : 3 : 5

We have,

$\frac{\mathrm{ }}{}$

 

 =  $\frac{1}{3}$

=>  $\frac{n!}{\left(r-2\right)!\left(n-r+2\right)!}$ ×  $\frac{\left(r-1\right)!\left(n-r+1\right)!}{n!}$ =  $\frac{1}{3}$

=>  $\frac{\left(r-1\right)\left(r-2\right)!\left(n-r+1\right)!}{\left(r-2\right)!\left(n-r+2\right)\left(n-r+1\right)!}$ =  $\frac{1}{3}$

=>  $\frac{\left(r-1\right)}{\left(n-r+2\right)}$ =  $\frac{1}{3}$

=> 3r – 3 = n – r + 2

=> 3r + r = n + 2 + 3

=> 4r = n + 5 -------------- (1)

And,

 

 =  $\frac{3}{5}$

=>  $\frac{n!}{\left(r-1\right)!\left(n-r+1\right)!}$ ×  $\frac{r!\left(n-r\right)!}{n!}$ =  $\frac{3}{5}$

=>  $\frac{r\left(r-1\right)!\left(n-r\right)!}{\left(r-1\right)!\left(n-r+1\right)\left(n-r\right)!}$ =  $\frac{3}{5}$

=>  $\frac{r}{n-r+1}$ =  $\frac{3}{5}$

=> 5r = 3n – 3r + 3

=> 5r + 3r = 3n + 3

=> 8r = 3n + 3 ----------------------- (2)

Multiplying equation (1) by 2 and subtracting from equation (2) we get,

8r – 8r = 3n + 3 – (2n + 10)

=> 0 = 3n + 3 – 2n – 10

=> 0 = n – 7

=>n = 7

Putting n = 7 in equation (1) we get,

=> 4r = 7 + 5

=>r =  $\frac{12}{4}$

=>r = 3

 

Q11. Prove that the coefficient of xⁿin the expansion of (1 + x)²ⁿis twice the coefficientof xⁿin the expansion of (1 + x)^2n-1.

A.11. General term of the expansion (1 + x)²ⁿ is

T_r+1 = ²ⁿC_r (1)^2n-r(x)^r

So, co-efficient of xⁿ (i.e. r = n) is ²ⁿC_n

Similarly general term of the expansion (1 + x)^2n–1 is

T_r+1 = ^2n-1C_r (1)^2n–1–rx^r

And co-efficient of xⁿ i.e. when r = n is ^2n-1C_n

Therefore,

$\frac{co-efficient of x^n in {\left(1+x\right)}^{2n}}{co-efficient of x^n in {\left(1+x\right)}^{2n-1}}$

=

=  $\frac{2n!}{n!\left(2n-n\right)!}$ ÷  $\frac{\left(2n-1\right)!}{n!\left(2n-1-n\right)!}$

=  $\frac{2n!}{n!n!}$ ×  $\frac{n!\left(n-1\right)!}{\left(2n-1\right)!}$

=  $\frac{2\mathrm{n\prime } <br>\left(2-1\right)!}{n!\prime <br>\mathrm{n\prime }<br>\left(n-1\right)!}$ ×  $\frac{n!\left(n-1\right)!}{\left(2n-1\right)!}$

=  $\frac{2n}{n}$

= 2

Thus, co-efficient of  $x^n$ in  ${\left(1+x\right)}^{2n}$ = 2x co-efficient of  $x^n$ in  ${\left(1+x\right)}^{2n-1}$

 

Q.12. Find a positive value of m for which the coefficient of x²in the expansion (1 + x)^m is 6.

A.12. The general term of the expansion  ${\left(1+x\right)}^m$ is given by,

T_r+1 = ^mC_r $1^{m-r} x^r$

= ^mC_rx^r

At r = 2,

T₂₊₁ = ^mC₂x²

Given that, co-efficient of x² = 6

=>^mC₂ = 6

=>  $\frac{m!}{2!\left(m-2\right)!}$ = 6

=>  $\frac{\mathrm{m\prime } <br>(m-1)\prime <br>\left(m-2\right)!}{\mathrm{2\prime } <br>\mathrm{1\prime }<br>\left(m-2\right)!}$ = 6

=>  $\frac{\mathrm{m\prime } <br>\left(m-1\right)}{2}$ = 6

=>m² – m = 12

=>m² – m – 12 = 0

=>m² + 3m – 4m – 12 = 0

=>m(m + 3) – 4(m+ 3) = 0

=> (m – 4)(m + 3) = 0

=>m = 4 and m = –3

Since, we need a positive value of m we have,

m = 4

 

Miscellaneous Exercise

Q1. Find a, b and n in the expansion of (a + b)ⁿ if the first three terms of the expansion are 729, 7290 and 30375, respectively.

A.1.The general term of the expansion (a + b)ⁿ is given by

T_{r +1} = ⁿC_ra^n–rb^r

So, T₁ = ⁿC₀aⁿ = aⁿ

T₂ = ⁿC₁a^n-1b = $\frac{n!}{1!\left(n-1\right)!}$ a^n-1 b = $\frac{n\times \left(n-1\right)!}{\left(n-1\right)!}$ a^n-1b = na^n-1b

T₃ = ⁿC₂a^n-2b² = $\frac{n!}{2!\left(n-2\right)[!}$ a^n-2b² = $\frac{n \times \left(n-1\right)\times \left(n-2\right)!}{2\times 1\times \left(n-2\right)!}$ a^n-2b² = $\frac{n\left(n-1\right)}{2}$ a^n-2b²

Given,

T₁ = 729

=>aⁿ = 729 ------------------ (1)

T₂ = 7290

=>na^n–1b = 7290 ------------- (2)

T₃ = 30375

=>  $\frac{n\left(n-1\right)}{2}$ a^n–2b² = 30375 ------------------- (3)

Dividing equation (2) by (1) we get,

$\frac{n{a}^{n-1}b}{a^n}$ =  $\frac{7290}{729}$

=>  $\frac{nb}{a}$ = 10

Similarly dividing equation (3) by (2) we get,

$\frac{n\left(n-1\right)}{2}$ a^n–2b² ÷ na^n–1b = $\frac{30375}{7290}$

=>  $\frac{n\left(n-1\right)}{2}$ a^n–2b²×  $\frac{1}{n{a}^{n-1}b}$ =  $\frac{30375}{7290}$

=>  $n\left(n-1\right)a^{n-2}{b}^2$ ×  $\frac{1}{n{a}^{n-1}b}$ =  $\frac{30375}{7290}$ × 2

=>  $\frac{\left(n-1\right)b}{a}$ =  $\frac{2\mathrm{5\prime } \times 2}{6}$

=>  $\frac{nb}{a}$  $-\frac{b}{a}$ =  $\frac{25}{3}$

=> 10 –  $\frac{b}{a}$ =  $\frac{25}{3}$ [since,  $\frac{nb}{a}$ = 10]

=>  $\frac{b}{a}$ = 10 –  $\frac{25}{3}$

=  $\frac{30-25}{3}$

=  $\frac{5}{3}$ --------------- (5)

Putting equation (5) in (4) we get,

n×  $\frac{5}{3}$ = 10

=>n = 10 ×  $\frac{3}{5}$

=>n = 6

So putting the value of n in equation (1) we get,

a6 = 729

=>a⁶= 36

=>a = 3

And putting a = 3 in equation (5) we get,

$\frac{b}{a}$ =  $\frac{5}{3}$

=>b =  $\frac{5}{3}$ ×a

=  $\frac{5}{3}$ × 3

= 5

 

Q2. Find a if the coefficients of x²and x³in the expansion of (3 + ax)⁹are equal.

A.2. The general term of the expansion  ${\left(3+ax\right)}^9$ is

T_r+1 = ⁹C_r $3^{\left(9-r\right)}$  ${\left(ax\right)}^r$

= ⁹C_r $3^{\left(9-r\right)}{a}^r{x}^r$

At r = 2,

T₂₊₁ = ⁹C₂ $3^{\left(9-2\right)}{a}^2{x}^2$

=  $\frac{9!}{2!\left(9-2\right)!}$ 3⁷a²x²

=  $\frac{\mathrm{9\prime }<br>\mathrm{8\prime }<br>7!}{\mathrm{2\prime }<br>\mathrm{1\prime }<br>7!}$ 3⁷a²x²

= 36 ×3⁷a²x²

At r = 3,

T₃₊₁ = ⁹C₃ $3^{\left(9-3\right)}{a}^3{x}^3$

=  $\frac{9!}{3!\left(9-3\right)!}$ 3⁶a³x³

=  $\frac{\mathrm{9\prime }<br>\mathrm{8\prime }<br>\mathrm{7\prime }<br>6!}{\mathrm{3\prime } <br>\mathrm{2\prime }<br>\mathrm{1\prime }<br>6!}$ 3⁶a³x³

= 84 ×3⁶a³x³

Given that,

Co-efficient of  $x^2$ = co-efficient of  $x^3$

=> 36 ×  $3^7{a}^2$ = 84 ×  $3^6{a}^3$

=>  $\frac{a^3}{a^2}$ =  $\frac{3\mathrm{6\prime }<br>3^7}{8\mathrm{4\prime } <br>3^6}$

=>  $a$ =  $\frac{\mathrm{3\prime }<br>3}{7}$

=  $\frac{9}{7}$

 

Q3. Find the coefficient of x5in the product (1 + 2x)⁶(1 – x)⁷using binomial theorem.

A.3. We first expand each of the factors of the given product using Binomial theorem. We have,

(1 + 2x)⁶= ⁶C₀ (1)⁶  +  ⁶C₁ (1)⁵(2x)  +  ⁶C₂ (1)⁴(2x)²  +  ⁶C₃ (1)³(2x)³  +  ⁶C₄ (1)²(2x)⁴  +  ⁶C₅ (1)(2x)⁵  +  ⁶C₆(2x)⁶

=  $\left[\frac{6!}{0!\left(6-0\right)!}\prime 1\right]$ +  $\left[\frac{6!}{1!\left(6-1\right)!}\prime 1\prime \left(2x\right)\right]$ +  $\left[\frac{6!}{2!\left(6-2\right)!}\prime 1\prime 4x^2\right]$ +  $\left[\frac{6!}{3!\left(6-3\right)!}\prime 1\prime 8x^3\right]$ +  $\left[\frac{6!}{4!\left(6-4\right)!}\prime 1\prime 16x^4\right]$ +  $\left[\frac{6!}{5!\left(6-5\right)!}\prime 1\prime 32x^5\right]$ +  $\left[\frac{6!}{6!\left(6-6\right)!}\prime 64x^6\right]$

= [1] +  $\left[\frac{\mathrm{6\prime } <br>5!}{\mathrm{1\prime } <br>5!}\prime (2x)\right]$ +  $\left[\frac{\mathrm{6\prime }<br>\mathrm{5\prime }<br>4!}{\mathrm{2\prime }<br>\mathrm{1\prime }<br>4!}\prime 4x^2\right]$ +  $\left[\frac{\mathrm{6\prime }<br>\mathrm{5\prime }<br>\mathrm{4\prime }<br>3!}{\mathrm{3\prime } <br>\mathrm{2\prime }<br>\mathrm{1\prime }<br>3!}\prime \left(8x^3\right)\right]$ +  $\left[\frac{\mathrm{6\prime }<br>\mathrm{5\prime }<br>4!}{4!\prime <br>2!}\prime 16x^4\right]$ +  $\left[\frac{\mathrm{6\prime }<br>5!}{5!\prime <br>1!}\prime (32x^5)\right]$ + [1 ×64x6]

= 1 + 12  $x$ + 60x² + 160x³ + 240x⁴ + 192x⁵ + 64x⁶

And,

(1 – x)⁷= ⁷C₀ (1)⁷  +  ⁷C₁ (1)⁶(–x) + ⁷C₂ (1)⁵(–x)² + ⁷C₃ (1)⁴(–x)³  +  ⁷C₄ (1)³(–x)⁴  +  ⁷C₅ (1)²(–x)⁵  +  ⁷C₆ (1) (–x)⁶ + ⁷C₇(–x)⁷

=  $\left[\frac{7!}{0!\left(7-0\right)!}\prime 1\right]$ +  $\left[\frac{7!}{1!\left(7-1\right)!}\prime 1\prime \left(-x\right)\right]$ +  $\left[\frac{7!}{2!\left(7-2\right)!}\prime 1\prime x^2\right]$ +  $\left[\frac{7!}{3!\left(7-3\right)!}\prime 1\prime {\left(–x\right)}^{}\right]$ +  $\left[\frac{7!}{4!\left(7-4\right)!}\prime 1\prime x^4\right]$ +  $\left[\frac{7!}{5!\left(7-5\right)!}\prime 1\prime {\left(-x\right)}^5\right]$ +  $\left[\frac{7!}{6!\left(7-6\right)!}\prime 1\prime x^6\right]$ + [  $\left[\frac{7!}{7!\left(7-7\right)!}\prime {\left(-x\right)}^7\right]$

= [1] +  $\left[\frac{\mathrm{7\prime } <br>6!}{\mathrm{1\prime } <br>6!}\prime \left(-x\right)\right]$ +  $\left[\frac{\mathrm{7\prime } <br>\mathrm{6\prime }<br>5!}{\mathrm{2\prime }<br>\mathrm{1\prime }<br>5!}\prime x^2\right]$ +  $\left[\frac{\mathrm{7\prime }<br>\mathrm{6\prime }<br>\mathrm{5\prime }<br>4!}{\mathrm{3\prime } <br>\mathrm{2\prime }<br>\mathrm{1\prime }<br>4!}\prime (-x^3)\right]$ +  $\left[\frac{\mathrm{7\prime } <br> \mathrm{6\prime }<br>\mathrm{5\prime }<br>4!}{4!\prime <br>3!}\prime x^4\right]$ +  $\left[\frac{\mathrm{7\prime }<br>\mathrm{6\prime }5!}{5!\prime <br>2!}\prime \left(-x^5\right)\right]$ +  $\left[\frac{\mathrm{7\prime }<br>6!}{6!\prime <br>1!}\prime x^6\right]$ + [1 ×(–x)7]

= 1 – 7x + 21x² – 35x³ + 35x⁴– 21x⁵ + 7x⁶– x⁷

Thus,  ${\left(1+2x\right)}^6{\left(1-x\right)}^7$

= (1 + 12x + 60x² + 160x³ + 240x⁴ + 192x⁵ + 64x⁶)( 1 – 7x + 21x² – 35x³ + 35x⁴– 21x⁵ + 7x⁶– x⁷)

We need to find only the term involving x⁵ i.e. x^rx^{5 – r}

The terms with x⁵ are

= (1)(–21x⁵) + (12x)(35x⁴) + (60x²)(–35x³) + (160x³)(21x²) + (240x⁴)(–7x) + (192x⁵)(1)

= –21x⁵ + 420x⁵– 2100x⁵ + 3360x⁵– 1680x⁵ + 192x⁵

= 3972x⁵– 3801x⁵

= 171x⁵

Hence, co-efficient of x⁵ is 171.

 

Q4. If a and b are distinct integers, prove that a – b is a factor of aⁿ– bⁿ, whenever n is a positive integer.

[Hint: write aⁿ= (a – b + b)n and expand]

A.4. For (a – b) to be a factor of aⁿ – b ⁿwe need to show (aⁿ – bⁿ) = (a – b)k as k is a natural number.

We have, for positive n

aⁿ = ${(a-b+b)}^n$ =  ${\left[\left(a-b\right)+b\right]}^n$

=>aⁿ = ⁿC₀(a – b)ⁿ + ⁿC₁(a – b)^{n -1}b + ⁿC₂(a – b)^{n – 2}b² + ………… +ⁿC_n-1  $\left(a-b\right) b^{n-1}$ + ⁿC_nbⁿ

=>aⁿ= ${\left(a-b\right)}^n$ + ⁿC₁ ${\left(a-b\right)}^{n-1} b$ + ⁿC₂ ${\left(a-b\right)}^{n-2} b^2$ + …………….…+ ⁿC_n-1 $\left(a-b\right) b^{n-1}$ +  $b^n$ [Since, ⁿC₀ = 1 and ⁿC_n = 1]

=>  $a^n-b^n$ =  ${\left(a-b\right)}^n$ +ⁿC₁  ${\left(a-b\right)}^{n-1} b$ + ⁿC₂ ${\left(a-b\right)}^{n-2} b^2$ + ……………… + ⁿC_n-1 $\left(a-b\right) b^{n-1}$

=>  $a^n-b^n$ =  $\left(a-b\right)$ [  ${\left(a-b\right)}^{n-1}$ + ⁿC₁ ${\left(a-b\right)}^{n-2} b$ + ⁿC₂ ${\left(a-b\right)}^{n-3} b^2$ +………..…… + ⁿC_n-1 $b^{n-1}$ ]

=>  $a^n-b^n$ =  $\left(a-b\right)$ k where k = [  ${\left(a-b\right)}^{n-1}$ + ⁿC₁ ${\left(a-b\right)}^{n-2} b$ + ⁿC₂ ${\left(a-b\right)}^{n-3} b^2$ +………..…… + ⁿC_n-1 $b^{n-1}$ ] is a natural number.

Therefore (a – b) is a factor of aⁿ – bⁿwhere n is positive integer.

 

 

Q7. Find an approximation of (0.99)⁵using the first three terms of its expansion.

A.7. (0.99)⁵ = (1 – 0.01)⁵

By binomial theorem expanding upto first three terms, we get

(1 – 0.01)⁵ = ⁵C₀ (1)⁵ + ⁵C₁ (1)⁴(-0.01) + ⁵C₂ (1)³(-0.01)²

=  $\left(\frac{5!}{0!\left(5-0\right)!}\prime 1\right)$ +  $\left[\frac{5!}{1!\left(5-1\right)!}\prime 1\prime \left(-0.01\right)\right]$ +  $\left(\frac{5!}{2!\left(5-2\right)!}\prime 1\prime 0.0001\right)$

= (1 × 1) –  $\left(\frac{\mathrm{5\prime }<br>4!}{\mathrm{1\prime }<br>4!}\prime 1\prime 0.01\right)$ +  $\left(\frac{\mathrm{5\prime }<br>\mathrm{4\prime }<br>3!}{\mathrm{2\prime }<br>\mathrm{1\prime }<br>3!}\prime 1\prime 0.0001\right)$

= 1 – 0.05 + 0.001

= 1.001 – 0.05

= 0.951

 

Q9. Expand using Binomial Theorem ${\left(1+\frac{x}{2}-\frac{2}{x}\right)}^4,x\ne 0.$

A.9.  ${\left(1+ \frac{x}{2}-\frac{2}{x}\right)}^4$

Using binomial theorem we have,

${\left[\left(1+\frac{x}{2}\right)-\frac{2}{x}\right]}^4$

= ⁴C₀ ${\left(1+\frac{x}{2}\right)}^4$ + ⁴C₁ ${\left(1+\frac{x}{2}\right)}^3\left(-\frac{2}{x}\right)$ + ⁴C₂ ${\left(1+\frac{x}{2}\right)}^2{\left(-\frac{2}{x}\right)}^2$ + ⁴C₃ $\left(1+\frac{x}{2}\right){\left(-\frac{2}{x}\right)}^3$ + ⁴C₄ ${\left(-\frac{2}{x}\right)}^4$

=  $\left[\frac{4!}{0!\left(4-0\right)!}{\left(1+\frac{x}{2}\right)}^4\right]$ –  $\left[\frac{4!}{1!\left(4-1\right)!}{\left(1+\frac{x}{2}\right)}^3\left(\frac{2}{x}\right)\right]$ +  $\left[\frac{4!}{2!\left(4-2\right)!}{\left(1+\frac{x}{2}\right)}^2\left(\frac{4}{x^2}\right)\right]$ –  $\left[\frac{4!}{3!\left(4-3\right)!}\left(1+\frac{x}{2}\right)\left(\frac{8}{x^3}\right)\right]$ +  $\left[\frac{4!}{4!\left(4-4\right)!} \left(\frac{16}{x^4}\right)\right]$

=  $\mathrm{1\prime }<br>{\left(1+\frac{x}{2}\right)}^4$ –  $\left[{\left(1+\frac{x}{2}\right)}^3\left(\frac{2}{x}\right)\right]$ +  $\left[\frac{\mathrm{4\prime }<br>\mathrm{3\prime }<br>2!}{\mathrm{2\prime }<br>\mathrm{1\prime }<br>2!}{\left(1+\frac{x}{2}\right)}^2\left(\frac{4}{x^2}\right)\right]$ –  $\left[\frac{43!}{3!1!}\left(1+\frac{x}{2}\right)\left(\frac{8}{x^3}\right)\right]$ +  $\left[\mathrm{1\prime }<br>\left(\frac{16}{x^4}\right)\right]$

=  ${\left(1+\frac{x}{2}\right)}^4$ – 4  $\left[{\left(1+\frac{x}{2}\right)}^3\left(\frac{2}{x}\right)\right]$ + 6  $\left[{\left(1+\frac{x}{2}\right)}^2\left(\frac{4}{x^2}\right)\right]$ – 4  $\left[\left(1+\frac{x}{2}\right)\left(\frac{8}{x^3}\right)\right]$ +  $\left(\frac{16}{x^4}\right)$ ----- (1)

Now,

${\left(1+\frac{x}{2}\right)}^4$

= ⁴C₀(1)⁴ + ⁴C₁ (1)³ $\left(\frac{x}{2}\right)$ + ⁴C₂(1)² ${\left(\frac{x}{2}\right)}^2$ + ⁴C₃(1) ${\left(\frac{x}{2}\right)}^3$ + C₄ ${\left(\frac{x}{2}\right)}^4$

=  $\left(\frac{4!}{0!\left(4-0\right)!}\prime 1\right)$ +  $\left(\frac{4!}{1!\left(4-1\right)!}\prime \mathrm{1\prime }<br>\frac{x}{2}\right)$ +  $\left(\frac{4!}{2!\left(4-2\right)!}\prime \mathrm{1\prime }<br>\frac{x^2}{4}\right)$ +  $\left(\frac{4!}{3!\left(4-3\right)!}\prime \mathrm{1\prime }<br>\frac{x^3}{8}\right)$ +  $\left(\frac{4!}{4!\left(4-4\right)!}\prime \mathrm{1\prime }<br>\frac{x^4}{16}\right)$

= 1 +  $\left(\frac{\mathrm{4\prime }<br>3!}{\mathrm{1\prime }<br>3!}\prime \frac{x}{2}\right)$ +  $\left(\frac{\mathrm{4\prime }<br>\mathrm{3\prime }<br>2!}{\mathrm{2\prime }<br>\mathrm{1\prime }<br>2!}\prime \frac{x^2}{4}\right)$ +  $\left(\frac{\mathrm{4\prime }<br>3!}{3!1!}\prime \frac{x^3}{8}\right)$ +  $\left(\mathrm{1\prime }<br>\frac{x^4}{16}\right)$

= 1 + 2x +  $\frac{3x^2}{2}$ +  $\frac{x^3}{2}$ +  $\frac{x^4}{16}$ ------------------- (2)

${\left(1+\frac{x}{2}\right)}^3$

= ³C₀(1)³ + ³C₁ (1)² $\left(\frac{x}{2}\right)$ +³C₂ (1)  ${\left(\frac{x}{2}\right)}^2$ + ³C₃ ${\left(\frac{x}{2}\right)}^3$

=  $\left(\frac{3!}{0!\left(3-0\right)!}\prime 1\right)$ +  $\left(\frac{3!}{1!\left(3-1\right)!}\prime \mathrm{1\prime }<br>\frac{x}{2}\right)$ +  $\left(\frac{3!}{2!\left(3-2\right)!}\prime \mathrm{1\prime }<br>\frac{x^2}{4}\right)$ +  $\left(\frac{3!}{3!\left(3-3\right)!}\prime \mathrm{1\prime }<br>\frac{x^3}{8}\right)$

= 1 +  $\left(\frac{\mathrm{3\prime }<br>2!}{\mathrm{1\prime }<br>2!}\prime \frac{x}{2}\right)$ +  $\left(\frac{\mathrm{3\prime }<br>2!}{2!1!}\prime \frac{x^2}{4}\right)$ +  $\left(\mathrm{1\prime }<br>\frac{x^3}{8}\right)$

= 1 +  $\frac{3x}{2}$ +  $\frac{3x^2}{4}$ +  $\frac{x^3}{8}$ ------------------------ (3)

And,  ${\left(1+\frac{x}{2}\right)}^2$

= ²C₀(1)² + ²C₁ (1) $\left(\frac{x}{2}\right)$ + ²C₂ ${\left(\frac{x}{2}\right)}^2$

=  $\left(\frac{2!}{0!\left(2-0\right)!}\prime 1\right)$ +  $\left(\frac{2!}{1!\left(2-1\right)!}\prime \mathrm{1\prime }<br>\frac{x}{2}\right)$ +  $\left(\frac{2!}{2!\left(2-2\right)!}\prime \frac{x2}{4}\right)$

= 1 +  $\left(\frac{\mathrm{2\prime } <br>1}{\mathrm{1\prime }<br>1!}\prime \mathrm{1\prime }<br>\frac{x}{2}\right)$ +  $\frac{x^2}{4}$

= 1 + x +  $\frac{x^2}{4}$ ---------------------------- (4)

Putting (2), (3) and (4) in (1) we get,

${\left(1+ \frac{x}{2}-\frac{2}{x}\right)}^4$

= 1 + 2x +  $\frac{3x^2}{2}$ +  $\frac{x^3}{2}$ +  $\frac{x^4}{16}$ – 4  $\left[\left(1+\frac{3x}{2}+\frac{3x^2}{2}+\frac{x^3}{2}\right)\left(\frac{2}{x}\right)\right]$ + 6  $\left[\left(1+x+\frac{x^2}{4}\right)\left(\frac{4}{x^2}\right)\right]$ – 4  $\left[\left(1+\frac{x}{2}\right)\left(\frac{8}{x^3}\right)\right]$ +  $\left(\frac{16}{x^4}\right)$

= 1 + 2x +  $\frac{3x^2}{2}$ +  $\frac{x^3}{2}$ +  $\frac{x^4}{16}$ –  $\frac{8}{x}$ – 12 – 6x –x² +  $\frac{24}{x^2}$ +  $\frac{24}{x}$ + 6 –  $\frac{32}{x^3}$ –  $\frac{16}{x^2}$ +  $\frac{16}{x^4}$

=  $\frac{16}{x}$ +  $\frac{8}{x^2}$ –  $\frac{32}{x^3}$ +  $\frac{16}{x^4}$ – 4x+  $\frac{x^2}{2}$ +  $\frac{x^3}{2}$ +  $\frac{x^4}{16}$ – 5

 

Q10. Find the expansion of (3x²– 2ax + 3a²)³using binomial theorem.

A.10.  ${\left[3x^2-2ax+3a^2\right]}^3$

=  ${\left[3x^2+a\left(-2x+3a\right)\right]}^3$

We know that by binomial theorem,

${(a+b)}^3$ =  $a^3+b^3+3ab\left(a+b\right)$

=  $a^3+b^3+3a^{2}b+3a{b}^2$

Then,

${\left[3x^2+a\left(-2x+3a\right)\right]}^3$

= (3x²)³ + ${\left[a\left(-2x+3a\right)\right]}^3$ +  $\left[3(3x^2){}^2 a\left(-2x+3a\right)\right]$ +  $\left[ 3(3x^2){\left\{a\left(-2x+3a\right)\right\}}^2\right]$

= 27x⁶ + $\left[a^3{\left(-2x+3a\right)}^3\right]$ +  $\left[3(9x^4)\left(-2ax+3a^2\right)\right]$ +  $\left[3(3x^2)\left\{a^2{\left(3a-2x\right)}^2\right\}\right]$

= 27x⁶ + $\left[a^3\left\{-8x^3+27a^3+3\left(4x^2\right)\left(3a\right)+3\left(-2x\right)\left(9a^2\right)\right\}\right]$ +  $\left[-54a{x}^5+81a^2{x}^4\right]$ + [  $\left(9a^2{x}^2\right)$  $\left(9a^2+4x^2-12ax\right)$ ]

= 27x⁶ + [ $-8a^3{x}^3+27a^6+36a^4{x}^2-54a^{5}x$ ] + [  $-54a{x}^5+81a^2{x}^4$ ] + [  $(81a^4{x}^2+36a^2{x}^4-108a^3{x}^3$ ]

= 27x⁶ $- 8a^3{x}^3+27a^6+36a^4{x}^2-54a^{5}x$  $- 54a{x}^5+81a^2{x}^4$ +  $81a^4{x}^2+36a^2{x}^4-108a^3{x}^3$

= 27x⁶– 54ax⁵ $+ 117a^2{x}^4$  $- 116a^3{x}^3$ +  $117a^4{x}^2$  $- 54a^{5}x$  $+ 27a^6$