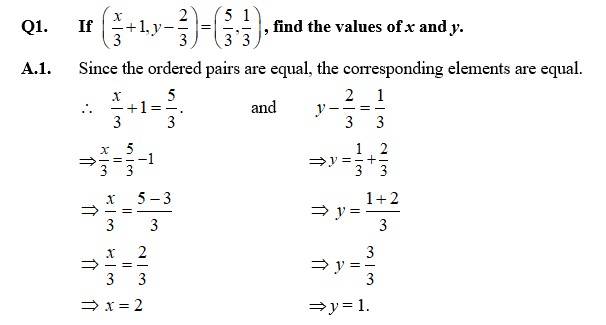
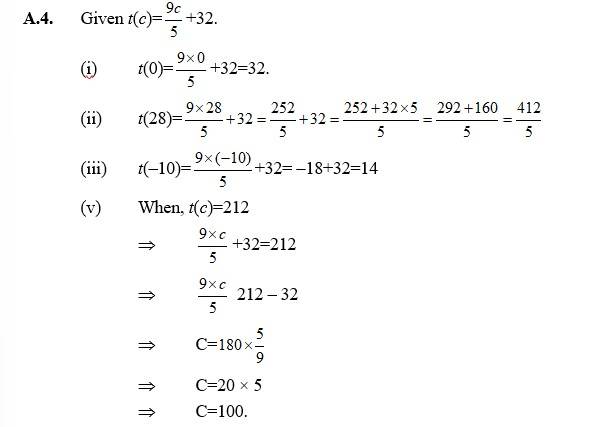Salviya AntonySenior Executive - Content
NCERT Solutions Class 11 Maths Relations and Functions: We have provided Class 11 Mathematics NCERT Solutions Chapter 2 Relations and Functions on this page. Students are recommended to learn the NCERT Solutions Class 11 Maths Relations and Functions. This is a chapter of great importance as far as the Class 11 board exams and other engineering entrance exams are concerned. The Class 11 NCERT Maths Chapter 2 Solutions Relations and Functions are designed by our team of expert teachers. This chapter contains 2 exercises and miscellaneous exercises. The NCERT Solutions Class 11 Maths Relations and Functions solutions are created in a step by step manner with detailed explanation. Practicing the NCERT Solutions help students to achieve better results. Students can access Class 11 Maths Relations and Functions for free.
A relation R from a set A to a set B is a subset of the Cartesian Product A × B obtained by describing a relationship between the first element x and the second element y of the ordered pairs in A × B. A function f from a set A to a set B is a specific type of relation for which every element x of set A has one and only one image y in set B. Students can check the NCERT Solutions Maths Class 11 Chapter 2 Relations and Functions for free. Also check the NCERT Class 11 Solutions Maths.
NCERT Solutions Class 11 Maths Relations and Functions: Solutions and FAQs
Exercise 2.1
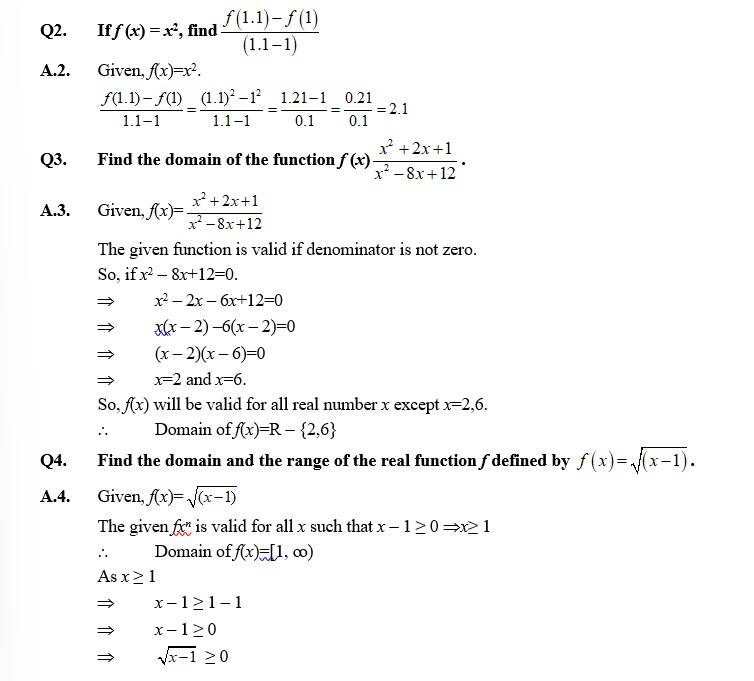
Q2. If the set A has 3 elements and the set B = {3, 4, 5}, then find the number of elements in (A×B).
A.2. Given, n(A) = 3
n(B) = 3 or B = {3,4,5}
So, number of elements in A× B = n(A× B) = n(A)× n(B) = 3 ×3 = 9.
Q3. If G = {7, 8} and H = {5, 4, 2}, find G × H and H × G.
A.3. Given, G = {7, 8} and H = {5, 4, 2}
By the definition of the Cartesian product,
G ×H = {(x, y): x∈G and y = ∈ H}
= {((7, 5), (7, 4), (7, 2), (8, 5),(8,4), (8,2)}
H× G = {(x, y): x∈ H and y ∈G}
= {(5, 7), (5, 8), (4,7), (4, 8), (2, 7), (2,8)}
Q4. State whether each of the following statements are true or false. If the statement is false, rewrite the given statement correctly.
(i) If P = {m, n} and Q = { n, m}, then P × Q = {(m, n),(n, m)}.
(ii) If A and B are non-empty sets, then A × B is a non-empty set of ordered pairs (x, y) such that x A and y B.
(iii) If A = {1, 2}, B = {3, 4}, then A ×
A.4. (i) False. Here P = {m, n}, n(p)=2
Q = {n, m}, n(Q)=2
n(P× Q) = n(P)× n(Q) = 2× 2 = 4.
So, P ×Q = {((m, n),(m, m),(n, n),(n, m)}
(ii) True.
(iii) True. { A ×(B
= n(A) ×0 {∵
=
Q5. If A = {–1, 1}, find A × A × A.
A.5. Given, A = {1,1}
So, A× A = {(1,1),(1,1),(1,1),(1,1)}
A ×A ×A = {(1,1),(1,1),(1,1),(1,1)} ×{1,1}
= {(1,1.1),(1, 1, 1),(1, 1, 1,),(1,1,1), (1,1,1), (1, 1,1), (1, 1,1), (1,1,1)}
Q6. If A × B = {(a, x),(a , y), (b, x), (b, y)}. Find A and B.
A.6. Given,
A ×B = {(a, x),(a, y),(b, x),(b, y)}
We know that,
A ×B = {(p, q); p ∈ A and q ∈ B}
So, A = {a, b} and B = {x, y}.
Q7. Let A = {1, 2}, B = {1, 2, 3, 4}, C = {5, 6} and D = {5, 6, 7, 8}. Verify that (i) A × (B∩ C) = (A × B)∩ (A × C). (ii) A × C is a subset of B × D.
A.7.Given,
A={1, 2}, B = {1,2,3,4}, C={5,6} and D={5,6,7,8}
(i)L.H. S = A ×(B∩ C) = {1,2} [{1,2,3,4} ∩{5,6}]
={1,2}×
=
R.H.S = (A× B)∩ (A ×C)=[{1,2}×{1,2,3,4}]∩[{1,2} {5,6}]
=[{(1 , 1), (1, 2), (1, 3), (1, 4), (2, 1), (2, 2), (2, 3), (2, 4)]∩ [{1,5),(1,6),(2,5), (2,6)}]
=
Hence, L.H.S= R.H.S.
(ii)
A× C ={1, 2}× {5,6}
={(1,5),(1,6),(2,5),(2,6)}
B× D ={1,2,3,4} ×{5,6,7,8}
={(1,5),(1,6),(1,7),(1,8),(2,5),(2,6),(2,7),(2,8),(3,5),(3,6),(3,7),(3,8),(4,5),(4,6),(4,7),(4,8)}
As every element of A C is also an element of B× D.
A ×C B ×D
Q8. Let A = {1, 2} and B = {3, 4}. Write A × B. How many subsets will A × B have? List them.
A.8. A Given, A={1,2}
B={3,4}
So, A× B={(1,3),(1,4),(2,3),(2, 4)}
i.e., n(A ×B)=4
A ×B will have subset =24=16.They are,
Φ,{(1,3)},{(1,4)},{(2, 3)},{(2,4)},{(1,3),(1,4)},{(1,3),(2,3)},
{(1,3),(2, 4)},{(1,4),(2, 3)},{(1,4),(2, 4)}, {(2,3),(2, 4)},
{(1,3),(1,4),(2, 3)},{(1,3),(1,4),(2,4)}, {(1,3),(2,3),(2, 4)},{(1,4),(2,3),(2,4)},
and {(1,3),(1,4),(2,3),(2,4)}
Q9. Let A and B be two sets such that n(A) = 3 and n(B) = 2. If (x, 1), (y, 2), (z, 1) are in A × B, find A and B, where x, y and z are distinct elements.
A.9. Given, n(A)=3
n(B)= 2
So, n(Ax B)=n(A).n(B)=3x 2=6
as (x, 1),(y, 2),(z, 1) ∈Ax B={(x, y), x∈Aand y∈B}.
A={x, y, z} and B={1,2}
As n(A) = 3as n(B) = 2
Q10. The Cartesian product A × A has 9 elements among which are found (–1, 0) and (0,1). Find the set A and the remaining elements of A × A.
A.10. Given, n(A × A)=9
n(A) ×n(A) = 9.
n(A)2 = 32.
n(A) = 3 .
And (–1,0),(0,1) A × A i.e., A × A = {(x, y), x A, y B}
⸫A={–1,0,1}
And A × A={–1,0,1} × {–1,0,1}
={(–1, –1),( –1,0),( –1,1),(0, –1),(0,0),(0,1),(1, –1),(1,0),(1,1)}
Exercise 2.2
Q1. Let A = {1, 2, 3,...,14}. Define a relation R from A to A by R = {(x, y) : 3x – y = 0, where x, y A}. Write down its domain, co domain and range.
A.1. Given,A ={1,2,3, …, 14}
R ={(x, y): 3x – y = 0; x, y A}
={(x, y): 3x = y; x, y A}.
= {(1,3),(2,6),(3,9),(4,12)}
Domain of R is the set of all the first elements of the ordered pairs in R
So, domain of R={1,2,3,4}
Codomain of R is the whole set A.
So, codomain of R={1,2,3, …, 14}
Range of R is the set of all the second elements of the ordered pains in R.
So, range of R={3,6,9,12}
Q2. Define a relation R on the set N of natural numbers by R = {(x, y) : y = x + 5, x is a natural number less than 4; x, y N}. Depict this relationship using roster form. Write down the domain and the range.
A.2. Given,R ={(x, y): y = x + 5, x is a natural number less than 4; x, y N}
={(x, y): y = x + 5; x, y N and x < 4}.
={(1,1+5), (2,2+5), (3,3+5)}
={(1,6), (2,7), (3,8)}
So, domain of R = {1,2,3}
range of R = {6,7,8}
Q3. A = {1, 2, 3, 5} and B = {4, 6, 9}. Define a relation R from A to B by R = {(x, y): the difference between x and y is odd; x A, y B}. Write R in roster form.
A.3. Given,A={1,2,3,5}
B={4,6,9}
R={(x, y) : the difference of x & y is odd; x A, y B}.
={(x, y):|x – y| is odd and x A, y B}
={(1,4),(1,6),(2,9),(3,4),(3,6),(5,4),(5,6)}.
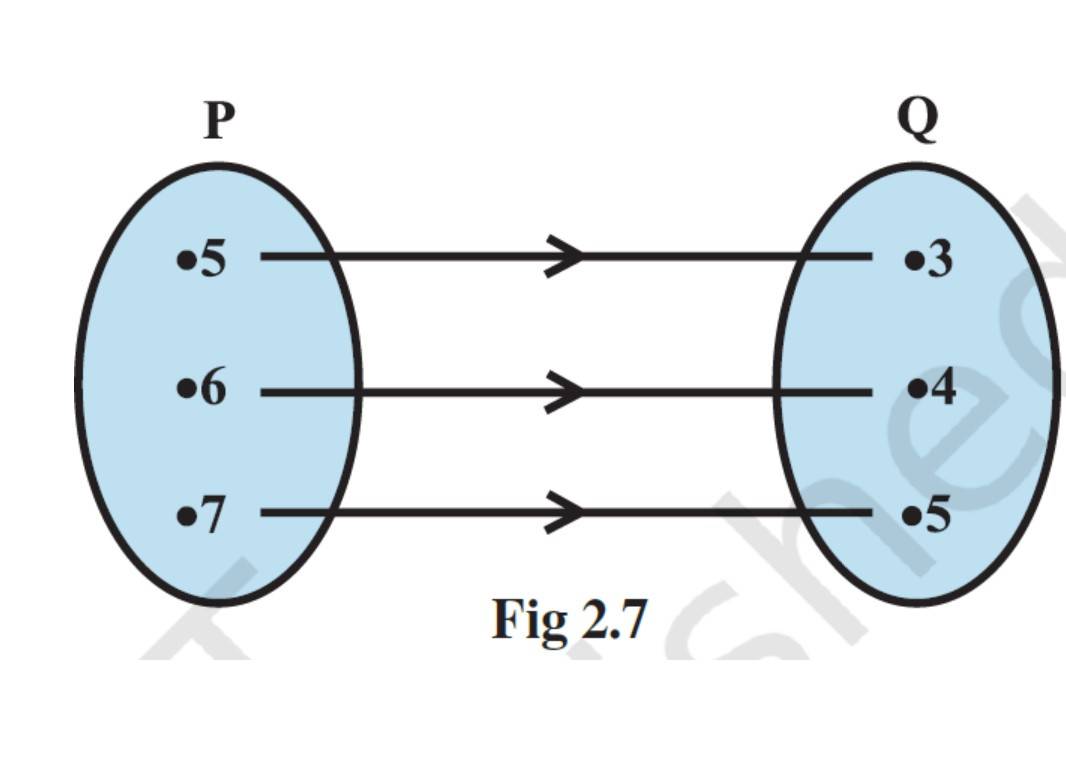
Q4. The Fig 2.7 shows a relationship between the sets P and Q. Write this relation
(i) in set-builder form (ii) roster form. What is its domain and range?
A.4. As R is a relation from set P to Q.
(i)R = {(x, y): x – 2 = y ; 5 ≤ x ≤ 7}
(ii)R = {(5,3),(6,4),(7,5)}
Domain of R={5,6,7}
range of R={3,4,5}
Q5. Let A = {1, 2, 3, 4, 6}. Let R be the relation on A defined by {(a, b): a , b A, b is exactly divisible by a}.
(i) Write R in roster form
(ii) Find the domain of R
(iii) Find the range of R.
A.5. Given, A={1,2,3,4,6}
R={(a, b): a, b A, b is exactly divisible by a}
(i)R={(1,1),(1,2),(1,3),(1,4),(1,6),(2,2),(2,4),(2,6), (3,3),(3,6),(4,4),(6,6)}
(ii)Domain of R={1,2,3,4,6}
(iii)Range of R={1,2,3,4,6}
Q6. Determine the domain and range of the relation R defined by R = {(x, x + 5) : x {0, 1, 2, 3, 4, 5}}.
A.6. Given,R ={(x, x+5): x {0,1,2,3,4,5}}
={(0,0+5), (1,1+5), (2,2+5), (3,3+5), (4,4+5), (5,5+5)}
={(0,5),(1,6),(2,7),(3,8),(4,9),(5,10)}
So, domain of R={0,1,2,3,4,5}
range of R={5,6,7,8,9,10}
Q7. Write the relation R = {(x,x3) : x is a prime number less than 10} in roster form.
A.7. GivenR={(x, x3) : x is a prime number less than 10}
R ={(x, x3) : x = 2,3,5,7}
={(2,23),(3,33),(5,53),(7,73)}
={(2,8),(3,27),(5,125),(7,343)}
Q8. Let A = {x, y, z} and B = {1, 2}. Find the number of relations from A to B.
A.8. Given, A={x, y, z}so, n(A)=3
B={1,2} so n(B)=2
⸫ n(A × B)=n(A) ×n(B)=3 × 2=6
Hence, no. of relation from A to B=Number of subsets of A × B
=26
=64.
Q9. Let R be the relation on Z defined by R = {(a,b): a, b Z, a – b is an integer}. Find the domain and range of R.
A.9. Given,R={(a, b): a, b z and a – b is an integer}
We know that, the difference of two integers is also an integer.
R={(a, b): a – b z & a, b z}
Domain of R=Z.
Range of R= Z.
Exercise 2.3
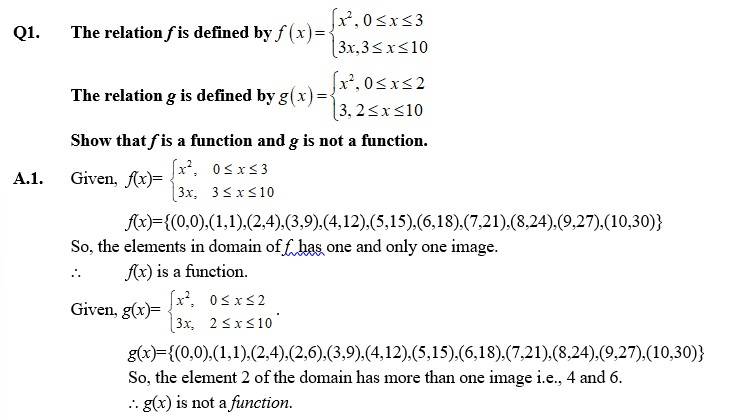
Q1. Which of the following relations are functions? Give reasons. If it is a function, determine its domain and range.
(i) {(2,1), (5,1), (8,1), (11,1), (14,1), (17,1)}
(ii) {(2,1), (4,2), (6,3), (8,4), (10,5), (12,6), (14,7)}
(iii) {(1,3), (1,5), (2,5)}.
A.1. (i)Domain of the given relation ={2,5,8,11,14,17}
Since every element of the domain has one and only one image, the given relation is a fxn.
So, domain ={2,5,8,11,14,17}
range ={1}
(ii)Domain of the given relation ={2,4,6,8,10,12,14}
Since every element of the domain has one and only one image, the given relation is a fxn.
So, domain = {2, 4, 6, 8, 10, 12, 14}
range ={1,2,3,4,5,6,7}
(iii)Domain of the given relation ={1,2}
As element 1 has more than one image i.e., 3 and 5, the given relation is not a fxn.
Q3. A function f is defined by f(x) = 2x –5. Write down the values of
(i) f (0), (ii) f (7), (iii) f (–3).
A.3. Give, f(x) = 2x – 5.
(i)f(0)=(2 × 0) –5=0 – 5= –5
(ii)f(7)=(2 × 7) –5=14 – 5=9
(iii)f(–3)=2 ×(–3) –5= –6 – 5= –11.
Q4. The function ‘t’ which maps temperature in degree Celsius into temperature in degree Fahrenheit is defined by t(C) =
Find (i) t(0) (ii) t(28) (iii) t(–10) (iv) The value of C, when t(C) = 212.
Q5. Find the range of each of the following functions.
(i) f (x) = 2 – 3x, x R, x > 0.
(ii) f (x) = x2+ 2, x is a real number.
(iii) f (x) = x, x is a real number.
A.5. (i)f(x)=2 – 3x, x R, x>0.
Given, x>0
3x>3 × 0
3x>0
(–1) × 3x<(–1) × 0.
–3x<0
2 – 3x<0+2
2 – 3x<2
i.e., f(x) < 2
Hene, range of f(x) = (– ꝏ, 2)
(ii)Given, f(x) = x2+2, x is a real number.
Since, x is a real number,
x2 ≥ 0 (x2=0 for x=0)
x2+2 ≥ 0+2
x2+2 ≥ 2
f(x) ≥ 2
⸫Range of f(x) = [2, ꝏ)
(iii)Given, f(x) = x, x is a real number.
As, f(x) = x, the range of f(x) is also real.
i.e., Range of f(x) = R.
Miscellaneous
f(x) ≥ 0
So, range of f(x)=[0, ꝏ)
Q5. Find the domain and the range of the real function f defined by
A.5. Given, f(x)=|x – 1|.
The given function is defined for all real number x.
Hence, domain of f(x)=R.
As f(x)=|x – 1|, x R is a non-negative no.
Range of f(x)=[0, ꝏ), if positive real numbers.
Q7. Let f, g : R→R be defined, respectively by f(x) = x + 1, g(x) = 2x – 3. Find f + g, f – g and
A.7. Given, f(x) = x+1. and g(x) = 2x – 3.
So, (f +g)(x) = f(x)+g(x) = (x+1)+(2x – 3) = x+1+2x – 3 = 3x – 2
(f – g)(x) = f(x) –g(x) = (x+1)–(2x–3) = x+1 – 2x+3 = 4 – x
Q8. Let f = {(1,1), (2,3), (0,–1), (–1, –3)} be a function from Z to Z defined by f(x) = ax + b, for some integers a, b. Determine a, b.
A.8. Given, f(x) = (ax + b)
= {(1,1),(2,3),(0, – 1),(–1, –3)} .
As (1,1) f.
Then, f(1)=1 [⸪ f(x) = y for (x, y)]
a × 1+b=1
a+b=1…… (1)
and (0, – 1) f .
Then, f(0)= –1
a× 0+b= –1
b= –1……..(2)
Putting value of (2) in (1) we gets
a – 1=1
a=1+1
a=2
So, (a, b)=(2, –1)
Q9. Let R be a relation from N to N defined by R = {(a, b) : a, b N and a = b2}. Are the following true?
(i) (a,a) R, for all a _ N (ii) (a,b) R, implies (b,a) R
(iii) (a,b) R, (b,c) R implies (a,c) R.
Justify your answer in each case.
A.9. Given, R={(a, b): a, b N and a = b2}
(i)Let a = 2 N
Then b = 22 = 4 N
but a ≠ b.
Hence the given statement is not true.
(ii)For a=b2 the inverse b=a2 may not hold true
Example (4,2) R, a=4, b=2 and a=b2
but (2,4) R.
Hence, the given statement is not true.
(iii)If (a,b) R
a=b2……(1)
and (b, c) R
b=c2…….(2)
so for (1) and (2),
a=(c2)2=c4.
is, a ≠c2,
Hence, (a, c) R.
⸫The given statement is false.
Q10. Let A ={1,2,3,4}, B = {1,5,9,11,15,16} and f = {(1,5), (2,9), (3,1), (4,5), (2,11)} Are the following true?
(i) f is a relation from A to B (ii) f is a function from A to B.
Justify your answer in each case.
A.10. Given,
A={1,2,3,4}
B={1,5,9,11,15,16}
f={(1,5),(2,9),(3,1),(4,5),(2,11)}.
(i)As every element of f is an element of A × B
We can clearly say that f A × B.
⸫f is a relation from A to B.
(ii)As the element 2 of the domain has two image i.e., 9 and 11. f is not a function.
Q11. Let f be the subset of Z × Z defined by f = {(ab, a + b) : a, b _ Z}. Is f a function from Z to Z? Justify your answer.
A.11. Given, f={(ab, a+b): a, b z}
Let a=1 and b=1; a, b z.
So, ab=1 × 1=1
a+b=1+1=2.
So, we have the order pair (1,2).
Now, let a= –1 and b= –1; a, b z
So, ab=(–1) × (–1)=1
a+b=(–1)+(–1)= –2
So, the ordered pair is (1, –2).
⸫The element 1 has two image i.e., 2 and –2.
Hence, f is not a function.
Q.12. Let A = {9,10,11,12,13} and let f : A→N be defined by f (n) = the highest prime factor of n. Find the range of f.
A.12. Given, A={9,10,11,12,13}.
f(x)=the highest prime factor of n.
and f: A → N.
Then, f(9)=3 [⸪ prime factor of 9=3]
f (10)=5 [⸪ prime factor of 10=2,5]
f(11)=11 [⸪ prime factor of 11 = 11]
f(12)=3 [⸪ prime factor of 12 = 2, 3]
f(13)=13 [⸪ prime factor of 13 = 13]
⸫Range of f=set of all image of f(x) = {3,5,11,13}.
Explore exams which ask questions on Maths Ncert Solutions class 11th
Select your preferred stream
News & Updates
Maths Ncert Solutions class 11th Exam
Student Forum
Popular Courses After 12th
Exams: BHU UET | KUK Entrance Exam | JMI Entrance Exam
Bachelor of Design in Animation (BDes)
Exams: UCEED | NIFT Entrance Exam | NID Entrance Exam
BA LLB (Bachelor of Arts + Bachelor of Laws)
Exams: CLAT | AILET | LSAT India
Bachelor of Journalism & Mass Communication (BJMC)
Exams: LUACMAT | SRMHCAT | GD Goenka Test