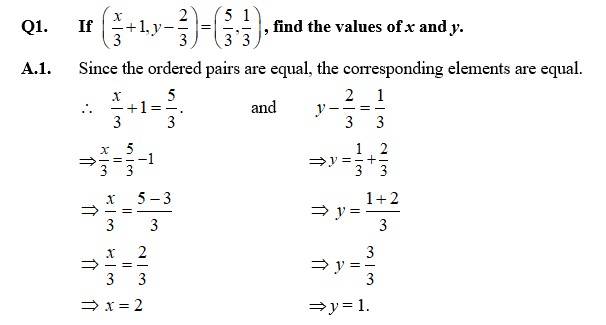
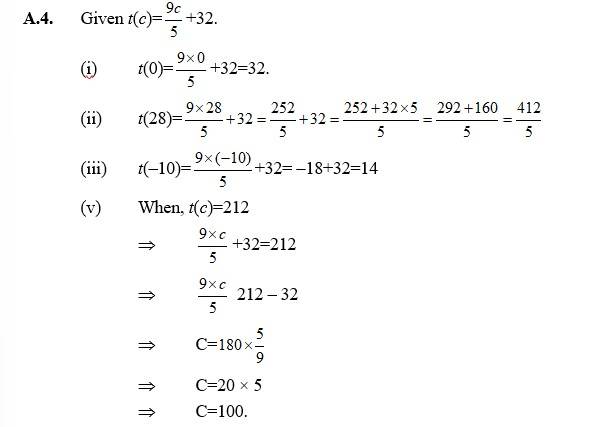Class 11 Chapter 2 Relation and Function NCERT Solutions: Shiksha has provided a complete solution for NCERT Class 11 Maths Chapter 2 Relation and Function prepared by our experts. Class 11 Maths Chapter 2 Relation and Function is one of the most important topics in Class 11 Mathematics which prepares the basics of the upcoming chapters. Shiksha brings detailed Relation and Function NCERT Solutions of class 11th to help students get a clear understanding of the concepts.
Students can access and download the NCERT class 11 Maths Relation and Function Solutions pdf available on this page. Relation and function Class 11 Solutions PDF will be very helpful for students conceptually and in problem-solving skills not only for annual exams but also competitive exams such as JEE Main, BITSAT, etc... aspirants. Students must check NCERT Class 11 Maths Chapter 1 solutions for better practice so that they can get better score in exams. Students must check the Class 11 Chapter 2 Relation and Function solutions along with Chapter 1 Sets solutions to have an inclusive understanding of the whole unit.
The Class 11 Relation and Function chapter includes many important topics such as introduction to relations, Types of relations;( reflexive, symmetric & others), ordered pairs, Types of functions, range, domain, and others. A relation R from a set A to a set B is a subset of the Cartesian Product A × B; Where there is a specific relationship between the first element x and the second element y of the ordered pairs in A × B. A function can be defined as a relation f from set A to set B where every element of set A has only one image in set B.
Students can check exercise-wise Class 11 Maths NCERT solutions & Class 12 Math NCERT Solutions of all chapters on Shiksha. Students can check the complete article for more information;
| Class 11th Math Other Important Chapters | ||
|---|---|---|
| Class 11 Chapter 1 Sets Solutions | Class 11 Chapter 3 Trigonometric Functions | Class 11 Chapter 7 Binomial Theorem |
- Class 11 Math Chapter 2 Relation and Function: Key Topics, Weightage & Important Formulae
- Class 11 Math Chapter 2 Relation and Function Solution PDF
- Class 11 Math Chapter 2 Relation and Function Exercise -wise solutions
- Class 11 Math Chapter 2 Relation and Function Exercise 2.1 solutions
- Class 11 Math Chapter 2 Relation and Function Exercise 2.2 solutions
- Class 11 Math Chapter 2 Relation and Function Exercise 2.3 solutions
- Class 11 Math Chapter 2 Relation and Function Miscellaneous Exercise solutions
- Mathematics Prep Tips and Recommended Books
Class 11 Math Chapter 2 Relation and Function: Key Topics, Weightage & Important Formulae
Class 11 Math Chapter 1 Relation and Function carries 6-10 marks weightage in the annual exams. Chapter 2 Relation and Function is also important to understand complex JEE Mains topics such as calculus and inverse trigonometry. Students can check several key topics are discussed in the exercises;
Class 11 Relation and Function Key Topics
- Relations and Cartesian Product of Sets: Ordered pairs, Cartesian product of two sets (A × B), Representation of relations
- Types of Relations: Empty Relation, Universal Relation, Symmetric Relation, Transitive Relation, Reflexive Relation, and Equivalence Relation.
- Functions and Their Representation; Definition of a function, Domain, co-domain, and range
- Types of Functions; One-One, Onto, Bijective, and into functions
Relation and Function Important Formulae for CBSE and Competitive Exams
Number of Relations:
Number of Functions:
One-One Function Condition:
Class 11 Math Chapter 2 Relation and Function Solution PDF
Students can find the solutions to all the exercises of class 11 relation and function in one place compiled by our experts. This class 11 Relation and Function NCERT solution PDF is very useful for all categories of students whether on boards or competitive exams. access the PDF below;
Class 12 Math Chapter 1 Relation and Function Solution: Free PDF Download
Explore exams which ask questions on Maths Ncert Solutions class 11th
Select your preferred stream
Class 11 Math Chapter 2 Relation and Function Exercise -wise solutions
The class 11 Relation and Function chapter 1 exercises deal with many important topics, such as relations and function basics, type of relations, symmetric and asymmetric relations, functions, their domain, and ranges. Students will encounter problems related to different concepts and properties of relations and functions in the exercises. Candidates can check the exercise-wise Chapter 2 Relation and Function Exercise-wise solutions below;
Class 11 Math Chapter 2 Relation and Function Exercise 2.1 solutions
Class 11 Relation and Function Exercise 2.1 focuses on fundamental concepts such as the introduction of relations, definition, ordered pairs, and the Cartesian product of sets. This chapter develops an understanding of how elements from two sets are paired and how different types of relations are formed. Class 11 Relation and Function Exercise 2.1 will consist of 10 solved Questions in simple language. Students can check the complete solutions of exercise 2.1 below;
Class 11 Math Chapter 2 Relation and Function Exercise 2.1 solutions
Q2. If the set A has 3 elements and the set B = {3, 4, 5}, then find the number of elements in (A×B).
A.2. Given, n(A) = 3
n(B) = 3 or B = {3,4,5}
So, number of elements in A× B = n(A× B) = n(A)× n(B) = 3 ×3 = 9.
Q3. If G = {7, 8} and H = {5, 4, 2}, find G × H and H × G.
A.3. Given, G = {7, 8} and H = {5, 4, 2}
By the definition of the Cartesian product,
G ×H = {(x, y): x∈G and y = ∈ H}
= {((7, 5), (7, 4), (7, 2), (8, 5),(8,4), (8,2)}
H× G = {(x, y): x∈ H and y ∈G}
= {(5, 7), (5, 8), (4,7), (4, 8), (2, 7), (2,8)}
Q4. State whether each of the following statements are true or false. If the statement is false, rewrite the given statement correctly.
(i) If P = {m, n} and Q = { n, m}, then P × Q = {(m, n),(n, m)}.
(ii) If A and B are non-empty sets, then A × B is a non-empty set of ordered pairs (x, y) such that x A and y B.
(iii) If A = {1, 2}, B = {3, 4}, then A ×
A.4. (i) False. Here P = {m, n}, n(p)=2
Q = {n, m}, n(Q)=2
n(P× Q) = n(P)× n(Q) = 2× 2 = 4.
So, P ×Q = {((m, n),(m, m),(n, n),(n, m)}
(ii) True.
(iii) True. { A ×(B
= n(A) ×0 {∵
=
Q5. If A = {–1, 1}, find A × A × A.
A.5. Given, A = {1,1}
So, A× A = {(1,1),(1,1),(1,1),(1,1)}
A ×A ×A = {(1,1),(1,1),(1,1),(1,1)} ×{1,1}
= {(1,1.1),(1, 1, 1),(1, 1, 1,),(1,1,1), (1,1,1), (1, 1,1), (1, 1,1), (1,1,1)}
Q6. If A × B = {(a, x),(a , y), (b, x), (b, y)}. Find A and B.
A.6. Given,
A ×B = {(a, x),(a, y),(b, x),(b, y)}
We know that,
A ×B = {(p, q); p ∈ A and q ∈ B}
So, A = {a, b} and B = {x, y}.
Q7. Let A = {1, 2}, B = {1, 2, 3, 4}, C = {5, 6} and D = {5, 6, 7, 8}. Verify that (i) A × (B∩ C) = (A × B)∩ (A × C). (ii) A × C is a subset of B × D.
A.7.Given,
A={1, 2}, B = {1,2,3,4}, C={5,6} and D={5,6,7,8}
(i)L.H. S = A ×(B∩ C) = {1,2} [{1,2,3,4} ∩{5,6}]
={1,2}×
=
R.H.S = (A× B)∩ (A ×C)=[{1,2}×{1,2,3,4}]∩[{1,2} {5,6}]
=[{(1 , 1), (1, 2), (1, 3), (1, 4), (2, 1), (2, 2), (2, 3), (2, 4)]∩ [{1,5),(1,6),(2,5), (2,6)}]
=
Hence, L.H.S= R.H.S.
(ii)
A× C ={1, 2}× {5,6}
={(1,5),(1,6),(2,5),(2,6)}
B× D ={1,2,3,4} ×{5,6,7,8}
={(1,5),(1,6),(1,7),(1,8),(2,5),(2,6),(2,7),(2,8),(3,5),(3,6),(3,7),(3,8),(4,5),(4,6),(4,7),(4,8)}
As every element of A C is also an element of B× D.
A ×C B ×D
Q8. Let A = {1, 2} and B = {3, 4}. Write A × B. How many subsets will A × B have? List them.
A.8. A Given, A={1,2}
B={3,4}
So, A× B={(1,3),(1,4),(2,3),(2, 4)}
i.e., n(A ×B)=4
A ×B will have subset =24=16.They are,
Φ,{(1,3)},{(1,4)},{(2, 3)},{(2,4)},{(1,3),(1,4)},{(1,3),(2,3)},
{(1,3),(2, 4)},{(1,4),(2, 3)},{(1,4),(2, 4)}, {(2,3),(2, 4)},
{(1,3),(1,4),(2, 3)},{(1,3),(1,4),(2,4)}, {(1,3),(2,3),(2, 4)},{(1,4),(2,3),(2,4)},
and {(1,3),(1,4),(2,3),(2,4)}
Q9. Let A and B be two sets such that n(A) = 3 and n(B) = 2. If (x, 1), (y, 2), (z, 1) are in A × B, find A and B, where x, y and z are distinct elements.
A.9. Given, n(A)=3
n(B)= 2
So, n(Ax B)=n(A).n(B)=3x 2=6
as (x, 1),(y, 2),(z, 1) ∈Ax B={(x, y), x∈Aand y∈B}.
A={x, y, z} and B={1,2}
As n(A) = 3as n(B) = 2
Q10. The Cartesian product A × A has 9 elements among which are found (–1, 0) and (0,1). Find the set A and the remaining elements of A × A.
A.10. Given, n(A × A)=9
n(A) ×n(A) = 9.
n(A)2 = 32.
n(A) = 3 .
And (–1,0),(0,1) A × A i.e., A × A = {(x, y), x A, y B}
⸫A={–1,0,1}
And A × A={–1,0,1} × {–1,0,1}
={(–1, –1),( –1,0),( –1,1),(0, –1),(0,0),(0,1),(1, –1),(1,0),(1,1)}
Class 11 Math Chapter 2 Relation and Function Exercise 2.2 solutions
Class 11 Math Relation and Function Exercise 2.2 focuses on different types of relations, including reflexive, symmetric, transitive, and equivalence relations. The Relation and Function Exercise 2.2 deals with application of the concepts of relations in real-world-based problem-solving. Class 11 Math Chapter 2 Relation and Function Exercise 2.2 consists of 9 Questions. Students can check the solutions below;
Q1. Let A = {1, 2, 3,...,14}. Define a relation R from A to A by R = {(x, y) : 3x – y = 0, where x, y A}. Write down its domain, co-domain and range.
A.1. Given,A ={1,2,3, …, 14}
R ={(x, y): 3x – y = 0; x, y A}
={(x, y): 3x = y; x, y A}.
= {(1,3),(2,6),(3,9),(4,12)}
Domain of R is the set of all the first elements of the ordered pairs in R
So, domain of R={1,2,3,4}
Codomain of R is the whole set A.
So, codomain of R={1,2,3, …, 14}
Range of R is the set of all the second elements of the ordered pains in R.
So, range of R={3,6,9,12}
Q2. Define a relation R on the set N of natural numbers by R = {(x, y) : y = x + 5, x is a natural number less than 4; x, y N}. Depict this relationship using roster form. Write down the domain and the range.
A.2. Given,R ={(x, y): y = x + 5, x is a natural number less than 4; x, y N}
={(x, y): y = x + 5; x, y N and x < 4}.
={(1,1+5), (2,2+5), (3,3+5)}
={(1,6), (2,7), (3,8)}
So, domain of R = {1,2,3}
range of R = {6,7,8}
Q3. A = {1, 2, 3, 5} and B = {4, 6, 9}. Define a relation R from A to B by R = {(x, y): the difference between x and y is odd; x A, y B}. Write R in roster form.
A.3. Given,A={1,2,3,5}
B={4,6,9}
R={(x, y) : the difference of x & y is odd; x A, y B}.
={(x, y):|x – y| is odd and x A, y B}
={(1,4),(1,6),(2,9),(3,4),(3,6),(5,4),(5,6)}.
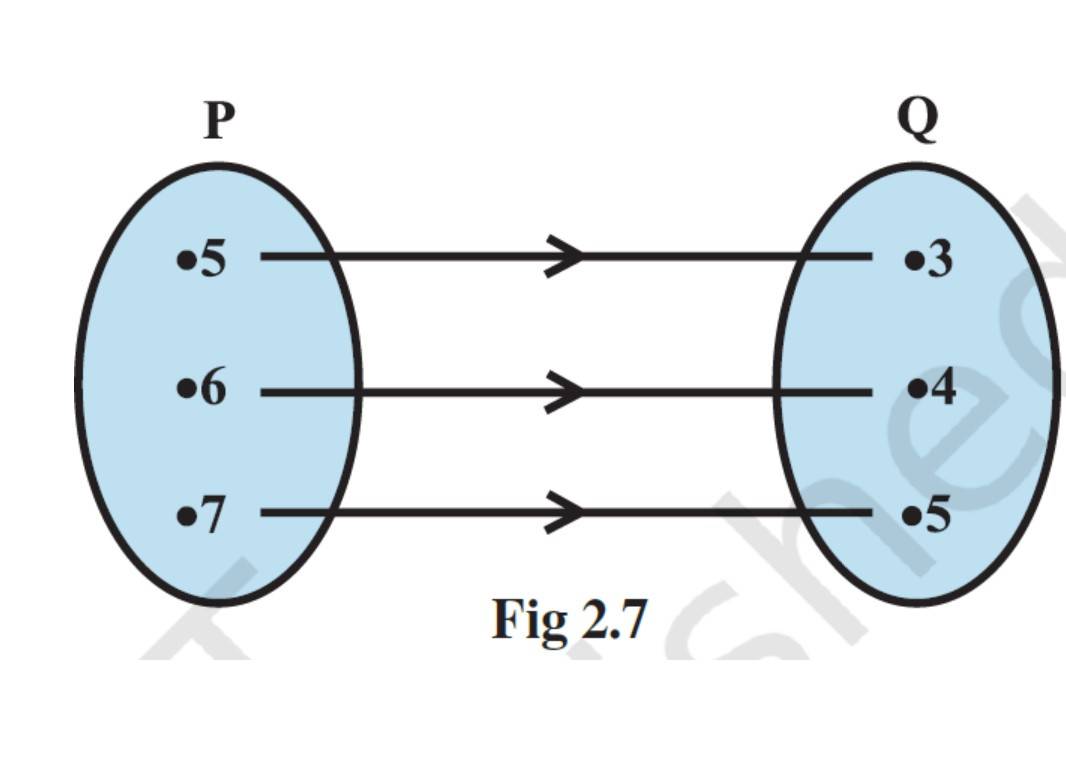
Q4. The Fig 2.7 shows a relationship between the sets P and Q. Write this relation
(i) in set-builder form (ii) roster form. What is its domain and range?
A.4. As R is a relation from set P to Q.
(i)R = {(x, y): x – 2 = y ; 5 ≤ x ≤ 7}
(ii)R = {(5,3),(6,4),(7,5)}
Domain of R={5,6,7}
range of R={3,4,5}
Q5. Let A = {1, 2, 3, 4, 6}. Let R be the relation on A defined by {(a, b): a , b A, b is exactly divisible by a}.
(i) Write R in roster form
(ii) Find the domain of R
(iii) Find the range of R.
A.5. Given, A={1,2,3,4,6}
R={(a, b): a, b A, b is exactly divisible by a}
(i)R={(1,1),(1,2),(1,3),(1,4),(1,6),(2,2),(2,4),(2,6), (3,3),(3,6),(4,4),(6,6)}
(ii)Domain of R={1,2,3,4,6}
(iii)Range of R={1,2,3,4,6}
Q6. Determine the domain and range of the relation R defined by R = {(x, x + 5) : x {0, 1, 2, 3, 4, 5}}.
A.6. Given,R ={(x, x+5): x {0,1,2,3,4,5}}
={(0,0+5), (1,1+5), (2,2+5), (3,3+5), (4,4+5), (5,5+5)}
={(0,5),(1,6),(2,7),(3,8),(4,9),(5,10)}
So, domain of R={0,1,2,3,4,5}
range of R={5,6,7,8,9,10}
Q7. Write the relation R = {(x,x3) : x is a prime number less than 10} in roster form.
A.7. GivenR={(x, x3) : x is a prime number less than 10}
R ={(x, x3) : x = 2,3,5,7}
={(2,23),(3,33),(5,53),(7,73)}
={(2,8),(3,27),(5,125),(7,343)}
Q8. Let A = {x, y, z} and B = {1, 2}. Find the number of relations from A to B.
A.8. Given, A={x, y, z}so, n(A)=3
B={1,2} so n(B)=2
⸫ n(A × B)=n(A) ×n(B)=3 × 2=6
Hence, no. of relation from A to B=Number of subsets of A × B
=26
=64.
Q9. Let R be the relation on Z defined by R = {(a,b): a, b Z, a – b is an integer}. Find the domain and range of R.
A.9. Given,R={(a, b): a, b z and a – b is an integer}
We know that, the difference of two integers is also an integer.
R={(a, b): a – b z & a, b z}
Domain of R=Z.
Range of R= Z.
Class 11 Math Chapter 2 Relation and Function Exercise 2.3 solutions
Class 11 Math Chapter 2 Relation and Function Exercise 2.3 introduces the concept of functions, their definition, and how they differ from general relations. This exercise helps students understand important terms like domain, co-domain, and range while exploring different types of functions. Chapter 2 Relation and Function Exercise 2.3 consists of 5 solved questions. Students can check complete solutions below;
Class 11 Math Chapter 2 Relation and Function Exercise 2.3 Solutions
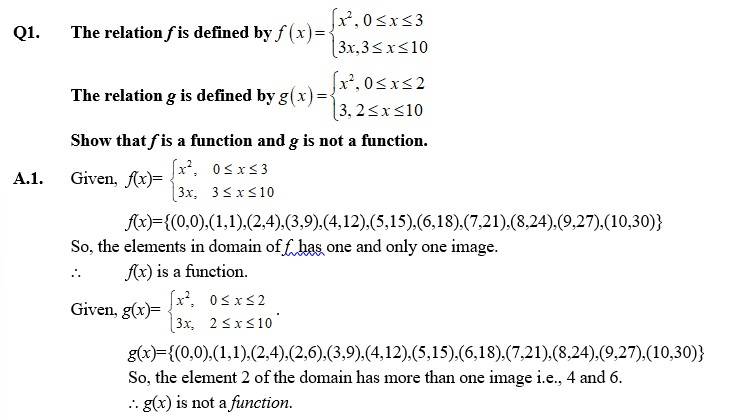
Q1. Which of the following relations are functions? Give reasons. If it is a function, determine its domain and range.
(i) {(2,1), (5,1), (8,1), (11,1), (14,1), (17,1)}
(ii) {(2,1), (4,2), (6,3), (8,4), (10,5), (12,6), (14,7)}
(iii) {(1,3), (1,5), (2,5)}.
A.1. (i)Domain of the given relation ={2,5,8,11,14,17}
Since every element of the domain has one and only one image, the given relation is a fxn.
So, domain ={2,5,8,11,14,17}
range ={1}
(ii)Domain of the given relation ={2,4,6,8,10,12,14}
Since every element of the domain has one and only one image, the given relation is a fxn.
So, domain = {2, 4, 6, 8, 10, 12, 14}
range ={1,2,3,4,5,6,7}
(iii)Domain of the given relation ={1,2}
As element 1 has more than one image i.e., 3 and 5, the given relation is not a fxn.
Q3. A function f is defined by f(x) = 2x –5. Write down the values of
(i) f (0), (ii) f (7), (iii) f (–3).
A.3. Give, f(x) = 2x – 5.
(i)f(0)=(2 × 0) –5=0 – 5= –5
(ii)f(7)=(2 × 7) –5=14 – 5=9
(iii)f(–3)=2 ×(–3) –5= –6 – 5= –11.
Q4. The function ‘t’ which maps temperature in degree Celsius into temperature in degree Fahrenheit is defined by t(C) =
Find (i) t(0) (ii) t(28) (iii) t(–10) (iv) The value of C, when t(C) = 212.
Q5. Find the range of each of the following functions.
(i) f (x) = 2 – 3x, x R, x > 0.
(ii) f (x) = x2+ 2, x is a real number.
(iii) f (x) = x, x is a real number.
A.5. (i)f(x)=2 – 3x, x R, x>0.
Given, x>0
3x>3 × 0
3x>0
(–1) × 3x<(–1) × 0.
–3x<0
2 – 3x<0+2
2 – 3x<2
i.e., f(x) < 2
Hene, range of f(x) = (– ꝏ, 2)
(ii)Given, f(x) = x2+2, x is a real number.
Since, x is a real number,
x2 ≥ 0 (x2=0 for x=0)
x2+2 ≥ 0+2
x2+2 ≥ 2
f(x) ≥ 2
⸫Range of f(x) = [2, ꝏ)
(iii)Given, f(x) = x, x is a real number.
As, f(x) = x, the range of f(x) is also real.
i.e., Range of f(x) = R.
Class 11 Math Chapter 2 Relation and Function Miscellaneous Exercise solutions
Class 11 Math Chapter 2 Miscellaneous Exercise brings together all fundamental and advanced concepts of relations and functions such as types of relations, different functions, domain and range, and function composition. Miscellaneous Exercise of Class 11 Chapter 2 will contain Solutions 12 Questions. Students can check the complete solution below;
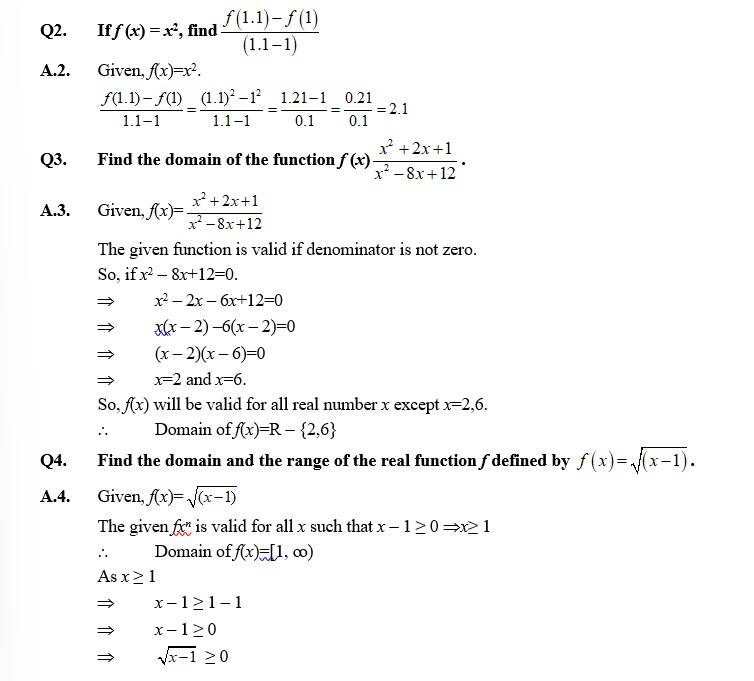
Class 11 Math Chapter 2 Relation and Function Exercise 2.1 solutions
f(x) ≥ 0
So, range of f(x)=[0, ꝏ)
Q5. Find the domain and the range of the real function f defined by
A.5. Given, f(x)=|x – 1|.
The given function is defined for all real number x.
Hence, domain of f(x)=R.
As f(x)=|x – 1|, x R is a non-negative no.
Range of f(x)=[0, ꝏ), if positive real numbers.
Q7. Let f, g : R→R be defined, respectively by f(x) = x + 1, g(x) = 2x – 3. Find f + g, f – g and
A.7. Given, f(x) = x+1. and g(x) = 2x – 3.
So, (f +g)(x) = f(x)+g(x) = (x+1)+(2x – 3) = x+1+2x – 3 = 3x – 2
(f – g)(x) = f(x) –g(x) = (x+1)–(2x–3) = x+1 – 2x+3 = 4 – x
Q8. Let f = {(1,1), (2,3), (0,–1), (–1, –3)} be a function from Z to Z defined by f(x) = ax + b, for some integers a, b. Determine a, b.
A.8. Given, f(x) = (ax + b)
= {(1,1),(2,3),(0, – 1),(–1, –3)} .
As (1,1) f.
Then, f(1)=1 [⸪ f(x) = y for (x, y)]
a × 1+b=1
a+b=1…… (1)
and (0, – 1) f .
Then, f(0)= –1
a× 0+b= –1
b= –1……..(2)
Putting value of (2) in (1) we gets
a – 1=1
a=1+1
a=2
So, (a, b)=(2, –1)
Q9. Let R be a relation from N to N defined by R = {(a, b) : a, b N and a = b2}. Are the following true?
(i) (a,a) R, for all a _ N (ii) (a,b) R, implies (b,a) R
(iii) (a,b) R, (b,c) R implies (a,c) R.
Justify your answer in each case.
A.9. Given, R={(a, b): a, b N and a = b2}
(i)Let a = 2 N
Then b = 22 = 4 N
but a ≠ b.
Hence the given statement is not true.
(ii)For a=b2 the inverse b=a2 may not hold true
Example (4,2) R, a=4, b=2 and a=b2
but (2,4) R.
Hence, the given statement is not true.
(iii)If (a,b) R
a=b2……(1)
and (b, c) R
b=c2…….(2)
so for (1) and (2),
a=(c2)2=c4.
is, a ≠c2,
Hence, (a, c) R.
⸫The given statement is false.
Q10. Let A ={1,2,3,4}, B = {1,5,9,11,15,16} and f = {(1,5), (2,9), (3,1), (4,5), (2,11)} Are the following true?
(i) f is a relation from A to B (ii) f is a function from A to B.
Justify your answer in each case.
A.10. Given,
A={1,2,3,4}
B={1,5,9,11,15,16}
f={(1,5),(2,9),(3,1),(4,5),(2,11)}.
(i)As every element of f is an element of A × B
We can clearly say that f A × B.
⸫f is a relation from A to B.
(ii)As the element 2 of the domain has two image i.e., 9 and 11. f is not a function.
Q11. Let f be the subset of Z × Z defined by f = {(ab, a + b) : a, b _ Z}. Is f a function from Z to Z? Justify your answer.
A.11. Given, f={(ab, a+b): a, b z}
Let a=1 and b=1; a, b z.
So, ab=1 × 1=1
a+b=1+1=2.
So, we have the order pair (1,2).
Now, let a= –1 and b= –1; a, b z
So, ab=(–1) × (–1)=1
a+b=(–1)+(–1)= –2
So, the ordered pair is (1, –2).
⸫The element 1 has two image i.e., 2 and –2.
Hence, f is not a function.
Q.12. Let A = {9,10,11,12,13} and let f : A→N be defined by f (n) = the highest prime factor of n. Find the range of f.
A.12. Given, A={9,10,11,12,13}.
f(x)=the highest prime factor of n.
and f: A → N.
Then, f(9)=3 [⸪ prime factor of 9=3]
f (10)=5 [⸪ prime factor of 10=2,5]
f(11)=11 [⸪ prime factor of 11 = 11]
f(12)=3 [⸪ prime factor of 12 = 2, 3]
f(13)=13 [⸪ prime factor of 13 = 13]
⸫Range of f=set of all image of f(x) = {3,5,11,13}.
Mathematics Prep Tips and Recommended Books
Maths Ncert Solutions class 11th Exam
Student Forum
Popular Courses After 12th
Exams accepted
CA FoundationExams accepted
ICSI ExamExams accepted
BHU UET | GLAET | GD Goenka TestBachelor of Business Administration & Bachelor of Law
Exams accepted
CLAT | LSAT India | AIBEExams accepted
IPMAT | NMIMS - NPAT | SET
Exams accepted
BHU UET | KUK Entrance Exam | JMI Entrance ExamBachelor of Design in Animation (BDes)
Exams accepted
UCEED | NIFT Entrance Exam | NID Entrance ExamBA LLB (Bachelor of Arts + Bachelor of Laws)
Exams accepted
CLAT | AILET | LSAT IndiaBachelor of Journalism & Mass Communication (BJMC)
Exams accepted
LUACMAT | SRMHCAT | GD Goenka Test