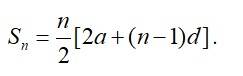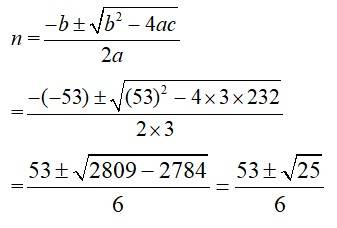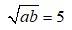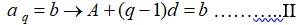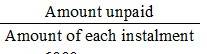NCERT Solutions for Class 11 Maths Chapter 8 Sequences and Series: Topics, Questions, Preparation

Ex. 9.1

Write the first five terms of each of the sequences in Exercises 1 to 6 whose nth terms are:

Q1. a_n = n (n + 2)

A.1. Here a_n = n (n + 2)

Substituting n=1,2,3,4,5 we get,

a₁=1(1+2)=1 × 3=3

a₂=2(2+2)=2 × 4=8

a₃=3(3+2)=3 × 5=15

a₄=4(4+2)=4 × 6=24

a₅=5(5+2)=5 × 7=35.

Hence, the first five terms are 3,8,15,24 and 35

 

Q2. a_n= $\frac{n}{n+1}$

A.2. Here, a₁= $\frac{n}{n+1}$

Substituting n=1,2,3,4,5 we get,

$a_1=\frac{1}{1+1}=\frac{1}{2}$

$a_2=\frac{2}{2+1}=\frac{2}{3}$

$a_3=\frac{3}{3+1}=\frac{3}{4}$

$a_4=\frac{4}{4+1}=\frac{4}{5}$

$a_5=\frac{5}{5+1}=\frac{5}{6}$ .

Hence the first five terns are  $\frac{1}{2},\frac{2}{3},\frac{3}{4},\frac{4}{5}$ and  $\frac{5}{6}$ .

 

Q3. a_n=2ⁿ

A.3. Here a_n=2ⁿ

Substituting n=1,2,3,4,5 we get,

a₁=2¹=2

a₂=2²=4

a₃=2³=8

a₄=a⁴=16

a₅=2⁵=32.

Hence the first five terns are 2,4,16,32 and 64.

 

Q4.  $a_n=\frac{2n-3}{6}$

A.4. Here, $a_n=\frac{2n-3}{6}$

Putting n=1,2,3,4,5 we get,

$a_1=\frac{2\times 1-3}{6}=\frac{2-3}{6}=-\frac{1}{6}$

$a_2=\frac{2\times 2-3}{6}=\frac{4-3}{6}=\frac{1}{6}$

$a_3=\frac{2\times 3-3}{6}=\frac{6-3}{6}=\frac{3}{6}=\frac{1}{2}$

$a_4=\frac{2\times 4-3}{6}=\frac{8-3}{6}=\frac{5}{6}$

$a_5=\frac{2\times 5-3}{6}=\frac{7}{6}$

Hence, the first five terms are  $-\frac{1}{6},\frac{1}{6},\frac{1}{2},\frac{5}{6},and\frac{7}{6}$

 

Q5. a_n = (–1)^{n – 1}  5^{n + 1}

A.5. Here, a_n=(–1)^{n – 1} . 5ⁿ⁺¹

        Putting n=1,2,3,4,5 we get,

                        a₁=(–1)^{1 – 1}.5¹⁺¹=(–1)⁰× 5²=25

                        a₂=(–1)^{2 – 1}.5²⁺¹=(–1)¹× 5³= –125

                        a₃=(–1)^{3 – 1}. 5³⁺¹=(–1)²× 5⁴=625

                        a₄=(–1)^{4 – 1}. 5⁴⁺¹=(–1)³. 5⁵= –3125

                        a₅=(–1)^{5 – 1}. 5⁵⁺¹=(–1)⁴. 5⁶=15625.

    Hence, the first five terms are 25, –125,625, –3125and 15625.

 

Q6. a_n = n $\frac{n^2+5}{4}$

A.6. Here, a_n=n $\frac{x^2+5}{4}$

Putting n=1,2,3,4,5 we get,

$a_1=1\times \frac{\left(1^2+5\right)}{4}=1\times \frac{6}{4}=\frac{3}{2}$

$a_2=2\times \left(\frac{2^2+5}{4}\right)=2\times \frac{(4+5)}{4}=\frac{9}{2}$

$a_3=3\times \frac{\left(3^2+5\right)}{4}=3\times \frac{(9+5)}{4}=3\times \frac{14}{4}=\frac{21}{2}$

$a_4=4\times \frac{\left(4^2+5\right)}{4}=4\times \frac{(16+5)}{4}=4\times \frac{21}{4}=21$

$a_5=5\times \frac{\left(5^2+5\right)}{4}=5\times \frac{(25+5)}{4}=5\times \frac{30}{4}=\frac{75}{2}$

Hence, the first five terms are  $\frac{3}{2},\frac{9}{2},\frac{21}{2},21,\frac{75}{2}$ .

 

Q7. a_n = 4_n – 3; a₁₇, a₂₄

A.7. a_n=4_n – 3.

            Putting n=17, we get

                        a₁₇=4 × 17 – 3=68 – 3=65.

            and putting n=24 we get,

                        a₂₄=4 × 24 – 3= 96 – 3=93

 

Q8. $a_n=\frac{n^2}{2^n}$ ; a7

A.8. $a_n=\frac{n^2}{2^n}$

Put n=7,

$a_7=\frac{7^2}{2^7}=\frac{49}{128}$ .

 

Q9. a_n = ( –1)^{n – 1}n³; a₉

A9. a_n=( –1)^{n – 1} .n³.

            Put n=9 we get,

                        a_q=(–1)^{9 – 1}.9³=(–1)⁸. 729=729.

 

Q10. $a_n=\frac{n(n-2)}{n+3}$ ;a₂₀

A.10. $a_n=\frac{x(x-2)}{x+3}$ .

Put n=20,

$a_{20}=\frac{20(20-2)}{20+3}=\frac{20\times 18}{23}=\frac{360}{23}$

Write the first five terms of each of the sequences in Exercises 11 to 13 and obtain thecorresponding series:

 

Q11. a₁= 3, a_n= 3a_{n – 1}+ 2 for all n > 1

A.11. Given, a₁=3

            a_n=3a_{n – 1}.+2                  "n>1.

            Puttingn=2,3,4,5 we get,

                        a₂=3a_{2 – 1}+2=3a₁+2=3 × 3+2=9+2=11

                        a₃=3a_{3 – 1}+2=3a₂+2=3 × 11+2=33+2=35

                        a₄=3a_{4 – 1}+2=3a₃+2=3 × 35+2=105+2=107.

                        a₅=3a_{5 – 1}+2=3a₄+2=3 × 107+2=321+2=323.

            Hence, the first five terms of the sequence are 3,11,35,107,323.

            And the series is 3+11+35+107+323+ …

 

Q12. a1 = –1, $a_n=\frac{a_{n-1}}{n},n\ge 2$

A.12. Given, a₁= –1,

$a_n=\frac{a_{n-1}}{n},n\ge 2$

Putting n=2,3,4,5 we get,

$a_2=\frac{a_{2-1}}{2}=\frac{a_1}{2}=-\frac{1}{2}$

$a_3=\frac{a_{3-1}}{3}=\frac{a_2}{3}=\frac{-1/2}{3}=-\frac{1}{6}$

$a_4=\frac{a_{4-1}}{4}=\frac{a_3}{4}=\frac{-1/6}{4}=-\frac{1}{24}.$

$a_5=\frac{a_{5-1}}{5}=\frac{a_4}{5}=\frac{-1/24}{5}=-\frac{1}{120}$ .

So the first five terms of the sequence are –1,  $-\frac{1}{2},-\frac{1}{6},-\frac{1}{24}$ and  $-\frac{1}{120}$ .

And the series is  $(-1)+\left(-\frac{1}{2}\right)+\left(-\frac{1}{6}\right)+\left(-\frac{1}{24}\right)+\left(-\frac{1}{120}\right)+\dots$ .

 

Q13. a₁ = a₂= 2, a_n = a_{n – 1}–1, n > 2

A.13. Given,

            a₁=a₂=2.

            a_n=a_{n – 1} – 1

            Putting n=3,4,5.

                        a₃=a_{3 – 1} – 1=a_{3 – 1} – 1=a₂ – 1=2 – 1=1

                        a₄=a_{4 – 1} – 1=a₃ – 1=1 – 1=0

                        a₅=a_{5 – 1} – 1=a₄ – 1=0 – 1= –1 .

            Hence the first five forms of the sequence are 2,2,1,0, –1.

            And the series is 2+2+1+0+(–1)+ ….

 

Q14. The Fibonacci sequence is defined by

1 = a₁ = a₂ and a_n= a_{n – 1} + a_{n – 2}, n > 2.

Find  $\frac{a_{n+1}}{a_n}$ , for n = 1, 2, 3, 4, 5.

A.14. Given, a₁=1=a₂.

                        a_n=a_{n – 1}+a_{n – 2},n>2.

We need to find,  $\frac{a_{n+1}}{n}$

Putting n=3,4,5,6 in an=an – 1+an – 2 we have,

a₃=a_{3 – 1}+a_{3 – 2}=a₂+a₁=1+1=2.

a₄=a_{4 – 1}+a_{4 – 2}=a₃+a₂=2+1=3.

a₅=a_{5 – 1}+a_{5 – 2}=a₄+a₃=3+2=5.

a₆=a_{6 – 1}+a_{6 – 2}=a₅+a₄=5+3=8.

Now, to find  $\frac{a_{n+1}}{n}$ ,

Substitute n=1,2,3,4,5.

$\frac{a_{1+1}}{a_1}=\frac{a_2}{a_1}=\frac{1}{1}=1$

$\frac{a_{2+1}}{a_2}=\frac{a_3}{a_2}=\frac{2}{1}=2$

$\frac{a_{3+1}}{a_3}=\frac{a_4}{a_3}=\frac{3}{2}$

$\frac{a_{4+1}}{a_4}=\frac{a_5}{a_4}=\frac{5}{3}$

$\frac{a_{5+1}}{a_5}=\frac{a_6}{a_5}=\frac{8}{5}$ .

 

Ex .9.2

Q1. Find the sum of odd integers from 1 to 2001.

A.1. Sum of odd integers from 1 to 2001

=1+3+5+ … +2001

So, a=1

d=3 – 1=2

⸫ For n^th term,

a_n=a+(n – 1)d.

⸪ the last n^th term is 2001,

2001 =1+(n – 1)2.

(n – 1)2 =2001 – 1

n – 1=  $\frac{2000}{2}=1000$

n =1000+1

n =1001.

⸫ Sum of n terms, S_n= $\frac{n}{2}$ (a + l); l = last term.

⸫ Required sum =  $\frac{1001}{2}(1+2001)=\frac{1001}{2}\times 20021001\times 1001$ =1002001

 

Q2. Find the sum of all natural numbers lying between 100 and 1000, which aremultiples of 5.

A.2. Sum of all natural number between 100 and 1000 which are multiple of 5.

=105+110+115+ … +995.

So,a=105.

a=110 – 105=5.

As the last term is 995 which is the nthterm,

a+(n – 1)d =995.

105+(n – 1) × 5 =995.

(n – 1) × 5=995 – 105.

(n – 1)5 =890

n – 1 =  $\frac{890}{5}=178$

n =178+1

n =179

So, required sum S_n $\frac{n}{2}$ (a+l); l=last term.

$=\frac{179}{2}(105+995)$

$=\frac{179}{2}\times 1100$

= 179 × 550

=98450.

 

Q3. In an A.P., the first term is 2 and the sum of the first five terms is one-fourth ofthe next five terms. Show that 20^thterm is –112.

A.3. Given, a=2

Let A1, A2, A3, A4, A5, A6, A1, A6, A7, A10 be the first ten terms. So, A1=a.

Given A1+A2+A3+A4+A5=  $\frac{1}{4}$ (A6+A1+A8+A1+A10)

a+(a+d)+(a+2d)+(a+3d)+(a+4d)

=  $\frac{1}{4}$ [(a+5d)+(a+6d)+(a+7d)+(a+8d)+(a+9d)]

5a+10d=  $\frac{1}{4}$ [5a+35d].

4[5a+10d]=9a+35d.

20a+40d=5a+35d.

40d – 35d=5a – 20a

5d= –15a

d= –3a

d= –3 × 2 [as a=2]

d= –6

So, A₂₀=a+(20 – 1)d

=2+19 × (–6)

=2 – 114

= –112.

 

Q4. How many terms of the A.P. – 6, $-\frac{11}{2}$ , –5, ….are needed to give the sum –25?

A.4. Let the sum of n farms of the A.P –6, $-\frac{11}{2}$ , –5, …. gives –25.

Then, 

 

From the given A.P.

a= –6

d= –  $\frac{11}{2}-(-6)=-\frac{11}{2}+6=-\frac{11+12}{2}=\frac{1}{2}$

So, –25=  $\frac{n}{2}\left[2\times (-6)+(n-1)\left(\frac{1}{2}\right)\right]$

–25 × 2=n  $\left[-12+\frac{n-1}{2}\right]$

–50=n  $\left[\frac{-12\times 2+(n-1)}{2}\right]$

–50=n  $\left[\frac{-24+n-1}{2}\right]$

–50 × 2 =n[n – 25]=n² – 25n

n² – 25n+100=0.

n² – 5n – 20n+100=0

n(n – 5) – 20(n – 5)=0

(n – 5)(n – 20)=0

So, n=5 and n=20.

When n=5

$S_5=\frac{5}{2}\left[-12+(5-1)\frac{1}{2}\right]=\frac{5}{2}\left[-12+4\times \frac{1}{2}\right]=\frac{5}{2}[-12+2]=\frac{5}{2}\times (-10)$ = –25.

When n=20

$S_{20}=\frac{20}{2}\left[-12+(20-1)\frac{1}{2}\right]=10\left[-12+\frac{19}{2}\right]$ =  $-120+\frac{19\times 10}{2}$ = –120 + 19 × 5 = –120 + 95 = –25.

 

Q5. In an A.P., if p^th term is $\frac{1}{q}$ and qth term is  $\frac{1}{q}$ , prove that the sum of first pqterms is  $\frac{1}{2}$ (pq+1), where p ≠ q.

A.5. Let a and d be the first term and the common difference of an A.P.

Then, given a_p = $\frac{1}{q}$

a+(p – 1)d =  $\frac{1}{q}$ --------(1)

and a_q = $\frac{1}{p}$

a+(q – 1)d =  $\frac{1}{p}$ ---------(2)

Subtracting eqn (2) from (1) we get,

a+(p – 1)d – [a+(q – 1)d]=  $\frac{1}{q}-\frac{1}{p}$

a+(p – 1)d– a–(q– 1)d=  $\frac{p-q}{pq}$

[(p – 1)–(q – 1)]d=  $\frac{p-q}{pq}$

[p – 1 – q+1]d=  $\frac{p-q}{pq}$

[p – q]d=  $\frac{p-q}{pq}$ .

d=  $\frac{1}{pq}$ .

Putting d=  $\frac{1}{pq}$ in eqn (1) we get,

$a+(p-1)\frac{1}{pq}=\frac{1}{q}$ .

$a+\frac{1}{pq}-\frac{1}{pq}=\frac{1}{q}$

$a+\frac{1}{q}-\frac{1}{pq}=\frac{1}{q}$

$a=\frac{1}{q}-\frac{1}{q}+\frac{1}{pq}$  $a=\frac{1}{pq}$ .

So, sum of first pq terms,

$S_{pq}=\frac{pq}{2}\left[2\times \frac{1}{pq}+(pq-1)\frac{1}{pq}\right]$

=  $\frac{pq}{2}\times \frac{1}{pq}[2+pq-1]$ .

=  $\frac{1}{2}[pq+1]$

 

Q6. If the sum of a certain number of terms of the A.P. 25, 22, 19, … is 116. Find thelast term.

A.6. The given A.P. is 25,22,19, …

So,a=25

d=22 – 25= –3

Given that, sum of first n terms of the AP=116.

 

n[2 × 25+(n – 1)(–3)]=116 × 2

n[50 – 3n+3]=232

n[53 – 3n]=232 .

53n – 3n²=232.

3n² – 53n+232.

Using quadratic formula, a=3, b= –53, c=232.

 

n =  $\frac{53+5}{6}$ or  $\frac{53-5}{6}$

=  $\frac{58}{6}$ or  $\frac{48}{6}$

=  $\frac{29}{3}$ or 8.

As n  $\in$ N, n=8.

⸫ Last term=a+(n – 1)d

=a+(8 – 1)d

=25+7×(–3)

=25 – 21

=4.

 

Q7. Find the sum to n terms of the A.P., whose k^thterm is 5k + 1.

A.7. Given,a_k =5k+1

Putting k =1,

a₁ =5 × 1+1=5+1=6.

a_n =5n+1=l

So, sum of unto n teams of the AP,S_n = $\frac{n}{2}[2a+l]$

S_n = $\frac{n}{2}[6+5n+1]$

=  $\frac{n}{2}[7+5n]$ .

Q8. If the sum of n terms of an A.P. is (pn+ qn²), where p and q are constants,find the common difference.

A.8. Given, sum of n terms of A.P, S_n=(pⁿ+qn²), p&q are constants substituting n=1,

S₁=p× 1+q× 1²=p+q=a₁ [sum upto1^st term only]

            And substituting n=2,

            S₂=p× 2+q× 2²=2p+4q=a₁+q₂  [sum upto 2^ndtern only]

            So, a₁+a₂=2p+4q

            Þ        a₂=2p+4q – a₁=2p+4q – (p+q)=2p – p+4q – q

            Þ        a₂=p+3q

            So, common difference, d=a₂ – a₁

                                                =(p+3q) – (p+q)

                                                =p+3q – p – q

                                                =2q.

 

Q9. The sums of n terms of two arithmetic progressions are in the ratio5n + 4 : 9n + 6. Find the ratio of their 18th terms.

A.9. Let a1, a2 be the first terms and d1, d2 be the common difference of the first term A.P.S

So,  $\frac{\mathrm{Sum\; ofntermofIst}{}^{}AP}{\mathrm{Sum\; of\; n\; terms\; of\; 2nd\; AP}}=\frac{5n+4}{9n+6}$

$\frac{\frac{n}{2}\left[2a_1+(n-1)d_1\right]}{\frac{n}{2}\left[2a_2+(n-1)d_2\right]}=\frac{5n+4}{9n+6}$

$\frac{2a_1+(n-1)d}{2a_2+(n-1)d}=\frac{5n+4}{9n+6}$ ---------------(1)

The ratio of their 18thterms.

$\frac{a_{1\mathrm{8offirstA.P}}}{a_{1\mathrm{8ofsecondA.P}}}=\frac{a_1+(18-1)d_1}{a_2+(18-1)d_2}$

=  $\frac{a_1+17d_1}{a_2+17d_2}$

=  $\frac{a_1+17d_1}{a_2+17d_2}\times \frac{2}{2}$

=  $\frac{2a_1+34d_1}{2a_2+35d_2}$ --------(2)

Comparing eqn (1) and (2),

$\frac{2a_1+34d_1}{2a_2+35d_2}=\frac{2a_1+(35-1)d_1}{2a_2+(35-1)d_1}=\frac{5\times 35+4}{9\times 35+6}$ =  $\frac{179}{321}$

So, ratio of their 18th terms =  $\frac{179}{321}$

 

Q10. If the sum of first p terms of an A.P. is equal to the sum of the first q terms, thenfind the sum of the first (p + q) terms.

A.10. Let a and d be the first elements and common different of the A.P.

Then, Sum of first p terms of the AP=Sum of first q terms of A.P

Sp=Sq

$\frac{p}{2}[2a+(p-1)d]=\frac{q}{2}[2a+(q-1)d]$

p[2a+pd – d]=q[2a+qd – d]

                 2ap+p²d – pd=2aq+q²d – qd

                 2ap – 2aq+p²d – q²d – pd+qd=0

                2a(p – q)+[p² – q² – p+q]d=0.

                2a(p – q)+[(p – q)(p+q) – (p – q)]d=0

                (p – q){2a+[(p+q) – 1]d}=0

Deviding both sides by p – q,

2a+[(p+q) –1]d=0.

And multiplying by P+Q/2 we get,

$\frac{p+q}{2}\{2a+[(p+q)-1]d\}=0$ which in in the form  $\frac{n}{2}\{2a+(n-1)d\}$ where n=p+q.

S_p+q=0.

 

Q11. Sum of the first p, q and r terms of an A.P. are a, b and c, respectively.

Prove that  $\frac{a}{p}(q-r)+\frac{b}{p}(r-p)$ +  $\frac{c}{r}(p-q)$ = 0

A.11. Let e and d the first term and common difference of an AP.

Then,

Sum of first p terms, S_p=a.

$\frac{p}{2}[2e+(p-1)d]=a$

$\frac{1}{2}[2e+(p-1)d]=\frac{a}{p}$ .

Similarly, S_q=b

$\frac{q}{2}[2e+(q-1)d]=b$

$\frac{1}{2}[2e+(q-1)d]=\frac{b}{q}$ ----------------(2)

And. S_r=C

$\frac{r}{2}[2e+q(r-1)d]=c$

$\frac{1}{2}[2c+(r-1)d]=\frac{c}{r}$ -----------------(3)

So, L.H.S.  $\frac{a}{p}(q-r)+\frac{b}{p}(n-p)$ +  $\frac{c}{r}(p-q)$ (⸪ given)

=  $\frac{1}{2}[2e+(p-1)d](q-r)$ +  $\frac{1}{2}[2e+(q-1)d](r-p)$ +  $\frac{1}{2}[2e+(r-1)d](p-q)$

=  $\frac{2e}{2}(q-r)+\frac{d}{2}(p-1)(q-r)+\frac{2e}{2}(n-p)$ +  $\frac{d}{2}(q-1)(r-p)$ +  $\frac{2e(p-q)}{2e}+\frac{d}{2}(r-1)(p-q)$

=c[q – r+r – p+p – q]+  $\frac{d}{2}$ [(p – 1)(q – r)+(q – 1)(r – p)+(r – 1)(p – q)]

=C × 0 +  $\frac{d}{2}$ [pq – pr – q+r+qr – pq – r+p+pr=qr – p+q]

=0+  $\frac{d}{2}$ [pq – pq – pr+pr – q+q+r – r+qr+qr+p – p]

=  $\frac{d}{2}[0]$

=0.

=R.H.S.

 

Q12. The ratio of the sums of m and nterms of an A.P. is m² :n². Show that the ratioof mthand nth term is (2m – 1) : (2n – 1).

A.12. Let a and d be the first term and common difference of A.P.

Then,  $\frac{\mathrm{Sum\; of\; M\; terms\; of\; A.P.}}{\mathrm{SumofntermsofA.P.}}=\frac{m^2}{n^2}$

$\frac{\frac{m}{2}[2a+(m-1)d]}{\frac{m}{2}[2a+(n-1)d]}=\frac{m^2}{n^2}$

$\frac{m[2a+(m-1)d]}{n[2a+(n-1)d]}=\frac{m^2}{n^2}$

Dividing both sides by m/n we get,

$\frac{2a+(m-1)d}{2a+(n-1)d}=\frac{m}{n}$

[2a+(n – 1)d]n=m[2a+(n – 1)d].

2an+dmn – dn=2am+dmn – dm.

2an – 2am=dn – dm+2mn – dmn

2a(n – m)=d(n – m)

d=  $\frac{2a(n-m)}{(n-m)}$

d=2a.

Now, Ratio of m^th  and n^thterm

=  $\frac{a+(m-1)d}{a+(n-1)d}$

Putting d=2a in the above we get,

ratio of mth and nth term =  $\frac{a+(m-1)2a}{a+(n-1)2a}$

=  $\frac{a+2am-2a}{a+2an-2a}$

=  $\frac{2am-a}{2an-a}$

=  $\frac{a[2m-1]}{a[2n-1]}$

=  $\frac{2m-1}{2n-1}$ .

 

Q13. If the sum of n terms of an A.P. is 3n² + 5n and its m^th term is 164, find the valueof m.

A.13. Given,

Sum of n terms of AP, S_n=3n²+5n

Put n=1,

                        S₁=3 × 1²+5 × 1=3+5=8=a₁ (⸪  S₁=sum of 1* terms of AP)

            Put n=2,

                        S₂=3 × 2²+5 × 2=3 × 4+10=12+10 =22

                                                =a₁+a₂(⸪  Sum of first two term of A.P)

            So, a₁+a₂=22

                 8 +a₂=22

                a₂=22 – 8

               a₂=14

            ⸫          First term, a=a₁=8

            Common difference, d=a₂ – a₁=14 – 8=6

            Now, given that,a_m = 164.

                a+(m – 1)d=164.

               8+(m – 1)6=164.

               (m – 1)6=164 – 8.

m – 1=  $\frac{156}{6}$

m=26+1

m=27.

 

Q14. Insert five numbers between 8 and 26 such that the resulting sequence is an A.P.

A.14. Let A₁, A₂, A₃, A₄and A₅ be five numbers between 8 and 26.

            So, the A.P is 8, A₁, A₂, A₃, A₄, A₅, 26. with n=7 terms.

Also,a = 8.

l=26, last term.

a+(7 – 1)d=26

8+6d=26

6d=26 – 8

d=  $\frac{18}{6}$

d=3

So,

A₁=a+d=8+3=11

                        A₂=a+2d=8+2 × 3=8+6=14

                        A₃=a+3d=8+3 × 3=8+9=17.

                        A₄=a+9d=8+4 × 3=8+12=20

                        A₅=a+5d=8+5 × 3=8+15=23.

Hence the reqd. five nos are 11,14,17,20 and 23.

 

Q15. If $\frac{a^n+b^n}{a^{n-1}+b^{n-1}}=$ is the A.M. between a and b, then find the value of n.

A.15. Given,

A.M betwna and b=  $\frac{a+b}{2}$

And  $\frac{a^n+b^n}{a^{n-1}+b^{n-1}}=\frac{a+b}{2}$

2[aⁿ+bⁿ]=(a+b)(a^{n – 1}+b^{n – 1})

                    2aⁿ+2bⁿ=a^{n – 1}.a+ab^{n – 1}+ba^{n – 1} + b^{n – 1}.b

                    2aⁿ+2bⁿ=a^{n – 1 + 1}+ ab^{n – 1}+ba^{n – 1} + b^{n – 1 + 1}

                   2aⁿ+2bⁿ=aⁿ+ ab^{n – 1}+b^{n – 1} + bⁿ

                    2aⁿ – aⁿ – ban ^{– 1} = ab^{n – 1} + bⁿ– 2bⁿ

                    aⁿ – ba^{n – 1}=ab^{n – 1} – bn

                   a^{n – 1}[a – b]=b^{n – 1}[a – b] [⸪  aⁿ=a^{n – 1+1}=a^{n – 1} .a¹]

                   a^{n – 1}=b^{n – 1}.

$\frac{a^{n-1}}{b^{n-1}}=1$ .

${\left(\frac{a}{b}\right)}^{n-1}={\left(\frac{a}{b}\right)}^0$ [⸪a⁰=1].

n – 1=0

n=1.

 

Q16. Between 1 and 31, m numbers have been inserted in such a way that the resultingsequence is an A. P. and the ratio of 7th and (m – 1)th numbers is 5 : 9. Find thevalue of m.

A.16. Let A₁, A₂, A₃ ... A_m be the m terms such that,

            1, A₁, A₂, A₃, ... A_m, 31 is an A.P.

So, a=1, first term of A.P

n=m+2, no. of term of A.P

l=31, last term of A.P. or (m+2)th term.

a+[(m+2) –1]d =31

1 +[m+1]d =31

(m+1)d =31 – 1=30

d =  $\frac{30}{m+1}$

The ratio of 7th and (m – 1) number is

$\frac{a+7d}{a+(m-1)d}=\frac{5}{9}$ ⸪ a₁term=a

a₂ =A1=a+d

a₃ =A2=a+2d

:

a₈ = A₇ = a + 7d

9[a+7d]=5[a+(m – 1)d]

9a+63d=5a+5(m – 1)d.

9a – 5a=5(m – 1)d – 63d.

4a=[5(m – 1) –63]d.

So, putting a=1 and d=  $\frac{30}{m+1}$

4 × 1=[5(m – 1) –63] ×  $\frac{30}{m+1}$

4(m+1)=[5(m – 1) –63] × 30

4m+4=[5m – 5 – 63] × 30

4m+4 =150m – 68 × 30  => 150m – 2040

150m – 4m =2040+4

146m =2044.

m =  $\frac{2044}{146}$

m =14.

 

Q17. A man starts repaying a loan as first instalment of Rs. 100. If he increases the instalment by Rs 5 every month, what amount he will pay in the 30th instalment?

A.17. Since the man starts paying installmentas ₹ 100 and increases ₹ 5 every month.

a=100

d=5.

So, the A.P is 100,105,110,115, ….

⸫Amount of 30thinstallment=30th term of A.P =a₃₀

=a+(30 – 1)d

=100+29 × 5.

=100+145

= ₹245

i.e., He will pay ₹ 245 in his 30th instalment.

 

Q18. The difference between any two consecutive interior angles of a polygon is 5°.If the smallest angle is 120° , find the number of the sides of the polygon.

A.18. Let 'n' be the no. of sides of a polygon.

So, sum of all angles of a polygon with sides n

=(2n – 4) × 90°

=(n – 2) × 2 × 90°

=(n – 2)180°

As the smallest angle is 120° and the difference between 2 consecutive interior angle is 5°. We have,

a =120°

d =5°

So, sum of n sides =180°(n – 2).

$\frac{n}{2}[2a+(n-1)d]=180^{°}(n-2)$

$\frac{n}{2}\left[2\times 120^{°}+(n-1)5^{°}\right]=180^{°}(n-2)$

n[240°+5°n – 5°]=360°n– 720°

n[5°n+235°]=360°n – 720°

5°n²+235°n=360°n – 720°

n²+47°n=72n – 144    [⸪ dividing by 5]

n²+47n – 72n+144=0.

n² – 25n+144=0

n² – 9n– 16n+144=0

n(n – 9) –16(n – 9)=0

(n – 9)(n – 16)=0

So, n=9,16.

When n=9,

Largest angle =a+(9 – 1)d=120°+8 × 5° = 120° + 40° = 160°< 180°

Hence, n=9 is permissible

When n=16,

Lorgest angle =a+(16 – 1)d=120°+15 × 5° = 120° + 75° = 195°> 180°.

which is not permissible as no angle in polygon can exceed 180°.

Hence, number of sides in polygon, n=9.

 

Ex. 9.3

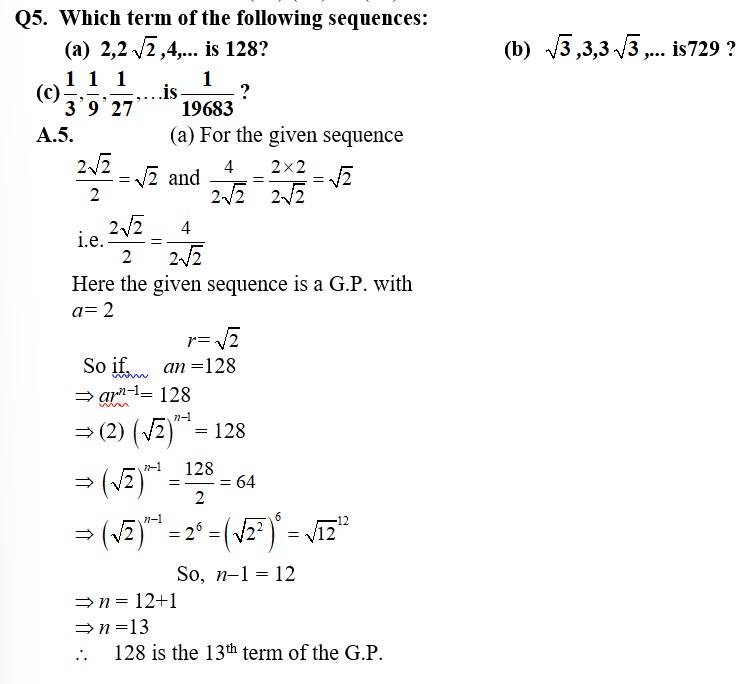
Q1. Find the 20th and nth terms of the G.P.  $\frac{5}{2},\frac{5}{4},\frac{5}{8},...$

A.1. Here, a= $\frac{5}{2}$

=  $$  $r=\frac{\raisebox{1ex}{$5$}\!\left/ \!\raisebox{-1ex}{$4$}\right.}{\raisebox{1ex}{$5$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}=\frac{1}{2}$

so, a_n =  ar^n-1

i.e  a₂₀=ar^20-1= $\left(\frac{5}{2}\right)\times {\left(\frac{1}{2}\right)}^{19}=\frac{5}{2}\times \frac{1}{2^{19}}=\frac{5}{2^{19+1}}=\frac{5}{2^{20}}$

and an= ar^n-1= $\left(\frac{5}{2}\right)\times {\left(\frac{1}{2}\right)}^{n-1}=\frac{5}{2}\times \frac{1}{2^{n-1}}=\frac{5}{2^{n-1+1}}=\frac{5}{2^n}$

 

Q2. Find the 12th term of a G.P. whose 8th term is 192 and the common ratio is 2.

A.2. Given, r=2

Let a be the first term,

Then,  $$a₈= 92

$\Rightarrow$ ar^8-1=192

$\Rightarrow$a (2)⁷ = 192

$\Rightarrow$ a =  $\frac{192}{2^7}=\frac{192}{128}=\frac{3}{2}$

so,a =  $\frac{3}{\begin{array}{l}2\\ \end{array}}$

$\therefore$ a₁₂ = ar^12-1=  $\begin{array}{l}\left(\frac{3}{2}\right)\\ \end{array}$ (2)¹¹ = 3 2^11-1

= 3×2¹⁰

= 31024

= 3072.

 

Q3. The 5th, 8th and 11th terms of a G.P. are p, q and s, respectively. Showthat q² = ps.

A.3. Let a and r be the first term and the common ratio btw G.P.

So, a₅= p $\Rightarrow$ a r^t-1 $$  $$ = p  $\Rightarrow$ ar⁴ = p

a₈ = q $\Rightarrow$ a r^8-1 =q $\Rightarrow$ ar⁷= q

a₁₁= r $\Rightarrow$ ar^11-1 = r $\Rightarrow$ ar¹⁰= 5

So, L.H.S. q^{2 =}  (ar⁷)² = a²r¹⁴

R.H. S. = p.s= (ar⁴) (ar)¹⁰ = a¹⁺¹ r⁴⁺¹⁰  = a²r¹⁴

$\therefore$ L.H.S. = R.H.S.

 

Q4. The 4th term of a G.P. is square of its second term, and the first term is – 3.Determine its 7th term.

A.4. Given, a = 3

Let r be the common ratio of the G.P.

Then,a₄ = (a₂)²

$\Rightarrow$ar^4-1 =  (ar^2-1)²

$\Rightarrow$ ar³=  (ar)²

$\Rightarrow$ ar³=  a²r²

$\Rightarrow$  $\frac{r^3}{r^2}=\frac{a^2}{2}$

$\Rightarrow$ r = a = 3

$\therefore$ a₇ = ar^7-1  = ar⁶= (-3) (-3)⁶  = (-3)⁷ = -2187.

 

 

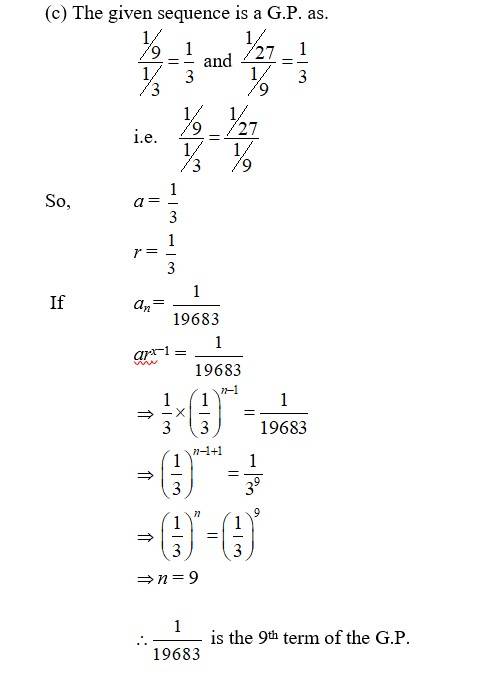
Q6. For what values of x, the numbers  $-\frac{2}{7}$ ,  $x$ ,  $-\frac{7}{2}$ are in G.P.?

A.6. For $\frac{-2}{7}$ ,  $x$ ,  $\frac{-7}{2}$ to be in G.P. we have the condition,

$\Rightarrow$  $\frac{x}{-2/7}=\frac{-7/2}{x}$

$\Rightarrow$  $x^2=\left(\frac{-7}{2}\right)\times \left(\frac{-2}{7}\right)$

$\Rightarrow$  $x^2=1$

$\Rightarrow$  $x=+1$

Find the sum to indicated number of terms in each of the geometric progressions inExercises 7 to 10:

 

Q7. 0.15, 0.015, 0.0015, ... 20 terms.

A.7. Here a = 0.15 = $\frac{15}{100}$

$r=\frac{0.015}{0.15}=\frac{\frac{15}{1000}}{\frac{15}{150}}=\frac{1}{10}$ <1

n = 20

So, Sum to term of G.P.,  $s_n=\frac{a(1-r^n)}{1-r}$

$\Rightarrow$  $s_{20}=\frac{\frac{15}{100}\left[1-{\left(\frac{1}{10}\right)}^{20}\right]}{1-\frac{1}{10}}$

=  $\frac{\frac{15}{100}\left[1-{(0.1)}^{20}\right]}{\frac{9}{10}}$

=  $\frac{15}{100}\times \frac{10}{9}\left[1-{(0.1)}^{20}\right]$

=  $\frac{1}{6}\left[1-{(0.1)}^{20}\right]$

 

 

Q9. 1, – a,a², – a³, ... n terms (if a ≠ – 1).

A.9. Here, $a_1=1$

$r=\frac{-9}{1}=-a$

So,  $s_n=\frac{a_1\left[1-r^n\right]}{1-r}$

=  $\frac{1\left[1-{\left(-a\right)}^n\right]}{1-\left(-a\right)}$

=  ${\frac{1-\left(a\right)}{1+a}}^n$

 

Q10.  .  x³, x⁵, x⁷, ... n terms (if x ≠ ± 1).

A.10. Here, $a=x^3$

 

So,  $s_n=\frac{a\left[1-r^n\right]}{1-r}$

$$ =  $\frac{x^3\left[1-x^2\right]}{1-x^2}$

 

Q11. Evaluate  ${\displaystyle \underset{k=1}{\overset{11}{\sum }}}\left(2+3^x\right).$

A.11.  ${\displaystyle \underset{}{\overset{11}{\sum }}}\left(2+3^x\right)=\left(2+3^1\right)+\left(2+3^2\right)+.........+\left(2+3^{11}\right)$

$x=1$ [2+2+ …..+(11lines)] + (3¹+ 3²⁺……+3¹¹)

=  $11\times 2+\frac{3\left(3^{11}-1\right)}{3-1}\left[\because s_n=\frac{a\left(r^n-1\right)}{r-1},r\ge 1\right]$

=  $22+\frac{3\left(3^{11}-1\right)}{2}$

 

Q12. The sum of first three terms of a G.P. is  $\frac{39}{10}$ and their product is 1. Find the common ratio and the terms.

A.12. Let the three terms of the G.P be $\frac{a}{r},a,ar.$

So,  $\frac{a}{r}a.ar=1$

=  $a^3=1$

=  $a=1$

And  $\frac{a}{r}+a+ar=\frac{39}{10}$

=  $\frac{1}{r}+1+r=\frac{39}{10}$ (as a = 1)

=  $\frac{1+r+r^2}{r}=\frac{39}{10}$

=  $\left(r^2+r+1\right)\times 10=39\times r$

=  $10r^2+10r+10=39r$

=  $10r^2+10r-39r+10=0$

=  $10r^2-29r+10=0$

=  $10r^2-25r-4r+10=0$

=  $5r\left(2r-5\right)-2\left(2r-5\right)=10$

$=\left(2x-5\right)\left(5r-2\right)=0$

=  $\left(2x-5\right)=0$ or  $\left(5r-2\right)=0$

=  $r=\frac{5}{2}$ or  $r=\frac{2}{5}$

When  $a=1,$  $r=\frac{5}{2}$ the terms are,

$$  $\frac{1}{\raisebox{1ex}{$5$}\!\left/ \!\raisebox{-1ex}{$2$}\right.},1,$  $1\times \frac{5}{2}=\frac{2}{5},1,\frac{5}{2}$

When  $a=1,$  $r=\frac{2}{5}$ the terms are,

$\frac{1}{\raisebox{1ex}{$2$}\!\left/ \!\raisebox{-1ex}{$5$}\right.},$ 1,  $1\times \frac{2}{5}=\frac{5}{2},$ 1,  $\frac{2}{5}$

 

Q13. How many terms of G.P. 3, 3², 3³, … are needed to give the sum 120?

A.13. Given, a=3

$r=\frac{3^3}{3}=3$>1

If s_n=120

Then  $\frac{a\left(r^n-1\right)}{r-1}=120$

$\Rightarrow$  $\frac{3\left(3^n-1\right)}{3-1}=120$

$\Rightarrow$  $\frac{3\left(3^n-1\right)}{2}=120$

$\Rightarrow$  $3^n-1=120\times \frac{2}{3}$

$\Rightarrow$3ⁿ= 80+1

$\Rightarrow$ 3ⁿ= 81

= 3ⁿ = 34

$\therefore r=4$

So, 120 is the sum of first 4 terms of the G.P

 

Q14. The sum of first three terms of a G.P. is 16 and the sum of the next three terms is128. Determine the first term, the common ratio and the sum to n terms of the G.P.

A.14. Let a be the first term and r be the common ratio of the G.P.

Then  $a_1+a_2+a_3=16$

$a+ar+a{r}^2+16^{}$

$a\left(1+r+r^2\right)=16$ ………. I

Also  $a_4+a_5+a_6=128$

$a{r}^3+a{r}^4+a{r}^5=128$

$a{r}^3\left(1+r+r^2\right)=128$ …… II

Dividing II and I we get,

$\frac{a{r}^3\left(1+r+r^2\right)}{a\left(1+r+r^2\right)}=\frac{128}{16}$

r³= 8

r³ = 2³

 r = 2 >1

So, putting r = 2 in I we get,

$a\left(1+2+2^2\right)=16$

$\Rightarrow$  $a\left(1+2+4\right)=16$

$\Rightarrow$  $a\left(7\right)=16$

$\Rightarrow$  $a=\frac{16}{7}$

And  $s_x=\frac{a\left(r^n-1\right)}{r-1}$

$\Rightarrow$  $\frac{\frac{16}{7}\left(2^n-1\right)}{2-1}$

$\Rightarrow$  $\frac{16}{7}\left(2^n-1\right)$

 

Q15. Given a G.P. with a = 729 and 7th term 64, determine S₇.

A.15. Given, a= 729

$a_7=a{r}^6=64$

$\Rightarrow$ 729. r⁶ = 64

$\Rightarrow$  $r^6=\frac{64}{729}$

$\Rightarrow$  $r^6={\left(\frac{2}{3}\right)}^6$

$\Rightarrow$  $r=+\frac{2}{3}$ <1

When  $r=\frac{2}{3}$

=  $s_7=\frac{a\left(1-r^7\right)}{4-r}=\frac{729\left(1-{\left(\frac{2}{3}\right)}^7\right)}{1-\frac{2}{3}}=\frac{729\left[1-\frac{128}{2187}\right]}{\frac{3-2}{2}}$

=  $729\times \frac{3}{1}\times \left[\frac{2187-128}{2187}\right]$

=  $729\times \frac{3\times 2059}{2187}$

= 2059

When  $r=\frac{2}{3}$

$s_7=\frac{a\left(1-r^7\right)}{1-r}=\frac{729\left[1-{\left(\frac{-2}{3}\right)}^7\right]}{1-\left(\frac{-2}{3}\right)}=\frac{729\left[1+\frac{128}{2187}\right]}{1+\frac{2}{3}}$

=  $\frac{729\left[\frac{2187+128}{2187}\right]}{\frac{3+2}{3}}$

=  $729\times \frac{3}{5}\times \left[\frac{2315}{2187}\right]$

= 463

 

Q16. Find a G.P. for which sum of the first two terms is – 4 and the fifth term is4 times the third term.

A.16. Let a and r be the first term and common ratio.

Then,  $\Rightarrow$  $a_1+a_2=-4$

$\Rightarrow$  $a+ar=-4$

$\Rightarrow$  $a\left(1+r\right)=-4$

$\Rightarrow$ a (1+ n) = 4

$\Rightarrow$  $a{r}^4=4a{r}^2$

$\Rightarrow$ r² = 4

$\Rightarrow$ r² =2²

$\Rightarrow$ r = ±2

When r =2

a (1+2)=-4

a 3 = -4

$a=\frac{-4}{3}$

So, the G.P. is a, ar, ar²,…………. $\Rightarrow$  $\frac{-4}{3},\frac{-4}{3}\times 2,\frac{-4}{3}{\left(2\right)}^2...........$

$\Rightarrow$  $\frac{-4}{3},\frac{-8}{3},\frac{-16}{3},.........$

When  $r=-2$

$a=\left(1-2\right)=-4$

a = (-) = -4

a = 4

So, the reqd. by G.P. is a, ar, ar²…………. $\Rightarrow$ 4, 4 (-2), 4 (-2)², .................

$\Rightarrow$ 4, 8, 16, ..........

 

Q17. If the 4th, 10th and 16th terms of a G.P. are x, y and z, respectively. Prove that x, y, z are in G.P.

A.17. Let aand r be the first term and common ration of the G.P.

So,  $a_4=x$

$\Rightarrow$  $a{r}^3=x$ ----------------- I

And a₁₀ = y

$\Rightarrow$  $a{r}^9=y$ ---------------- II

Also a 16 = z

$a{r}^{15}=z$ -------------------- III

So,  $\frac{y}{x}=\frac{a{r}^9}{a{r}^3}=r^{9-3}=r^6$

and  $\frac{z}{y}=\frac{a{r}^{15}}{a{r}^9}=r^{15-9}=r^6$

$\because \frac{y}{x}=\frac{z}{y}$

$\because$ x, y, z are in G.P

 

Q18. Find the sum to n terms of the sequence, 8, 88, 888, 8888… .

A.18. The given sequence, 8, 88, 888, 8888, …., upto xterm is not a G.P. so we can such that it will be changed to a G.P. by the following .

Sum of x terms, s_x = 8+ 88+888+8888 …..upto x term

=  $8\left[1+11+111+1111+......\right]$ upto x term

Multiplying the numerator and denumerator by 9 we get,

=  $\frac{8}{9}\left[9+99+999+9999+.........\right]$ x terms

=  $\frac{8}{9}\left[\left(10-1\right)+\left(10^2-1\right)+\left(10^3-1\right)+\left(10^4-1\right)+.....\right]$ upto x terms

=  $\frac{8}{9}$ [(10 + 1010 + 103 +104 ……..upto, x terms) (1 +1 + 1 + 1 …… upto, x terms)]

=  $\frac{8}{9}\left[\frac{10\left(10^n-1\right)}{10-1}-1\times n\right]$

=  $\frac{8}{9}\left[\frac{10\left(10^n-1\right)}{9}-n\right]$

=  $\frac{80}{81}\left(10^n-1\right)-\frac{8}{9}n$

 

Q19. Find the sum of the products of the corresponding terms of the sequences 2, 4, 8,16, 32 and 128, 32, 8, 2,  $\frac{1}{2}$ .

A.19. The sum of product of corresponding terms of the given

Sequences =  $\left(2\times 128\right)+\left(4\times 32\right)+\left(8\times 8\right)+\left(16\times 2\right)+\left(32\times \frac{1}{2}\right)$

= 256+128+69+32+16

The above is a G.P. of a = 256,  $r=\frac{128}{256}=\frac{1}{2}$< 1 and x = 5

Sum required =  $\frac{256\left(1-{\left(\frac{1}{2}\right)}^5\right)}{1-\frac{1}{2}}$

=  $\frac{256\left(1-\frac{1}{32}\right)}{\frac{2-1}{2}}$

=  $\frac{256\times \left(\frac{32-1}{32}\right)}{\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}=256\times 2\times \frac{31}{32}=496$

 

Q20. Show that the products of the corresponding terms of the sequences a, ar, ar²,…ar^{n– 1} and A, AR, AR²,…AR^{n – 1} form a G.P, and find the common ratio.

A.20. The product of corresponding terms of the given sequence are

=  $a\times A+\left(ar\right)\times AR+\left(a{r}^2\right)\times A{R}^2+......\left(a{r}^{x-1}\right)\left(A{R}^{x-1}\right)$

=  $aA+\left(aA\right)+\left(rR\right)+\left(aA\right)$  $......+\left(aA\right){\left(rR\right)}^{x-1}$

So, looking at the sequence forms a G.P.

(above the)

With common ratio =  $\frac{\left(aA\right)\left(rR\right)}{\left(aA\right)}=rR$

 

Q21. Find four numbers forming a geometric progression in which the third term is greater than the first term by 9, and the second term is greater than the 4th by 18.

A.21. Let the four numbers is G.P. be a, ar, ar², ar³

Given,  $a{r}^2-a=9$

$\Rightarrow$  $a\left(r^2-1\right)=9$ I

And  $\Rightarrow$  $ar-a{r}^3=18$

$\Rightarrow$  $ar\left(1-r^2\right)=18$

$\Rightarrow$  $\left(-1\right)ar\left(x^2-1\right)=18$

$\Rightarrow$  $ar\left(x^2-1\right)=-18$ II

Dividing II by I we get,

$\frac{ar\left(r^2-1\right)}{a\left(r^2-1\right)}=\frac{-18}{9}$

r = 2

So, putting r = 2 in I we get ,

$\Rightarrow$  $a\left[{\left(-2\right)}^2-1\right]=9$

$\Rightarrow$  $a\left[4-1\right]=9$

$\Rightarrow$  $a\times 3=9$

$\Rightarrow$  $a\frac{9}{3}$

$\Rightarrow$ a = 3

$\therefore$ The four numbers are 3, 3´(-2), 3´(-2)², 3´(-2)³

$\Rightarrow$ 3, 6, 12, 24

 

Q22. If the pth, qth and rth terms of a G.P. are a, b and c, respectively. Prove that

a^{q – r}b^{r – p}c^{P – q}= 1

A.22. Let a and r be the first term and common ratio of the G.P.

Then, ap = a

$A{R}^{p-1}=a$ …… I

and aq = b

$A{R}^{q-1}=b$ …….II

Also, ar = c

$A{R}^{r-1}=c$ …..III

Given, L.H.S. =  $a^{a-r}$  $b^{r-p}$  $c^{p-q}$

${\left(A{R}^{p-1}\right)}^{q-r}$  ${\left(A{R}^{q-1}\right)}^{r-p}$  $\left(A{R}^{r-1}\right)$  $\because$ using I, II and III

$A^{q-r}$  $R^{\left(p-1\right)}$  $A^{r-p}$  $R^{\left(q-1\right)\left(r-p\right)}$  $A^{p-q}$  $R^{\left(r-1\right)\left(p-q\right)}$

$A^{\left[q-r+r-p+p-q\right]}$  $R^{\left[\left(p-1\right)\left(q-r\right)+\left(q-1\right)\left(n-p\right)+\left(x-1\right)\left(p-q\right)\right]}$

A0 R[pqprq+r+qrpqr+p+prqrp+q]  $$

$1\times$  $R^{\left[pq-pq+pr-pr+q-q+r-r+qr-qr+p-p\right]}$

= R

= 1

= R.H.S

 

Q23. If the first and the nth term of a G.P. are a and b, respectively, and if P is the product of n terms, prove

that P² = (ab)ⁿ.

A.23. Given, first term and xth term and a and b

Let r be the common ratio of the G.P.

Then,

Product of x terms, p=  $\left(a\right)$  $\left(ar\right)$  $\left(a{r}^2\right)$ ……  $\left(a{r}^{n-1}\right)$

p=  $\left(a\right)$  $\left(ar\right)\left(a{r}^2\right)$ ….  $\left(a{r}^{n-2}\right)\left(a{r}^{n-1}\right)$

= (a ×a× ….n term)(r´r²´r³ ….. $r^{n-2}\times r^{n-1}$ )

=  $a^x$  $r^{\left[1+2+3+....\left(n-2\right)+\left(n-1\right)\right]}$

p =  $a^n$  $r^{n\left(\frac{n-1}{2}\right)}$

So,  $p^2={\left[a^n{r}^n\left(\frac{n-1}{2}\right)\right]}^2$ [We know that,

=  $n\frac{\left(n-1\right)}{2}$ [  $1+2+3+...+n=\frac{\left(n-1\right)n}{2}$

=  $a^{2n}{r}^{2n}\left(\frac{n-1}{2}\right)$ [So,  $1+2+3+....+\left(n-1\right)=\frac{\left(n-1+1\right)\left(n-1\right)}{2}=\frac{n(n-1)}{2}$

=  $a^{2n}\times r^{n\left(n-1\right)}$

=  ${\left(a\times a{r}^{n-1}\right)}^n$  $[\because$  $a{n}^{n-1}=$ last term = b (given)]

=  ${\left(a\times b\right)}^n$

 

Q24. Show that the ratio of the sum of first nterms of a G.P. to the sum of terms from(n + 1)^th
to (2n)^th  term is $\frac{1}{r^n}$

A.24. Let a and r be the first term and common difference of the G.P

Thus, Sum of the first on term,  $S_n=\frac{a\left(1-r^n\right)}{1-r}$

Let Sn = sum of term from (n+1)^th to (2n)^th  term

=  $a{r}^n+a{r}^{n-1}+......+a{r}^{2n-1}$

=  $\frac{a{r}^n\left[1-r^n\right]}{1-r}$

[  $\because$ the above is a G.P. with first term ar^x and common ratio =  $\frac{a{r}^{n+1}}{a{r}^n}=r^{n+1-n}=r$

and number of term from (2n)^th to (n+1)^th = n

So,  $\frac{S_n}{S_n}=\frac{\frac{a\left(1-r^n\right)}{1-r}}{\frac{a{r}^n\left(1-r^n\right)}{1-r}}$

=  $\frac{a}{a{r}^n}$

=  $\frac{1}{r^n}$

 

Q25. If a, b, c and d are in G.P. show that (a² + b² + c²) (b² + c² + d²) = (ab + bc+ cd)² .

A.25. Let r be the common ratio of the G.P. then,

First term = a₁ = a = a

$a_2=ar=b$

$a_3=a{r}^2=c$

$a_4=a{r}^3=d$

So, L.H.S.=  $\left(a^2+b^2+c^2\right)\left(b^2+c^2+d^2\right)$

=  $\left[a^2+{\left(ar\right)}^2+{\left(a{r}^2\right)}^2\right]\left[{\left(ar\right)}^2+{\left(a{r}^2\right)}^2+{\left(a{r}^3\right)}^2\right]$

=  $\left[a^2+a^2{r}^2+a^2{r}^4\right]\left[a^2{r}^2+a^2{r}^4+a^2{r}^6\right]$

=  $a^2\left[1+r^2+r^4\right]a^2{r}^2\left[1+r^2+r^4\right]$

=  $a^4{r}^2{\left[1+r^2+r^4\right]}^2$

R.H.S.=  ${\left(ab+bc+cd\right)}^2$

=  ${\left[a\times ar+ar.a{r}^2+a{r}^{2}a{r}^3\right]}^2$

=  ${\left[a^{2}r+a^2{r}^3+a^2{r}^5\right]}^2$  $$

= {  $a^{2}r{\left[1+r^2+r^4\right]}^2$ }

=  $a^4{r}^2{\left[1+r^2+r^4\right]}^2$

L.H.S. = R.H.S.

 

Q26. Insert two numbers between 3 and 81 so that the resulting sequence is G.P.

A.26. Let G₁ and G₂ be the two numbers between 3 and 81 so that 3, G₁, G₂, 81 is in G.P.

So, a = 3

a₄ = ar³ = 81 (when r = common ratio)

$\Rightarrow$  $r^3=\frac{81}{a}=\frac{81}{3}$

$\Rightarrow$ r³ = 27

$\Rightarrow$ r³ = 3³

$\Rightarrow$ r = 3

So, G1 = ar = 33=9 and G₂ = ar² 3´(3)² =27

 

 

 

 

Q30. The number of bacteria in a certain culture doubles every hour. If there were 30bacteria present in the culture originally, how many bacteria will be present at the end of 2^nd hour, 4^th hour and n^th hour ?

A.30. Since the numbers of bacteria doubles every hour. The number after every hour will be a G.P

So, a=30

r=2

$\therefore$ At end of 2^nd hour, a₃ (or 3^rd term) = $a{r}^2=30\times 2^2$

= 30×2⁴

= 120

At end of 4^th hour, a₅ (r 4^th  term) = $a{r}^4$

= 30×2⁴

= 30×16

= 480

Following the trend,

And at the end of nth hour, an+1=  $a{r}^{n+1-1}=a{r}^n$

= 30 ×2ⁿ

 

Q31. What will Rs 500 amounts to in 10 years after its deposit in a bank which pays annual interest rate of 10‰compounded annually?

A.31. Given,

Principal value, amount deposited, P= ₹500

Interest Rate, R= 10

Using compound interest = simple interest +  $\frac{P\times R\times time}{100}$

Amount at the end of 1st year

=  $500+\frac{500\times 10+1}{100}=500\left(1+0.1\right)=500\times 1.1$

= ₹500×(1.1)

Amount at the end of 2nd year

=  $500\left(1.1\right)+\frac{500\times 1.1\times 10\times 1}{100}$

=  $500\left(1.1\right)\left(1+0.1\right)$

= ₹500 (1.1)²

Similarly,

Amount at the end of 3rd year = ₹500(1.1)³

So, the amount will form a G.P.

₹ 500 (1.1), ₹ 500(1.1)² , ₹500 (1.1)³, ……….

$\therefore$ After 10 years = ₹500 (1.1)¹⁰

 

Q32. If A.M. and G.M. of roots of a quadratic equation are 8 and 5, respectively, then obtain the quadratic equation.

A.32. Let a and b be the roots of quadratic equation

So, A.M = 8

$\Rightarrow$  $\frac{a+b}{2}=8$ a + b =16 …..I

G.M. =  $\mathrm{<br>}$

$\Rightarrow$ ab = 25….. II

We know that is a quadratic equation

$x^2-x$ (sum of roots) + product of roots = 0

$x^2-x\left(a+b\right)+ab=0$

$x^2-16x+25=0$ using I and II

Which is the reqd. quadratic equation  $$

 

Ex 9.4

Find the sum to n terms of each of the series in Exercises 1 to 7.

Q1. 1 × 2 + 2 × 3 + 3 × 4 + 4 × 5 +...

A.1. Given series is 1×2+2 ×3+3× 4+4× 5+…

So, an (nth term of A.P 1, 2, 3…)(nth term of A.P. 2, 3, 4, 5…)

i e, a = 2, d = 2 -1 = 1i e, a = 2, d = 3 - 2 = 1

= [1 + (n- 1) 1] [2 + (n -1) 1]

= [1 + n- 1] [2 + n -1]

= n(n -1)

= n²-n.

S_n (sum of n terms of the series) = ∑n² + ∑n.

S_n = $\frac{n(n+1)(2n+1)}{6}$ +  $\frac{n(x+1)}{2}$

=  $\frac{n(n+1)}{2}\left[\frac{2n+1}{3}+1\right]$

=  $\frac{n(n+1)}{2}\left[\frac{2n+1+3}{3}\right]$

$=\frac{n(n+1)}{2}\times \frac{(2n+4)}{3}$

$=\frac{n(n+1)\times 2(n+2)}{2\times 3}$

$=\frac{n(n+1)(n+2)}{3}$

 

Q2. 1 × 2 × 3 + 2 × 3 × 4 + 3 × 4 × 5 + ...

A.2. Given series is 1× 2× 3 + 2× 3 ×4 + 3× 4 ×5 + … tonterm

Solution:

a_n = (n^th term of A. P. 1, 2, 3, …) ´× (n^th terms of A. P. 2, 3, 4) ×

i e, a = 1, d = 2- 1 = 1i e, a = 2, d = 3- 2 = 1

(nth term of A. P. 3, 4, 5)

i e, a = 3, d = 3 -4 = 1.

= [1 + (n -1) 1] ×[2 + (n -1):1]× [3 + (n- 1) 1]

= (1 + n -1)×(2 + n -1)×(3 + n -1)

= n (n + 1)(n + 2)

= n(n² + 2n + n + 2)

=n³ + 2n² + 2n.

S_n = ∑n³ + 3 ∑n² + 2 ∑n

$={\left[\frac{m(n+1)}{2}\right]}^2+\frac{3n(n+1)(2n+1)}{6}+\frac{2n(n+1)}{2}$

=  $\frac{n(n+1)}{2}\left[\frac{n(n+1)}{2}+\frac{3}{3}(2n+1)+2\right]$

=  $\frac{n(n+1)}{2}\left[\frac{n^2+n}{2}+2n+1+2\right]$

$=\frac{n(n+1)}{2}\left[\frac{n^2+n+2\times 2n+2\times 3}{2}\right]$

$=\frac{n(n+1)}{2}\left[\frac{x^2+n+4x+6}{2}\right]$

$=\frac{n(n+1)}{2}\times \frac{\left[n^2+5n+6\right]}{2}$

$=\frac{n(n+1)}{4}\left[n^2+2n+3n+6\right]$

$=\frac{n(n+1)}{4}[n(n+2)+3(n+2)]$

$=\frac{n(n+1)(n+2)(n+3)}{4}$

 

Q3. 3 × 1² + 5 × 2² + 7 × 3² + ...

A.3. The given series is 3 × 1² + 5 × 2² + 7 × 3² + …..

So,a_n = (n^th term of A P 3, 5, 7, ..) (n^th term of A P 1, 2, 3, ….)²

a = 3, d = 5 -3 = 2a = 1, d = 2 -1 = 1.

= [3 + (n- 1) 2] [1 + (n- 1) 1]²

=[3 + 2n- 2] [1 + n- 1]²

(2n + 1)(n)²

= 2n³ + n²

So, = 5n2∑n³ + ∑n²

$=2·{\left[\frac{n^{}(n+1)}{2}\right]}^2+\left[\frac{n(n+1)(2n+1)}{6}\right]$

$=\frac{n(n+1)}{2}\left[2·\frac{n(n+1)}{2}+\frac{(2n+1)}{3}\right]$

$=\frac{n(n+1)}{2}\left[\frac{3n(n+1)+(2n+1)}{3}\right]$

$=\frac{n(n+1)}{2}\left[\frac{3n^2+3n+2n+1}{3}\right]$

$=\frac{n}{2}(n+1)·\left[\frac{3n^2+5n+1}{3}\right]$

$\frac{n(n+1)\left(3n^2+5n+1\right)}{6}$

 

Q4.  $\frac{1}{1\times 2}+\frac{1}{2\times 3}+\frac{1}{3\times 4}+\cdots$

A.4. The given series is $\frac{1}{1\times 2}+\frac{1}{2\times 3}+\frac{1}{3\times 4}+\cdots$

So, an =  $\frac{1}{\left(n^{}1,2,3\dots \right)}\times \frac{1}{\left(n^{ }ten 2,3,4\dots \right)}$

$=\frac{1}{[1+(n-1)1]}\times \frac{1}{[2+(n-1)1]}$

$=\frac{1}{(1+x-1)}\times \frac{1}{(2+n-1)}$

$=\frac{1}{n(n+1)}$

$=\frac{(n+1)-n}{n(n+1)}$

$=\frac{(n+1)}{n(n+1)}-\frac{n}{n(n+1)}$

$a_n=\frac{1}{n}-\frac{1}{n+1}$

So. Putting n = 1, 2, 3….n.

a₁ = $\frac{1}{1}-\frac{1}{2}$

a₂ = $\frac{1}{2}-\frac{1}{3}$

a₃ = $\frac{1}{3}-\frac{1}{4}\dots .$

So, adding. L.H.S and R.H.S. up ton terms

a₁ + a² + a₃ + … + a_n = $\left[\frac{1}{1}+\frac{1}{2}+\frac{1}{3}+\cdots \frac{1}{n}\right]-[\frac{1}{2}+\frac{1}{3}+\frac{1}{4}+\cdots ·\frac{1}{n}+\frac{1}{n+1}]$

 S_n = 1 $\frac{1}{n+1}$ { equal terns cancelled out}

 S_n = $\frac{n+1-1}{.n-1}$

 S_n = $\frac{n}{n+1}$

 

Q5. 5² + 6² + 7² + ... + 20²

A.5. The given series is 5² + 6² + 7² + … + 20²

This can be rewritten as(1² +2² + 3² + 4² + 5² + 6² + 7² + … + 20²) - (1² + 2² + 3² + 4²)

So, sum = (1² + 2² + 3² + 4² … + 20²) - (1² + 2²+ 3² + 4²)

$=$ ${\displaystyle \underset{}{\overset{}{}}}$ ${\displaystyle \underset{i=1}{\overset{20}{\sum }}{n}^2}-{\displaystyle \underset{i=1}{\overset{4}{\sum }}{n}^2}$

$=\frac{20(20+1)(2\times 20+1)}{6}-\frac{4(4+1)(4\times 2+1)}{6}$

$=\frac{20\times 21\times 41}{6}-\frac{4\times 5\times 9}{6}$

= 2870 30

= 2840.

 

Q6. 3 × 8 + 6 × 11 + 9 × 14 + ...

A.6. The given series is 3 8 + 11 + 9 14 + …

So, a_n= (nth term of 3, 6, 9, …)(nth term of 8, 11, 14, …)

i e, a = 3, d = 6- 3 = 3i e, a= 8, d = 11- 8 = 3

= [3 + (n- 1) 3] [8 + (n -1) 3]

= [3 + 3n- 3] [8 + 3n -3]

= 3n (3n + 5)

= 9n2 + 15n.

So, S_n = 9∑n2 + 15∑ n.

$=9\times \frac{n(n+1)(2n+1)}{6}+15\times \frac{n(n+1)}{2}$

$=\frac{3}{2}n(n+1)(2n+1)+\frac{15}{2}n(n+1)$

$=\frac{3n}{2}(n+1)[2n+1+5]$

$=\frac{2n(n+1)}{2}[2n+6]$

 

= 3n (n + 1)(n + 3)

 

Q7. 1² + (1² + 2²) + (1² + 2² + 3²) + ...

A.7. The given series is 1² + (1² + 2²) + (1² + 2² + 3²) + …

So, nth term well be

a_n =1² + 2² + 3² + … + n².

$=\frac{n(x+1)(2n+1)}{6}$

$=\frac{n\left(2x^2+n+2n+1\right)}{6}$

$=\frac{n\left(2n^2+3n+1\right)}{6}$

$=\frac{2n^3+3n^2+n}{6}$

$=\frac{x^3}{3}+\frac{x^2}{2}+\frac{x}{6}$

So, S_n = $\frac{1}{3}\sum n^3+\frac{1}{2}\sum n^2+\frac{1}{6}{\displaystyle \underset{i=1}{\overset{n}{\sum }}n}$

$=\frac{1}{3}{n}^2\frac{{(n+1)}^2}{4}+\frac{1}{2}\frac{n(n+1)(2n+1)}{6}+\frac{1}{6}·\frac{n(n+1)}{2}$

$=\frac{n(x+1)}{6}\left[\frac{n(x+1)}{2}+\frac{(2n+1)}{2}+\frac{1}{2}\right]$

$=\frac{n(n+1)}{6}\left[\frac{n^2+n+2n+1+1}{2}\right]$

$=\frac{n(n+1)}{12}\left[n^2+5n+2n+2\right]$

$=\frac{n(n+1)(n+1)(n+2)}{12}$

$=\frac{n{(n+1)}^2(n+2)}{12}$

Find the sum to n terms of the series in Exercises 8 to 10 whose nth terms is given by

 

Q8. n(n+1) (n+4).

A.8. Given that,

an = n(n + 1)(n + 4)

= n(n² + 4n + n + 4)

=n(n² + 5n + 4)

= x³ + 5x² + 4x

So, sum of terms, S_n = $\sum n^3+5\sum n^2+4{\displaystyle \underset{}{\overset{}{\sum }}n}$

$={\left[\frac{n(n+1)}{2}\right]}^2+5\times \frac{n(n+1)(2n+1)}{6}+\frac{4\stackrel{\dot{}}{n}(n+1)}{2}$

$=\frac{n(n+1)}{2}\left[\frac{n(n+1)}{2}+\frac{5(2n+1)}{3}+4\right]$

$=\frac{n(n+1)}{2}\left[\frac{3n(n+1)+2\times 5(2n+1)+6\times 4}{6}\right].$

$=\frac{n(n+1)}{2}\left[\frac{3n^2+3n+20n+10+24}{6}\right]$

$=\frac{n(n+1)}{2}\left[\frac{3n^2+23n+34}{6}\right]$

$=\frac{n(n+1)\left(3n^2+23n+34\right)}{12}$

$=\frac{n(n+1)}{12}$  $\left(3n^2+6n+17n+34\right)$

$=\frac{n(n+1)}{12}[3n^2+23n+34]$

 

Q9. n² + 2^n.

A.9. Given,

a_n = n² + 2^n.

Substituting, n = 1, 2, 3, ….,n we get,

a₁ = 1² + 2¹

a₂ = 2² + 2²

a₃ = 3² + 2³

a_n = n² + 2ⁿ

Adding all the L.H.S and R.H.S correspondingly we get,

a₁ + a₂ + a₃ + … + a_n = (1² + 2² + 3² + …. + n²) + (2¹ + 2² + 2³ … + 2ⁿ)

 S_n $=\sum n^2+\frac{2\left(2^n-1\right)}{2-1}$ 2¹ + 2² +2³ + … form a G. P. with

a = 2

$n=\frac{2^2}{2}=2>1$

$=\frac{n(n+1)(2n+1)}{6}+\frac{2\left(2^n-1\right)}{1}$ and have n terms

Sum of G.P, S_n = $\frac{a\left(r^n-1\right)}{r-1}$

$=\frac{n}{6}(n+1)(2n+1)+2\left(2^n-1\right)$

 

Q10. (2n – 1)²

A.10. Given,

a_n = (2n- 1)²

=4n²- 2 ´ 2n´ 1 + (1)²

=4n²- 4n + 1

So, S_n = $4\sum n^2-4{\displaystyle \underset{}{\overset{}{\sum }}n}+{\displaystyle \underset{}{\overset{}{\sum }}1}$

$=\frac{4n(n+1)(2n+1)}{6}-4·\frac{n(n+1)}{2}+n$

$=n\left[\frac{2(n+1)(2n+1)}{3}-2(n+1)+1\right]$

$=n\left[\frac{2\left(2n^2+n+2n+1\right)-2\times 3(n+1)+3.}{3}\right]$

$=n\left[\frac{4n^2+6n+2-6n-6+3}{3}\right]$

$=\frac{n\left(4n^2-1\right)}{3}$

$=\frac{n}{3}\left[{(2n)}^2-1^2\right]$

$=\frac{n}{3}[(2n-1)(2n+1)]$  $[\because a^2-b^2=\left(\begin{array}{cc}\hfill a-b)\hfill & \hfill (a+b)\hfill \end{array}\right]$

 

Miscellaneous 9.

Q1. Show that the sum of (m + n)th and (m – n)th terms of an A.P. is equal to twice the m^th term.

A.1. Let a and d be the first term and common difference of the A.P.

So,  $a(m+n)+a(m-n)=\{a+[(m+n)-1]d\}+\{a+[[m-n)-1]d\}$

$=a+\left(m+n-1\right)d+a+\left(m-n-1\right)d$

$=2a+\left(m+n-1+m-n-1\right)d$

$=2a+\left(2m+0-2\right)d$

 

$=2[a+(m-1)d]$

= 2 am

 

Q2. If the sum of three numbers in A.P., is 24 and their product is 440, find the numbers.

A.2. Let the three numbers a  $-$ d, a, a + d be in A.P.

Then,  $\left(a-d\right)+a+\left(a+d\right)=24$

$\Rightarrow$ 3a = 24

$\Rightarrow$ a = 8

and,  $(a-d)\times a\times (a+d)=440$

$\Rightarrow (a-d)\left(a^2+ad\right)=440$

$\Rightarrow a^3+a^{2}d-a^{2}d-a{d}^2=440$

$\Rightarrow a^3-a{d}^2=440.$

$\Rightarrow a\left(a^2-d^2\right)=440$

Put,  $a=8,\Rightarrow 8\left(a^2-d^2\right)=440$

$\Rightarrow 64-d^2=\frac{440}{8}$

$\Rightarrow 64-d^2=55$

$d^2=64-55$

$\Rightarrow d^2=9$

$\Rightarrow d=\pm 3$

When d = 3, a = 8 the three number are.

8  $-$ 3, 8, 8 + 3 5,8,11

When d =  $-$ 3, a = 8 the three numbers are.

$8-(-3),8,8+(-3)\Rightarrow 8+3,8,8-3\Rightarrow 11,8,5$

 

Q3. Let the sum of n, 2n, 3n terms of an A.P. be S1, S2 and S3, respectively, show that S₃ = 3(S₂ – S₁)

A.3. Let a and d be the first term and common difference of an A.P.

Then,  $$

$S_n=\frac{n}{2}[2a+(n-1)d]=S_1$

$S_{2n}=\frac{2n}{2}[2a+(2n-1)d]=S_2$

$S_{3n}=\frac{3n}{2}[2a+(3n-1)d]=S_3$

Now, R.H.S  $=3\left(S_2-S_1\right)$

$=3\left\{\frac{2n}{2}[2a+(2n-1)d]-\frac{x}{2}[2a+(n-1)d]\right\}$

$=\frac{3n}{2}\{2[2a+(2n-1)d-[2a+(x-1)d]\}$

$=\frac{3n}{2}\{4a+(4x-2)d-2a-(x-1)d\}$

$=\frac{3n}{2}\{2a+\left[4x-2\left(n-1\right)d\right]\}$

$=\frac{3n}{2}\{2a+[4n-2-n+1]d\}$

$=\frac{3n}{2}\{2a+[3n-1]d\}=S_3$

$\therefore S_3=3\left(S_2-s_1\right)$

 

Q4. The smallest and largest no. between 200 and 400 which are divisible by 7 is

A.4.

$\begin{array}{ccccc}\hfill 7\frac{28}{200}\hfill & \hfill \frac{57}{400}\hfill & \hfill \frac{14}{\frac{60}{\frac{56}{4}}}\hfill & \hfill \frac{35}{\begin{array}{l}50\\ \frac{49}{1}\\ \end{array}}\hfill & \hfill \hfill \\ \hfill \hfill & \hfill Smallest =200-4+7=203\hfill & \hfill \hfill & \hfill Largest =400-1=399\hfill & \hfill \hfill \end{array}$

So we can form an A.P. 203,203+7, …..., 399-7, 399

So, i.e. a = 203 and d = 7  $$

l = 399

$\Rightarrow a+(x-1)d=399$

$\Rightarrow 203+(x-1)7=399$

$\Rightarrow (x-1)7=399-203$

$\Rightarrow x-1=\frac{196}{7}$

$\Rightarrow x=28+1$

$\Rightarrow x=29$

Sum of the 29 number of the AP = Required sum =  $\frac{\eta }{2}[a+l)$

$=\frac{29}{2}[203+399]$

$=\frac{29}{2}\times 602$

= 8729.

 

Q5. Find the sum of integers from 1 to 100 that are divisible by 2 or 5.

A.5.  An A.P. of numbers from 1 to 100 divisible by 2 is

2, 4, 6, ……….98, 100.

So, a = 2 and d = 4-2 = 2

$l=$ 100

$\Rightarrow a+(n-1)d=100$

$\Rightarrow 2+(n-1)2=100$

$\Rightarrow \frac{2}{2}+(n-1)\frac{2}{2}=\frac{100}{2}$

$\Rightarrow 1+(n-1)=50$

$\Rightarrow n=50$

Let,  $s_1=2+4+6\cdots +9+100=\frac{50}{2}[2+100]\left\{\because S_n=\frac{n}{2}(a+1)\right\}$

$=\frac{50\times 102}{2}$

= 2550

Similarly, an A.P. of numbers from 1 to 100 divisible by 5 is

5,10,15, …….. 95,100

So, a = 5 and d = 100

$l=$ 100

$\Rightarrow a+(n-1)d=100$

$\Rightarrow 5+(n-1)5=100$

$\Rightarrow 1+(n-1)=20$

$\Rightarrow n=20$

$\therefore S_2=5+10+15\dots +95+100=\frac{20}{2}[5+100]$

$=\frac{20\times 105}{2}$

= 1050

As there are also no divisible by both 2 and 5 , i.e., LCM of 2 and 5 = 10 An A.P. of no. from 1 to 100 divisible by 10 is 10, 20, …………100

So, a = 10, d = 10

$l=100$

$\Rightarrow a+(x-1)l=100$

$\Rightarrow 10+(x-1)10=100$

$\Rightarrow 1+(x-1)=10$

$\Rightarrow x=10$

So,  $S_3=10+20+30+\dots +100=\frac{10}{2}[10+100]$

= 550

The required sum of number  $=S_1+S_2{S}_3=2550+1050-550=3050$

= 3050

 

Q6. Find the sum of all two digit numbers which when divided by 4, yields 1 as remainder

A.6.Two digits no. when divided by 4 yields 1 as remainder are, 12+1, 16+1, 20+1 ….., 96+1

13, 17, 21, ………97 which forms an A.P.

So, a = 13

$d=17-13=4$

$l=97$

$\Rightarrow a+(x-1)d=97$

$\Rightarrow 13+(x-1)4=97$

$\Rightarrow \left(x-1\right)4=97-13=84$

$\Rightarrow x-1=\frac{84}{4}=21$

$\Rightarrow x=21+1=22$

Sum of numbers in A.P. =  $\frac{x}{2}(a+l)$

$=\frac{22}{2}(13+97)$

= 11× 110

= 1210

 

Q7. If f is a function satisfying f (x +y) = f(x) f(y) for all x, y  $\in$ N such that f(1) = 3 and  ${\displaystyle \underset{x=1}{\overset{n}{\sum }}f}(x)=120$ , find the value of n.

A.7. Given, $f(x+y)=f(x)\cdot f(y).Ux,y\in N$ and  $f\left(1\right)=3$

Putting (x, y) = (1+1) we get

Putting (x, y) = (1,1) we get,

$f(1+1)=f(1)\cdot f(1)=3\cdot 3=9$

$\Rightarrow f(2)=9$

And putting  $(x,y)=(1,2)$ we get,

$f(1+2)=f(1)\cdot f(2)=3\times 9=27$

$f(3)=27$

$f(1)+f(2)+f(3)+\cdots +f(x)={\displaystyle \underset{x=1}{\overset{n}{\sum }}f}(x)=120$ (Given)

As, With a = 3

$r=\frac{9}{3}=3>1$

We can write equation I as ,

$\frac{a\left(r^n-1\right)}{r-1}=120$

$\Rightarrow \frac{3\left(3^n-1\right)}{3-1}=120$

$\frac{3}{2}\left(3^n-1\right)=120$

$\Rightarrow \left(3^n-1\right)=120\times \frac{2}{3}$

3ⁿ 1 = 80

3ⁿ = 81 +1

3ⁿ = 81

3ⁿ = 3⁴

n = 4

 

Q8. The sum of some terms of G.P. is 315 whose first term and the common ratio are 5 and 2, respectively. Find the last term and the number of terms.

A.8. Given, a = 5

$r=2>1$

$s_n=315$

So,  $\frac{a\left(r^n-1\right)}{r-1}=315$

$\Rightarrow \frac{5\left(2^n-1\right)}{2-1}=315$

$\Rightarrow 2^n-1=\frac{315}{5}$

$\Rightarrow 2^n=63+1$

$\Rightarrow 2^n=64=26$

$\therefore n=6$

Hence, last term  $=a_6=a{r}^{6-1}=a{r}^5=5\times 2^5=5\times 32$

= 160

 

Q9. The first term of a G.P. is 1. The sum of the third term and fifth term is 90. Find the common ratio of G.P.

A.9. Given, a = 1

$a_3+a_5=90$

Let r be the common ratio of the G.P.

So,

Let r be the common ratio of the G.P.

So,

$a_3+a_5=a{r}^{3-1}+a{r}^{5-1}=90$

$\Rightarrow a\left[r^2+r^4\right]=90$

$\Rightarrow 1\left[r^2+r^4\right]=90\{\because a=1\}$

$\Rightarrow r^4+r^2-90=0$

Let  $x=r^2$ so we can write above equation as

$x^2+x-90=0;x=r^2$

 

 

Q10. The sum of three numbers in G.P. is 56. If we subtract 1, 7, 21 from these numbers in that order, we obtain an arithmetic progression. Find the numbers.

A.10. Let a, ar and $a{r}^2$ be the three nos. which is in G.P.

Then, a + ar + ar² = 56

a ( 1 + r + r²) =56  -I

Given, that a1, ar 7, ar² - 21 from an AP we have,

$(ar-7)-(a-1)=\left(a{r}^2-21\right)-(ar-7)$

$\Rightarrow ar-7-a+1=a{r}^2-21-ar+7$

$\Rightarrow ar-a-6=a{r}^2-ar-14$

$\Rightarrow a{r}^2-ar-ar+a-a-14-6$

$\Rightarrow a{r}^2-2ar+a=8$

$\Rightarrow a\left(r^2-2r+1\right)=8$ ………………. II

Now, dividing equation I by II we get,

$\Rightarrow \frac{a\left(1+n+n^2\right)}{a\left(r^2-2r+1\right)}=\frac{56}{8}$

$\Rightarrow 1+r+r^2=7\left(n^2-2n+1\right)$

$\Rightarrow 1+r+r^2=7r^2-14n+7$

$\Rightarrow 7r^2-14n+7-1-x-r^2=0$

$\Rightarrow 6r^2-15r+6=0$

$\Rightarrow 2r^2-5r+2=0$ (dividing by 3 throughout)

$\Rightarrow 2r^2-4r-r+2=0$

$\Rightarrow 2r(r-2)-(r-2)=0$

$\Rightarrow (r-2)(2r-1)=0$

$\Rightarrow r-2=02r-1=0$

$\Rightarrow r=2r=\frac{1}{2}$

So, when r = 2, putting in equation I,

$\Rightarrow a\left(1+2+2^2\right)=56$

$\Rightarrow a(1+2+4)=56$

$\Rightarrow a(7)=56$

$\Rightarrow a=\frac{56}{7}=8$

The numbers are 8, 8× 2, 8× 2² = 8, 16, 32.

And When  $r=\frac{1}{2}$ putting in equation I,

$a\left(1+\frac{1}{2}+\frac{1}{2^2}\right)=56$

$\Rightarrow a\left(1+\frac{1}{2}+\frac{1}{4}\right)=56$

$\Rightarrow a\left(\frac{4+2+1}{4}\right)=56$

$\Rightarrow a\times \frac{7}{4}=56$

$\Rightarrow a=56\times \frac{4}{7}=32$

So, the numbers are  $32,32\times \frac{1}{2},32\times {\left(\frac{1}{2}\right)}^2\Rightarrow 32,16,8$

 

Q11. A G.P. consists of an even number of terms. If the sum of all the terms is 5 times the sum of terms occupying odd places, then find its common ratio.

A.11. Let a and r be the first term and common ratio of G.P.

Then, number of term = 2n (even).

$a_1+a_2+\cdots +a_{2n}=5\left(a_1+a_3+\cdots +a_{2n-1}\right)[\because ]$

$\Rightarrow a+ar+.........+a{r}^{2n-1}=5\left(a+a{r}^2+.......+a{r}^{2n-1-1}\right)$

$\Rightarrow \frac{a\left(1-r^{2n}\right)}{1-n}=5\times \frac{a\left[1-{\left(r^2\right)}^n\right]}{1-r^2}$ { series on R.H.S. has  $\frac{2n}{2}=n$ terms and common ratio  $\frac{a{r}^2}{a}=r^2$ }

$\Rightarrow \frac{1-r^{2n}}{1-r}=\frac{5\left[1-r^{2n}\right]}{1-r^2}$ (eliminating a)

$\Rightarrow \frac{1-r^{2r}}{1-r}=\left[\frac{1-r^{2r}}{1-r}\right]\times \frac{5}{1+r}\left\{\because a^2-b^2=(a-b)(a+b)\right\}$  $$

$\Rightarrow 1=\frac{5}{1+r}\{Eliminatingsameterm\}$

$\Rightarrow 1+r=5$

$\Rightarrow r=5-1$

r = 4

 

Q12. The sum of the first four terms of an A.P. is 56. The sum of the last four terms is 112. If its first term is 11, then find the number of terms.

A.12. Given, a = 11

Let d and l be the common difference & last term of the A.P.

Then,  $a+(a+d)+(a+2d)+(a+3d)=56$ [first 4 terms sum]

$\Rightarrow 4a+6d=56$

$\Rightarrow 6d=56-4a=56-4\times 11=56-44=12$

$\Rightarrow d=\frac{12}{6}=2$

And,  $l+(l-d)+(l-2d)+(l-3d)=112$

$\Rightarrow 4l-6d=112$

$\Rightarrow 4l=112+6d=112+6\times 2=112+12=124$ [last 4 terms sum]

$\Rightarrow l=\frac{124}{4}=31$

So,  $l=31$

$\Rightarrow a+{(n-1)}^d=31$

$\Rightarrow 11+{(n-1)}^2=31$

$\Rightarrow (n-1)2=31-11=20$

$\Rightarrow n-1=\frac{20}{2}=10$

$\Rightarrow n=10+1$

$\Rightarrow n=11$

the A.P. has 11 number of terms.

 

Q13. If  $\frac{a+bx}{a-bx}=\frac{b+cx}{b-cx}=\frac{c+dx}{c-dx}$ ( x ≠ 0) , then show that a, b, c and d are in G.P.

A.13. Here, $\frac{a+bx}{a-bx}=\frac{b+cx}{b-cx}$

$\Rightarrow (a+bx)(b-cx)=(b+cx)(a-bx)$

$\Rightarrow ab-acx+b^{2}x-bc{x}^2=ab-b^{2}x+acx-bc{x}^2$

$\Rightarrow ab-ab-acx-acx=-b^{2}x-b^{2}x+bc{x}^2-bc{x}^2$

$\Rightarrow -2acx=-2b^{2}x$

$\Rightarrow ac=b^2$

$\Rightarrow \frac{c}{b}=\frac{b}{a}$ …………I

And  $\frac{b+cx}{b-cx}=\frac{c+dx}{c-dx}$

$\Rightarrow (b+cx)(c-dx)=(c+dx)(b-cx)$

$\Rightarrow bc-bdx+c^{2}x-cdx=bc-c^{2}x+bdx-cdx$

$\Rightarrow bc-bc+c^{2}x+c^{2}x=bdx+bdx-cdx+cdx$  $$

$\Rightarrow 2c^{2}x=2bdx$

$\Rightarrow c^2=bd$

$\Rightarrow \frac{c}{b}=\frac{d}{c}$ ……………II

From I and II

$\frac{a}{a}=\frac{c}{b}=\frac{d}{c}$

a, b, c and d are in G.P.

 

Q14. Let S be the sum, P the product and R the sum of reciprocals of n terms in a G.P.

Prove that P²Rⁿ = Sⁿ.

A.14. Let a and r be the first term & common ratio of the G.P.

So, S = a +ar + ar² +……… upto n terms.

$S=\frac{a\left(1-r^n\right)}{1-r}$

and P = a .ar. ar² ar .... upton n terms.

$=a^n{r}^{1+2+3+\dots +(n-1)}$

$=a^{n}r\frac{(n-1)(n-1+1)}{2}$

$=a^{n}r\frac{n(n-1)}{2}$

And R = sum of reciprocal of n terms (  $\frac{1}{a}+\frac{1}{a{r}^n}+...........$ upto n terms)

$=\frac{\frac{1}{a}\left[{\left(\frac{1}{r}\right)}^n-1\right]}{\frac{1}{r}-1}$  $$ As r <1

$\frac{1}{r}$ >1

$=\frac{\frac{1}{a}\left[\frac{1}{r^n}-1\right]}{\frac{1-r}{r}}=\frac{1}{a}\left[\frac{1-r^n}{r^n}\right]\times \frac{r}{1-r}$

$=\frac{1-r^n}{a{r}^n}\times \frac{r}{1-r}$

$=\frac{1-r^n}{a(1-r)r^{n-1}}$ …. III

Now, L.H.S. = P² Rⁿ

$={\left[a^{n}r\frac{n\left(n-1\right)}{2}\right]}^2·{\left[\frac{1-x^n}{a(1-n)r^{n-1}}\right]}^n$ { equation II & III}

$=a^{2n}\cdot r^{n(n-1)}\times \frac{{\left[1-r^n\right]}^n}{a^n(1-n){}^n}{r}^{n(n-1)}$

$=a^{2x-n}\times \frac{r^{n(x-1)}}{r^{n(n-1)}}\times \frac{{\left[1-x^n\right]}^n}{{(1-r)}^n}$

$=\frac{a^n{\left[1-r^n\right]}^n}{{(1-r)}^n}$

$={\left[\frac{a\left[1-r^n\right]}{(1-r)}\right]}^n$

$=S^n=$R.H.S { $\because$ equation I}

 

Q15. The p^th, q^th and r^th te.rms of an A.P. are a, b, c, respectively. Show that (q – r )a + (r – p )b + (p – q )c = 0

A.15. Let A and d be the first term & common difference of the A.P.

Then,

$ap=a\to A+(p-1)d=a$ ………I

$a_r=c\to A+(M-1)d=c$ …………III

So, L.H.S.  $=(q-1)a+(n-p)b+(q-q)c$

$=(q-r)[A+(p-1)d]+(n-p)[A+(q-1)d]+(p-q)[A+(r-1)d]$

{putting value for I, II, III}

$\Rightarrow Aq+q(p-1)d-Ar-r(p-1)d+Ar+r\left(q-1\right)d-Ap-p\left(q-1\right)d$

$+Ap+p\left(r-1\right)d-Aq-q\left(r-1\right)d$

$\Rightarrow pqd-qd-rpd+rd+rqd-rd-pqd+pd+rpd-pd-rqd+qd$

$=0=$ R.H.S $$

 

Q16. If  $a\left(\frac{1}{b}+\frac{1}{c}\right),b\left(\frac{1}{c}+\frac{1}{a}\right),c\left(\frac{1}{a}+\frac{1}{b}\right)$ are in A.P., prove that a, b, c are in A.P

A.16. Given, $a\left(\frac{1}{b}+\frac{1}{c}\right),b\left(\frac{1}{c}+\frac{1}{a}\right),c\left(\frac{1}{a}+\frac{1}{b}\right)$ are in A.P.

So,  $\frac{a(c+b)}{bc},\frac{b(a+c)}{ac},\frac{c(b+a)}{ab}$ are in A.P.

$\Rightarrow \frac{ac+ab}{bc},\frac{ab+bc}{ac},\frac{bc+ac}{ab}$ are in A.P.

If we add 1 to all each terms of the sequence it will given be an A.P of common difference 1.

So,  $\frac{ac+ab}{bc}+1,\frac{ab+bc}{ac}+1,\frac{bc+ac}{ab}+1$ are in A.P.

$\Rightarrow \frac{ac+ab+bc}{bc},\frac{ab+bc+ac}{ac},\frac{Bc+ac+ab}{ab}$ are in A.P.

Dividing add of the sum by ab + bc + ac will conserve.

then A.P so,

$\Rightarrow \frac{1}{bc},\frac{1}{ac},\frac{1}{ab}$ are in A.P.

Similarly multiplying each term by abc we get,

$\Rightarrow \frac{abc}{bc},\frac{abc}{ac},\frac{abc}{ab}$ are in A.P.

a, b, c, are in A.P.

Hence proved

 

Q17. If a, b, c, d are in G.P, prove that (aⁿ + bⁿ), (bⁿ + cⁿ), (cⁿ + dⁿ) are in G.P.

A.17. Let r be the common ratio of the G.P.

Then, a, b, c, d a, ar, ar², ar³

So,  $a^n+b^n=a^n+{(ar)}^n=a^n+a^n{r}^n=a^n\left(1+r^n\right)-(1)$

$b^n+c^n={(ar)}^n+{\left(a{r}^2\right)}^n=a^n{r}^n+a^n{r}^{2n}=a^n{r}^n\left(1+r^n\right)$ (2)

$c^n+d^n={\left(a{r}^2\right)}^n+{\left(a{r}^3\right)}^n=a^n{r}^{2n}+a^n{r}^{3n}=a^n{r}^{2n}\left(1+r^n\right)$ (3)

Hence,  $\frac{b^n+c^n}{a^n+b^n}=\frac{a^n{r}^n\left(1+r^n\right)}{a^n\left(1+r^n\right)}=r^n$ {from (2) and (1)}

and  $$  $\frac{c^n+d^n}{b^n+c^n}=\frac{a^n{r}^{2n}\left(1+r^2\right)}{a^n{r}^n\left(1+r^2\right)}=r^n$ {from (3) and (2)}

i.e.,  $\frac{b^n+c^n}{a^x+b^n}=\frac{c^n+d^n}{b^x+c^n}$

$\therefore a^n+b^n,b^n+c^n,c^n+d^n$ are in A.P

 

Q18. If a and b are the roots of x² – 3x + p = 0 and c, d are roots of x² – 12x + q = 0, where a, b, c, d form a G.P. Prove that (q + p) : (q – p) = 17:15.

A.18. Given, ab are roots of $x^2-3x+p=0$

and c & d are roots of  $x^2-12x+q=0$

So,  $\Rightarrow a+b=\frac{-\left(-3\right)}{1}$ and  $ab=\frac{P}{1}$

a + b = +3 …………..I and ab = P…………….II {  $\because$ sum of roots =  $\frac{-B}{A}$ , Product of roots =  $\frac{-C}{A}$ }

Similarly, c + d  $=\frac{-(-12)}{1}$ and  $cd=\frac{q}{1}$

c + d = 12 ……….III ad cd = q (4)

As a, b, c, d from a G.P and if r be the common ratio

a = a

b = ar

c = ar²

d = ar³

So, from equation, (1),

$a+b=3\Rightarrow a+ar=3\Rightarrow a(1+r)=3$ (5)

And  $c+d=12\Rightarrow a{r}^2+a{r}^3=12\Rightarrow a{r}^2(1+r)=12$ (6)

Dividing equation (6) and (5) we get,

$\frac{a{r}^2(1+r)}{a(1+r)}=\frac{12}{3}$

 r² = 4

Now, L.H.S.  $=\frac{q+p}{q-p}=\frac{cd+ab}{cd-ab}$ {from (4) and (5)}

$=\frac{a{r}^2\times a{r}^3+a\times ar}{a{r}^2+a{r}^3-a\times ar}$

$=\frac{a^{2}r\left(r^4+1\right)}{a^{2}r\left(r^4-1\right)}$

$=\frac{{\left(r^2\right)}^2+1}{{\left(r^2\right)}^2-1}=\frac{4^2+1}{4^2-1}=\frac{16+1}{16-1}=\frac{17}{15}$ = R.H.S.

 

 

Q20. If a, b, c are in A.P.; b, c, d are in G.P. and  $\frac{1}{c},\frac{1}{d},\frac{1}{e}$ are in A.P. prove that a, c, e are in G.P.

A.20. As a, b, c are in A.P. we can write,

$b-a=c-b$

$\Rightarrow b+b=a+c$

$\Rightarrow 2b=a+c$ I

As b, c, d are in G.P. we can write,

$\frac{c}{b}=\frac{d}{c}$

$\Rightarrow c^2=bd$ II

And ar  $\frac{1}{c},\frac{1}{d},\frac{1}{e}$ are in A.P. we can write,

$\Rightarrow \frac{1}{d}-\frac{1}{c}=\frac{1}{e}-\frac{1}{d}$

$\Rightarrow \frac{1}{d}+\frac{1}{d}=\frac{1}{c}+\frac{1}{e}$

$\Rightarrow \frac{2}{d}=\frac{e+c}{ce}$

$\Rightarrow \frac{d}{2}=\frac{ce}{e+c}$

$\Rightarrow d=\frac{2ce}{c+e}$ …………. III

Now,  $c^2=Bd$ from II

$\Rightarrow =\frac{a+c}{2}\times \frac{2ce}{c+c}$ {from 1 and 3}

$\Rightarrow c^2=\frac{(a+c)ce}{c+e}$

$\Rightarrow c=\frac{(a+c)e}{(c+e)}$

$\Rightarrow c(c+e)=(a+c)e$

$\Rightarrow c^2+ce=ac+ce$

$\Rightarrow c^2=ae$

$\Rightarrow \frac{c}{a}=\frac{e}{c}$

i.e., a, c and e are in G.P.

 

Q21. Find the sum of the following series up to n terms:

(i) 5 + 55 +555 + … (ii) .6 +. 66 +. 666+…

A.21. (i) Sn = 5+55+555+…………… upto n term

=5(1+11+111+ …………….upto n term )

Multiplying and dividing by 9 we get

$=\frac{5}{9}(9+99+999+\dots upto\mathrm{nterms})$

 

$=\frac{5}{9}\left[\frac{10\left(10^n-1\right)}{10-1}-n\right]$ {  $\because 10+10^2+10^3+\dots \mathrm{ntermsisaG.Pof}a=10,r=10>1\mathrm{nterms}$ }

$=\frac{5}{9}\times \left[\frac{10}{9}\left(10^n-1\right)-n\right]$

$=\frac{50}{81}\left(10^n-1\right)-\frac{5n}{9}$

ii)  $S_n=0.6+0.66+0.666+\dots n$

$=6[0.1+0.11+0.111+\cdots uptonterms\mathrm{<br>}]$

$=\frac{6}{9}[0.9+0\cdot 99+0.999+\cdots \mathrm{<math>\; <mrow>\; <mo>\; uptonterms\; </mo>\; </mrow>\; </math>}]$ {multiplying and dividing by 9}

$=\frac{6}{9}[(1+1+1\dots n)-(0.1+0.01+0.001+\dots \mathrm{<math>\; <mrow>\; <mo>\; uptonterms\; </mo>\; </mrow>\; </math>})]$

$=\frac{6}{9}\left[n-\left(\frac{1}{10}+\frac{1}{10}\times \frac{1}{10}+\frac{1}{10}\times {\left(\frac{1}{10}\right)}^2+\cdots \mathrm{<math>\; <mrow>\; <mo>\; uptonterms\; </mo>\; </mrow>\; </math>}\right)\right]$

$=\frac{6}{9}\left[n-\frac{\frac{1}{10}\left(1-{\left(\frac{1}{10}\right)}^n\right)}{1-\frac{1}{10}}\right]$

$=\frac{6}{9}\left[n-\frac{1-{\left(\frac{1}{10}\right)}^n}{10-1}\right]$

$=\frac{6}{9}\left[x-\frac{1}{9}\left(1-\frac{1}{10^n}\right)\right]$

$=\frac{6}{9}\times \frac{1}{9}\left[9x-\left(1-\frac{1}{10^n}\right)\right]$

$=\frac{2}{27}\left[9n-\left(1-\frac{1}{10^n}\right)\right]$

$=\frac{2}{27}\left[9n-1+\frac{1}{10^n}\right]$

 

Q22. Find the 20th term of the series 2 × 4 + 4 × 6 + 6 × 8 + ... + n terms.

A.22. Given, series is $2\times 4+4\times 6+6\times 8+\dots +n$ terms.

So, So, a₂₀ = ( 20th term of A.P. 2, 4, 6 …..) ( 20th term of A.P. 4,6,8 ………)

i.e. a = 2, d = 4 -2 = 2 i.e. a =4, d = 6- 4 = 2

= [2 + (20- 1)2] [4 + (20-1)2]

= [2+192] [4 +19 2]

= (2+38) (4+38)

= 40 42 = 1680

 

Q23. Find the sum of the first n terms of the series: 3+ 7 +13 +21 +31 +…

A.23. The given series is 3+7+13+21+31+ …….. upto n terms

So, Sum, S_n = 3+7+13+21+31+ ………….+ an1 + an

Now, taking,

S_n = S_n = [ 3 + 7 + 13 + 21 + 31 + ………. an1 + an ] [ 3+ 7 + 21 + 31 + … a_n-1 + a_n]

0 = [ 3+ (7 3) + (13 7) + (13 7) + (21 13) + …….+ (an an1) an]

0 = 3 + [ 4 + 6 + 8 +……..+ (n-1) terms] a_n

$\Rightarrow a_n=3+\left\{\frac{n-1}{2}[2\times 4+[(n-1)-1]2]\right\}$ {  $\because$ 4 + 6 + 8 + …….. is sum of A.P. of n 1 terms with a = 4, d = 6- 4 = 2}

$\Rightarrow a_n=3+\left\{\frac{n-1}{2}[8+(n-2)2]\right\}$

$\Rightarrow a_n=3+\left\{\frac{n-1}{2}\times 2[4+n-2]\right\}=3+(n-1)(n+2)$

a_n = 3 + n² + (1 + 2) n + (-1) (2) { $\because$ (a + b) (a + b) = a2 + (b + c) a + bc }

a_n = 3 + n² + n- 2 = n² + n +1

sum of series,  $S_n={\displaystyle \underset{}{\overset{}{\sum }}{a}_n}={\displaystyle \underset{}{\overset{}{\sum }}{n}^2}+{\displaystyle \underset{}{\overset{}{\sum }}n}+{\displaystyle \underset{}{\overset{}{\sum }}1}$

$=\frac{n(n+1)(2n+1)}{6}+\frac{n(x+1)}{2}+n$

$=n\left[\frac{(n+1){(2n+1)}^{}}{6}+\frac{n+1}{2}+1\right]$

$=n\left[\frac{(n+1)(2n+1)+3(n+1)+6}{6}\right]$

$=n\left[\frac{2n^2+n+2n+1+3n+3+6}{6}\right]$

$$  $=n\left[\frac{2n^2+6n+10]}{6}\right]$

$=n\left[\frac{2\left(n^2+3n+5\right)}{6}\right]$

$=\frac{n\left(n^2+3n+5\right)}{3}$

 

Q24. If S₁, S₂, S₃ are the sum of first n natural numbers, their squares and their cubes, respectively, show that 9 S₂² = S₃ (1 + 8S₁).

A.24. Given,

S₁ = 1 + 2 + 3 + ………. + n $=n+\frac{(n+1)}{2}$

S₂ = 1² + 2² + 3² + ………… + n²  $=\frac{n(n+1)(2n+1)}{6}$

S₃ = 1³ + 2³ + 3³ + …………. + n³ = ${\left[\frac{n(n+1)}{2}\right]}^2$

So, L.H.S.  $=9S_2^2=9\times {\left[\frac{n(n+1)(2n+1)}{6}\right]}^2$

$=\frac{9\times n^2(n+1){}^2(2n+1){}^2}{36}$

$=\frac{n^2(n+1){}^2(2n+1){}^2}{4}$

R.H.S.  $=S_3\left(1+8S_1\right)={\left[\frac{n(n+1)}{2}\right]}^2\left[1+8\times \frac{n(n+1)}{2}\right]$

$=\frac{n^2(n+1){}^2}{4}\times [1+4n(n+1)]$

$=\frac{n^2(n+1){}^2}{4}\left[1+4n^2+4n\right]$

$=\frac{n^2(n+1){}^2}{4}\left[{(2n)}^2+2(2n)1+1^2\right]$

$=\frac{n^2(n+1){}^2}{4}{(2n+1)}^2=$ L.H.S.

So, 9(S₂)² = S₃ ( 1 + 8S₁)

 

Q25. Find the sum of the following series up to n terms:

$\frac{1^3}{1}+\frac{1^3+2^3}{1+3}+\frac{1^3+2^3+3^3}{1+3+5}+\dots$

A.25. The given series is.

$\frac{1^3}{1}+\frac{1^3+2^3}{1+3}+\frac{1^3+2^3+3^3}{1+3+5}$ +………….. upto nth term,

The nth term is ,

$a_n=\frac{1^3+2^3+3^3+........+n^3}{1+3+5+........+n...terms}$ { A.P. of n terms and a = 1, d = 5-3 = 2}

$a_n=\frac{{\left[\frac{n(n+1)}{2}\right]}^2}{\frac{n}{2}[2\times 1+(n-1)2]}$

$a_n=\frac{\frac{n^2(n+1){}^2}{4}}{\frac{n}{2}[2+(n-1)2]}=\frac{\frac{n^2(n+1){}^2}{4}}{n[1+n-1]}$

$a_n=\frac{\frac{n^2(n+1){}^2}{4}}{n^2}=\frac{{(n+1)}^2}{4}$

$a_n=\frac{n^2+2x+1}{4}=\frac{n^2}{4}+\frac{n}{2}+\frac{1}{4}$

$\therefore Sn={\displaystyle \underset{}{\overset{}{\sum }}an}=\frac{1}{4}{\displaystyle \underset{}{\overset{}{\sum }}{x}^2}+\frac{1}{2}{\displaystyle \underset{}{\overset{}{\sum }}n+{\displaystyle \underset{}{\overset{}{\sum }}1}}$

$=\frac{1}{4}\times \frac{9(n+1)(2n+1)}{6}+\frac{1}{2}\times \frac{n(x+1)}{2}+\frac{1}{4}\times n$

$=\frac{n}{4}\left[\frac{(n+1)(2n+1)}{6}+(n+1)+1\right]$

$=\frac{n}{4}\left[\frac{2n^2+n(n+1)(2n+1)+6(x+1)+6}{6}\right]$

$=\frac{n}{4}\left[\frac{2n^2+n+2n+1+6n+6+6}{6}\right]$

$=\frac{n}{4}\left[\frac{2n^2+9n+13}{6}\right]$

$=\frac{n\left(2n^2+9n+13\right)}{24}$

 

Q26. Show that  $\frac{1\times 2^2+2\times 3^2+\cdots +n{(n+1)}^2}{1^2\times 2+2^2+3+\cdots +n^2(n+1)}=\frac{3n+5}{3n+1}$

A.26. Given, $\frac{1\times 2^2+2\times 3^2+\cdots +n{(n+1)}^2}{1^2\times 2+2^2+3+\cdots +n^2(n+1)}$

For numerator,

a  (n^th term) = n (n + 1)² = n (n + 1)² = n (n² + 2n +1) = n³ + 2n² + n

So,  $S_n={\displaystyle \underset{}{\overset{}{\sum }}{a}_n}={\displaystyle \underset{}{\overset{}{\sum }}{n}^3}+2{\displaystyle \underset{}{\overset{}{\sum }}{n}^2}+{\displaystyle \underset{}{\overset{}{\sum }}n}$

$=\frac{n^2(n+1){}^2}{4}+\frac{2\cdot (n+1)(2n+1)n}{6}+\frac{n(n+1)}{2}$

$=\frac{n(n+1)}{2}\left[\frac{n(n+1)}{2}+\frac{2(2n+1)}{3}+1\right]$

$=\frac{n(n+1)}{2}\left[\frac{3n(n+1)+2\times 2(2n+1)+6}{6}\right]$

$=\frac{n(n+1)}{12}\left[3n^2+3n+8n+4n+6\right]$

$=\frac{n(n+1)}{12}\left[3n^2+11n+10\right]$

$=\frac{n(n+1)}{12}\left[3n^2+6n+5n+10\right]$

$=\frac{n(n+1)}{12}[(33n(n+2)+5(n+2)]$

$=\frac{n(n+1)(n+2)(3n+5)}{12}$

For denominator,

a_n (n^th term) = n² (n + 1) = n³ + n²

So,

$S_n={\displaystyle \underset{}{\overset{}{\sum }}{a}_n}={\displaystyle \underset{}{\overset{}{\sum }}{n}^3}+{\displaystyle \underset{}{\overset{}{\sum }}{n}^2}$

$=\frac{n^2(n+1){}^2}{4}+\frac{n(n+1)(2n+1)}{6}$

$=\frac{n(n+1)}{2}\left[\frac{n(n+1)}{2}+\frac{(2n+1)}{3}\right]$

$=\frac{n(n+1)}{2}\left[\frac{3n(n+1)+2(2n+1)}{6}\right]$

$=\frac{n(n+1)}{2}\left[\frac{3n^2+3n+4n+2}{6}\right]$

$=\frac{n(n+1)}{2}\left[\frac{3n^2+6n+1n+2}{6}\right]$

$=\frac{n(n+1)}{2}\left[\frac{3n(n+2)+(n+2)}{6}\right]$

$=\frac{n(n+1)(n+2)(3n+1)}{12}]$

So,  $\frac{1\times 2^2+2\times 3^2+\cdots +n{(n+1)}^2}{1^2\times 2+2^2\times 3+\cdots +n^2(n+1)}=\frac{\frac{n(n+1)(n+2)(3n+5)}{12}}{\frac{n(n+1)(n+2)(3n+1)}{12}}$

$=\frac{3n+5}{3n+1}$

 

Q27. A farmer buys a used tractor for Rs 12000. He pays Rs 6000 cash and agrees to pay the balance in annual instalments of Rs 500 plus 12% interest on the unpaid amount. How much will the tractor cost him?

A.27. Given,

cost of tractor = ₹ 12,000

Amount paid = ₹ 62,000

Amount unpaid = ₹ 12000 - ₹ 6000 = ₹ 6000

So, number of instalments  $= \; \;$

 

$=$ ₹  $\frac{6000}{500}$

= 12 = n

Now, intrest on 1st installment = interest on unpaid amount ( i.e. ₹ 6000) for 1 Year.

= ₹  $\frac{6000\times 12\times 1}{100}$ = ₹720

Similarly,

Interest on 2nd installment = interest on (₹ 6000 - ₹ 500) for the next 1 year

= ₹  $\frac{5500\times 12\times 1}{100}$ = ₹660

And,

Interest on 2nd installment = ₹  $\frac{(5500-500)\times 12\times 1}{100}$

= ₹ 600

Here, Total (interest per) installment paid = ₹ (720 + 660 + 600 + ……. upto 12 terms)

$=\frac{12}{2}[2(720)+(12-1)(-60)]$ {  $\because$ 720 + 660 + 600 …….is an A.P. of a = 720 , d = 660 - 720  = - 60}

= 6 [ 1440 - 660]

= 6 × 780

= ₹ 4680

So, the total cost of tractor = cost price + installments

= ₹ 12,000 + ₹ 4680

= ₹ 16680

 

Q28. Shamshad Ali buys a scooter for Rs 22000. He pays Rs 4000 cash and agrees to pay the balance in annual instalment of Rs 1000 plus 10% interest on the unpaid amount. How much will the scooter cost him?

A.28. Given, cost of scooter = ₹22,000

Amount paid = ₹ 4000

Amount unpaid = ₹ 22,000 - ₹ 4000 = ₹ 18,000

Now,

Number of installments =Amount unpaid/Amount of each instalment $<br>\frac{}{}=\frac{15000}{1000}=18$

As he per 10% interest on up unpaid amount and ₹1000 each installment

Amount of 1st instalment = ₹ 1000 + ₹  $\frac{18000\times 10\times 1}{100}$ = ₹1000 + ₹1800

= ₹ 2800

Amount of 2nd installment = ₹ 1000 + ₹  $\frac{(18000-1000)\times 10\times 1}{100}$

= ₹ 1000 + ₹ 1700 = ₹ 2700

Similarly,

Amount of 3rd installment = ₹ 1000 + ₹  $\frac{\left(17000-1000\right)\times 10\times 1}{100}$

= ₹ 1000 + ₹ 1600 = ₹ 2600

So, Total installment paid = ₹ 2800 + ₹ 1600 = ₹ 2600 + …… upto 18 installment

$=\frac{18}{2}[2\times 2800+(18-1)(-100)]$

= 9 [5600 - 1700]

= 9 × 3900

= ₹ 35,000

Total cost of scooter = Amount + Total installment paid

= ₹ 4000 + ₹ 35,100

= ₹ 39100

 

Q29.  A person writes a letter to four of his friends. He asks each one of them to copy the letter and mail to four different persons with instruction that they move the chain similarly. Assuming that the chain is not broken and that it costs 50 paise to mail one letter. Find the amount spent on the postage when 8th set of letter is mailed.

A.29. As the number of letters mailed forms a G.P. i.e. , 4, 4², ……., 4⁸

We have,

a = 4

$r=\frac{4^2}{4}=4$

and n = 8

So, Total numbers of letters = 4 + 4³ + ……. + 4⁸

$=\frac{4\left(4^8-1\right)}{4-1}$

$=\frac{4\times (65536-1)}{3}$

$=\frac{4}{3}\times 65535$

$=\frac{4}{8}\times 21,845$

= 87380

As amount spent on one postage = 50 paise  $=$ ₹  $\frac{50}{100}$

So, for reqd. postage = ₹  $\frac{50}{100}\times 87380$

= ₹ 43690

 

Q30. A man deposited Rs 10000 in a bank at the rate of 5% simple interest annually. Find the amount in 15th year since he deposited the amount and also calculate the total amount after 20 years.

A.30. Given,

Principal amount = ₹ 10000

Amount at end of 1 year = ₹  $\left(10000+\frac{10000+5\times 1}{100}\right)$

= ₹ (10000 + 500)

= ₹ 10500 {Amount paid = principal + S.I. in a year}

$S.I=\frac{Principal\times rate\times time}{100}$

Amount at end of 2nd year

= ₹  $\left(10000+\frac{10000\times 5\times 2}{100}\right)\left\{\frac{Principal\times rate\times time}{100}\right\}$

= ₹ (10000 + 1000)10500 + 500

= ₹ 11000

Amount at end of 3rd year

= ₹  $\left(10000+\frac{10000\times 5\times 3}{100}\right)$

= ₹ (1000 + 1500)

= ₹ 11500

So, amount at end of 1^st, 2^nd , 3^rd ………, n^th year forms as A.P.

i.e. ₹ 10500, ₹ 11000, ₹ 115000, ………. with

a = 10500

d = 11000 - 10500 = 500

Now, Amount in 15th year = Amount at end of 14th year

= ₹ 10500 + ( 14 - 1) × 500

= ₹ 10500 + 13 × 500

= ₹ 10500 + 6500

= ₹ 17000

Similarly amount after 20th year, = ₹ 10500+ (20 - 1) × 500

= ₹ 10500 + 19 × 500

= ₹ 10500 + 9500

= ₹ 20,000

 

Q31. A manufacturer reckons that the value of a machine, which costs him Rs. 15625, will depreciate each year by 20%. Find the estimated value at the end of 5 years.

A.31. Given,

Cost of machine = ₹ 15625

depreciation rate = 20 % each year.

We have,

Depreciated value after 1st year = ₹ 15625 - 20 % of 15625

= ₹  $15625-\frac{20}{100}\times$ ₹15625

= ₹  $15625\left(1-\frac{20}{100}\right)$

= ₹  $15625\times \left(1-\frac{1}{5}\right)$

= ₹  $15625\times \frac{4}{5}$

Similarly,

Depreciated value after 2nd year = ₹ 15625  $\times {\left(\frac{4}{5}\right)}^2$ and so on.

This, Depreciated value at end of 5 years

= ₹ 15625  $\times {\left(\frac{4}{5}\right)}^5$

= ₹ 15625  $\times \frac{1024}{3125}$

= ₹ 5120

 

Q.32. 150 workers were engaged to finish a job in a certain number of days. 4 workers dropped out on second day, 4 more workers dropped out on third day and so on. It took 8 more days to finish the work. Find the number of days in which the work was completed.

A.32. Let ‘x’ be the no of days in which 150 workers took to finish the job.

If 150 workers worked for x days then number of workers for x days =150 x.

But given that number of works dropped 4 on 2^nd day, then 4 on 3^rd day and so on taking 8 more

days to finish the work. i.e., x + 8 days we can express as.

150 x = 150 + (150  $-$ 4) + (150  $-$ 4  $-$ 4)+……+ (x + 8) days.

150 x = 150 + 146 + 142 +……… (x+8) days which

R.H.S. from as A.P. of

a = 150

d = -4 and n = x +8

So, S_n = 150 x

$\Rightarrow \frac{n}{2}[2\times 150+(n-1)(-4)]=150x$

n [ 150 + (n - 1) (-2)] = 150 (n - 8) [ $\because$ n = x +8 x  $-$ 8  $-$ x]

150n 2n (n - 1) 150n 1200

2n² + 2n 1200 =0

n² - n - 600 = 0 [ dividing by -2 throughout]

n² - 25 n + 24 n 600 = 0

n (n - 25) + 24 (n - 25) = 0

(n - 25) (n + 24) = 0

n = 25 or n = -24 (which is not possible)

So, n = 25 days

i.e. x + 8 = 25 days