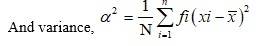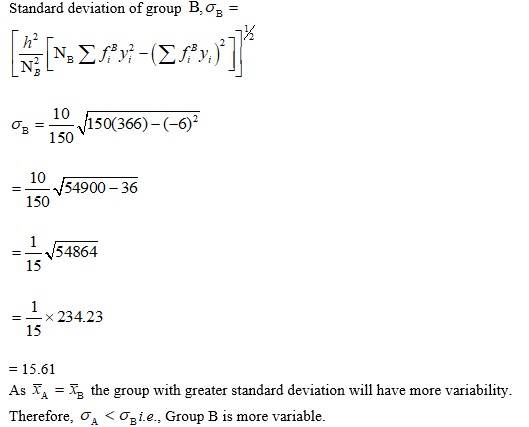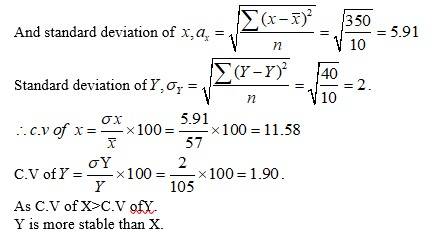Statistics Class 11 NCERT solutions: Students can check the NCERT Solutions Maths Class 11 Chapter 13 Statistics on this page. The NCERT Statistics Class 11 Maths Solutions Chapter 13 are given in a detailed manner. The Statistics Class 11 solutions are beneficial for those who are preparing for the Class 11 board exams. We provide accurate solutions of Class 11 NCERT Maths Chapter 13 Statistics prepared by experts. Students are advised to solve and practice these solutions for better results.
Statistics is an easy chapter in Class 11 Maths. Students can easily score full marks from this chapter, if they learn the formulas in this chapter. We have provided the solutions of five exercises and miscellaneous exercises on this page. Range, Quartile deviation, mean deviation, variance, standard deviation are the measures of dispersion. Students can also download NCERT Solutions Maths Class 11 Chapter 13 Statistics PDF from this page. Students can access our NCERT Solutions for free.
Topics Covered - Statistics
- Measures of Dispersion
- Range
- Mean Deviation
- Variance and Standard Deviation
- Analysis of Frequency Distributions
Check the NCERT Solutions Maths Class 11 Chapter 13 Statistics below.
Q 15.1 Find the mean deviation about the mean for the data in 4, 7, 8, 9, 10, 12, 13, 17
A 15.1 Mean of the given observation is.
x¯ = (4+7+8+9+10+12+13+17) / 8
= 80 / 8
= 10
Deviation of the respective observation about the mean x¯ i.e., xi - x¯ are 4–10,7–10,8–10,9–10,10–10,12–10,13–10,17–10
=-6,-3,-2,-1,0,2,3,7
The absolute value of the deviation i.e.,| xi - x¯ | are 6,3,2,1,0,2,3,7.
Therefore, the required mean deviation about the mean is
M.D. = (x¯) = 1/n ∑ | xi - x¯ | = (6+3+2+1+0+2+3+7) / 8
=24 / 8
= 3.
Q 15.2 Find the mean deviation about the mean for the data in 38, 70, 48, 40, 42, 55, 63, 46, 54, 44
A 15.2 Mean of the given observation is:
x¯ = (38+70+48+40+42+55+63+46+54+44) / 10
= 500 / 10
=50
So,
| xi |
38 |
10 |
48 |
40 |
42 |
55 |
63 |
46 |
54 |
44 |
| | xi - 50 | | 12 |
20 |
2 |
10 |
8 |
5 |
13 |
4 |
4 |
6 |
Therefore, the required mean deviation about the mean is
M.D. = (x¯) = 1/n ∑ | xi - x¯ |
= (12+20+2+10+8+5+13+4+4+6) / 10
=84/10
= 8.4
Q 15.3 Find the mean deviation about the median for the data in Exercises 3 and 4.
13, 17, 16, 14, 11, 13, 10, 16, 11, 18, 12, 17
A 15.3 Arranging the data in ascending order we get,
10,11,1112,13,13,14,16,16,17,17,18
As n=12, even
So, median is the mean of (M/2)th and (M/2+1)th observation.
Median = (6th Observation + 7th Observation) / 2
M = (13+14) / 2 = 27/2 = 13.5
So, deviation of respective observation about the median M, |x¯ -M| are
| xi |
10 |
11 |
11 |
12 |
13 |
13 |
14 |
16 |
16 |
17 |
17 |
18 |
| | x¯ -M| | 3.5 |
2.5 |
2.5 |
1.5 |
0.5 |
0.5 |
0.5 |
2.5 |
2.5 |
3.5 |
3.5 |
4.5 |
Therefore the mean deviation about the mean is
M.D. = (M) = 1/n X ∑ |x¯ -M|
= 1/12 X (3.5+2.5+2.5+1.5+0.5+0.5+0.5+2.5+2.5+3.5+3.5+4.5
=28/12
= 2.33
Download HereNCERT Class 11th Maths Chapter 13 Statistics Solutions PDF
- Statistics Class 11 NCERT Solutions and FAQs
Statistics Class 11 NCERT Solutions and FAQs
Ex.15.1
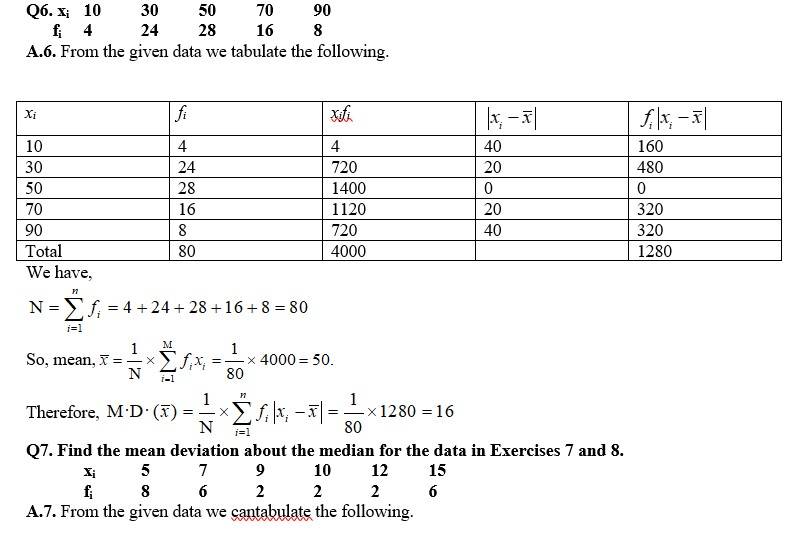
Q1. Find the mean deviation about the mean for the data in Exercises 1 and 2.
4, 7, 8, 9, 10, 12, 13, 17
A.1. Mean of the given observation is.
Deviation of the respective observation about the mean i.e., are 4–10,7–10,8–10,9–10,10–10,12–10,13–10,17–10
=6,-3,-2,-1,0,2,3,7
The absolute value of the deviation i.e., are 6,3,2,1,0,2,3,7.
Therefore, the required mean deviation about the mean is
= 3.
Q2. 38, 70, 48, 40, 42, 55, 63, 46, 54, 44
A.2. Mean of the given observation is.
So,
| xi |
38 |
10 |
48 |
40 |
42 |
55 |
63 |
46 |
54 |
44 |
| 12 |
20 |
2 |
10 |
8 |
5 |
13 |
4 |
4 |
6 |
Therefore, the required mean deviation about the mean is
= 8.4
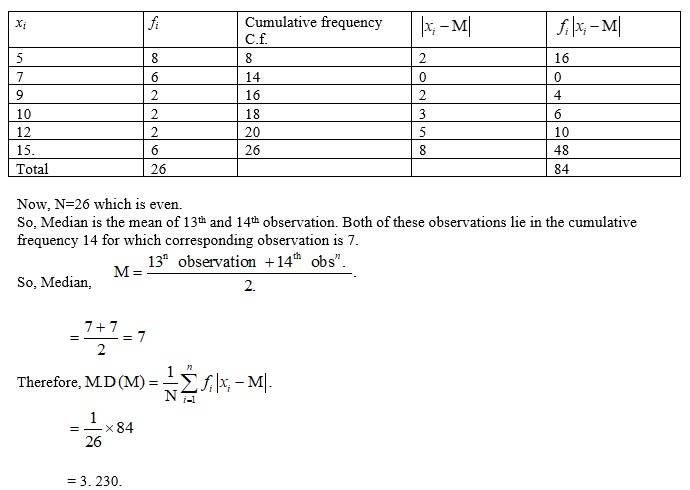
Q3. Find the mean deviation about the median for the data in Exercises 3 and 4.
13, 17, 16, 14, 11, 13, 10, 16, 11, 18, 12, 17
A.3. Arranging the data in ascending order we get,
10,11,1112,13,13,14,16,16,17,17,18
As n=12, even
So, median is the mean of and observation.
So, deviation of respective observation about the median. are
| xi |
10 |
11 |
11 |
12 |
13 |
13 |
14 |
16 |
16 |
17 |
17 |
18 |
| 3.5 |
2.5 |
2.5 |
1.5 |
0.5 |
0.5 |
0.5 |
2.5 |
2.5 |
3.5 |
3.5 |
4.5 |
Therefore the mean deviation about the mean is
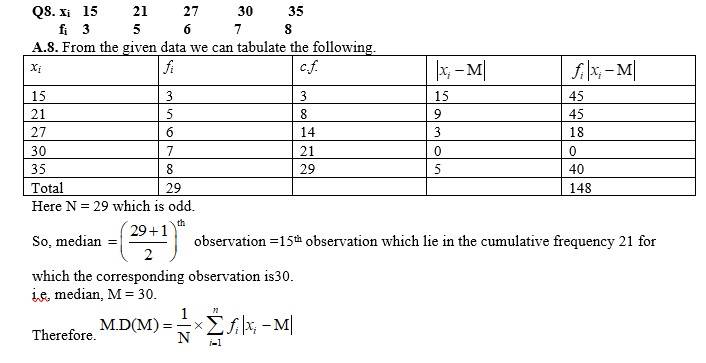
Q4. 36, 72, 46, 42, 60, 45, 53, 46, 51, 49
A.4. Arranging the given data in ascending order we get,
36,42,45,46,46,49,51,53,60,72
As n = 10 (even)
= 47.5
| 36 |
42 |
45 |
46 |
46 |
49 |
51 |
53 |
60 |
72 |
|
| 11.5 |
5.5 |
2.5 |
1.5 |
1.5 |
1.5 |
3.5 |
5.5 |
12.5 |
24.5 |
M.D. (M)
Q5. Find the mean deviation about the mean for the data in Exercises 5 and 6.
= 5. 10
Ex.15.2
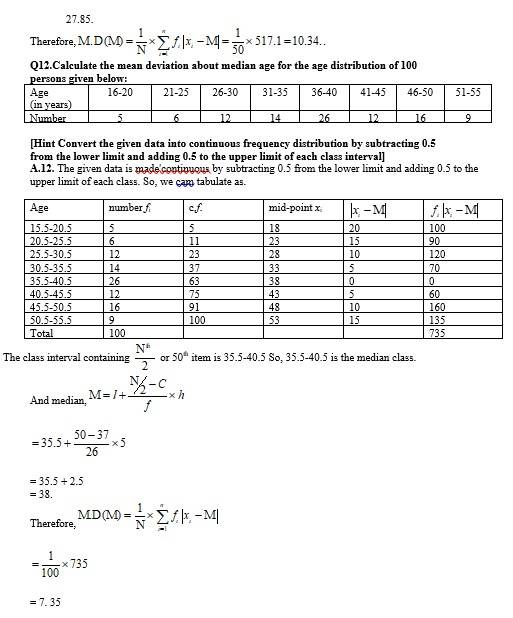
Q1. Find the mean and variance for each of the data in Exercies 1 to 5.
6, 7, 10, 12, 13, 4, 8, 12
A.1. The given data can be tabulated as.
we have,
mean,
So, variance,
Q2. First n natural numbers
A.2. We know that,
Sum of first'n ' natural no
So, mean,
So, Variance,
So,
And
Putting (2), (3) and (4) in (1) we get,
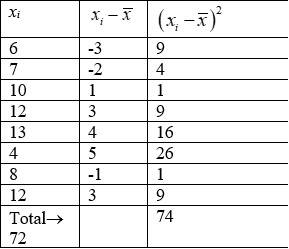
Q3. First 10 multiples of 3A.3. We have, first 10 multiples of 3=3,6,9,12,15,18,21,24,27,30.
So,
We can now tabulate the given data as following.
| Total |
|
742.25 |
Therefore, variance,
= 74.25.
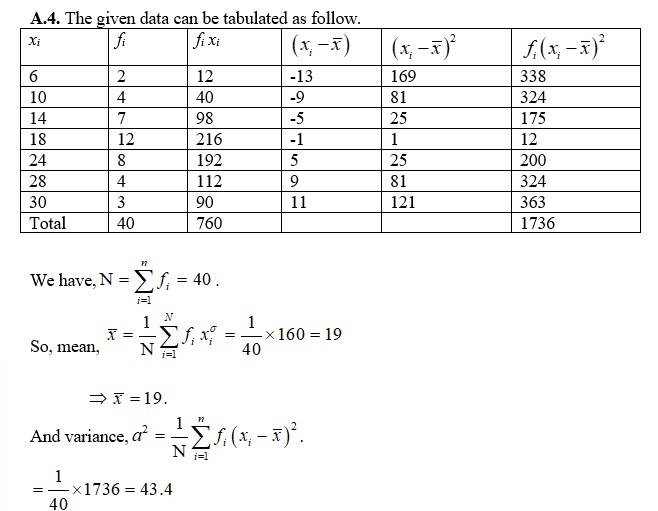
Q4.
| xi |
6 |
10 |
14 |
18 |
24 |
28 |
30 |
| fi |
2 |
4 |
7 |
12 |
8 |
4 |
3 |
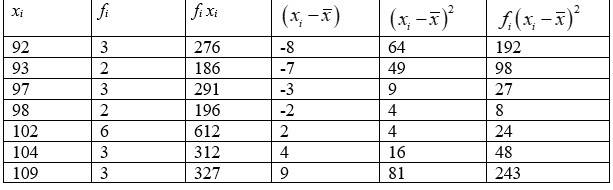
Q5.
| xi |
92 |
93 |
97 |
98 |
102 |
104 |
109 |
| fi |
3 |
2 |
3 |
2 |
6 |
3 |
3 |
A.5. The given data can be tabulated as follow
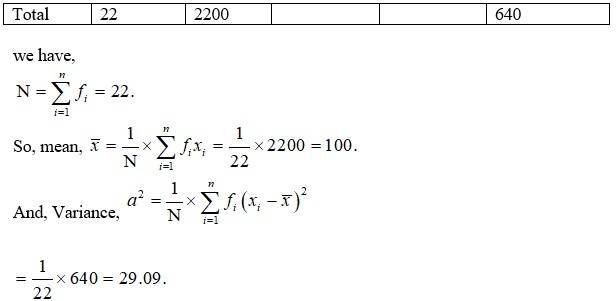
Q6.Find the mean and standard deviation using short-cut method.
| xi |
60 |
61 |
62 |
63 |
64 |
65 |
66 |
67 |
68 |
| fi |
2 |
1 |
12 |
29 |
25 |
12 |
10 |
4 |
5 |
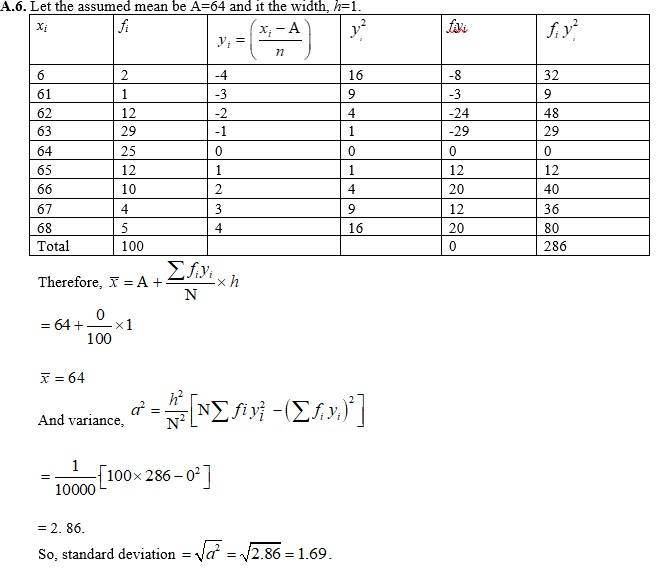
Q7. Find the mean and variance for the following frequency distributions in Exercises7 and 8.
| Classes |
0-30 |
30-60 |
60-90 |
90-120 |
120-150 |
150-180 |
180-210 |
| Frequencies |
2 |
3 |
5 |
10 |
3 |
5 |
2 |
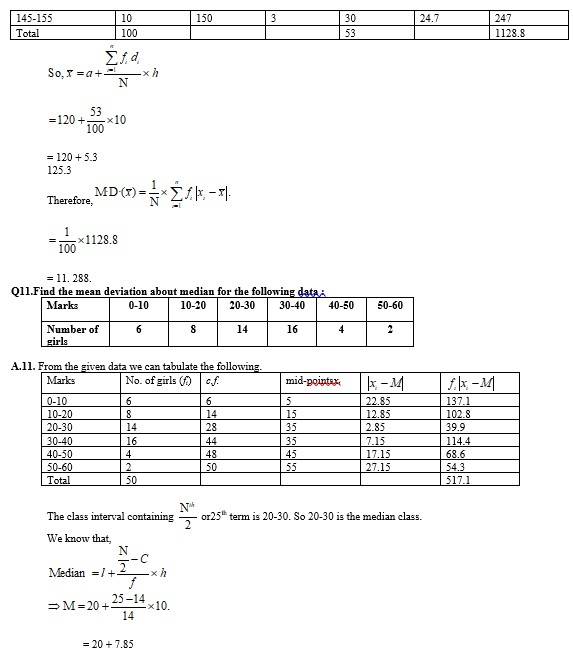
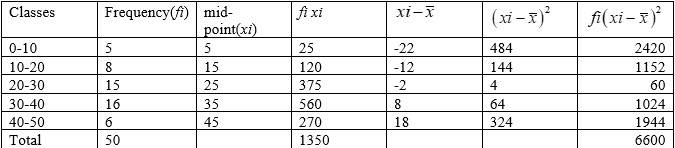
Q8.
| Classes |
0-10 |
10-20 |
20-30 |
30-40 |
40-50 |
| Frequencies |
5 |
8 |
15 |
16 |
6 |
A.8.
We have, .
So, mean,
= 132.
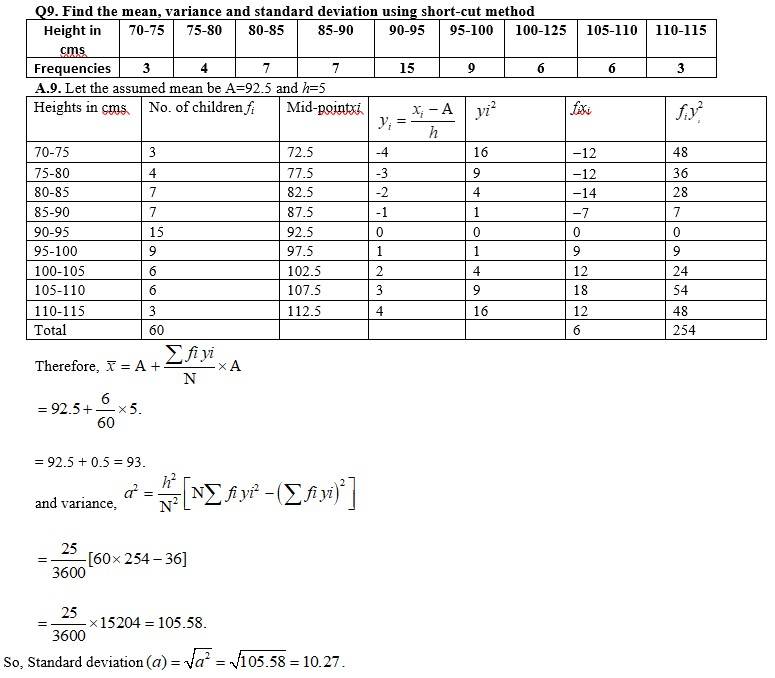
Q10. The diameters of circles (in mm) drawn in a design are given below:
| Diameters |
33-36 |
37-40 |
41-44 |
45-48 |
49-52 |
| No. of circles |
15 |
17 |
21 |
22 |
25 |
Calculate the standard deviation and mean diameter of the circles.
[ Hint First make the data continuous by making the classes as 32.5-36.5, 36.5-40.5,
40.5-44.5, 44.5 - 48.5, 48.5 - 52.5 and then proceed.]
A.10. The given data is converted into continuous frequency duration by subtracting and adding 0.5 from lower and upper limit respectively. Lit the assumed mean be A=42.5 and h=4
Ex. 15.3
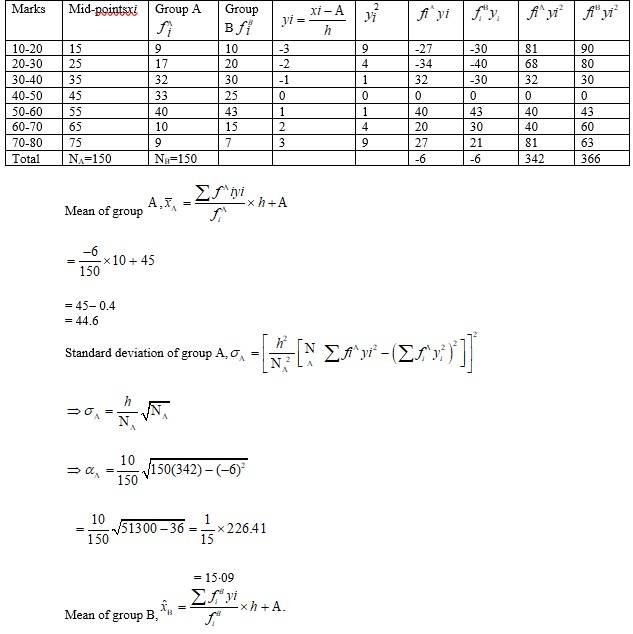
Q1. From the data given below state which group is more variable, A or B?
| Marks |
10-20 |
20-30 |
30-40 |
40-50 |
50-60 |
60-70 |
70-80 |
| Group A |
9 |
17 |
32 |
33 |
40 |
10 |
9 |
| Group B |
10 |
20 |
30 |
25 |
43 |
15 |
7 |
A.1. Let the assumed mean be A=45 and h=10. Then we can tabulate the given data as following.
= 44.6
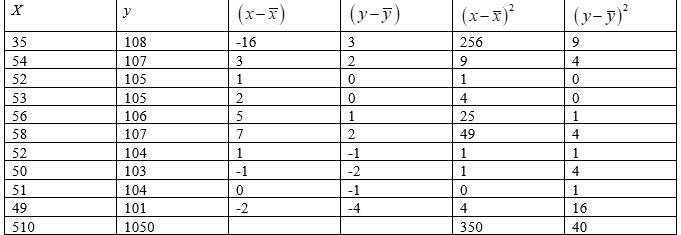
Q2. From the prices of shares X and Y below, find out which is more stable in value:
| X |
35 |
54 |
52 |
53 |
56 |
58 |
52 |
50 |
51 |
49 |
| Y |
108 |
107 |
105 |
105 |
106 |
107 |
104 |
103 |
104 |
101 |
A.2. We can tabulate the given data as follows.
Total =
Mean of
Mean of .
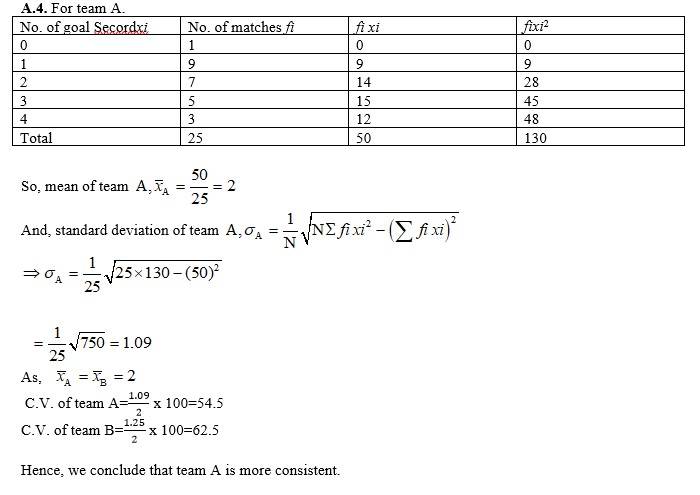
Q4. The following is the record of goals scored by team A in a football session:
| No. of goals scored |
0 |
1 |
2 |
3 |
4 |
| No. of matches |
1 |
9 |
7 |
5 |
3 |
For the team B, mean number of goals scored per match was 2 with a standard
deviation 1.25 goals. Find which team may be considered more consistent?
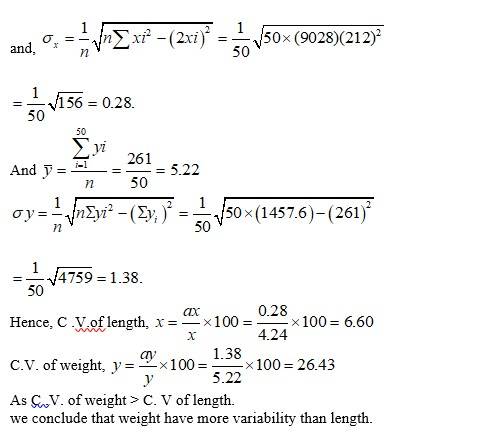
Q5. The sum and sum of squares corresponding to length x (in cm) and weight y
(ingm) of 50 plant products are given below:
Which is more varying, the length or weight?
A.5. Give, n=50.
So,
Miscellaneous Example
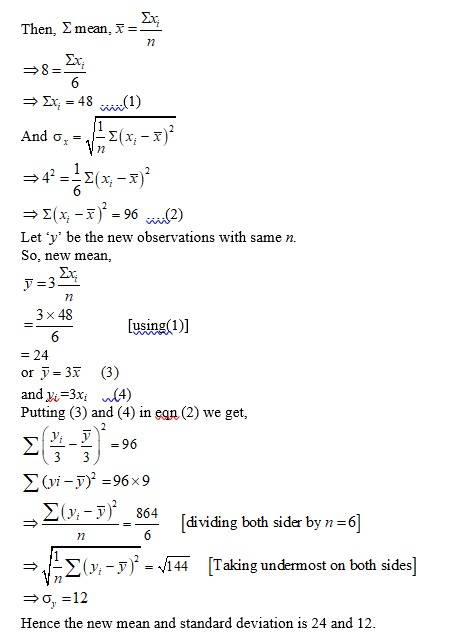
Q1. The mean and variance of eight observations are 9 and 9.25, respectively. If six of the observations are 6, 7, 10, 12, 12 and 13, find the remaining two observations.
A.1. Let the other two observation be x and y.
Therefore, the series is 6, 7, 10, 12, 12, 13, x, y.
So, Mean
….. (1)
And, variance = 9.25
9 + 4 + 1 + 9 + 9 + 16 + x2 + 81 - 18x + y2 + 81 - 18y = 74
[ equation (1)]
……(2)
Squaring Equation (1) we get,
(3)
Subtracting (3) from (2) we get,
……(4)
From (1) and (4)
Case I, x + y =12 and x - y = 4
x = 8 and y = 4
Case II,x + y =12 and x – y = -4
x = 4 and y = 8.
Hence, the remaining observations are 8 and 4.
Q2. The mean and variance of 7 observations are 8 and 16, respectively. If five of the observations are 2, 4, 10, 12, 14. Find the remaining two observations.
A.2. Let the other two observation be x and y. Then, the series is 2, 4, 10, 12, 14, x, y.
So, mean,
….(1)
And variance =
….(2)
Squaring eqn (1) we get,
…..(3)
Subtracting eqn (3) from (2) we get,
….(4)
Using eqn (1) and (2) we get,
Case I. x + y = 14 and x – y = 2
x = 8 and y = 6
Case II. x + y = 14 and x – y = -2
x = 6 and y = 8
Hence, the remaining observations are 6 and 8.
Q3. The mean and standard deviation of six observations are 8 and 4, respectively. If each observation is multiplied by 3, find the new mean and new standard deviation of the resulting observations.
A.3. Let ‘x’ be the given observations with n = 6.
Q4. Given that is the mean and σ2 is the variance of n observations x1, x2, ...,xn. Prove that the mean and variance of the observations ax1, ax2, ax3, ...., axn are a and a2 σ2, respectively, (a ≠ 0).
A.4. For n observations x1, x2,……., xn .
We have mean =
and variance =
Let yi be the new observations with same n.
So, yi = axi (3)
Now mean,
So
And, putting (3) and (4) in (2) we get,
Hence, the mean and variance of ax1, ax2, ……, axn are and a2 σ2 .
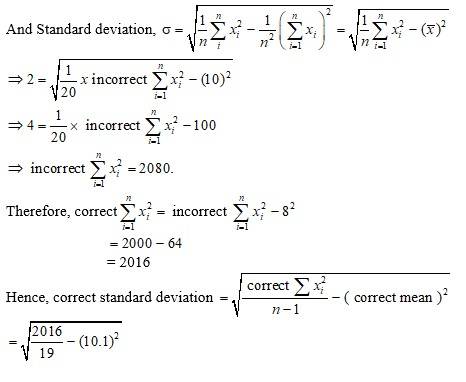
Q5. The mean and standard deviation of 20 observations are found to be 10 and 2, respectively. On rechecking, it was found that an observation 8 was incorrect. Calculate the correct mean and standard deviation in each of the following cases:
(i) If wrong item is omitted. (ii) If it is replaced by 12.
A.5. (i) Given, n = 20.
Incorrect mean
Incorrect standard deviation
We know that,
So, incorrect sum of observation = 200.
correct sum of observation =200 – 8 = 192
And correct mean
Q6. The mean and standard deviation of marks obtained by 50 students of a class in three subjects, Mathematics, Physics and Chemistry are given below:
Subject Mathematics Physics Chemistry
Mean 42 32 40.9
Standard 12 15 20
deviation
Which of the three subjects shows the highest variability in marks and which
shows the lowest?
A.6. C.V in mathematics =
C.V in Physics =
C.V in chemistry =
Chemistry has highest variability and mathematics has lowest variability.
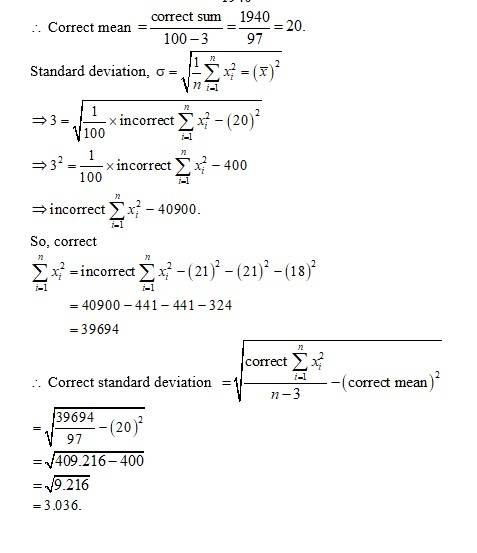
Q7. The mean and standard deviation of a group of 100 observations were found to be 20 and 3, respectively. Later on it was found that three observations were incorrect, which were recorded as 21, 21 and 18. Find the mean and standard deviation if the incorrect observations are omitted.
A.7. Given, n = 100.
incorrect mean ( ) = 20.
incorrect standard deviation (σ) = 3
We know that,
So, incorrect sum of observation = 2000
Correct sum of observation
= 1940
Explore exams which ask questions on Maths Ncert Solutions class 11th
Select your preferred stream
Maths Ncert Solutions class 11th Exam
Student Forum
Popular Courses After 12th
Exams accepted
CA FoundationExams accepted
ICSI ExamExams accepted
BHU UET | GLAET | GD Goenka TestBachelor of Business Administration & Bachelor of Law
Exams accepted
CLAT | LSAT India | AIBEExams accepted
IPMAT | NMIMS - NPAT | SET
Exams accepted
BHU UET | KUK Entrance Exam | JMI Entrance ExamBachelor of Design in Animation (BDes)
Exams accepted
UCEED | NIFT Entrance Exam | NID Entrance ExamBA LLB (Bachelor of Arts + Bachelor of Laws)
Exams accepted
CLAT | AILET | LSAT IndiaBachelor of Journalism & Mass Communication (BJMC)
Exams accepted
LUACMAT | SRMHCAT | GD Goenka Test