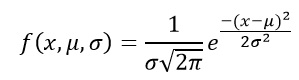Normal Distribution: Overview, Questions, Preparation

In Mathematics, Under Probability theory; a normal distribution or a gaussian distribution is a continuous probability distribution for a real random variable. The property of normal distribution lies under the probability density function. To understand this better, consider a probability random function say, f(x). Here x can be any random variable for that probability function, denoted by f. Now, determine the range between which the probability function will be represented. Let the range be x to x+kx.

f(x) ≥ 0 ∀ x ϵ (−∞,+∞)

And -∞∫+∞ f(x) = 1

The formula for Normal distribution is as follows: 

In the formula,
x is the variable
µ is the mean
σ is the standard deviation

Normal Distribution Curve

When understanding the normal distribution set, the random variables follow a particular pattern used to study and evaluate the values over a range of a sequence.
For example, When a doctor wants to estimate a particular patient’s height, he can evaluate it using a scale, with a specific range - between 0 and 6 feet.

But when talking about normal distribution, we don’t even bother about the range. It can extend from positive infinity to negative infinity, and we still obtain a smooth curve. The random set of variables are called continuous variables.

This topic is a part of the Probability of class XII. Normal Distribution normally does not come for the final examination, but the whole chapter has a weightage of 6-8 marks. The normal distribution is often asked as Multiple Choice Questions or 2- marks questions. 

Question 1. X is a normally distributed variable with a mean of 30 and a standard deviation of 4. Find the P (x

Solution:  To find- the area under the normal distribution curve.  For x=40, the z value can be found out. Z= 2.5

Hence P(x

Question 2.A device is used to measure the speed of cars on a highway. The speed is normally distributed with a 90km/hr mean and a standard deviation of 10. What is the probability that the car picked was travelling at more than 100km/hr?

Solution: Let x be the random variable that represents the speed of cars. x has μ = 90 and σ = 10. We have to find the probability that x is higher than 100 or P(x > 100)

For x = 100 , z = (100 - 90) / 10 = 1

P(x > 90) = P(z > 1) = [total area] - [area to the left of z = 1]

= 1 - 0.8413 = 0.1587

Question 3. A machine produces an instrument with a particular life length. It has a normal distribution with a mean of 12 months. The standard deviation is for two months. What is the probability that instrument A made by this machine will last more than seven months?

Solution: P(7

= 0.493

Q: What is Normal Distribution?

Q: What are the other names of Normal Distribution?

Q: What is the normal distribution curve like?

Q: What are the applications of Normal Distribution?