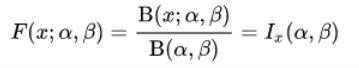Rachit Kumar SaxenaManager-Editorial
What is Beta distribution?
The beta distribution is a continuous probability distribution, defined by two positive parameters in probability and statistics. It’s a type of distribution of probability used to describe results or random percentage behaviour. Moreover, a beta distribution in statistics expresses all the possible values of a probability.
The beta distributions are a family having two positive parameters of shape, α, and β, on the interval [0,1]. The beta distribution of these two parameters is the random variable’s exponents, and the distribution shape is regulated. The basic distribution is generally referred to as the first kind of beta distribution, and the second kind is needed for the main beta distribution.
Equations of Beta Distribution
The beta distribution serves to track the behaviour of random variables that in several disciplines are confined to intervals of finite time. This distribution is essentially characterised by probability density, cumulative density function, moment generating function, variance, and expectations. All the formulas are provided below:
A. Probability density function
In beta distribution, the probability density function is a function of the x variable and its projection (1 - x).
B. Cumulative distribution function
The formula for cumulative distribution function in beta distribution is given as:
Characteristics of Beta Distribution
1. The model of a beta distribution is expressed as α - 1/ α + β - 2, where α and β are greater than 1 and correspond to the value of probability density function.
2. The median of a beta distribution is expressed as α - ⅓ / α + β - ⅔, where α and β are greater than or equal to 1 and correspond to the value of probability density function.
3. The mean of a beta distribution is expressed as 1/ 1 + β/α, where the mean is the function of two parameters α and β and their ratio β/α.
Applications of Beta Distribution
The beta distribution is most widely used to model the ambiguity surrounding the probability of a random experiment’s progress.
A three-point ‘beta distribution’ methodology is used in project management, acknowledging ambiguity in the project time determination.
It offers powerful quantitative instruments and simple statistics to measure engagement levels over the planned term.
Weightage of Beta Distribution
The topic ‘Beta Distribution’ is from chapter 13, Probability. Apart from this topic, the chapter contains Bayes’ theorem, multiplication theorem on probability independent events, and total probability. Moreover, the chapter accounts for pivotal 8 marks in the 12th standard final examinations.
Illustrative Example on Beta Distribution
1. A coin is tossed thrice. Find the probability distribution of the number of heads in it.
Solution.
The given condition states that a coin is tossed thrice.
Therefore, S = {HHH, HHT, HTH, HTT, THH, THT, TTH, TTT}
Y expresses the number of heads.
Therefore, Y is a function ranging {0, 1, 2, 3}.
P (Y = 0) = P (HHH) = ⅛
P (Y = 1) = P (HHT) + P (HTH) + P (HHT) = ⅛ + ⅛ + ⅛ = ⅜
P (Y = 2) = P (HTT) + P (THT) + P (HTH) = ⅜
P (Y = 3) = P (TTT) = ⅛
Hence, the required probability distribution is:
| Y |
0 |
1 |
2 |
3 |
| P(Y) |
⅛ |
⅜ |
⅜ |
⅛ |
2. Determine whether the following probability distribution is of a random variable or not.
| Y |
0 |
1 |
2 |
| P (Y) |
0.6 |
0.1 |
0.3 |
Solution.
We know that the sum of the probability distribution of a random variable is always 1.
Therefore, we need to check whether these random variables add up to 1 or not.
Sum = 0.6 + 0.1 + 0.3 = 1
Therefore, the following probability distribution table is for random variables.
3. Determine whether the following probability distribution is of a random variable or not.
| Y |
0 |
1 |
2 |
3 |
| P (Y) |
0.3 |
0.4 |
0.5 |
0.1 |
We know that the sum of the probability distribution of a random variable is always 1.
Therefore, we need to check whether these random variables add up to 1 or not.
Sum = 0.3 + 0.4 + 0.5 + 0.1 = 1.3 ≠ 1
Therefore, the following probability distribution table not is for random variables.
FAQs on Beta Distribution
Q: What is the formula of variance in beta distribution?
Q: How beta distribution is used for Bayesian analysis?
A; It is used as a prior distribution for binomial proportions.
Q: Who devised the calculator for beta distribution?
A: The name of the person is Dr Borgar.
Q: How can we simplify the beta function?
A: We can simplify the beta function using the gamma function.
Q: What are the roles of α and β in beta distribution?
A: They help to predict the changes in beta distribution curves.
News & Updates
Probability Exam
Student Forum
Popular Courses After 12th
Exams: BHU UET | KUK Entrance Exam | JMI Entrance Exam
Bachelor of Design in Animation (BDes)
Exams: UCEED | NIFT Entrance Exam | NID Entrance Exam
BA LLB (Bachelor of Arts + Bachelor of Laws)
Exams: CLAT | AILET | LSAT India
Bachelor of Journalism & Mass Communication (BJMC)
Exams: LUACMAT | SRMHCAT | GD Goenka Test