Box and Whisker Plot: Overview, Questions, Preparation

Box and whisker plots are suitable for contrasting distributions since the core, spread, and total array are immediately identifiable. A box and whisker plot summarises a set of data calculated on an interval scale. This technique is also used for the analysis of explanatory data. 

Definition of Box & Whisker Plot

It is a graph exhibiting data from the five-numbers set, including central tendency metrics. The distribution is not as precise as the results of histogram or stem and leaf plot. 

Steps to Build a Box and Whisker Plot

1. Arrange the given set of data in ascending order, i.e., smallest to largest.
2. Calculate the median of the set of data.
3. Find the quartiles of the data set, i.e., the median of the upper and lower half.
4. Calculate the extremes of the data, i.e., the greatest and least values.
5. Now, Plot the values of median, extremes, and quartiles below a number line. Moreover, you can use a ruler to mark the points with even space.
6. Draw the whiskers and the plots.  

What is the use of the Box and Whisker Plot?

1. Can easily handle a large dataset.
2. A practical method for presenting a clear summary of the data.
3. The best technique for displaying outliers.
4. Helps to show the central value, distribution shape, and variability.

This topic is known as an essential concept of statistics of Grade 12. After studying the concept and examples, students will learn how to evaluate elaborated details of various data set distributions. 

1. Evaluate the first & third quartiles of the set of data {3, 7, 8, 5, 12, 14, 21, 13, 18).

Solution:
Arrange the data in ascending order: 3, 5, 7, 8, 12, 13, 14, 18, 21
The numbers of terms are 9 i.e., odd in number.
 Therefore, Median = 12
 The Q₁ = median of lower half
     = 5+7/2 = 12/2 = 6
The Q₃ = median of upper half 
              = 14+18/2 = 32/2 = 16
 So, Q₁ and Q₃ = 6 and 16

 2. Find the interquartile range and  range of {7, 3, 8, 12, 21, 5, 14, 18, 15, 13, 14}

Solution:
Arrange the data in ascending order: 3, 5, 7, 8, 12, 14, 14, 15, 18, and 21
Range = Maximum – Minimum = 21-3 = 18.
Q₁= 7 and Q₃ = = 15.
Therefore, Interquartile Range = Q₃ = – Q₁ = 15-7 = 8
So, the range =18 and the interquartile range = 8.

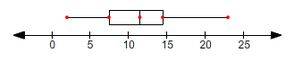
3. Find Q₁, Q₂, and Q₃ = for the given data and also draw a box-and-whisker plot. {2,6,7, 11, 8,8,12,13,14,15,22,23}
Solution:

Total number of observations = 12
The Middle terms are 11 and 12.
Therefore, Median Q₂ = 11+12/2 = 23/2 = 11.5
Q₁ =  Lower halves median i.e. (2, 6, 7, 8, 8, 11) = 7.5
Q₃ = Upper halves median i.e. (12, 13, 14, 15, 22, 23) = 14.5
Extremes = Minimum and Maximum values of the data set.
                   i.e., 2 and 23