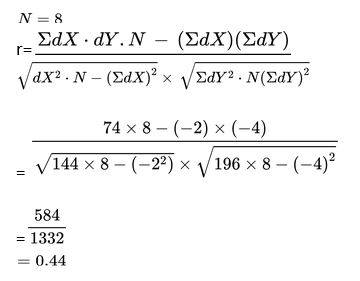Correlation and Regression: Overview, Questions, Preparation

1.7K Views

This chapter has multiple topics listed below:

Statistics Standard Deviation Correlation Bar Graph Chi Square Test Measures of Central Tendency Variance Extrapolation Null Hypothesis Pareto Chart Harmonic Mean How to find Median Statistics Definition Data Collection Methods Frequency Polygons Central Tendency Confidence Interval Data Handling Statistical Inference Quota Sampling Interquartile Range Quartiles Variance and Standard deviation Cluster Analysis Correlation and Regression Graphical Representation Least Square Method Level of Significance Paired T Test Z Score Table Assumed Mean Method Box and Whisker Plot Gamma Distribution Sample Size T Distribution Tests of Significance Types of Statistics Continuous Variable Sample Statistic Exponential Smoothing T Test Table Bivariate Analysis Relative Frequency Difference between Average and ...Difference between Mean and Med...Difference between Parametric a...Grouping data Statistics pdf Mean Absolute Deviation Population and Sample

Rachit Kumar SaxenaManager-Editorial

Updated on Aug 5, 2021 09:15 IST

Table of Contents

What is Correlation?
Weightage of Correlation and Regression
Illustrative examples on Correlation and Regression
FAQs on Correlation and Regression

What is Correlation?

Correlation, a statistical concept, is used to calculate the value of the relationship between variables. A real-life example of what this technique calculates is the relationship between price and demand.

Types of Correlation

Correlation can be classified into the following categories.

Positive and Negative Correlation
Linear and Non-Linear Correlation
Simple, Multiple and Partial Correlation

Techniques for measuring correlation

The various techniques used to measure correlation are :

Scatter diagram
Karl Pearson’s Coefficient of Correlation represented by the formula

Regression

Linear regression is a statistical tool used to represent a relationship between a dependent variable (usually defined by Y) and a set of other variables (independent variables). It holds immense value in the real world by aiding financial managers to make various investment decisions concerning aspects such as stocks, commodity prices, etc.

Weightage of Correlation and Regression

The concept of correlation and regression is introduced to the students of grade 11 as a part of statistics. The following concept generates high amounts of knowledge, especially in the field of data science. Regression is not evaluated while correlation is tested for 4-8 marks.

Illustrative examples on Correlation and Regression

1. Calculate the correlation coefficient between the heights of fathers in inches (X) and their sons (Y)

X	65	66	57	67	68	69	70	72
Y	67	56	65	68	72	72	69	71

Solution.

X	dx(d from AM=67)	dX²	Y	dY(d from AM=68)	dY²	dXdY
65	-2	4	67	-1	1	2
66	-1	1	56	-12	144	12
57	-10	100	65	-3	9	30
67	0	0	68	0	0	0
68	+1	1	72	4	16	4
69	+2	4	72	4	16	8
70	+3	9	69	1	1	3
72	5	25	71	3	9	15
ΣX = 534	ΣdX = -2	ΣdX² = 144	ΣY = 540	ΣdY = -4	ΣrdY² = 196	ΣdXdY=74

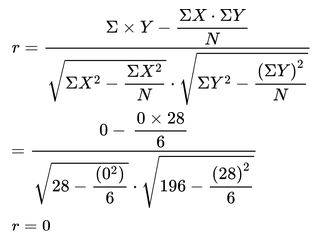
2. Calculate the correlation coefficient between X and Y and comment on their relationship.

X	-3	-2	-1	1	2	3
Y	9	4	1	1	4	9

Solution.

X	Y	X²	Y²	XY
-3	9	9	81	-27
-2	4	4	16	-8
-1	1	1	1	-1
1	1	1	1	1
2	4	4	16	8
3	9	9	81	27

There is no linear correlation between the two variables, as X and Y are uncorrected.

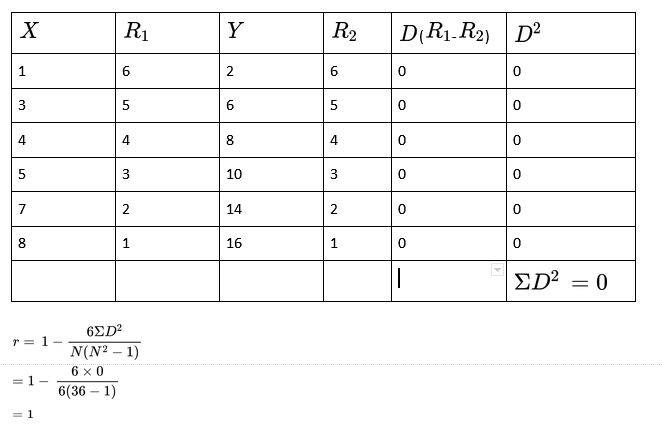
3. Calculate the correlation coefficient between X and Y and comment on their relationship

X	1	3	4	5	7	8
Y	2	6	8	10	14	16

Solution.

∴The two variables are perfectly positive correlated.

FAQs on Correlation and Regression

Q: When two variables move in the same direction, what is the nature of the correlation of two variables?

A: The value of the correlation would be positive in nature.

Q: What is the arithmetical representation of the Coefficient of correlation existing between -1 and +1?

A: The correlation is perfectly negative when the value of the coefficient of correlation is -1, and the correlation is perfectly positive when the value of the coefficient of correlation is +1.

Q: When is the method of rank correlation used for calculation?

A: When variables are qualitative such as wisdom, beauty, bravery and so on are used.

Q: Define Regression

A: Linear regression can be defined as a statistical model used to represent the relationship between a scalar variable and an independent/dependent variable.

Q: Define the line of best fit.

A: The line of the best fit is the line that passes through the scattered points such that it covers up or represents most of these points. Approximately half of the points that are scattered should be on either side of this line.