Measures of Central Tendency: Overview, Questions, Preparation

Measures of Central Tendency is used to describe a larger set of data by identifying a central value in that dataset, and is sometimes also called summary statistics. 

There are 3 common measures of central tendency - Mean, Median and Mode which are considered to be accurate but in certain situations, some of them become more suitable to use than others.

Arithmetic Mean 

Arithmetic mean is the average value of an entire data set and is amongst the most common measures of central tendency. Arithmetic mean can further be divided into the following - 

Simple arithmetic mean 

The arithmetic mean is derived after adding values in a data set together and dividing it by the total number of observations. In case of raw data, mean is calculated using below formula - 

Weighted arithmetic mean 

When some components in an equation contribute more weight to the overall equation, it is known as weighted arithmetic mean. To find the weighted mean in a given problem, we'll first multiply numbers within the data set with weights and then add the results. 

Median
Median is defined as a central value that divides a series of data into two halves even as the data has been arranged in descending or ascending order of succession.

Mode 

Mode is the measure that calculates the number of times a single value occurs in a distribution.

How to calculate mode for individual series? 

When calculating mode for an individual series, you need to count the frequency of times a number appears in a distribution. The number which occurs most becomes the mode of that distribution. 

How to calculate mode for a discrete series? 

When it comes to calculating mode for a discrete series, we are provided with the frequencies of items and to calculate the mode, we simply have to see the value of the item having the highest frequency. 

In the 10^th board examination paper, measures of central tendency has a total weightage of 15 marks. The chapter in the textbook explains the use of mean, median and mode in a detailed manner, and the students will be taught how to calculate the 3 measures for raw, arrayed and even grouped data.

Illustrative Examples on Measures of Central Tendency

1.  A class 12th B, has 25 students who took an English test. Amongst them, 10 students had an average score of 80, while other students had an average score of 60. What will be the average score of the whole class?

Solution. In the first step, we will multiply average score with number of students who had that score and then add them. 

Thus :-

80x10+ 60x15 = 800+900 = 1700 
Number of observations = 25

1700/25 = 68

Ans : The average score of class was 68. 

2: Find the median of following series- 

5, 7, 6, 1, 8, 10, 12, 4, and 3.

Solution. 
To find median, we need to follow the below mentioned steps - 

Step 1 : Arranging the numbers in ascending order 

=  1, 3, 4, 5, 6, 7, 8, 10, 12.                     
Step 2 : Find the central value; hence it becomes the median

Since 6 is central value in the series, the median = 6. 

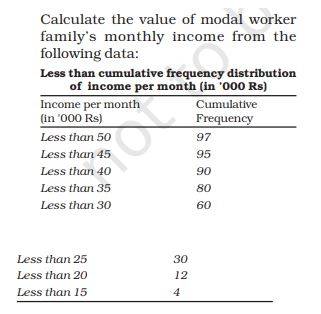
3. Calculate the value of modal worker family’s monthly income from the following data: 

Solution.
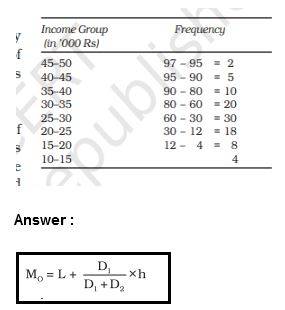
Firstly, considering that the table is a cumulative frequency distribution; we will have to convert it into a exclusive series & an ordinary frequency table - 

Hence, we derive the following from above formula - 

L = 25, D1 = (30 – 18) = 12, D2 = (30 – 20) = 10, h = 5 

= 25+12/12+10x5 

= Rs. 27.273 

Q: Which is the most suitable average for qualitative measurement? 

Q: Which measure of central tendency is affected most by extreme items of data? 

Q: State 3 advantages of using median as a measure of central tendency. 

Q: Why do we measure central tendency of a frequency distribution? 

Q: Which is considered to be the best measure of central tendency?